Free-Energy Synopsis

All of the self-gravitating configurations considered below have an associated Gibbs-like free-energy that can be expressed analytically as a power-law function of the dimensionless configuration radius, <math>~x</math>. Specifically,

<math>~\mathfrak{G}^*_\mathrm{type}</math>

<math>~-ax^{-1} + b x^{-3/n} + c x^{-3/j} + \mathfrak{G}_0 \, .</math>

Equilibrium Radii and Critical Radii

The first and second (partial) derivatives with respect to <math>~x</math> are, respectively,

<math>~\frac{\partial\mathfrak{G}^*_\mathrm{type}}{\partial x}</math>	<math>~=</math>	<math>~ax^{-2} - \biggl(\frac{ 3b}{n}\biggr) x^{-3/n -1} -\biggl(\frac{3 c}{j}\biggr) x^{-3/j-1} </math>
	<math>~=</math>	<math>~\frac{1}{x^2} \biggl[ a - \biggl(\frac{ 3b}{n}\biggr) x^{(n-3)/n } -\biggl(\frac{3 c}{j}\biggr) x^{(j-3)/j} \biggr] \, ,</math>
<math>~\frac{\partial^2 \mathfrak{G}^*_\mathrm{type}}{\partial x^2}</math>	<math>~=</math>	<math>~-2ax^{-3} + \biggl(\frac{ 3b}{n}\biggr) \biggl( \frac{n+3}{n}\biggr) x^{-3/n -2} + \biggl(\frac{3 c}{j}\biggr)\biggl( \frac{j+3}{j}\biggr) x^{-3/j-2} </math>
	<math>~=</math>	<math>~ \frac{1}{x^3} \biggl\{ \biggl(\frac{ 3b}{n}\biggr) \biggl( \frac{n+3}{n}\biggr) x^{(n-3)/n} + \biggl(\frac{3 c}{j}\biggr)\biggl( \frac{j+3}{j}\biggr) x^{(j-3)/j} -2a\biggr\} \, . </math>

Equilibrium configurations are identified by setting the first derivative to zero. This gives,

<math>~0</math>	<math>~=</math>	<math>~a - \biggl(\frac{ 3b}{n}\biggr) x^{(n-3)/n }_\mathrm{eq} -\biggl(\frac{3 c}{j}\biggr) x^{(j-3)/j}_\mathrm{eq} </math>
<math>~\Rightarrow ~~~x^{(n-3)/n }_\mathrm{eq}</math>	<math>~=</math>	<math>~\biggl(\frac{n}{ 3b}\biggr) \biggl[a -\biggl(\frac{3 c}{j}\biggr) x^{(j-3)/j}_\mathrm{eq} \biggr] \, .</math>
<math>~\Rightarrow ~~~\frac{ b}{nc}\cdot x^{(n-3)/n }_\mathrm{eq} - \frac{a}{3c} + \frac{1}{j}\cdot x^{(j-3)/j}_\mathrm{eq} </math>	<math>~=</math>	<math>~ 0 \, .</math>

We conclude, as well, that at this equilibrium radius, the second (partial) derivative assumes the value,

<math>~\biggl[ \frac{\partial^2 \mathfrak{G}^*_\mathrm{type}}{\partial x^2} \biggr]_\mathrm{eq}</math>	<math>~=</math>	<math>~ \frac{1}{x^3_\mathrm{eq} } \biggl\{ \biggl(\frac{ 3b}{n}\biggr) \biggl( \frac{n+3}{n}\biggr) x^{(n-3)/n} + \biggl(\frac{3 c}{j}\biggr)\biggl( \frac{j+3}{j}\biggr) x^{(j-3)/j} -2a\biggr\}_\mathrm{eq} </math>
	<math>~=</math>	<math>~ \frac{1}{x^3_\mathrm{eq} } \biggl\{ \biggl( \frac{n+3}{n}\biggr) \biggl[a -\biggl(\frac{3 c}{j}\biggr) x^{(j-3)/j}_\mathrm{eq} \biggr] + \biggl(\frac{3 c}{j}\biggr)\biggl( \frac{j+3}{j}\biggr) x^{(j-3)/j}_\mathrm{eq} -2a\biggr\} </math>
	<math>~=</math>	<math>~ \frac{1}{x^3_\mathrm{eq} } \biggl\{ \biggl(\frac{3 c}{j}\biggr) \biggl[ \biggl( \frac{j+3}{j}\biggr) -\biggl( \frac{n+3}{n}\biggr) \biggl] x^{(j-3)/j}_\mathrm{eq} + \biggl( \frac{3-n}{n}\biggr) a\biggr\} \, . </math>

Hence, equilibrium configurations for which the second (as well as first) derivative of the free energy is zero are found at "critical" radii given by the expression,

<math>~0</math>	<math>~=</math>	<math>~ \biggl(\frac{3 c}{j}\biggr) \biggl[ \biggl( \frac{j+3}{j}\biggr) -\biggl( \frac{n+3}{n}\biggr) \biggl] [x_\mathrm{eq}^{(j-3)/j}]_\mathrm{crit} + \biggl( \frac{3-n}{n}\biggr) a </math>
<math>~\Rightarrow ~~~[x_\mathrm{eq}^{(j-3)/j}]_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl[ \frac{j^2 a(n-3)}{3 c}\biggr] [ n(j+3) - j(n+3) ]^{-1} </math>
	<math>~=</math>	<math>~ \frac{a}{3^2c}\biggl[ \frac{j^2(n-3)}{n-j} \biggr] \, . </math>

Examples

Pressure-Truncated Polytropes

For pressure-truncated polytropes of index <math>~n</math>, we set, <math>~j = -1</math>, in which case,

<math>~\frac{ b}{nc}\cdot x^{(n-3)/n }_\mathrm{eq} - \frac{a}{3c} - x^{4}_\mathrm{eq}</math>	<math>~=</math>	<math>~0 \, ;</math>
<math>~\biggl[ \biggl(\frac{3}{4\pi}\biggr) \frac{ M_\mathrm{tot}}{M_\mathrm{SWS}} \biggr]^{(n+1)/n} x^{(n-3)/n }_\mathrm{eq} - \frac{3}{20\pi} \biggl( \frac{n+1}{n}\biggr)\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}} \biggr)^{2} - x^{4}_\mathrm{eq}</math>	<math>~=</math>	<math>~0 \, ;</math>
<math>~x^{(n-3)/n }_\mathrm{eq}</math>	<math>~=</math>	<math>~\biggl(\frac{n}{ 3b}\biggr) \biggl[a + 3cx^{4}_\mathrm{eq} \biggr] \, ;</math>
	and
<math>~[x_\mathrm{eq}]_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl[ \frac{a(n-3)}{3^2 c (n+1)} \biggr]^{1/4} \, . </math>

Case M

More specifically, the expression that describes the "Case M" free-energy surface is,

<math>~\mathfrak{G}_{K,M}^* \equiv \frac{\mathfrak{G}_{K,M}}{E_\mathrm{norm}} </math>

<math>~ -3\mathcal{A} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^{-1} +~ n\mathcal{B} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^{-3/n} +~ \biggl( \frac{4\pi}{3} \biggr) \frac{P_e}{P_\mathrm{norm}} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^3 \, . </math>

Hence, we have,

<math>~a</math>	<math>~\equiv</math>	<math>~3\mathcal{A} = \frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2}\, , </math>
<math>~b</math>	<math>~\equiv</math>	<math>~n\mathcal{B} = n\biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} \, , </math>
<math>~c</math>	<math>~\equiv</math>	<math>~\frac{4\pi}{3}\biggl( \frac{P_e}{P_\mathrm{norm}} \biggr) \, , </math>

where the structural form factors for pressure-truncated polytropes are precisely defined here. We immediately conclude that,

<math>~3c[x_\mathrm{eq}]^4_\mathrm{crit} </math>	<math>~=</math>	<math>~\frac{(n-3)}{5(n+1)} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} </math>
<math>~\Rightarrow ~~~ \biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4_\mathrm{crit}</math>	<math>~=</math>	<math>~\frac{1}{20\pi} \biggl( \frac{n-3}{n+1} \biggr) \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} \, .</math>

ASIDE: Let's see what this requires for the case of <math>~n=5</math>, where everything is specifiable analytically. We have gathered together:

Form factors from here.
Hoerdt's equilibrium expressions from here.
Conversion from Horedt's units to ours as specified here.

<math>~{\tilde\mathfrak{f}}_M</math>	<math>~=</math>	<math>~ ( 1 + \ell^2 )^{-3/2} </math>
<math>~{\tilde\mathfrak{f}}_W</math>	<math>~=</math>	<math>~ \frac{5}{2^4} \cdot \ell^{-5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr)(1 + \ell^2)^{-3} + \tan^{-1}(\ell ) \biggr] </math>
<math>~{\tilde\mathfrak{f}}_A</math>	<math>~=</math>	<math>~ \frac{3}{2^3} \ell^{-3} [ \tan^{-1}(\ell ) + \ell (\ell^2-1) (1+\ell^2)^{-2} ] </math>
<math>~\frac{R_\mathrm{eq}}{R_\mathrm{norm}} = \frac{R_\mathrm{eq}}{R_\mathrm{Horedt}} \biggl[ \frac{4\pi}{(n+1)^n} \biggr]^{1/(n-3)}</math>	<math>~=</math>	<math>~\biggl\{ 3 \biggl[ \frac{(\xi_e^2/3)^5}{(1+\xi_e^2/3)^{6}} \biggr] \biggr\}^{-1/2}\biggl[ \frac{4\pi}{(n+1)^n} \biggr]^{1/(n-3)}</math>
	<math>~=</math>	<math>~\biggl[ \frac{(1+\ell^2)^{3}}{\ell^{5}} \biggr] \biggl[ \frac{\pi}{2^3\cdot 3^6} \biggr]^{1/2}</math>
<math>~\frac{P_e}{P_\mathrm{norm}} = \frac{P_e}{P_\mathrm{Horedt}} \biggl[ \frac{(n+1)^3}{4\pi} \biggr]^{(n+1)/(n-3)}</math>	<math>~=</math>	<math>~3^3 \biggl[ \frac{(\xi_e^2/3)^3}{(1+\xi_e^2/3)^{4}} \biggr]^3 \biggl[ \frac{(n+1)^3}{4\pi} \biggr]^{(n+1)/(n-3)}</math>
	<math>~=</math>	<math>~\biggl[ \frac{\ell^{18}}{(1+\ell^2)^{12}} \biggr] \biggl[ \frac{2 \cdot 3^4}{\pi} \biggr]^{3}</math>

So, the radius of the critical equilibrium state should be,

<math>~\biggl[\frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr]^4_\mathrm{crit} </math>	<math>~=</math>	<math>~\frac{(n-3)}{3\cdot 5(n+1)} \biggl(\frac{3}{2^2\pi}\biggr) \biggl(\frac{P_e}{P_\mathrm{norm}}\biggr)^{-1}\cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} </math>
	<math>~=</math>	<math>~\frac{1}{2^2\cdot 3\cdot 5 \pi} \biggl\{\frac{(1+\ell^2)^{12}}{\ell^{18}} \biggl[ \frac{\pi}{2 \cdot 3^4} \biggr]^{3}\biggr\} (1+\ell^2)^3 \cdot \biggl\{ \frac{5}{2^4} \cdot \ell^{-5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr)(1 + \ell^2)^{-3} + \tan^{-1}(\ell ) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{\pi^2}{2^9\cdot 3^{13}} \biggl\{\frac{(1+\ell^2)^{12}}{\ell^{23}} \biggr\} \cdot \biggl\{ \biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr) + (1 + \ell^2)^{3}\tan^{-1}(\ell ) \biggr] \biggr\} \, ; </math>

whereas, each equilibrium configuration has,

<math>~\biggl[\frac{R_\mathrm{eq}}{R_\mathrm{norm}}\biggr]^4 </math>

<math>~\frac{\pi^2}{2^6\cdot 3^{12}} \biggl[ \frac{(1+\ell^2)^{12}}{\ell^{20}} \biggr] \, .</math>

So the equilibrium state that marks the critical configuration must have a value of <math>~\ell</math> that satisfies the relation,

<math>~\frac{\pi^2}{2^6\cdot 3^{12}} \biggl[ \frac{(1+\ell^2)^{12}}{\ell^{20}} \biggr] </math>	<math>~=</math>	<math>~\frac{\pi^2}{2^9\cdot 3^{13}} \biggl\{\frac{(1+\ell^2)^{12}}{\ell^{23}} \biggr\} \cdot \biggl\{ \biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr) + (1 + \ell^2)^{3}\tan^{-1}(\ell ) \biggr] \biggr\} </math>
<math>~\Rightarrow ~~~2^3\cdot 3 \ell^3</math>	<math>~=</math>	<math>~ \ell ( \ell^4 - \frac{8}{3}\ell^2 - 1 ) + (1 + \ell^2)^{3}\tan^{-1}(\ell ) </math>
<math>~\Rightarrow ~~~\biggl[ \frac{(1 + \ell^2)^{3}}{\ell} \biggr] \tan^{-1}(\ell ) </math>	<math>~=</math>	<math>~1 + \frac{80}{3}\cdot \ell^2 -\ell^4 \, . </math>

The solution is: <math>~\ell_\mathrm{crit} \approx 2.223175 \, .</math>

In addition, we know from our dissection of Hoerdt's work on detailed force-balance models that, in the equilibrium state,

<math>~\biggl(\frac{P_e}{P_\mathrm{norm}}\biggr) \biggl(\frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4</math>	<math>~=</math>	<math> \biggl[ \frac{\tilde\theta^{n+1} }{(4\pi)(n+1)( -\tilde\theta' )^{2}} \biggr] </math>
<math>~\Rightarrow ~~~ 3c x_\mathrm{eq}^4</math>	<math>~=</math>	<math> \biggl[ \frac{\tilde\theta^{n+1} }{(n+1)( -\tilde\theta' )^{2}} \biggr] \, . </math>

This means that, for any chosen polytropic index, the critical equilibrium state is the equilibrium configuration for which (needs to be checked),

<math>~2(9-2n){\tilde\theta}^{n+1}</math>

<math>~ 3(n-3)\biggl[ (- {\tilde\theta}^')^2 - \frac{\tilde\theta(-{\tilde\theta}^')}{\tilde\xi}\biggr] \, . </math>

We note, as well, that by combining the Horedt expression for <math>~x_\mathrm{eq}</math> with our virial equilibrium expression, we find (needs to be checked),

<math>~x_\mathrm{eq}^{n-3}</math>

<math>~\frac{4\pi}{3}\biggl[ \frac{3}{(n+1)\tilde\xi^2} + \frac{{\tilde\mathfrak{f}}_{W} - {\tilde\mathfrak{f}}_{M}}{5\tilde\mathfrak{f}_A} \biggr]^{n} {\tilde\mathfrak{f}}_{M}^{1-n} \, .</math>

Case P

Alternatively, let's examine the "Case P" free-energy surface. Drawing on Stahler's presentation, we adopt the following radius and mass normalizations:

<math>M_\mathrm{SWS} = \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \, ,</math>

<math> R_\mathrm{SWS} = \biggl( \frac{n+1}{n} \biggr)^{1/2} G^{-1/2} K_n^{n/(n+1)} P_\mathrm{e}^{(1-n)/[2(n+1)]} \, . </math>

In terms of these new normalizations, we have,

<math>~R_\mathrm{norm} \equiv \biggl[\biggl( \frac{G}{K} \biggr)^n M_\mathrm{tot}^{(n-1)} \biggr]^{1/(n-3)}</math>	<math>~=</math>	<math>~ \biggl( \frac{G}{K} \biggr)^{n/(n-3)} M_\mathrm{tot}^{(n-1)/(n-3)} R_\mathrm{SWS} \biggl( \frac{n+1}{n} \biggr)^{-1/2} G^{1/2} K_n^{-n/(n+1)} P_\mathrm{e}^{-(1-n)/[2(n+1)]} </math>
		<math>~+ M_\mathrm{SWS}^{-(n-1)/(n-3)} \biggl[ \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \biggr]^{(n-1)/(n-3)} </math>
	<math>~=</math>	<math>~R_\mathrm{SWS} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{[3(n-1)-(n-3)]/[2(n-3)]} G^{[2n+(n-3)-3(n-1)]/[2(n-3)]} </math>
		<math>~+ K_n^{n[2(n-1) - (n+1) - (n-3)]/[(n+1)(n-3)]} P_\mathrm{e}^{-(n-1)(3-n)/[2(n+1)(n-3)]} P_\mathrm{e}^{(n-1)(3-n)/[2(n+1)(n-3)]} </math>
	<math>~=</math>	<math>~R_\mathrm{SWS} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \, . </math>

and,

<math>~P_\mathrm{norm} \equiv \biggl[ \frac{K^{4n}}{G^{3(n+1)} M_\mathrm{tot}^{2(n+1)} } \biggr]^{1/(n-3)}</math>	<math>~=</math>	<math>~ \biggl[ \frac{K^{4n}}{G^{3(n+1)} } \biggr]^{1/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{-2(n+1)/(n-3)} \biggl\{ \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]}\biggr\}^{-2(n+1)/(n-3)} </math>
	<math>~=</math>	<math>~ \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{-2(n+1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{-3(n+1)/(n-3)} K^{4n/(n-3)} G^{-3(n+1)/(n-3)} </math>
		<math>~\times~ G^{3(n+1)/(n-3)} K_n^{-4n/(n-3)} \biggl\{ P_\mathrm{e}^{-(n-3)/[2(n+1)]}\biggr\}^{-2(n+1)/(n-3)} </math>
	<math>~=</math>	<math>~P_e \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{-2(n+1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{-3(n+1)/(n-3)} \, . </math>

Rewriting the expression for the free energy gives,

<math>~\mathfrak{G}_{K,M}^* \equiv \frac{\mathfrak{G}_{K,M}}{E_\mathrm{norm}} </math>	<math>~=</math>	<math>~ -3\mathcal{A} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-1} \biggl(\frac{R_\mathrm{norm}}{R_\mathrm{SWS}} \biggr) +~ n\mathcal{B} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-3/n} \biggl(\frac{R_\mathrm{norm}}{R_\mathrm{SWS}} \biggr)^{3/n} +~ \biggl( \frac{4\pi}{3} \biggr) \frac{P_e}{P_\mathrm{norm}} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^3 \biggl(\frac{R_\mathrm{norm}}{R_\mathrm{SWS}}\biggr)^{-3} </math>
	<math>~=</math>	<math>~ -3\mathcal{A} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-1} \biggl[ \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)}\biggr] </math>
		<math>~ +~ n\mathcal{B} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-3/n} \biggl[ \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)}\biggr]^{3/n} </math>
		<math>~ +~ \biggl( \frac{4\pi}{3} \biggr) \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{2(n+1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{3(n+1)/(n-3)} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^3 \biggl[ \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)}\biggr]^{-3} </math>
	<math>~=</math>	<math>~ -3\mathcal{A} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-1} +~ n\mathcal{B} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{3(n-1)/[n(n-3)]} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-3/n} </math>
		<math>~ +~ \biggl( \frac{4\pi}{3} \biggr) \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{(5-n)/(n-3)} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^3 \, . </math>

Therefore, in this case, we have,

<math>~a</math>	<math>~=</math>	<math>~\frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \, , </math>
<math>~b</math>	<math>~=</math>	<math>~n\biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{3(n-1)/[n(n-3)]} \, , </math>
<math>~c</math>	<math>~=</math>	<math>~\frac{4\pi}{3} \biggl( \frac{n+1}{n} \biggr)^{3/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{(5-n)/(n-3)} \, , </math>

where the structural form factors for pressure-truncated polytropes are precisely defined here. We immediately conclude that,

<math>~\biggl[\frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr]^4_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl[ \frac{(n-3)}{3^2 (n+1)} \biggr] \frac{a}{c} </math>
	<math>~=</math>	<math>~\biggl[ \frac{(n-3)}{3^2 (n+1)} \biggr] \biggl[ \frac{3}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} \biggl( \frac{n+1}{n} \biggr)^{n/(n-3)} \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{(n-1)/(n-3)} \biggr] \biggl[ \frac{3}{4\pi} \biggl( \frac{n+1}{n} \biggr)^{-3/(n-3)} \biggl( \frac{M_\mathrm{tot} }{M_\mathrm{SWS}} \biggr)^{(n-5)/(n-3)} \biggr] </math>
	<math>~=</math>	<math>~\biggl[ \frac{(n-3)}{20\pi (n+1)} \biggr] \biggl(\frac{n+1}{n}\biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} </math>

Also from Stahler's work we know that the equilibrium mass and radius are,

<math> ~\frac{M_\mathrm{tot}}{M_\mathrm{SWS}} </math>	<math>~=~</math>	<math> \biggl( \frac{n^3}{4\pi} \biggr)^{1/2} \biggl[ {\tilde\theta}_n^{(n-3)/2} {\tilde\xi}^2 (-{\tilde\theta}^') \biggr] \, , </math>
<math> ~\frac{R_\mathrm{eq}}{R_\mathrm{SWS}} </math>	<math>~=~</math>	<math> \biggl( \frac{n}{4\pi} \biggr)^{1/2} \biggl[ \tilde\xi {\tilde\theta}_n^{(n-1)/2} \biggr] \, . </math>

Additional details in support of an associated PowerPoint presentation can be found here.

Reconcile

<math>~\biggl[\frac{R_\mathrm{eq}}{R_\mathrm{SWS}} \biggr]^4_\mathrm{crit} </math>

<math>~\biggl[ \frac{(n-3)}{20\pi (n+1)} \biggr] \biggl(\frac{n+1}{n}\biggr) \biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} </math>

<math>~\biggl( \frac{R_\mathrm{eq}}{R_\mathrm{norm}} \biggr)^4_\mathrm{crit}</math>

<math>~\frac{1}{20\pi} \biggl( \frac{n-3}{n+1} \biggr) \biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)^{-1} \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2} </math>

Taking the ratio, the RHS is,

<math>~\biggl( \frac{M_\mathrm{tot}}{M_\mathrm{SWS}}\biggr)^{2} \biggl( \frac{P_e}{P_\mathrm{norm}} \biggr)</math>	<math>~=</math>	<math>~P_e M_\mathrm{tot}^2 \biggl[ \frac{G^{3(n+1)} M_\mathrm{tot}^{2(n+1)} }{K^{4n}} \biggr]^{1/(n-3)} \biggl[ \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2} K_n^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \biggr]^{-2} \biggl( \frac{n+1}{n}\biggr)</math>
	<math>~=</math>	<math>~\biggl( \frac{n+1}{n} \biggr)^{-2}P_e M_\mathrm{tot}^2 \biggl[ G^{3} M_\mathrm{tot}^{2} \biggr]^{(n+1)/(n-3)} K_n^{-4n/(n-3)} \biggl[ G^{3} K_n^{-4n/(n+1)} P_\mathrm{e}^{(n-3)/(n+1)} \biggr]</math>
	<math>~=</math>	<math>~\biggl( \frac{n+1}{n} \biggr)^{-2} \biggl[ G^{3} M_\mathrm{tot}^{2} \biggr]^{[(n-3)+(n+1)]/(n-3)} \biggl[ K_n^{[(n+1)+(n-3)]/[(n+1)(n-3)] } \biggr]^{-4n} P_\mathrm{e}^{[(n+1)+ (n-3)]/(n+1)} </math>
	<math>~=</math>	<math>~\biggl( \frac{n+1}{n} \biggr)^{-2} M_\mathrm{tot}^{4(n-1)/(n-3)} G^{[6(n-1)]/(n-3)} K_n^{-8(n-1)/[(n+1)(n-3)] } P_\mathrm{e}^{2(n-1)/(n+1)} \, ;</math>

while the LHS is,

<math>~\biggl( \frac{R_\mathrm{norm}}{R_\mathrm{SWS}} \biggr)^{4}</math>	<math>~=</math>	<math>~ \biggl[\biggl( \frac{G}{K} \biggr)^n M_\mathrm{tot}^{(n-1)} \biggr]^{4/(n-3)} \biggl\{\biggl( \frac{n+1}{n} \biggr)^{1/2} G^{-1/2} K_n^{n/(n+1)} P_\mathrm{e}^{(1-n)/[2(n+1)]}\biggr\}^{-4} </math>
	<math>~=</math>	<math>~\biggl( \frac{n+1}{n} \biggr)^{-2} M_\mathrm{tot}^{4(n-1)/(n-3)} G^{[6(n-1)]/(n-3)} K^{-8n(n-1)/[(n-3)(n+1)] } P_\mathrm{e}^{2(n-1)/(n+1)} \, . </math>

Q.E.D.

By inspection, in the specific case of <math>~n=5</math> (see above), this critical configuration appears to coincide with one of the "turning points" identified by Kimura. Specifically, it appears to coincide with the "extremal in r₁" along an M₁ sequence, which satisfies the condition,

<math>~\biggl[ \frac{n-3}{n-1} \biggr]_{n=5}</math>	<math>~=</math>	<math>~\biggl[ \frac{\tilde\xi \tilde\theta^{n}}{(-\tilde\theta^')}\biggr]_{n=5}</math>
<math>~\Rightarrow ~~~\frac{1}{2} </math>	<math>~=</math>	<math>~3^{1/2}\ell \biggl[ (1 + \ell^2)^{-1/2} \biggr]^5 \biggl[ \frac{\ell}{3^{1/2}} (1+\ell^2 )^{-3/2} \biggr]^{-1}</math>
	<math>~=</math>	<math>~3(1 + \ell^2)^{-1} </math>
<math>~\Rightarrow~~~ \ell </math>	<math>~=</math>	<math>~5^{1/2} \, .</math>

Hence, according to Kimura, the turning point associated with the configuration with the largest equilibrium radius, corresponds to the equilibrium configuration having,

<math>~\ell |_\mathrm{R_{max}} = \sqrt{5} \approx 2.2360680 \, .</math>

This is, indeed, very close to — but decidedly different from — the value of <math>~\ell_\mathrm{crit}</math> determined, above!

Five-One Bipolytropes

For analytically prescribed, "five-one" bipolytropes, <math>~n = 5</math> and <math>~j =1</math>, in which case,

<math>~x^{2/5 }_\mathrm{eq}</math>	<math>~=</math>	<math>~\biggl(\frac{5}{ 3b}\biggr) \biggl[a -3 c x^{-2}_\mathrm{eq} \biggr] \, ;</math>
	and
<math>~[x_\mathrm{eq}]_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl[ \frac{18 c}{a }\biggr]^{1/2} \, . </math>

More specifically, the expression that describes the free-energy surface is,

<math>~\mathfrak{G}^*_{51} \equiv 2^4\biggl( \frac{q}{\nu^2}\biggr) \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}_{51}}{E_\mathrm{norm}} \biggr]</math>

<math>~ \frac{1}{\ell_i^2} \biggl[ \Chi^{-3/5} (5 \mathfrak{L}_i) +\Chi^{-3} (4\mathfrak{K}_i) -\Chi^{-1} (3\mathfrak{L}_i +12\mathfrak{K}_i ) \biggr] \, . </math>

Hence, we have,

<math>~a</math>	<math>~\equiv</math>	<math>~ 3\chi_\mathrm{eq}(\mathfrak{L}_i + 4\mathfrak{K}_i) \, , </math>
<math>~b</math>	<math>~\equiv</math>	<math>~ 5 \mathfrak{L}_i \chi_\mathrm{eq}^{3/5} \, , </math>
<math>~c</math>	<math>~\equiv</math>	<math>~ 4 \mathfrak{K}_i \chi_\mathrm{eq}^{3} \, , </math>

and conclude that,

<math>~[\chi_\mathrm{eq}]_\mathrm{crit}</math>	<math>~=</math>	<math>~\biggl[ \frac{18 (4 \mathfrak{K}_i \chi_\mathrm{eq}^{3} )}{ 3\chi_\mathrm{eq}(\mathfrak{L}_i + 4\mathfrak{K}_i)} \biggr]^{1/2}_\mathrm{crit} </math>
	<math>~=</math>	<math>~[\chi_\mathrm{eq}]_\mathrm{crit}\biggl[ \frac{24 \mathfrak{K}_i }{ (\mathfrak{L}_i + 4\mathfrak{K}_i)} \biggr]^{1/2} </math>
<math>~\Rightarrow~~~\biggl[ \frac{24 \mathfrak{K}_i }{ (\mathfrak{L}_i + 4\mathfrak{K}_i)} \biggr]_\mathrm{crit}</math>	<math>~=</math>	<math>~1 </math>
<math>~\Rightarrow~~~\biggl[ \frac{\mathfrak{L}_i }{ \mathfrak{K}_i } \biggr]_\mathrm{crit}</math>	<math>~=</math>	<math>~20 \, . </math>

Also, from our detailed force balance derivations, we know that,

<math>~\chi_\mathrm{eq} \equiv \frac{ R_\mathrm{eq}}{R_\mathrm{norm}}</math>

<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} \, .</math>

Zero-Zero Bipolytropes

General Form

In this case, we retain full generality making the substitutions, <math>~n \rightarrow n_c</math> and <math>~j \rightarrow n_e</math>, to obtain,

<math>~x^{(n_c-3)/n_c }_\mathrm{eq}</math>	<math>~=</math>	<math>~\frac{n_c}{ 3b} \biggl[a -\biggl(\frac{3 c}{n_e}\biggr) x^{(n_e-3)/n_e}_\mathrm{eq} \biggr] \, ;</math>
	and
<math>~[x_\mathrm{eq}^{(n_e-3)/n_e}]_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl\{\frac{n_e^2(n_c-3)}{3[ n_c (n_e+3) - n_e(n_c+3) ]}\biggr\} \frac{a}{c} </math>
	<math>~=</math>	<math>~\biggl[\frac{n_e^2(n_c-3)}{3^2(n_c - n_e)}\biggr] \frac{a}{c} \, . </math>

And here, the expression that describes the free-energy surface is,

<math>~\mathfrak{G}^*_{00} \equiv 5 \biggl(\frac{q}{\nu^2}\biggr) \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}_{00}}{E_\mathrm{norm}} \biggr]</math>

<math>~ \frac{5}{2q^3} \biggl[ n_c A_2\Chi^{-3/n_c} + n_e B_2\Chi^{-3/n_e} - 3C_2\Chi^{-1} \biggr] \, . </math>

Hence, we have,

<math>~a \equiv 3\chi_\mathrm{eq} \biggl(\frac{5}{2q^3} \biggr) C_2 </math>	<math>~=</math>	<math> 3f \chi_\mathrm{eq} \, , </math>
<math>~b \equiv n_c \chi_\mathrm{eq}^{3/n_c} \biggl(\frac{5}{2q^3} \biggr) A_2 </math>	<math>~\equiv</math>	<math> n_c \chi_\mathrm{eq}^{3/n_c} \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \, , </math>
<math>~c \equiv n_e \chi_\mathrm{eq}^{3/n_e} \biggl(\frac{5}{2q^3} \biggr) B_2 </math>	<math>~\equiv</math>	<math>~ n_e \chi_\mathrm{eq}^{3/n_e} \biggl(\frac{5}{2q^3} \biggr) \biggl[\frac{2}{5} q^3 f - A_2\biggr] </math>
	<math>~=</math>	<math>~ n_e \chi_\mathrm{eq}^{3/n_e} \biggl\{ f - \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \biggr\} \, , </math>

where the definitions of <math>~f</math> and <math>~\mathfrak{F}</math> are given below. We immediately deduce that the critical equilibrium state is identified by,

<math>~[x_\mathrm{eq}^{(n_e-3)/n_e}]_\mathrm{crit} </math>	<math>~=</math>	<math>~\biggl\{\frac{fn_e(n_c-3)}{3(n_c - n_e)}\biggr\} [\chi_\mathrm{eq}^{(n_e-3)/n_e}]_\mathrm{crit} \biggl\{ f - \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \biggr\}^{-1} </math>
<math>~\Rightarrow ~~~\frac{1}{f}\biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr]</math>	<math>~=</math>	<math>~ 1 - \biggl[ \frac{n_e(n_c-3)}{3(n_c-n_e)} \biggr] </math>
	<math>~=</math>	<math>~ \frac{n_c(3-n_e)}{3(n_c-n_e)} \, .</math>

From our associated detailed-force-balance derivation, we know that the associated equilibrium radius is,

<math>~\chi_\mathrm{eq}</math>

<math>~ \biggl\{ \biggl(\frac{\pi}{3}\biggr) 2^{2-n_c} \nu^{n_c-1} q^{3-n_c} \biggl[1 + \frac{2}{5} q^3(f-1-\mathfrak{F})\biggr]^{n_c} \biggr\}^{1/(n_c-3)} \, . </math>

Compare with Five-One

It is worthwhile to set <math>~n_c = 5</math> and <math>~n_e = 1</math> in this expression and compare the result to the comparable expression shown above for the "Five-One" Bipolytrope. Here we have,

<math>~\biggl[\chi_\mathrm{eq}\biggr]_{51}</math>	<math>~=</math>	<math>~ \biggl\{ \biggl(\frac{\pi}{3}\biggr) 2^{-3} \nu^{4} q^{-2} \biggl[1 + \frac{2}{5} q^3(f-1-\mathfrak{F})\biggr]^{5} \biggr\}^{1/2} </math>
	<math>~=</math>	<math>~ \biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{1}{\sqrt{3}} \biggl[1 + \frac{2}{5} q^3(f-1-\mathfrak{F})\biggr]^{5/2} \, ; </math>

whereas, rewriting the above relation gives,

<math>~\chi_\mathrm{eq}\biggr|_{51}</math>

<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{1}{\sqrt{3}} \biggl[\frac{(1+\ell_i^2)^{6/5}}{3\ell_i^2}\biggr]^{5/2} \, .</math>

And, here, we should conclude that the critical equilibrium configuration is associated with,

<math>~\frac{1}{f}\biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr]</math>

Free-Energy of Truncated Polytropes

In this case, the Gibbs-like free energy is given by the sum of three separate energies,

<math>~\mathfrak{G}</math>	<math>~=</math>	<math>~W_\mathrm{grav} + \mathfrak{S}_\mathrm{therm} + P_eV</math>
	<math>~=</math>	<math>~ - 3\mathcal{A} \biggl[\frac{GM^2}{R} \biggr] + n\mathcal{B} \biggl[ \frac{KM^{(n+1)/n}}{R^{3/n}} \biggr] + \frac{4\pi}{3} \cdot P_e R^3 \, ,</math>

where the constants,

<math>~\mathcal{A} \equiv \frac{1}{5} \cdot \frac{\tilde{\mathfrak{f}}_W}{\tilde{\mathfrak{f}}_M^2}</math>

and

<math>\mathcal{B} \equiv \biggl(\frac{4\pi}{3} \biggr)^{-1/n} \frac{\tilde{\mathfrak{f}}_A}{\tilde{\mathfrak{f}}_M^{(n+1)/n}} \, ,</math>

and, as derived elsewhere,

Structural Form Factors for Pressure-Truncated Polytropes <math>~(n \ne 5)</math>

<math>~\tilde\mathfrak{f}_M</math>	<math>~=</math>	<math>~ \biggl( - \frac{3\tilde\theta^'}{\tilde\xi} \biggr) </math>
<math>\tilde\mathfrak{f}_W</math>	<math>~=</math>	<math>\frac{3\cdot 5}{(5-n)\tilde\xi^2} \biggl[\tilde\theta^{n+1} + 3 (\tilde\theta^')^2 - \tilde\mathfrak{f}_M \tilde\theta \biggr] </math>
<math>~ \tilde\mathfrak{f}_A </math>	<math>~=</math>	<math>~\frac{1}{(5-n)} \biggl\{ 6\tilde\theta^{n+1} + (n+1) \biggl[3 (\tilde\theta^')^2 - \tilde\mathfrak{f}_M \tilde\theta \biggr] \biggr\} </math>

As we have shown separately, for the singular case of <math>~n = 5</math>,

<math>~\mathfrak{f}_M</math>	<math>~=</math>	<math>~ ( 1 + \ell^2 )^{-3/2} </math>
<math>~\mathfrak{f}_W</math>	<math>~=</math>	<math>~ \frac{5}{2^4} \cdot \ell^{-5} \biggl[ \ell \biggl( \ell^4 - \frac{8}{3}\ell^2 - 1 \biggr)(1 + \ell^2)^{-3} + \tan^{-1}(\ell ) \biggr] </math>
<math>~\mathfrak{f}_A</math>	<math>~=</math>	<math>~ \frac{3}{2^3} \ell^{-3} [ \tan^{-1}(\ell ) + \ell (\ell^2-1) (1+\ell^2)^{-2} ] </math>

where, <math>~\ell \equiv \tilde\xi/\sqrt{3} </math>

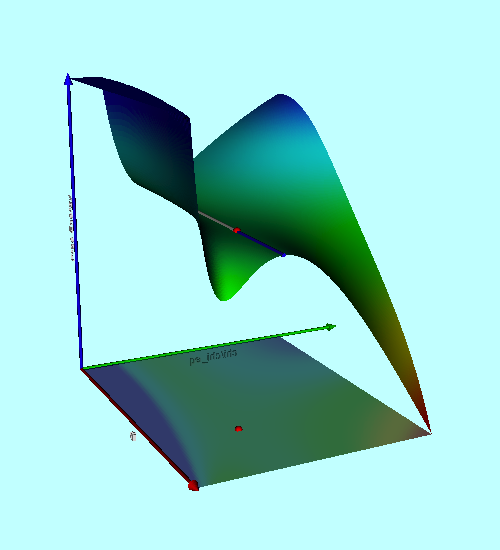
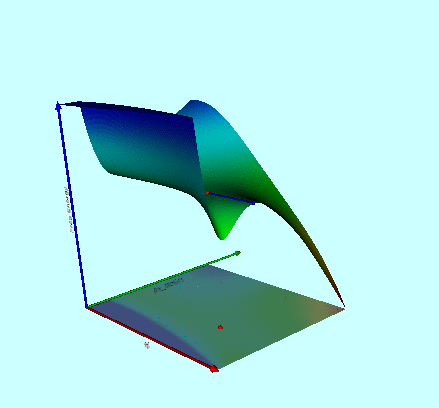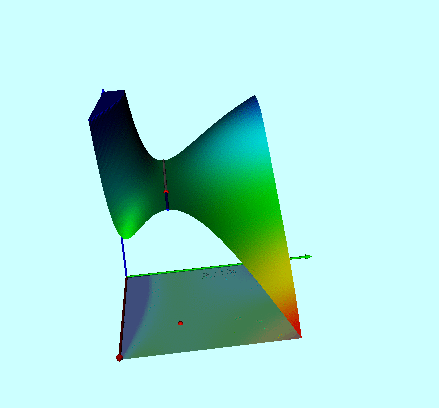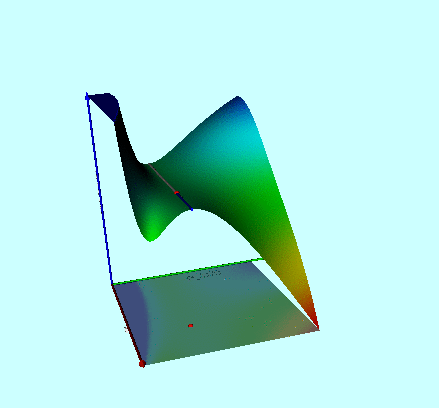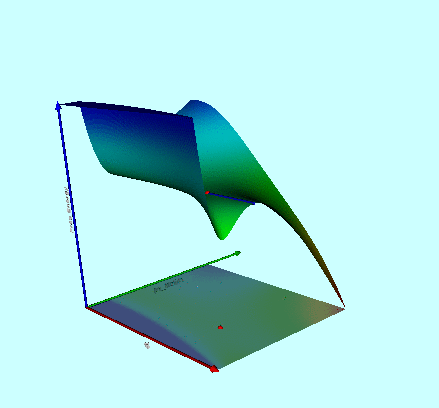
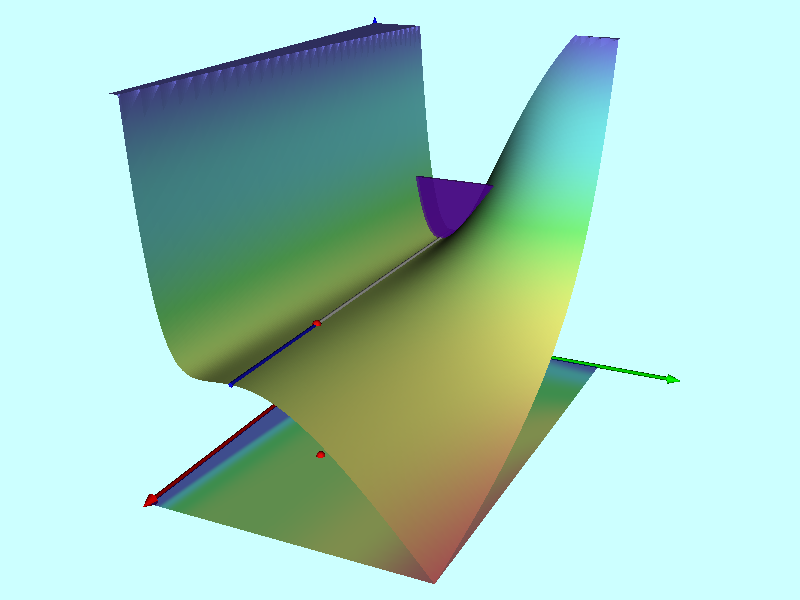
In general, then, the warped free-energy surface drapes across a four-dimensional parameter "plane" such that,

<math>~\mathfrak{G}</math>

<math>~\mathfrak{G}(R, K, M, P_e) \, .</math>

In order to effectively visualize the structure of this free-energy surface, we will reduce the parameter space from four to two, in two separate ways: First, we will hold constant the parameter pair, <math>~(K,M)</math>; giving a nod to Kimura's (1981b) nomenclature, we will refer to the resulting function, <math>~\mathfrak{G}_{K,M}(R,P_e)</math>, as a "Case M" free-energy surface because the mass is being held constant. Second, we will hold constant the parameter pair, <math>~(K,P_e)</math>, and examine the resulting "Case P" free-energy surface, <math>~\mathfrak{G}_{K,P_e}(R,M)</math>.

Case M Free-Energy Surface

It is useful to rewrite the free-energy function in terms of dimensionless parameters. Here we need to pick normalizations for energy, radius, and pressure that are expressed in terms of the gravitational constant, <math>~G</math>, and the two fixed parameters, <math>~K</math> and <math>~M</math>. We have chosen to use,

<math>~R_\mathrm{norm}</math>	<math>~\equiv</math>	<math>~\biggl[ \biggl( \frac{G}{K} \biggr)^n M_\mathrm{tot}^{n-1} \biggr]^{1/(n-3)} \, ,</math>
<math>~P_\mathrm{norm}</math>	<math>~\equiv</math>	<math>~\biggl[ \frac{K^{4n}}{G^{3(n+1)} M_\mathrm{tot}^{2(n+1)}} \biggr]^{1/(n-3)} \, ,</math>

which, as is detailed in an accompanying discussion, are similar but not identical to the normalizations used by Horedt (1970) and by Whitworth (1981). The self-consistent energy normalization is,

<math>~E_\mathrm{norm}</math>

<math>~\equiv</math>

<math>~P_\mathrm{norm} R^3_\mathrm{norm} \, .</math>

As we have demonstrated elsewhere, after implementing these normalizations, the expression that describes the "Case M" free-energy surface is,

<math> \mathfrak{G}_{K,M}^* \equiv \frac{\mathfrak{G}_{K,M}}{E_\mathrm{norm}} = -3\mathcal{A} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^{-1} +~ n\mathcal{B} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^{-3/n} +~ \biggl( \frac{4\pi}{3} \biggr) \frac{P_e}{P_\mathrm{norm}} \biggl(\frac{R}{R_\mathrm{norm}}\biggr)^3 \, , </math>

Given the polytropic index, <math>~n</math>, we expect to obtain a different "Case M" free-energy surface for each choice of the dimensionless truncation radius, <math>~\tilde\xi</math>; this choice will imply corresponding values for <math>~\tilde\theta</math> and <math>~\tilde\theta^'</math> and, hence also, corresponding (constant) values of the coefficients, <math>~\mathcal{A}</math> and <math>~\mathcal{B}</math>.

Case P Free-Energy Surface

Again, it is useful to rewrite the free-energy function in terms of dimensionless parameters. But here we need to pick normalizations for energy, radius, and mass that are expressed in terms of the gravitational constant, <math>~G</math>, and the two fixed parameters, <math>~K</math> and <math>~P_e</math>. As is detailed in an accompanying discussion, we have chosen to use the normalizations defined by Stahler (1983), namely,

<math>~R_\mathrm{SWS}</math>	<math>~\equiv</math>	<math>~\biggl( \frac{n+1}{nG} \biggr)^{1/2} K^{n/(n+1)} P_\mathrm{e}^{(1-n)/[2(n+1)]} \, ,</math>
<math>~M_\mathrm{SWS}</math>	<math>~\equiv</math>	<math>~\biggl( \frac{n+1}{nG} \biggr)^{3/2} K^{2n/(n+1)} P_\mathrm{e}^{(3-n)/[2(n+1)]} \, .</math>

The self-consistent energy normalization is,

<math>~E_\mathrm{SWS} \equiv \biggl( \frac{n}{n+1} \biggr) \frac{GM_\mathrm{SWS}^2}{R_\mathrm{SWS}}</math>

<math>~ \biggl( \frac{n+1}{n} \biggr)^{3/2} G^{-3/2}K^{3n/(n+1)} P_\mathrm{e}^{(5-n)/[2(n+1)]} \, .</math>

After implementing these normalizations — see our accompanying analysis for details — the expression that describes the "Case P" free-energy surface is,

<math>~\mathfrak{G}_{K,P_e}^* \equiv \frac{\mathfrak{G}_{K,P_e}}{E_\mathrm{SWS}}</math>

<math>~- 3 \mathcal{A} \biggl( \frac{n+1}{n} \biggr)\biggl( \frac{M}{M_\mathrm{SWS}}\biggr)^2 \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-1} + n\mathcal{B} \biggl(\frac{M}{M_\mathrm{SWS}}\biggr)^{(n+1)/n} \biggl(\frac{R}{R_\mathrm{SWS}}\biggr)^{-3/n} + \frac{4\pi}{3} \cdot \biggl( \frac{R}{R_\mathrm{SWS}}\biggr)^3 \, . </math>

Given the polytropic index, <math>~n</math>, we expect to obtain a different "Case P" free-energy surface for each choice of the dimensionless truncation radius, <math>~\tilde\xi</math>; this choice will imply corresponding values for <math>~\tilde\theta</math> and <math>~\tilde\theta^'</math> and, hence also, corresponding (constant) values of the coefficients, <math>~\mathcal{A}</math> and <math>~\mathcal{B}</math>.

Free-Energy of Bipolytropes

In this case, the Gibbs-like free energy is given by the sum of four separate energies,

<math>~\mathfrak{G}</math>

<math>~ \biggl[W_\mathrm{grav} + \mathfrak{S}_\mathrm{therm}\biggr]_\mathrm{core} + \biggl[W_\mathrm{grav} + \mathfrak{S}_\mathrm{therm}\biggr]_\mathrm{env} \, . </math>

In addition to specifying (generally) separate polytropic indexes for the core, <math>~n_c</math>, and envelope, <math>~n_e</math>, and an envelope-to-core mean molecular weight ratio, <math>~\mu_e/\mu_c</math>, we will assume that the system is fully defined via specification of the following five physical parameters:

Total mass, <math>~M_\mathrm{tot}</math>;
Total radius, <math>~R</math>;
Interface radius, <math>~R_i</math>, and associated dimensionless interface marker, <math>~q \equiv R_i/R</math>;
Core mass, <math>~M_c</math>, and associated dimensionless mass fraction, <math>~\nu \equiv M_c/M_\mathrm{tot}</math>;
Polytropic constant in the core, <math>~K_c</math>.

In general, the warped free-energy surface drapes across a five-dimensional parameter "plane" such that,

<math>~\mathfrak{G}</math>

<math>~\mathfrak{G}(R, K_c, M_\mathrm{tot}, q, \nu) \, .</math>

Order of Magnitude Derivation

Let's begin by providing very rough, approximate expressions for each of these four terms, assuming that <math>~n_c = 5</math> and <math>~n_e = 1</math>.

<math>~W_\mathrm{grav}\biggr\|_\mathrm{core}</math>	<math>~\approx</math>	<math>~- \mathfrak{a}_c \biggl[ \frac{GM_\mathrm{tot} M_c}{(R_i/2)} \biggr] = - 2\mathfrak{a}_c \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggl(\frac{\nu}{q}\biggr) \biggr] \, ;</math>
<math>~W_\mathrm{grav}\biggr\|_\mathrm{env}</math>	<math>~\approx</math>	<math>~- \mathfrak{a}_e \biggl[ \frac{GM_\mathrm{tot} M_e}{(R_i+R)/2} \biggr] = - 2\mathfrak{a}_e \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggl(\frac{1-\nu}{1+q}\biggr) \biggr] \, ;</math>
<math>~\mathfrak{S}_\mathrm{therm}\biggr\|_\mathrm{core} = U_\mathrm{int}\biggr\|_\mathrm{core} </math>	<math>~\approx</math>	<math>~\mathfrak{b}_c \cdot n_cK_c M_c ({\bar\rho}_c)^{1/n_c} = 5\mathfrak{b}_c \cdot K_c M_\mathrm{tot}\nu \biggl[ \frac{3M_c}{4\pi R_i^3} \biggr]^{1/5} </math>
	<math>~=</math>	<math>~\mathfrak{b}_c \biggl( \frac{3\cdot 5^5}{2^2\pi} \biggr)^{1/5} K_c (M_\mathrm{tot}\nu)^{6/5} (Rq)^{-3/5} \, ;</math>
<math>~\mathfrak{S}_\mathrm{therm}\biggr\|_\mathrm{env} = U_\mathrm{int}\biggr\|_\mathrm{env} </math>	<math>~\approx</math>	<math>~\mathfrak{b}_e \cdot n_eK_e M_\mathrm{env} ({\bar\rho}_e)^{1/n_e} = \mathfrak{b}_e \cdot K_e M_\mathrm{tot}(1-\nu) \biggl[ \frac{3M_\mathrm{env}}{4\pi (R^3-R_i^3)} \biggr] </math>
	<math>~=</math>	<math>~ \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr) K_e [M_\mathrm{tot}(1-\nu)]^2 [R^3(1-q^3)]^{-1} \, . </math>

In writing this last expression, it has been necessary to (temporarily) introduce a sixth physical parameter, namely, the polytropic constant that characterizes the envelope material, <math>~K_e</math>. But this constant can be expressed in terms of <math>~K_c</math> via a relation that ensures continuity of pressure across the interface while taking into account the drop in mean molecular weight across the interface, that is,

<math>~K_e ({\bar\rho}_e)^{(n_e+1)/n_e}</math>	<math>~\approx</math>	<math>~K_c ({\bar\rho}_c)^{(n_c+1)/n_c}</math>
<math>~\Rightarrow ~~~~ K_e \biggl[\biggl( \frac{\mu_e}{\mu_c} \biggr) {\bar\rho}_c\biggr]^{2}</math>	<math>~\approx</math>	<math>~K_c ({\bar\rho}_c)^{6/5}</math>
<math>~\Rightarrow ~~~~ \frac{K_e}{K_c} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{2}</math>	<math>~\approx</math>	<math>~\biggl[ \frac{3M_\mathrm{tot}\nu}{4\pi (Rq)^3} \biggr]^{-4/5} \, .</math>

Hence, the fourth energy term may be rewritten in the form,

<math>~\mathfrak{S}_\mathrm{therm}\biggr\|_\mathrm{env} = U_\mathrm{int}\biggr\|_\mathrm{env} </math>	<math>~\approx</math>	<math>~ \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr) \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} K_c\biggl[ \frac{3M_\mathrm{tot}\nu}{4\pi (Rq)^3} \biggr]^{-4/5} [M_\mathrm{tot}(1-\nu)]^2 [R^3(1-q^3)]^{-1} </math>
	<math>~=</math>	<math>~ \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr)^{1/5} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} K_c M_\mathrm{tot}^{6/5}R^{-3/5}\biggl[ \frac{q^3}{\nu} \biggr]^{4/5} \frac{(1-\nu)^2}{(1-q^3)} \, . </math>

Putting all the terms together gives,

<math>~\mathfrak{G}</math>	<math>~\approx</math>	<math>~ - 2\mathfrak{a}_c \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggl(\frac{\nu}{q}\biggr) \biggr] - 2\mathfrak{a}_e \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggl(\frac{1-\nu}{1+q}\biggr) \biggr] + \mathfrak{b}_c \biggl( \frac{3\cdot 5^5}{2^2\pi} \biggr)^{1/5} K_c (M_\mathrm{tot}\nu)^{6/5} (Rq)^{-3/5} </math>
		<math>~ + \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr)^{1/5} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} K_c M_\mathrm{tot}^{6/5}R^{-3/5}\biggl[ \frac{q^3}{\nu} \biggr]^{4/5} \frac{(1-\nu)^2}{(1-q^3)} </math>
	<math>~=</math>	<math>~ - 2 \mathcal{A}_\mathrm{biP} \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggr] + \mathcal{B}_\mathrm{biP} K_c \biggl[\frac{(\nu M_\mathrm{tot})^{2}}{ qR} \biggr]^{3/5} </math>
<math>~\Rightarrow ~~~~ \frac{\mathfrak{G}}{E_\mathrm{norm}}</math>	<math>~=</math>	<math>~ - 2 \mathcal{A}_\mathrm{biP} \biggl[ \frac{GM_\mathrm{tot}^2 }{R} \biggr] \biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} + \mathcal{B}_\mathrm{biP} \biggl(\frac{\nu^2}{q}\biggr)^{3/5} K_c \biggl[\frac{M_\mathrm{tot}^{2}}{ R} \biggr]^{3/5}\biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} </math>
	<math>~=</math>	<math>~ - 2 \mathcal{A}_\mathrm{biP} \biggl[ \frac{R_\mathrm{norm}}{R} \biggr] + \mathcal{B}_\mathrm{biP} \biggl(\frac{\nu^2}{q}\biggr)^{3/5} \biggl[\frac{R_\mathrm{norm}}{ R} \biggr]^{3/5} \, , </math>

where,

<math>~\mathcal{A}_\mathrm{biP}</math>	<math>~\equiv</math>	<math>~\biggl[ \mathfrak{a}_c\biggl(\frac{\nu}{q}\biggr) + \mathfrak{a}_e \biggl(\frac{1-\nu}{1+q}\biggr) \biggr] \, ,</math>
<math>~\mathcal{B}_\mathrm{biP}</math>	<math>~\equiv</math>	<math>~\biggl( \frac{3}{2^2\pi} \biggr)^{1/5} \biggl[5\mathfrak{b}_c + \mathfrak{b}_e \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \frac{q^3(1-\nu)^2}{\nu^2(1-q^3)} \biggr] \, .</math>

Equilibrium Radius

Order of Magnitude Estimate

This means that,

<math>~\frac{\partial\mathfrak{G}}{\partial R}</math>

<math>~ + 2 \mathcal{A}_\mathrm{biP}\biggl[ \frac{GM_\mathrm{tot}^2 }{R^2} \biggr] - \frac{3}{5} \mathcal{B}_\mathrm{biP} K_c \biggl[\frac{\nu^{2}}{ q} \biggr]^{3/5} M_\mathrm{tot}^{6/5} R^{-8/5} \, . </math>

Hence, because equilibrium radii are identified by setting <math>~\partial\mathfrak{G}/\partial R = 0</math>, we have,

<math>~\frac{R_\mathrm{eq}}{R_\mathrm{norm}}</math>

<math>~\biggl(\frac{2\cdot 5}{3}\biggr)^{5/2} \biggl[\frac{\mathcal{A}_\mathrm{biP} }{\mathcal{B}_\mathrm{biP}}\biggr]^{5/2} \biggl(\frac{ q} {\nu^{2}}\biggr)^{3/2} \, . </math>

Reconcile With Known Analytic Expression

From our earlier derivations, it appears as though,

<math>~\chi_\mathrm{eq} \equiv \frac{R_\mathrm{eq}}{R_\mathrm{norm}}</math>	<math>~=</math>	<math>~\biggl( \frac{3^8}{2^5\pi} \biggr)^{-1/2} \biggl(\frac{3}{2^4}\biggr) \biggl( \frac{q}{\ell_i}\biggr)^{5}\biggl(\frac{\nu}{q^3} \biggr)^2 \biggl( 1 + \ell_i^2 \biggr)^{3} </math>
	<math>~=</math>	<math>~\biggl(\frac{2\cdot 5}{3}\biggr)^{5/2} \biggl(\frac{q}{\nu^2} \biggr)^{3/2} \biggl[\biggl( \frac{\pi}{2^8 \cdot 3 \cdot 5^5} \biggr)^{1/2} \biggl(\frac{\nu^2}{q} \biggr)^{5/2} \frac{(1 + \ell_i^2)^3}{\ell_i^5} \biggr] \, . </math>

This implies that,

<math>~\frac{\mathcal{A}_\mathrm{biP} }{\mathcal{B}_\mathrm{biP}}</math>	<math>~\approx</math>	<math>~ \biggl[\biggl( \frac{\pi}{2^8 \cdot 3 \cdot 5^5} \biggr)^{1/2} \biggl(\frac{\nu^2}{q} \biggr)^{5/2} \frac{(1 + \ell_i^2)^3}{\ell_i^5} \biggr]^{2/5} </math>
	<math>~=</math>	<math>~\biggl(\frac{\nu^2}{q} \biggr) \biggl( \frac{\pi}{2^8 \cdot 3 \cdot 5^5} \biggr)^{1/5} \frac{(1 + \ell_i^2)^{6/5}}{\ell_i^2} </math>
<math>~\Rightarrow ~~~~ \biggl[ \mathfrak{a}_c\biggl(\frac{\nu}{q}\biggr) + \mathfrak{a}_e \biggl(\frac{1-\nu}{1+q}\biggr) \biggr] </math>	<math>~\approx</math>	<math>~\frac{1}{2^2\cdot 5}\biggl(\frac{\nu^2}{q} \biggr) \frac{(1 + \ell_i^2)^{6/5}}{\ell_i^2} \biggl[5\mathfrak{b}_c + \mathfrak{b}_e \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \frac{q^3(1-\nu)^2}{\nu^2(1-q^3)} \biggr] </math>
<math>~\Rightarrow ~~~~ \biggl[ \mathfrak{a}_c + \mathfrak{a}_e \cdot \frac{q(1-\nu)}{\nu(1+q)} \biggr] </math>	<math>~\approx</math>	<math>~\frac{\nu}{2^2\cdot 5} \frac{(1 + \ell_i^2)^{6/5}}{\ell_i^2} \biggl[5\mathfrak{b}_c + \mathfrak{b}_e \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \frac{q^3(1-\nu)^2}{\nu^2(1-q^3)} \biggr] </math>

Focus on Five-One Free-Energy Expression

Approximate Expressions

Let's plug this equilibrium radius back into each term of the free-energy expression.

<math>~\frac{W_\mathrm{grav}}{E_\mathrm{norm}}\biggr\|_\mathrm{core}</math>	<math>~\approx</math>	<math>~- 2\mathfrak{a}_c \biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} \biggl[ \frac{GM_\mathrm{tot}^2 }{R_\mathrm{eq}} \biggl(\frac{\nu}{q}\biggr) \biggr] </math>
	<math>~=</math>	<math>~- 2\mathfrak{a}_c \biggl(\frac{\nu}{q}\biggr) \biggl[ \frac{R_\mathrm{norm} }{R_\mathrm{eq}} \biggr] \, ;</math>
<math>~\frac{W_\mathrm{grav}}{E_\mathrm{norm}}\biggr\|_\mathrm{env}</math>	<math>~\approx</math>	<math>~- 2\mathfrak{a}_e \biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} \biggl[ \frac{GM_\mathrm{tot}^2 }{R_\mathrm{eq}} \biggl(\frac{1-\nu}{1+q}\biggr) \biggr] </math>
	<math>~=</math>	<math>~- 2\mathfrak{a}_e \biggl(\frac{1-\nu}{1+q}\biggr) \biggl[ \frac{R_\mathrm{norm} }{R_\mathrm{eq}} \biggr] \, ;</math>
<math>~\frac{S_\mathrm{core}}{E_\mathrm{norm}} = \biggl[\frac{3(\gamma_c-1)}{2}\biggr] \frac{U_\mathrm{int}}{E_\mathrm{norm}}\biggr\|_\mathrm{core} </math>	<math>~\approx</math>	<math>~\biggl[\frac{3}{2\cdot 5}\biggr]\mathfrak{b}_c \biggl( \frac{3\cdot 5^5}{2^2\pi} \biggr)^{1/5} \biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} K_c (M_\mathrm{tot}\nu)^{6/5} (R_\mathrm{eq}q)^{-3/5} </math>
	<math>~=</math>	<math>~ \biggl[\frac{3}{2\cdot 5}\biggr]\mathfrak{b}_c \biggl( \frac{3\cdot 5^5}{2^2\pi} \biggr)^{1/5} \biggl(\frac{\nu^2}{q}\biggr)^{3/5} \biggl(\frac{R_\mathrm{norm}}{R_\mathrm{eq}}\biggr)^{3/5} \, ;</math>
<math>~\frac{S_\mathrm{env}}{E_\mathrm{norm}} = \biggl[\frac{3(\gamma_e-1)}{2}\biggr] \frac{U_\mathrm{int}}{E_\mathrm{norm}}\biggr\|_\mathrm{env} </math>	<math>~\approx</math>	<math>~\biggl[\frac{3}{2}\biggr] \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr)^{1/5} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl(\frac{G^3}{K_c^5}\biggr)^{1/2} K_c M_\mathrm{tot}^{6/5}R_\mathrm{eq}^{-3/5}\biggl[ \frac{q^3}{\nu} \biggr]^{4/5} \frac{(1-\nu)^2}{(1-q^3)} </math>
	<math>~=</math>	<math>~\biggl[\frac{3}{2}\biggr] \mathfrak{b}_e \biggl( \frac{3}{2^2\pi } \biggr)^{1/5} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl[ \frac{q^3}{\nu} \biggr]^{4/5} \frac{(1-\nu)^2}{(1-q^3)} \biggl(\frac{R_\mathrm{norm}}{R_\mathrm{eq}}\biggr)^{3/5} \, . </math>

From Detailed Force-Balance Models

In the following derivations, we will use the expression,

<math>~\chi_\mathrm{eq} \equiv \frac{ R_\mathrm{eq}}{R_\mathrm{norm}}</math>

<math>~\biggl( \frac{\mu_e}{\mu_c} \biggr)^3 \biggl( \frac{\pi}{2^3} \biggr)^{1/2} \frac{1}{A^2\eta_s} = \biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} \, .</math>

Keep in mind, as well — as derived in an accompanying discussion — that,

<math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}} </math>

<math>~ (m_3^2 \ell_i^3) (1 + \ell_i^2)^{-1/2} [1 + (1-m_3)^2 \ell_i^2]^{-1/2} \biggl[ m_3\ell_i + (1+\ell_i^2) \biggl(\frac{\pi}{2} + \tan^{-1} \Lambda_i \biggr) \biggr]^{-1} \, ,</math>

where,

<math>m_3 \equiv 3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \, .</math>

From the accompanying Table 1 parameter values, we also can write,

<math>~q</math>	<math>~=</math>	<math>~\frac{\eta_i}{\eta_s} = \eta_i \biggl\{\frac{\pi}{2} + \eta_i + \tan^{-1}\biggl[ \frac{1}{\eta_i} - \ell_i \biggr] \biggr\}^{-1}</math>
	<math>~=</math>	<math>~ \eta_i \biggl\{\eta_i + \cot^{-1}\biggl[ \ell_i - \frac{1}{\eta_i} \biggr] \biggr\}^{-1} \, , </math>

where,

<math>~m_3 \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] \, .</math>

Let's also define the following shorthand notation:

<math>~\mathfrak{L}_i</math>	<math>~\equiv</math>	<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i \, ;</math>
<math>~\mathfrak{K}_i</math>	<math>~\equiv</math>	<math>~\frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] + \frac{\Lambda_i}{\eta_i} \, .</math>

Gravitational Potential Energy of the Core

Pulling from our detailed derivations,

<math>~\biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ - \biggl( \frac{3^8}{2^5\pi } \biggr)^{1/2} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) (1 + \ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr] \, .</math>
<math>~\Rightarrow ~~~~ -\chi_\mathrm{eq} \biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ \biggl( \frac{3^8}{2^5\pi } \biggr)^{1/2} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) (1 + \ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr] \biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} </math>
	<math>~=~</math>	<math>~ \biggl( \frac{3}{2^4} \biggr) \frac{\nu^2}{q} \cdot \frac{1}{\ell_i^5} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) + (1 + \ell_i^2)^{3}\tan^{-1}(\ell_i) \biggr] </math>

Out of equilibrium, then, we should expect,

<math>~\frac{W_\mathrm{core}}{E_\mathrm{norm}} </math>	<math>~=~</math>	<math>~ - \chi^{-1} \biggl( \frac{3}{2^4} \biggr) \frac{\nu^2}{q} \cdot \frac{1}{\ell_i^5} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) + (1 + \ell_i^2)^{3}\tan^{-1}(\ell_i) \biggr] </math>
	<math>~=~</math>	<math>~ - \chi^{-1} \biggl( \frac{3}{2^4} \biggr) \frac{\nu^2}{q} \cdot \frac{1}{\ell_i^2} \biggl[ \mathfrak{L}_i - \frac{8}{3} \biggr] \, , </math>

which, in comparison with our above approximate expression, implies,

<math>~\mathfrak{a}_c </math>

<math>~ \biggl( \frac{3}{2^5} \biggr) \frac{\nu}{\ell_i^5} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) + (1 + \ell_i^2)^{3}\tan^{-1}(\ell_i) \biggr] \, . </math>

Thermal Energy of the Core

Again, pulling from our detailed derivations,

<math>~\biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ \frac{1}{2} \biggl( \frac{3^8}{2^5\pi} \biggr)^{1/2} \biggl[ \ell_i (\ell_i^4 - 1 )(1+\ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr] </math>
<math>~\Rightarrow ~~~~ \chi_\mathrm{eq}^{3} \biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]^5_\mathrm{eq}</math>	<math>~=~</math>	<math>~ \frac{1}{2^5} \biggl( \frac{3^8}{2^5\pi} \biggr)^{5/2} \biggl[ \ell_i (\ell_i^4 - 1 )(1+\ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr]^5 \biggl[\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5}\biggr]^{3} </math>
	<math>~=~</math>	<math>~ \frac{1}{\pi}\biggl(\frac{3}{2^{2}}\biggr)^{11} \biggl(\frac{\nu^2}{q}\biggr)^{3} \biggl[ \ell_i (\ell_i^4 - 1 )(1+\ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr]^5 \biggl[\frac{(1+\ell_i^2)^9}{\ell_i^{15}}\biggr] \, . </math>

Out of equilibrium, we should then expect,

<math>~\frac{S_\mathrm{core}}{E_\mathrm{norm}}</math>

<math>~ \biggl(\frac{3}{2^2\pi} \biggr)^{1/5}\biggl[ \chi^{-1} \biggl(\frac{\nu^2}{q}\biggr) \frac{1}{(1+\ell_i^2)^{2}} \biggr]^{3/5} \biggl(\frac{3}{2^{2}}\biggr)^{2}\mathfrak{L}_i \, . </math>

In comparison with our above approximate expression, we therefore have,

<math>~ \biggl[ \biggl(\frac{3}{2\cdot 5}\biggr)\mathfrak{b}_c \biggl( \frac{3\cdot 5^5}{2^2\pi} \biggr)^{1/5} \biggl(\frac{\nu^2}{q}\biggr)^{3/5} \biggr]^5</math>	<math>~=~</math>	<math>~ \frac{1}{\pi}\biggl(\frac{3}{2^{2}}\biggr)^{11} \biggl(\frac{\nu^2}{q}\biggr)^{3} \biggl[ \ell_i (\ell_i^4 - 1 )(1+\ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr]^5 \biggl[\frac{(1+\ell_i^2)^9}{\ell_i^{15}}\biggr] </math>
<math>~\Rightarrow~~~~ \mathfrak{b}_c </math>	<math>~=~</math>	<math>~\frac{ 3 }{2^3\ell_i^{3}(1+\ell_i^2)^{6/5}} \biggl[ \ell_i (\ell_i^4 - 1 ) + (1+\ell_i^2)^{3}\tan^{-1}(\ell_i) \biggr] \, . </math>

Gravitational Potential Energy of the Envelope

Again, pulling from our detailed derivations and appreciating, in particular, that (see, for example, our notes on equilibrium conditions),

<math>~A</math>	<math>~=~</math>	<math>~\frac{\eta_i}{\sin(\eta_i - B)} \, ,</math>
<math>~(\eta_s - B)</math>	<math>~=~</math>	<math>~\pi \, ,</math>
<math>~\eta_i - B</math>	<math>~=~</math>	<math>~\frac{\pi}{2} - \tan^{-1}(\Lambda_i)\, ,</math>

<math>~\Rightarrow ~~~ \sin(\eta_i -B) = (1+\Lambda_i^2)^{-1/2}</math>

and

<math>~\sin[2(\eta_i-B)] = 2\Lambda_i(1 + \Lambda_i^2)^{-1} \ ,</math>

we have,

<math>~\biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ -\biggl( \frac{1}{2^3\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl\{ \biggl[6(\eta_s-B) - 3\sin[2(\eta_s - B)] -4\eta_s\sin^2(\eta_s-B) + 4B\biggr] </math>
		<math>~ - \biggl[6(\eta_i-B) - 3\sin[2(\eta_i - B)] -4\eta_i\sin^2(\eta_i-B) + 4B \biggr]\biggr\} </math>
	<math>~=~</math>	<math>~ -\biggl( \frac{1}{2^3\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl[\frac{\eta_i}{\sin(\eta_i - B)} \biggr]^2 \biggl\{ 6\pi - \biggl[6(\eta_i-B) - 3\sin[2(\eta_i - B)] -4\eta_i\sin^2(\eta_i-B) \biggr]\biggr\} </math>
	<math>~=~</math>	<math>~ -\biggl( \frac{1}{2^3\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2(1+\Lambda_i^2) \biggl\{ 6\pi - 6\biggl[\frac{\pi}{2} - \tan^{-1}(\Lambda_i)\biggr] + 6\biggl[ \frac{\Lambda_i}{(1 + \Lambda_i^2)} \biggr] + 4\eta_i \biggl[ \frac{1}{(1+\Lambda_i^2)} \biggr] \biggr\} </math>
	<math>~=~</math>	<math>~ -\biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1+\Lambda_i^2)\biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \Lambda_i + \frac{2}{3} \cdot \eta_i \biggr\} \, . </math>

So, in equilibrium we can write,

<math>~-\chi_\mathrm{eq}\biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ \biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1+\Lambda_i^2)\biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \Lambda_i + \frac{2}{3} \cdot \eta_i \biggr\} \biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} </math>
	<math>~=~</math>	<math>~ \frac{3}{2^2} \biggl(\frac{\eta_i}{m_3}\biggr)^3 \biggl\{ \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \frac{\Lambda_i}{\eta_i} + \frac{2}{3} \biggr\} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{\ell_i^5} </math>
	<math>~=~</math>	<math>~ \frac{3}{2^2} \biggl(\frac{\nu^2}{q} \biggr) \frac{1}{\ell_i^2} \biggl\{ \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \frac{\Lambda_i}{\eta_i} + \frac{2}{3} \biggr\} \, . </math>

And out of equilibrium,

<math>~\frac{W_\mathrm{env}}{E_\mathrm{norm}}</math>

<math>~ -\chi^{-1}\cdot \frac{3}{2^2} \biggl(\frac{\nu^2}{q} \biggr) \frac{1}{\ell_i^2} \biggl[\mathfrak{K}_i+ \frac{2}{3} \biggr] \, . </math>

This, in turn, implies that both in and out of equilibrium,

<math>~\mathfrak{a}_e </math>

<math>~ \frac{3}{2^3} \biggl[\frac{\nu^2(1+q)}{q(1-\nu)} \biggr] \frac{1}{\ell_i^2} \biggl\{ \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \frac{\Lambda_i}{\eta_i} + \frac{2}{3} \biggr\} \, . </math>

Thermal Energy of the Envelope

Again, pulling from our detailed derivations,

<math>~\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ ~ \biggl( \frac{1}{2^5\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl\{ \biggl[6(\eta_s - B) - 3\sin[2(\eta_s-B)] \biggr] - \biggl[6(\eta_i - B) - 3\sin[2(\eta_i-B)] \biggr] \biggr\}</math>
	<math>~=~</math>	<math>~ ~ \biggl( \frac{1}{2^5\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl[\frac{\eta_i}{\sin(\eta_i - B)} \biggr]^2 \biggl\{ 6\pi - 6(\eta_i - B) + 3\sin[2(\eta_i-B)] \biggr\}</math>
	<math>~=~</math>	<math>~ ~ \biggl( \frac{1}{2^5\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 (1 + \Lambda_i^2) \biggl\{ 6\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + 6\biggl[\Lambda_i(1 + \Lambda_i^2)^{-1} \biggr] \biggr\}</math>
	<math>~=~</math>	<math>~ ~\frac{1}{2} \biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1 + \Lambda_i^2)\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + \Lambda_i \biggr\} \, .</math>

So, in equilibrium we can write,

<math>~\chi_\mathrm{eq}^{3}\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ ~\frac{1}{2} \biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1 + \Lambda_i^2)\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + \Lambda_i \biggr\} \biggl[\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5}\biggr]^{3} </math>
	<math>~=~</math>	<math>~ ~\biggl(\frac{\nu^2}{q} \biggr)^3 \biggl( \frac{3^2\pi^2}{2^{12}} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^3 \biggl\{ \frac{(1 + \Lambda_i^2)}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + \frac{\Lambda_i}{\eta_i} \biggr\} \biggl[\frac{(1+\ell_i^2)^9}{3^9\ell_i^{15}}\biggr] </math>
	<math>~=~</math>	<math>~ ~\biggl(\frac{\nu^2}{q} \biggr)^3 \biggl( \frac{\pi}{2^{6}\cdot 3^5} \biggr) \biggl[\frac{(1+\ell_i^2)^6}{\ell_i^{12}}\biggr] \biggl\{ \frac{(1 + \Lambda_i^2)}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + \frac{\Lambda_i}{\eta_i} \biggr\} \, . </math>

And, out of equilibrium,

<math>~\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>

<math>~ ~ \chi^{-3}\biggl(\frac{\nu^2}{q} \biggr)^3 \biggl( \frac{\pi}{2^{6}\cdot 3^5} \biggr) \biggl[\frac{(1+\ell_i^2)^6}{\ell_i^{12}}\biggr]\mathfrak{K} \, . </math>

Combined in Equilibrium

Notice that, in combination,

<math>~\biggl[\frac{2S_\mathrm{env} + W_\mathrm{env}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=</math>	<math>~ - \frac{2}{3}\biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^3 </math>
	<math>~=</math>	<math>~ - \frac{2}{3}\biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl[3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \ell_i \biggl( 1 + \ell_i^2 \biggr)^{-1}\biggr]^3 </math>
	<math>~=</math>	<math>~ - \biggl( \frac{2\cdot 3^6}{\pi} \biggr)^{1/2} \biggl[\frac{\ell_i^3}{( 1 + \ell_i^2)^3}\biggr] \, . </math>

Also, from above,

<math>~\biggl[ \frac{2S_\mathrm{core}+W_\mathrm{core}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ - \biggl( \frac{3^8}{2^5\pi } \biggr)^{1/2} \biggl[ \ell_i \biggl(- \frac{8}{3} \ell_i^2 \biggr) (1 + \ell_i^2)^{-3} \biggr] </math>
	<math>~=~</math>	<math>~ + \biggl( \frac{2\cdot 3^6}{\pi } \biggr)^{1/2} \biggl[ \frac{\ell_i^3}{(1 + \ell_i^2)^{3}} \biggr] \, .</math>

So, in equilibrium, these terms from the core and envelope sum to zero, as they should.

Out of Equilibrium

And now, in combination out of equilibrium,

<math>~\frac{\mathfrak{G}}{E_\mathrm{norm}} </math>

<math>~ \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-1} \biggl\{ \biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} + \biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}\biggr\} +\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-3/5} \biggl(\frac{2n_c}{3}\biggr) \biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} +\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-3} \biggl(\frac{2n_e}{3}\biggr)\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} \, . </math>

Hence, quite generally out of equilibrium,

<math>~\frac{\partial}{\partial \chi} \biggl[ \frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr] </math>

<math>~ -\chi^{-1}\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-1} \biggl\{ \biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} + \biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}\biggr\} -\frac{3}{5}\chi^{-1}\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-3/5} \biggl(\frac{10}{3}\biggr) \biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} -3\chi^{-1}\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{-3} \biggl(\frac{2}{3}\biggr)\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} \, . </math>

Let's see what the value of this derivative is if the dimensionless radius, <math>~\chi</math>, is set to the value that has been determined, via a detailed force-balanced analysis, to be the equilibrium radius, namely, <math>~\chi = \chi_\mathrm{eq}</math>. In this case, we have,

<math>~\biggl\{\frac{\partial}{\partial \chi} \biggl[ \frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr] \biggr\}_\mathrm{\chi \rightarrow \chi_\mathrm{eq}}</math>

<math>~ -\chi_\mathrm{eq}^{-1}\biggl\{ \biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} + \biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} +2\biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} +2\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq} \biggr\} \, . </math>

But, according to the virial theorem — and, as we have just demonstrated — the four terms inside the curly braces sum to zero. So this demonstrates that the derivative of our out-of-equilibrium free-energy expression does go to zero at the equilibrium radius, as it should!

Summary51

In summary, the desired out of equilibrium free-energy expression is,

<math>~\frac{\mathfrak{G}}{E_\mathrm{norm}} </math>	<math>~=</math>	<math>~ \frac{W_\mathrm{core}}{E_\mathrm{norm}} + \frac{W_\mathrm{env}}{E_\mathrm{norm}} +\biggl(\frac{2n_c}{3}\biggr)\frac{S_\mathrm{core}}{E_\mathrm{norm}} +\biggl(\frac{2n_e}{3}\biggr)\frac{S_\mathrm{env}}{E_\mathrm{norm}} </math>
	<math>~=</math>	<math>~ - \chi^{-1} \biggl( \frac{3}{2^4} \biggr) \frac{\nu^2}{q} \cdot \frac{1}{\ell_i^2} \biggl[ \mathfrak{L}_i - \frac{8}{3} \biggr] -\chi^{-1}\cdot \frac{3}{2^2} \biggl(\frac{\nu^2}{q} \biggr) \frac{1}{\ell_i^2} \biggl[\mathfrak{K}_i+ \frac{2}{3} \biggr] </math>
		<math>~ + \biggl(\frac{2\cdot 5}{3}\biggr) \biggl(\frac{3}{2^2\pi} \biggr)^{1/5}\biggl[ \chi^{-1} \biggl(\frac{\nu^2}{q}\biggr) \frac{1}{(1+\ell_i^2)^{2}} \biggr]^{3/5} \biggl(\frac{3}{2^{2}}\biggr)^{2}\mathfrak{L}_i +\biggl(\frac{2}{3}\biggr) \chi^{-3}\biggl(\frac{\nu^2}{q} \biggr)^3 \biggl( \frac{\pi}{2^{6}\cdot 3^5} \biggr) \biggl[\frac{(1+\ell_i^2)^6}{\ell_i^{12}}\biggr]\mathfrak{K} </math>
	<math>~=</math>	<math>~ - \biggl( \frac{3}{2^4} \biggr) \biggl[\chi^{-1}\frac{\nu^2}{q} \cdot \frac{1}{\ell_i^2}\biggr] \biggl[ \mathfrak{L}_i + 4\mathfrak{K}_i \biggr] + \biggl(\frac{3}{2^2\pi} \biggr)^{1/5}\biggl(\frac{3\cdot 5}{2^3}\biggr) \biggl[ \chi^{-1} \biggl(\frac{\nu^2}{q}\biggr) \frac{1}{(1+\ell_i^2)^{2}} \biggr]^{3/5} \mathfrak{L}_i </math>
		<math>~ + \biggl( \frac{\pi}{2^{5}\cdot 3^6} \biggr) \biggl[\chi^{-1}\biggl(\frac{\nu^2}{q} \biggr) \frac{(1+\ell_i^2)^2}{\ell_i^{4}}\biggr]^3\mathfrak{K} \, . </math>

Or, in terms of the ratio,

<math>\Chi \equiv \frac{\chi}{\chi_\mathrm{eq}} \, ,</math>

and pulling from the above expressions,

<math>~\biggl[ \frac{W_\mathrm{core}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ - \biggl( \frac{3^8}{2^5\pi } \biggr)^{1/2} \biggl[ \ell_i \biggl(\ell_i^4 - \frac{8}{3} \ell_i^2 -1 \biggr) (1 + \ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr] </math>
	<math>~=~</math>	<math>~ - \biggl( \frac{3^8}{2^5\pi } \biggr)^{1/2} \biggl[ \frac{\ell_i}{(1+\ell_i^2)} \biggr]^{3} \biggl[ \mathfrak{L}_i - \frac{8}{3}\biggr] </math>
<math>~\biggl[\frac{W_\mathrm{env}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ -\biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1+\Lambda_i^2)\biggl[\frac{\pi}{2}+\tan^{-1}(\Lambda_i)\biggr] + \Lambda_i + \frac{2}{3} \cdot \eta_i \biggr\} </math>
	<math>~=~</math>	<math>~ -\biggl( \frac{3^8}{2^5\pi} \biggr)^{1/2} \biggl[ \frac{\ell_i}{(1+\ell_i^2)} \biggr]^{3} \biggl[4\mathfrak{K}_i + \frac{8}{3} \biggr] </math>
<math>~\biggl[ \frac{S_\mathrm{core}}{E_\mathrm{norm}}\biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ \frac{1}{2} \biggl( \frac{3^8}{2^5\pi} \biggr)^{1/2} \biggl[ \ell_i (\ell_i^4 - 1 )(1+\ell_i^2)^{-3} + \tan^{-1}(\ell_i) \biggr] </math>
	<math>~=~</math>	<math>~ \frac{1}{2} \biggl( \frac{3^8}{2^5\pi} \biggr)^{1/2} \biggl[ \frac{\ell_i}{(1+\ell_i^2)} \biggr]^{3}\mathfrak{L}_i </math>
<math>~\biggl[\frac{S_\mathrm{env}}{E_\mathrm{norm}} \biggr]_\mathrm{eq}</math>	<math>~=~</math>	<math>~ ~\frac{1}{2} \biggl( \frac{3^2}{2\pi} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \eta_i^2 \biggl\{ (1 + \Lambda_i^2)\biggl[\frac{\pi}{2} + \tan^{-1}(\Lambda_i) \biggr] + \Lambda_i \biggr\} </math>
	<math>~=~</math>	<math>~ ~\frac{1}{2} \biggl( \frac{3^8}{2^5\pi} \biggr)^{1/2} \biggl[ \frac{\ell_i}{(1+\ell_i^2)} \biggr]^{3} (4\mathfrak{K}_i) \, , </math>

we have the streamlined,

<math>~\biggl( \frac{2^5\pi}{3^6} \biggr)^{1/2} \biggl[ \frac{(1+\ell_i^2)}{\ell_i} \biggr]^{3} \biggl[\frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr]</math>

<math>~ +\Chi^{-3/5} (5 \mathfrak{L}_i) +\Chi^{-3} (4\mathfrak{K}_i) -\Chi^{-1} (3\mathfrak{L}_i +12\mathfrak{K}_i ) </math>

or, better yet,

Out-of-Equilibrium, Free-Energy Expression for BiPolytropes with <math>~(n_c, n_e) = (5, 1)</math>

<math>~2^4\biggl( \frac{q\ell_i^2}{\nu^2}\biggr) \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr]</math>

<math>~ \Chi^{-3/5} (5 \mathfrak{L}_i) +\Chi^{-3} (4\mathfrak{K}_i) -\Chi^{-1} (3\mathfrak{L}_i +12\mathfrak{K}_i ) </math>

where,

<math>~\mathfrak{L}_i</math>	<math>~\equiv</math>	<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i \, ,</math>
<math>~\mathfrak{K}_i</math>	<math>~\equiv</math>	<math>~\frac{\Lambda_i}{\eta_i} + \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \, ,</math>
<math>~\Lambda_i</math>	<math>~\equiv</math>	<math>~\frac{1}{\eta_i} - \ell_i \, ,</math>
<math>~\eta_i</math>	<math>~=</math>	<math>~3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] \, .</math>

From the accompanying Table 1 parameter values, we also can write,

<math>~\frac{1}{q}</math>	<math>~=</math>	<math>~\frac{\eta_s}{\eta_i} = 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \, ,</math>
<math>~\nu</math>	<math>~=</math>	<math>~ \frac{\ell_i q}{(1+\Lambda_i^2)^{1/2}} \, . </math>

Radial Derivatives

<math>~\frac{\partial \mathfrak{G}^*}{\partial \Chi}</math>	<math>~=</math>	<math>~ -\Chi^{-8/5} (3 \mathfrak{L}_i) -\Chi^{-4} (12\mathfrak{K}_i) +\Chi^{-2} (3\mathfrak{L}_i +12\mathfrak{K}_i ) </math>
<math>~\frac{\partial^2 \mathfrak{G}^*}{\partial \Chi^2}</math>	<math>~=</math>	<math>~\frac{3}{5}\biggl[ \Chi^{-13/5} (8\mathfrak{L}_i) +\Chi^{-5} (80\mathfrak{K}_i) -\Chi^{-1} (10\mathfrak{L}_i +40\mathfrak{K}_i )\biggr] </math>

Consistent with our generic discussion of the stability of bipolytropes and the specific discussion of the stability of bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>, it can straightforwardly be shown that <math>~\partial \mathfrak{G}/\partial \chi = 0</math> is satisfied by setting <math>~\Chi = 1</math>; that is, the equilibrium condition is,

<math>~\chi = \chi_\mathrm{eq}</math>

<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} \, .</math>

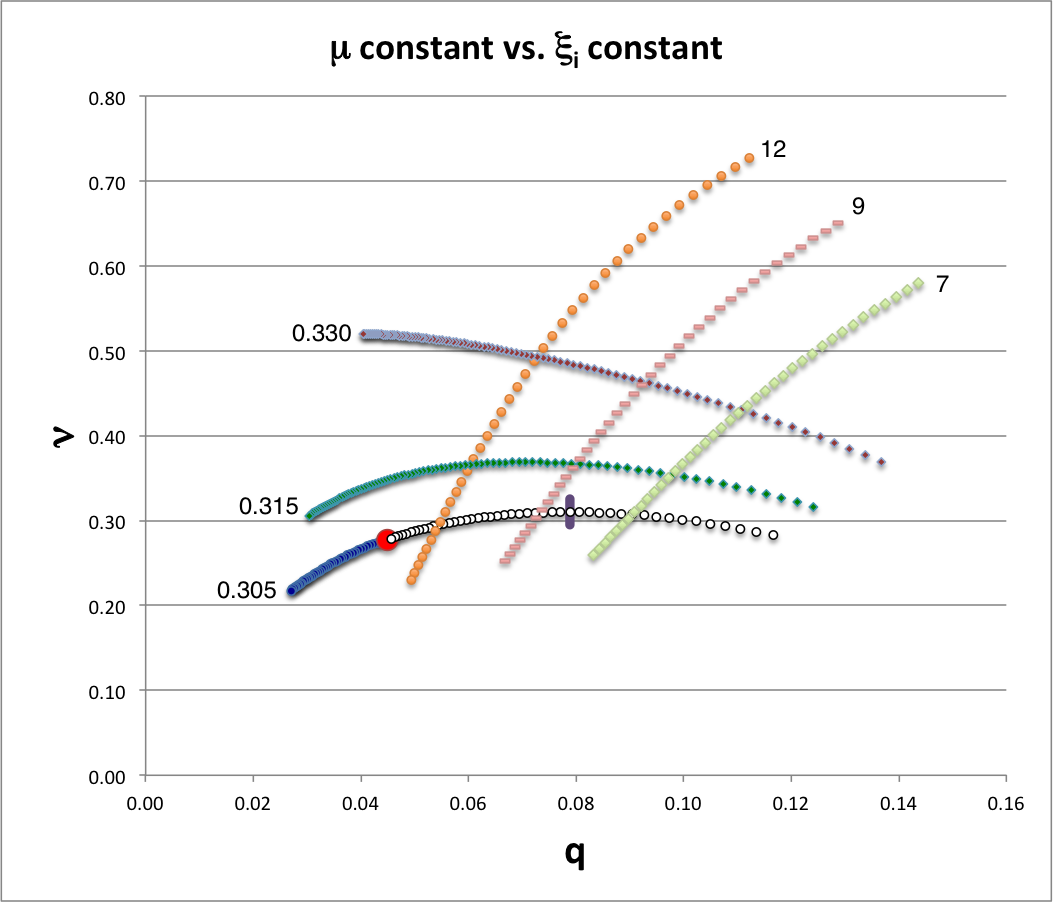
Furthermore, the equilibrium configuration is unstable whenever <math>~\partial^2 \mathfrak{G}/\partial \chi^2 < 0</math>, that is, it is unstable whenever,

<math>~\frac{ \mathfrak{L}_i}{\mathfrak{K}_i}</math>

Table 1 of an accompanying chapter — and the red-dashed curve in the figure adjacent to that table — identifies some key properties of the model that marks the transition from stable to unstable configurations along equilibrium sequences that have various values of the mean-molecular weight ratio, <math>~\mu_e/\mu_c</math>.

Focus on Zero-Zero Free-Energy Expression

Here, we will draw heavily from the following accompanying chapters:

From Detailed Force-Balance Models

Equilibrium Radius

First View

In an accompanying chapter we find,

<math>~ \frac{P_0 R_\mathrm{eq}^4}{G M_\mathrm{tot}^2 } </math>

<math>~\biggl( \frac{3}{2^3 \pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^2 \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] </math>

where,

<math>~f</math>	<math>~\equiv</math>	<math> 1+ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \biggl(\frac{1}{q^2}-1 \biggr) +\biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \biggl[ \frac{1}{q^5}-1 + \frac{5}{2} \biggl( 1-\frac{1}{q^2}\biggr)\biggr] \, , </math>
<math>~\mathfrak{F} </math>	<math>~\equiv</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ (-2q^2 + 3q^3 - q^5) + \frac{3}{5} \biggl( \frac{\rho_e}{\rho_c}\biggr) (-1 +5q^2 - 5q^3 + q^5) \biggr] \, , </math>
<math>~\frac{\rho_e}{\rho_c} </math>	<math>~=</math>	<math>~ \frac{q^3(1-\nu)}{\nu(1-q^3)} \, . </math>

Here, we prefer to normalize the equilibrium radius to <math>~R_\mathrm{norm}</math>. So, let's replace the central pressure with its expression in terms of <math>~K_c</math>. Specifically,

<math>~P_0</math>	<math>~=</math>	<math>~ K_c \rho_c^{\gamma_c} = K_c \biggl[ \frac{3M_\mathrm{core}}{4\pi R_i^3} \biggr]^{\gamma_c} = K_c \biggl[ \frac{3\nu M_\mathrm{tot}}{4\pi q^3 R_\mathrm{eq}^3} \biggr]^{(n_c+1)/n_c} ~~~\Rightarrow~~~ \frac{P_0}{P_\mathrm{norm}} = \biggl[ \frac{3}{4\pi}\biggl(\frac{\nu}{q^3}\biggr) \frac{1}{\chi_\mathrm{eq}^3}\biggr]^{(n_c+1)/n_c} </math>
<math>~\Rightarrow~~~K_c \biggl[ \frac{3\nu M_\mathrm{tot}}{4\pi q^3 R_\mathrm{eq}^3} \biggr]^{(n_c+1)/n_c} \frac{R_\mathrm{eq}^4}{G M_\mathrm{tot}^2 } </math>	<math>~=</math>	<math>~\biggl( \frac{3}{2^3 \pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^2 \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] </math>
<math>~\Rightarrow~~~R_\mathrm{eq}^{(n_c-3)/n_c} </math>	<math>~=</math>	<math>~ \biggl(\frac{G}{K_c}\biggr) M_\mathrm{tot}^{(n_c-1)/n_c} \biggl[ \frac{3\nu }{4\pi q^3 } \biggr]^{-(n_c+1)/n_c} \biggl( \frac{3}{2^3 \pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^2 \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] </math>
<math>~\Rightarrow~~~\chi_\mathrm{eq}^{(n_c-3)/n_c} \equiv \biggl[\frac{R_\mathrm{eq}}{R_\mathrm{norm}}\biggr]^{(n_c-3)/n_c}</math>	<math>~=</math>	<math>~ \frac{1}{2}\biggl(\frac{4\pi}{3} \biggr)^{1/n_c} \biggl( \frac{\nu}{q^3}\biggr)^{(n_c-1)/n_c} \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] \, . </math>

Or, in terms of <math>~\gamma_c</math>,

<math>~\chi_\mathrm{eq}^{4-3\gamma_c} </math>

<math>~ \frac{1}{2}\biggl(\frac{3}{4\pi} \biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3}\biggr)^{2-\gamma_c} \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] \, . </math>

Second View

Alternatively, from our derivation and discussion of analytic detailed force-balance models,

<math> \biggl[ \frac{R^4}{GM_\mathrm{tot}^2} \biggr] P_0</math>

<math>\biggl( \frac{3}{2^3\pi} \biggr) \frac{\nu^2 g^2}{q^4} \, ,</math>

where,

<math>~\equiv</math>

<math> 1 + \biggl(\frac{\rho_e}{\rho_0}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_0} \biggr) \biggl( 1-q \biggr) + \frac{\rho_e}{\rho_0} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] \, . </math>

In order to show that this expression is the same as the other one, above, we need to show that,

<math>~\biggl( \frac{3}{2^3 \pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^2 \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] </math>	<math>~=</math>	<math>\biggl( \frac{3}{2^3\pi} \biggr) \frac{\nu^2 g^2}{q^4} </math>
<math>~\Rightarrow~~~ f - 1-\mathfrak{F} </math>	<math>~=</math>	<math>~\frac{5}{2q^3} \biggl[g^2-1\biggr]</math>
	<math>~=</math>	<math>~\frac{5}{2q^3} \biggl(\frac{\rho_e}{\rho_0}\biggr) \biggl[ 2 \biggl(1 - \frac{\rho_e}{\rho_0} \biggr) \biggl( 1-q \biggr) + \frac{\rho_e}{\rho_0} \biggl(\frac{1}{q^2} - 1\biggr) \biggr] </math>
	<math>~=</math>	<math>~\frac{5}{2q^5} \biggl(\frac{\rho_e}{\rho_0}\biggr) \biggl\{ 2 ( q^2 - q^3 ) + \frac{\rho_e}{\rho_0}\biggl[ 1 - 3q^2+ 2q^3 \biggr] \biggr\} \, .</math>

Let's see …

<math>~ f - 1-\mathfrak{F} </math>	<math>~=</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \biggl(\frac{1}{q^2}-1 \biggr) +\biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \biggl[ \frac{1}{q^5}-1 + \frac{5}{2} \biggl( 1-\frac{1}{q^2}\biggr)\biggr] - \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ (-2q^2 + 3q^3 - q^5) + \frac{3}{5} \biggl( \frac{\rho_e}{\rho_c}\biggr) (-1 +5q^2 - 5q^3 + q^5) \biggr] </math>
	<math>~=</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \biggl(\frac{1}{q^2}-1 \biggr) - \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ (-2q^2 + 3q^3 - q^5) \biggr] </math>
		<math>~ - \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ \frac{3}{5} \biggl( \frac{\rho_e}{\rho_c}\biggr) (-1 +5q^2 - 5q^3 + q^5) \biggr] +\biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \biggl[ \frac{1}{q^5}-1 + \frac{5}{2} \biggl( 1-\frac{1}{q^2}\biggr)\biggr] </math>
	<math>~=</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl\{ (q^3- q^5 ) + (2q^2 - 3q^3 + q^5) \biggr\} </math>
		<math>~ + \frac{1}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \frac{1}{q^5} \biggl[ 3 (1 -5q^2 + 5q^3 - q^5) \biggr] +\frac{1}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \frac{1}{q^5} \biggl[ 2 - 2q^5 + 5\biggl( q^5-q^3\biggr)\biggr] </math>
	<math>~=</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ (q^3- q^5 ) + (2q^2 - 3q^3 + q^5) \biggr] + \frac{1}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \frac{1}{q^5} \biggl[ 3 (1 -5q^2 + 5q^3 - q^5)+2 - 2q^5 + 5( q^5-q^3) \biggr] </math>
	<math>~=</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ 2q^2 - 2q^3 \biggr] + \frac{5}{2q^5} \biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \biggl[ 1 - 3q^2 + 2q^3 \biggr] \, . </math>

Q.E.D.

Hence, the equilibrium radius can also be written as,

<math>~\chi_\mathrm{eq}^{4-3\gamma_c} </math>

<math>~ \frac{1}{2}\biggl(\frac{3}{4\pi} \biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3}\biggr)^{2-\gamma_c} q^2 g^2 \, ; </math>

or, in terms of the polytropic index,

<math>~\chi_\mathrm{eq}^{n_c-3} </math>

<math>~ \frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} (q g)^{2n_c} \, . </math>

Gravitational Potential Energy

Also from our accompanying discussion, we have,

<math>~\frac{W_\mathrm{grav}}{E_\mathrm{norm}} </math>	<math>~=</math>	<math> - \Chi^{-1} \biggl( \frac{3}{5}\biggr) \biggl(\frac{\nu}{q^3} \biggr)^2 q^5 \biggl[ \frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} (q g)^{2n_c} \biggr]^{-1/(n_c-3)} f(\nu,q) </math>
	<math>~=</math>	<math> - \Chi^{-1} \biggl( \frac{6}{5}\biggr) q^5 f \biggl[ 2^{n_c-(n_c-3)} \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{(1-n_c)+2(n_c-3)} b_\xi^{n_c} \biggr]^{1/(n_c-3)} </math>
	<math>~=</math>	<math> - \Chi^{-1} \biggl( \frac{6}{5}\biggr) q^5 f \biggl[ \biggl(\frac{6}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} b_\xi^{n_c} \biggr]^{1/(n_c-3)} \, . </math>

Internal Energy Components

First View

Before writing out the expressions for the internal energy of the core and of the envelope, we note from our separate detailed derivation that, in either case,

<math>~\biggl[\frac{P_i \chi^{3\gamma}}{P_\mathrm{norm}} \biggr]_\mathrm{eq} \chi^{3-3\gamma}</math>	<math>~=</math>	<math>~\biggl[\biggl(\frac{P_i }{P_0} \biggr) \biggl(\frac{P_0 }{P_\mathrm{norm}} \biggr)\chi^{3}\biggr]_\mathrm{eq} \biggl[\frac{\chi}{\chi_\mathrm{eq}}\biggr]^{3-3\gamma}</math>
	<math>~=</math>	<math>~\biggl\{\biggl(\frac{P_i }{P_0} \biggr) \biggl[ \frac{3}{4\pi } \biggl( \frac{\nu}{q^3} \biggr)\biggr]^{\gamma_c}\chi^{3-3\gamma_c}\biggr\}_\mathrm{eq} \Chi^{3-3\gamma} \, ,</math>

where, in equilibrium,

<math>~\biggl(\frac{P_i }{P_0} \biggr)_\mathrm{eq}</math>	<math>~=</math>	<math>~1 - b_\xi q^2</math>
<math>~b_\xi q^2</math>	<math>~=</math>	<math>~\biggl\{\frac{2}{5}q^3 f + \biggl[1 - \frac{2}{5} q^3( 1+\mathfrak{F} ) \biggr]\biggr\}^{-1} </math>
	<math>~=</math>	<math>~\biggl[1 + \frac{2}{5}q^3 (f - 1-\mathfrak{F} ) \biggr]^{-1} </math>

So, copying from our accompanying detailed derivation, we have,

<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{core}</math>	<math>~=</math>	<math> \frac{4\pi/3 }{({\gamma_c}-1)} \biggl\{\biggl(\frac{P_i }{P_0} \biggr) \biggl[ \frac{3}{4\pi } \biggl( \frac{\nu}{q^3} \biggr)\biggr]^{\gamma_c}\chi^{3-3\gamma_c}\biggr\}_\mathrm{eq} \Chi^{3-3\gamma_c} \biggl\{ \biggl( \frac{P_0}{P_{ic}} \biggr) \biggl[ q^3 - \biggl( \frac{3b_\xi}{5} \biggr) q^5 \biggr] \biggr\} </math>
	<math>~=</math>	<math> \frac{1 }{({\gamma_c}-1)} \biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr] \Chi^{3-3\gamma_c} q^3\biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] \, , </math>
<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{env}</math>	<math>~=</math>	<math> \frac{4\pi/3 }{({\gamma_e}-1)} \biggl\{\biggl(\frac{P_i }{P_0} \biggr) \biggl[ \frac{3}{4\pi } \biggl( \frac{\nu}{q^3} \biggr)\biggr]^{\gamma_c}\chi^{3-3\gamma_c}\biggr\}_\mathrm{eq} \Chi^{3-3\gamma_e} \biggl\{ (1-q^3) + b_\xi \biggl(\frac{P_0}{P_{ie} } \biggr) \biggl[\frac{2}{5} q^5 \mathfrak{F} \biggr] \biggr\} </math>
	<math>~=</math>	<math> \frac{1}{({\gamma_e}-1)} \biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr] \Chi^{3-3\gamma_e} \biggl(\frac{P_i }{P_0} \biggr) \biggl\{ (1-q^3) + b_\xi \biggl(\frac{P_0}{P_{ie} } \biggr) \biggl[\frac{2}{5} q^5 \mathfrak{F} \biggr] \biggr\} </math>
	<math>~=</math>	<math> \frac{1}{({\gamma_e}-1)} \biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr] \Chi^{3-3\gamma_e} \biggl\{ (1-b_\xi q^2)(1-q^3) + b_\xi \biggl[\frac{2}{5} q^5 \mathfrak{F} \biggr] \biggr\} </math>
	<math>~=</math>	<math> \frac{1}{({\gamma_e}-1)} \biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr] \Chi^{3-3\gamma_e} (1-q^3) \biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} \, . </math>

Furthermore,

<math>~\biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr]</math>	<math>~=</math>	<math>~ \biggl(\frac{3}{4\pi}\biggr)^{\gamma_c - 1} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c} \biggl\{\chi_\mathrm{eq}^{4-3\gamma_c}\biggr\}^{(3-3\gamma_c)/(4-3\gamma_c)} </math>
	<math>~=</math>	<math>~ \biggl(\frac{3}{4\pi}\biggr)^{\gamma_c - 1} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c} \biggl\{\frac{1}{2}\biggl(\frac{3}{4\pi} \biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3}\biggr)^{2-\gamma_c} \biggl[ q^2 + \frac{2}{5} q^5( f - 1-\mathfrak{F} )\biggr] \biggr\}^{(3-3\gamma_c)/(4-3\gamma_c)} </math>
	<math>~=</math>	<math>~ \biggl(\frac{3}{4\pi}\biggr)^{(\gamma_c - 1)/(4-3\gamma_c)} \biggl( \frac{\nu}{q^3} \biggr)^{(6-5\gamma_c)(4-3\gamma_c)} \biggl\{\frac{q^2}{2} \biggl[ 1 + \frac{2}{5} q^3( f - 1-\mathfrak{F} )\biggr] \biggr\}^{(3-3\gamma_c)/(4-3\gamma_c)} </math>
	<math>~=</math>	<math>~ \biggl(\frac{3}{4\pi}\biggr)^{1/(n_c-3)} \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)(n_c-3)} \biggl\{\frac{q^2}{2} \biggl[ 1 + \frac{2}{5} q^3( f - 1-\mathfrak{F} )\biggr] \biggr\}^{-3/(n_c-3)} </math>
	<math>~=</math>	<math>~ \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^3\biggr]^{1/(n_c-3)} \, . </math>

Hence, we have,

<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{core}</math>	<math>~=</math>	<math> n_c \biggl[\biggl(\frac{4\pi}{3}\biggr)^{1-\gamma_c} \biggl( \frac{\nu}{q^3} \biggr)^{\gamma_c}\chi_\mathrm{eq}^{3-3\gamma_c}\biggr] \Chi^{-3/n_c} q^3\biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] </math>
	<math>~=</math>	<math> n_c \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^3\biggr]^{1/(n_c-3)} \Chi^{-3/n_c} q^3\biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] \, , </math>
<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{env}</math>	<math>~=</math>	<math> n_e \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^3\biggr]^{1/(n_c-3)} \Chi^{-3/n_e} (1-q^3) \biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} \, . </math>

Second View

In our accompanying discussion of energies associated with detailed force balance models, we used the notation,

<math>~\equiv</math>

<math>~ \biggl(\frac{3}{2^3\pi}\biggr) \frac{GM_\mathrm{tot}^2}{R^4} \biggl(\frac{\nu}{q^3}\biggr)^2 = P_\mathrm{norm} \chi^{-4}\biggl(\frac{3}{2^3\pi}\biggr) \biggl(\frac{\nu}{q^3}\biggr)^2 \, , </math>

which allows us to rewrite the above quoted relationship between the central pressure and the radius of the bipolytrope as,

We also showed that, in equilibrium, the relationship between the central pressure and the interface pressure is,

<math>~P_0 =P_i + \Pi_\mathrm{eq} q^2 \, .</math>

This means that, in equilibrium, the ratio of the interface pressure to the central pressure is,

<math>~\biggl(\frac{P_i}{P_0}\biggr)_\mathrm{eq}</math>

<math>~1 - \frac{\Pi_\mathrm{eq} q^2}{P_0} = 1- \frac{1}{g^2} \, , </math>

or given that (see above),

<math>~

\frac{5}{2q^3} \biggl[g^2-1\biggr]

</math>

<math>~ f - 1-\mathfrak{F} </math>

<math>~\Rightarrow~~~~ g^2 </math>

<math>~ 1+\frac{2}{5} q^3 ( f - 1-\mathfrak{F} ) \, , </math>

we have,

<math>~\biggl(\frac{P_i}{P_0}\biggr)_\mathrm{eq}</math>

<math>~1 - \frac{\Pi_\mathrm{eq} q^2}{P_0} = 1- \biggl[ 1+\frac{2}{5} q^3 ( f - 1-\mathfrak{F} ) \biggr]^{-1} \, . </math>

This is exactly the pressure-ratio expression presented in our "first view" and unveils the notation association,

<math>~\leftrightarrow~</math>

From our separate derivation, we have, in equilibrium,

<math>~\mathfrak{G}_\mathrm{core} = \biggl(\frac{2n_c}{3}\biggr) S_\mathrm{core}</math>	<math>~=~</math>	<math>\biggl(\frac{2n_c}{3}\biggr) \biggl( \frac{4\pi}{5} \biggr) R_\mathrm{eq}^3 q^5 \biggl (\frac{5P_i}{2q^2} + \Pi \biggr)_\mathrm{eq} </math>
	<math>~=~</math>	<math>\biggl( \frac{ q^5n_c}{5} \biggr) R_\mathrm{eq}^3 \biggl( \frac{2^3\pi}{3} \biggr) \Pi_\mathrm{eq} \biggl[\frac{5}{2q^2} \biggl( \frac{P_i}{\Pi} \biggr)_\mathrm{eq} + 1 \biggr] </math>
	<math>~=~</math>	<math>\biggl( \frac{ n_c}{5} \biggr) \biggl[ R_\mathrm{norm}^3 P_\mathrm{norm} \biggr] \chi_\mathrm{eq}^{-1} \biggl(\frac{\nu^2}{q}\biggr) \biggl[\frac{5}{2q^2} \biggl( \frac{P_i}{P_0} \biggr)_\mathrm{eq}\biggl( \frac{P_0}{\Pi} \biggr)_\mathrm{eq} + 1 \biggr] </math>
<math>~\Rightarrow ~~~\biggl[ \frac{\mathfrak{G}_\mathrm{core} }{E_\mathrm{norm}}\biggr]_\mathrm{eq} </math>	<math>~=~</math>	<math>~\biggl( \frac{ n_c}{5} \biggr) \biggl(\frac{\nu^2}{q}\biggr) \biggl[\frac{5}{2q^2} \biggl( 1-\frac{1}{g^2} \biggr)\biggl( q^2g^2\biggr) + 1 \biggr] \chi_\mathrm{eq}^{-1} </math>
	<math>~=~</math>	<math>~\biggl( \frac{ n_c}{2} \biggr) \biggl(\frac{\nu^2}{q}\biggr) \biggl[ g^2-\frac{3}{5} \biggr] \biggl\{\frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} (q g)^{2n_c} \biggr\}^{-1/(n_c-3)}</math>
	<math>~=~</math>	<math>~n_c \biggl[ 1- \biggl(\frac{3}{5}\biggr) \frac{1}{g^2} \biggr] \biggl( \frac{ 1}{2} \biggr) \biggl(\frac{\nu^2}{q}\biggr) g^2 \biggl\{2^{n_c}\biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{1-n_c} (q g)^{-2n_c} \biggr\}^{1/(n_c-3)}</math>
	<math>~=~</math>	<math>~n_c \biggl[ 1- \biggl(\frac{3}{5}\biggr) \frac{1}{g^2} \biggr] \biggl\{2^{n_c}\cdot 2^{(3-n_c)}\biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{1-n_c} \biggl(\frac{\nu}{q^3}\biggr)^{2(n_c-3)} q^{5(n_c-3)} q^{-2n_c} g^{-2n_c} g^{2(n_c-3)} \biggr\}^{1/(n_c-3)}</math>
	<math>~=~</math>	<math>~n_c \biggl[ 1- \biggl(\frac{3}{5}\biggr) \frac{1}{g^2} \biggr] \biggl\{\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} q^{3n_c-15} g^{-6} \biggr\}^{1/(n_c-3)} \, .</math>

Finally, switching from the <math>~g</math> notation to the <math>~b_\xi</math> notation gives,

<math>~\biggl[ \frac{\mathfrak{G}_\mathrm{core} }{E_\mathrm{norm}}\biggr]_\mathrm{eq} </math>	<math>~=~</math>	<math>~n_c \biggl[ 1- \biggl(\frac{3}{5}\biggr) b_\xi q^2 \biggr] \biggl\{\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} q^{3n_c-15} b_\xi^3 q^{6} \biggr\}^{1/(n_c-3)} </math>
	<math>~=~</math>	<math>~n_c q^3 \biggl[ 1- \biggl(\frac{3}{5}\biggr) b_\xi q^2 \biggr] \biggl\{\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} b_\xi^3 \biggr\}^{1/(n_c-3)} \, ,</math>

which, after setting <math>~\Chi = 1</math>, precisely matches the above, "first view" expression. Also from our previous derivation, we can write,

<math>~\mathfrak{G}_\mathrm{env} = \biggl(\frac{2n_e}{3}\biggr) S_\mathrm{env}</math>	<math>~=~</math>	<math>~ 2\pi\biggl(\frac{2n_e}{3}\biggr) R_\mathrm{eq}^3 \Pi_\mathrm{eq} \biggl\{ (1-q^3) \biggl(\frac{P_i }{\Pi}\biggr)_\mathrm{eq} + \biggl( \frac{\rho_e}{\rho_0} \biggr)\biggl[ (-2q^2 + 3q^3 - q^5 ) + \frac{3}{5} \biggl( \frac{\rho_e}{\rho_0} \biggr) ( -1 + 5q^2 -5q^3 + q^5 )\biggr]\biggr\} </math>
	<math>~=~</math>	<math>~ 2\pi\biggl(\frac{2n_e}{3}\biggr) R_\mathrm{eq}^3 \biggl[ P_\mathrm{norm} \chi^{-4}\biggl(\frac{3}{2^3\pi}\biggr) \biggl(\frac{\nu}{q^3}\biggr)^2\biggr]_\mathrm{eq} \biggl\{ (1-q^3) q^2(g^2-1) + \biggl(\frac{2}{5}\biggr) q^5 \mathfrak{F} \biggr\} </math>
	<math>~=~</math>	<math>~ \biggl[ P_\mathrm{norm} R_\mathrm{norm}^3 \biggr] \frac{n_e}{2} \biggl(\frac{\nu^2}{q^4}\biggr)(1-q^3) \biggl\{ (g^2-1) + \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr\} \chi^{-1}_\mathrm{eq} </math>
<math>~\Rightarrow~~~\biggl[ \frac{\mathfrak{G}_\mathrm{env} }{E_\mathrm{norm}}\biggr]_\mathrm{eq} </math>	<math>~=~</math>	<math>~ n_e (1-q^3) \biggl\{ (g^2-1) + \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr\} \frac{q^2}{2}\biggl(\frac{\nu}{q^3}\biggr)^2 \biggl[\frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} (q g)^{2n_c}\biggr]^{-1/(n_c-3)} </math>
	<math>~=~</math>	<math>~ n_e (1-q^3) \biggl\{ (g^2-1) + \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr\} \biggl[2^{[n_c-(n_c-3)]} \biggl(\frac{3}{4\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{(1-n_c)+2(n_c-3)} q^{2(n_c-3)-2n_c} g^{-2n_c} \biggr]^{1/(n_c-3)} </math>
	<math>~=~</math>	<math>~ n_e (1-q^3) \biggl\{ (g^2-1) + \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr\} \biggl[\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} q^{-6} g^{-2n_c} \biggr]^{1/(n_c-3)} \, . </math>

And, finally, switching from the <math>~g</math> notation to the <math>~b_\xi</math> notation gives,

<math>~\biggl[ \frac{\mathfrak{G}_\mathrm{env} }{E_\mathrm{norm}}\biggr]_\mathrm{eq} </math>	<math>~=~</math>	<math>~ n_e (1-q^3) (b_\xi q^2)^{-1} \biggl\{ 1 - \biggl[1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr]b_\xi q^2\biggr\} \biggl[\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} q^{-6} (b_\xi q^2)^{n_c} \biggr]^{1/(n_c-3)} </math>
	<math>~=~</math>	<math>~ n_e (1-q^3) \biggl\{ 1 - \biggl[1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr]b_\xi q^2\biggr\} \biggl[\biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} q^{-6-2(n_c-3)+2n_c} b_\xi^{3-n_c+n_c} \biggr]^{1/(n_c-3)} </math>
	<math>~=~</math>	<math>~ n_e\biggl[\biggl(\frac{2\cdot 3}{\pi} \biggr)\biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} b_\xi^{3} \biggr]^{1/(n_c-3)} (1-q^3) \biggl\{ 1 - \biggl[1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3} \biggr)\mathfrak{F} \biggr]b_\xi q^2\biggr\} \, , </math>

which, after setting <math>~\Chi = 1</math>, precisely matches the above, "first view" expression.

Summary00

In summary, the desired out of equilibrium free-energy expression is,

<math>~\frac{\mathfrak{G}}{E_\mathrm{norm}} </math>

where,

<math>~A_0 \equiv \biggl( \frac{\mathfrak{S}_\mathrm{core}}{E_\mathrm{norm}} \biggr)_\mathrm{eq}</math>	<math>~=</math>	<math> \frac{n_c}{b_\xi} \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^{n_c}\biggr]^{1/(n_c-3)} q^3\biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] \, , </math>
<math>~B_0 \equiv \biggl( \frac{\mathfrak{S}_\mathrm{env}}{E_\mathrm{norm}} \biggr)_\mathrm{eq}</math>	<math>~=</math>	<math> \frac{n_e}{b_\xi} \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^{n_c} \biggr]^{1/(n_c-3)} (1-q^3) \biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} \, , </math>
<math>~C_0 \equiv \biggl( \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr)_\mathrm{eq} </math>	<math>~=</math>	<math> \biggl( \frac{6}{5}\biggr) q^5 f \biggl[ \biggl(\frac{2\cdot 3}{\pi} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-5} b_\xi^{n_c} \biggr]^{1/(n_c-3)} \, . </math>

Or, in a more compact form,

<math>~\mathfrak{G}^* \equiv \biggl[ \biggl(\frac{2\cdot 3}{\pi}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(n_c-5)} b_\xi^{n_c}\biggr]^{-1/(n_c-3)} \biggl[\frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr]</math>

where,

<math>~A_1 </math>	<math>~\equiv</math>	<math> \frac{1}{b_\xi} (q^3) \biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] \, , </math>
<math>~B_1 </math>	<math>~\equiv</math>	<math> \frac{1}{b_\xi} (1-q^3)\biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} \, , </math>
<math>~C_1 </math>	<math>~\equiv</math>	<math> \biggl( \frac{2}{5}\biggr) q^5 f \, . </math>

Let's examine the behavior of the first radial derivative.

<math>~\frac{\partial \mathfrak{G}^*}{\partial \Chi}</math>

<math>~\frac{3}{\Chi} \biggl[ - A_1\Chi^{-3/n_c} - B_1\Chi^{-3/n_e} + C_1\Chi^{-1} \biggr] \, .</math>

Let's see whether the sum of terms inside the square brackets is zero at the derived equilibrium radius, that is, when <math>~\Chi = 1</math> and, hence, when

<math>~\chi = \chi_\mathrm{eq}</math>	<math>~=</math>	<math>~ \biggl[ \frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} (q g)^{2n_c} \biggr]^{1/(n_c-3)} </math>
	<math>~=</math>	<math>~ \biggl[ \frac{1}{2^{n_c}}\biggl(\frac{4\pi}{3} \biggr) \biggl( \frac{\nu}{q^3}\biggr)^{n_c-1} b_\xi^{-n_c} \biggr]^{1/(n_c-3)} \, . </math>

<math>~ C_1 - A_1 - B_1 </math>	<math>~=</math>	<math>~ \biggl( \frac{2}{5}\biggr) q^5 f - \frac{1}{b_\xi} (q^3) \biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] - \frac{1}{b_\xi} (1-q^3)\biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} </math>
	<math>~=</math>	<math>~ \biggl( \frac{2}{5}\biggr) q^5 f - \frac{1}{b_\xi} \biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} + \frac{q^3}{b_\xi} \biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} - \frac{q^3}{b_\xi} \biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] </math>
	<math>~=</math>	<math>~ \biggl( \frac{2}{5}\biggr) q^5 f - \frac{1}{b_\xi} + \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]q^2 + \frac{q^3}{b_\xi} - \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]q^5 - \frac{q^3}{b_\xi} + \biggl( \frac{3}{5} \biggr) q^5 </math>
	<math>~=</math>	<math>~q^2\biggl\{ \biggl( \frac{2}{5}\biggr) q^3 f - \frac{1}{b_\xi q^2} + \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr] (1-q^3) + \biggl( \frac{3}{5} \biggr) q^3 \biggr\} </math>
	<math>~=</math>	<math>~q^2\biggl\{ \biggl( \frac{2}{5}\biggr) q^3 f - \biggl[ 1+\frac{2}{5} q^3(f-1-\mathfrak{F}) \biggr] + \biggl[ (1-q^3) - \frac{2}{5} q^3 \mathfrak{F} \biggr] + \biggl( \frac{3}{5} \biggr) q^3 \biggr\} </math>
	<math>~=</math>	<math>~q^2\biggl\{0\biggr\} \, . </math>

Q.E.D.

Even slightly better:

<math>~\frac{1}{q^2}\biggl[ \biggl(\frac{\pi}{2\cdot 3}\biggr) \biggl( \frac{\nu}{q^3} \biggr)^{(5-n_c)} b_\xi^{-n_c}\biggr]^{1/(n_c-3)} \biggl[\frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr]</math>

or, better yet,

Out-of-Equilibrium, Free-Energy Expression for BiPolytropes with Structural <math>~(n_c, n_e) = (0, 0)</math>

<math>~2\biggl(\frac{q^2}{\nu}\biggr)^2 \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}}{E_\mathrm{norm}} \biggr]</math>

where, keeping in mind that,

<math>~\biggl[1 + \frac{2}{5}q^3 (f - 1-\mathfrak{F} ) \biggr] \, , </math>

we have,

<math>~A_2 \equiv \frac{A_1}{q^2} </math>	<math>~\equiv</math>	<math> \frac{q^3}{(b_\xi q^2)} \biggl[ 1 - \biggl( \frac{3}{5} \biggr) b_\xi q^2 \biggr] </math>
	<math>~=</math>	<math> q^3 \biggl\{ \biggl[1 + \frac{2}{5}q^3 (f - 1-\mathfrak{F} ) \biggr] - \biggl( \frac{3}{5} \biggr) \biggr\} </math>
	<math>~=</math>	<math> \frac{2}{5}q^3 \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \, , </math>
<math>~B_2 \equiv \frac{B_1}{q^2} </math>	<math>~\equiv</math>	<math> \frac{1}{(b_\xi q^2)} (1-q^3)\biggl\{ 1- \biggl[ 1 - \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr]b_\xi q^2 \biggr\} </math>
	<math>~=</math>	<math> (1-q^3)\biggl\{ \frac{1}{(b_\xi q^2)} -1 + \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr\} </math>
	<math>~=</math>	<math> (1-q^3)\biggl\{ \biggl[1 + \frac{2}{5}q^3 (f - 1-\mathfrak{F} ) \biggr] - 1 + \frac{2}{5} \biggl(\frac{q^3}{1-q^3}\biggr) \mathfrak{F} \biggr\} </math>
	<math>~=</math>	<math> \frac{2}{5} q^3 \biggl\{ (1-q^3) (f - 1-\mathfrak{F} ) + \mathfrak{F} \biggr\} </math>
	<math>~=</math>	<math> \frac{2}{5} q^3 \biggl\{ f - \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr]\biggr\} </math>
	<math>~=</math>	<math> \frac{2}{5} q^3 f - A_2 \, , </math>
<math>~C_2 \equiv \frac{C_1}{q^2} </math>	<math>~\equiv</math>	<math> \frac{2}{5} q^3 f \, . </math>

As before, the equilibrium system is dynamically unstable if <math>~\partial^2 \mathfrak{G}/\partial \Chi^2 < 0</math>. We have deduced that the system is unstable if,

<math>~\frac{n_e}{3}\biggl[ \frac{3-n_e}{n_c-n_e} \biggr] </math>

<math>~ \frac{A_2}{C_2} = \frac{1}{f} \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \, . </math>

Overview

BiPolytrope51

Key Analytic Expressions

Out-of-Equilibrium, Free-Energy Expression for BiPolytropes with <math>~(n_c, n_e) = (5, 1)</math>

<math>~\mathfrak{G}^*_{51} \equiv 2^4\biggl( \frac{q}{\nu^2}\biggr) \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}_{51}}{E_\mathrm{norm}} \biggr]</math>

<math>~ \frac{1}{\ell_i^2} \biggl[ \Chi^{-3/5} (5 \mathfrak{L}_i) +\Chi^{-3} (4\mathfrak{K}_i) -\Chi^{-1} (3\mathfrak{L}_i +12\mathfrak{K}_i ) \biggr] </math>

where,

<math>~\mathfrak{L}_i</math>	<math>~\equiv</math>	<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i \, ,</math>
<math>~\mathfrak{K}_i</math>	<math>~\equiv</math>	<math>~\frac{\Lambda_i}{\eta_i} + \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \, ,</math>
<math>~\Lambda_i</math>	<math>~\equiv</math>	<math>~\frac{1}{\eta_i} - \ell_i \, ,</math>
<math>~\eta_i</math>	<math>~=</math>	<math>~3 \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggl[\frac{\ell_i }{(1+\ell_i^2)}\biggr] \, .</math>

From the accompanying Table 1 parameter values, we also can write,

<math>~\frac{1}{q}</math>	<math>~=</math>	<math>~\frac{\eta_s}{\eta_i} = 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \, ,</math>
<math>~\nu</math>	<math>~=</math>	<math>~ \frac{\ell_i q}{(1+\Lambda_i^2)^{1/2}} \, . </math>

Consistent with our generic discussion of the stability of bipolytropes and the specific discussion of the stability of bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>, it can straightforwardly be shown that <math>~\partial \mathfrak{G}^*_{51}/\partial \chi = 0</math> is satisfied by setting <math>~\Chi = 1</math>; that is, the equilibrium condition is,

<math>~\chi = \chi_\mathrm{eq}</math>	<math>~=</math>	<math>~\biggl(\frac{\pi}{2^3}\biggr)^{1/2} \frac{\nu^2}{q} \cdot \frac{(1+\ell_i^2)^3}{3^3\ell_i^5} </math>
	<math>~=</math>	<math>~\biggl\{ \biggl(\frac{\pi}{3}\biggr) 2^{2-n_c} \nu^{n_c-1} q^{3-n_c} \biggl[\frac{(1+\ell_i^2)^{6/5}}{(3\ell_i^2)} \biggr]^{n_c} \biggr\}^{1/(n_c-3)} \, ,</math>

where the last expression has been cast into a form that more clearly highlights overlap with the expression, below, for the equilibrium radius for zero-zero bipolytropes. Furthermore, the equilibrium configuration is unstable whenever,

<math>~\biggl[ \frac{\partial^2 \mathfrak{G}^*_{51}}{\partial \chi^2}\biggr]_{\Chi=1} < 0 \, ,</math>

that is, it is unstable whenever,

<math>~\frac{ \mathfrak{L}_i}{\mathfrak{K}_i}</math>

Table 1 of an accompanying chapter — and the red-dashed curve in the figure adjacent to that table — identifies some key properties of the model that marks the transition from stable to unstable configurations along equilibrium sequences that have various values of the mean-molecular weight ratio, <math>~\mu_e/\mu_c</math>.

Behavior of Equilibrium Sequence

Let's identify the location of two turning points along the <math>~\nu(q)</math> sequence — one defines <math>~q_\mathrm{max}</math> and the other identifies <math>~\nu_\mathrm{max}</math>. They occur, respectively, where,

and

In deriving these expressions, we will use the relations,

<math>~\frac{d\eta_i}{d\ell_i}</math>	<math>~=</math>	<math>~\frac{m_3 (1-\ell_i^2)}{(1+\ell_i^2)^2} \, ,</math>
<math>~\frac{d\Lambda_i}{d\ell_i}</math>	<math>~=</math>	<math>~- \frac{1}{m_3\ell_i^2} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \, ,</math>

where,

<math>~m_3 \equiv 3\biggl(\frac{\mu_e}{\mu_c}\biggr) \, .</math>

Given that,

<math>~\biggl\{ 1 + \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\}^{-1} \, ,</math>

we find,

<math>~\frac{d\ln q}{d\ln \ell_i}</math>	<math>~=</math>	<math>~\frac{\ell_i}{q} \cdot ( -q^2) \frac{d}{d\ell_i} \biggl\{ \frac{1}{\eta_i}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] \biggr\} </math>
	<math>~=</math>	<math>~-q\ell_i \biggl\{-\frac{1}{\eta_i^2}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr]\frac{d\eta_i}{d\ell_i} + \frac{1}{\eta_i(1+\Lambda_i^2)} \frac{d\Lambda_i}{d\ell_i} \biggr\} </math>
	<math>~=</math>	<math>~q\ell_i \biggl\{\frac{(1-\ell_i^2)}{m_3 \ell_i^2}\biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \frac{(1+\ell_i^2)}{m_3^2 \ell_i^3(1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \biggr\} </math>
	<math>~=</math>	<math>~ \frac{q}{m_3^2}{\ell_i^2}\biggl\{m_3 \ell_i (1-\ell_i^2) \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \frac{(1+\ell_i^2)}{(1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \biggr\} \, . </math>

And, given that,

<math>~ \frac{\ell_i q}{(1+\Lambda_i^2)^{1/2}} \, . </math>

we find,

<math>~\frac{d\ln \nu}{d\ln \ell_i}</math>	<math>~=</math>	<math>~\frac{\ell_i}{\nu} \biggl\{ \frac{q}{(1+\Lambda_i^2)^{1/2}} + \frac{q}{(1+\Lambda_i^2)^{1/2}} \frac{d\ln q}{d\ln \ell_i} - \frac{\ell_i q \Lambda_i }{(1+\Lambda_i^2)^{3/2}} \frac{d\Lambda_i}{d\ell_i} \biggr\} </math>
	<math>~=</math>	<math>~\frac{q \ell_i}{ \nu(1+\Lambda_i^2)^{1/2}}\biggl\{ 1 + \frac{d\ln q}{d\ln \ell_i} + \frac{\Lambda_i }{m_3 \ell_i (1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \biggr\} </math>

In summary, then, the <math>~q_\mathrm{max}</math> turning point occurs where,

<math>~ m_3 \ell_i (1-\ell_i^2) \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \frac{(1+\ell_i^2)}{(1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \, ; </math>

and the <math>~\nu_\mathrm{max}</math> turning point occurs where,

<math>~0</math>	<math>~=</math>	<math>~ 1 + \frac{\Lambda_i }{m_3 \ell_i (1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] + \frac{q \ell_i^3 (1-\ell_i^2)}{m_3} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \frac{q\ell_i^2}{m_3^2}\cdot \frac{(1+\ell_i^2)}{(1+\Lambda_i^2)} \biggl[ 1 - \ell_i^2(1-m_3) \biggr] </math>
	<math>~=</math>	<math>~ 1 + \frac{q \ell_i^3 (1-\ell_i^2)}{m_3} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \biggl[ \frac{\Lambda_i }{m_3 \ell_i (1+\Lambda_i^2)} + \frac{q\ell_i^2}{m_3^2}\cdot \frac{(1+\ell_i^2)}{(1+\Lambda_i^2)} \biggr] \cdot \biggl[ 1 - \ell_i^2(1-m_3) \biggr] </math>
	<math>~=</math>	<math>~ 1 + \frac{q \ell_i^3 (1-\ell_i^2)}{m_3} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] + \frac{1}{m_3 \ell_i} \biggl[ \frac{\Lambda_i }{(1+\Lambda_i^2)} + \frac{q\ell_i^3}{m_3}\cdot \frac{(1+\ell_i^2)}{(1+\Lambda_i^2)} \biggr] \cdot \biggl[ 1 - \ell_i^2(1-m_3) \biggr] \, . </math>

For the record, we repeat, as well, that the transition from stable to dynamically unstable configurations occurs along the sequence when,

<math>~\frac{(\ell_i^4-1)}{\ell_i^2} + \frac{(1+\ell_i^2)^3}{\ell_i^3} \cdot \tan^{-1}\ell_i </math>	<math>~=</math>	<math>~20 \biggl\{ \frac{\Lambda_i}{\eta_i} + \frac{(1+\Lambda_i^2)}{\eta_i} \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \biggr\} </math>
	<math>~=</math>	<math>~\frac{20(1+\Lambda_i^2)(1+\ell_i^2)}{m_3\ell_i} \biggl\{ \frac{\Lambda_i}{(1+\Lambda_i^2)} + \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \biggr\} </math>
<math>~\Rightarrow ~~~ m_3 \ell_i (\ell_i^4-1) + m_3(1+\ell_i^2)^3\cdot \tan^{-1}\ell_i </math>	<math>~=</math>	<math>~20\ell_i^2 (1+\Lambda_i^2)(1+\ell_i^2) \biggl\{ \frac{\Lambda_i}{(1+\Lambda_i^2)} + \biggl[\frac{\pi}{2} + \tan^{-1}\Lambda_i\biggr] \biggr\} \, .</math>

Graphical Depiction of Free-Energy Surface

Figure 1: Free-Energy Surface for <math>~(n_c,n_e) = (5,1)</math> and <math>~\frac{\mu_e}{\mu_c} = 1</math>

Left Panel: The free energy (vertical, blue axis) is plotted as a function of the radial interface location, <math>~\xi_i</math> (red axis), and the normalized configuration radius, <math>~\Chi \equiv \chi/\chi_\mathrm{eq}</math> (green axis). Right Panel: Same as the left panel, but animated in order to highlight undulations of the surface. The value of the free energy is indicated by color as well as by the height of the warped surface — red identifies the lowest depicted energies while blue identifies the highest depicted energies; these same colors have been projected down onto the <math>~z = 0</math> plane to present a two-dimensional, color-contour plot. A multi-colored line segment drawn parallel to the <math>~\xi_i</math> axis at the value, <math>~\Chi = 1</math>, identifies the configuration's equilibrium radius for each value of the interface location. Equilibrium configurations marked in white lie at the bottom of the principal free-energy "valley" and are stable, while configurations marked in blue lie at the top of a free-energy "hill," indicating that they are unstable; the red dot identifies the location along this equilibrium sequence where the transition from stable to unstable configurations occurs.

For purposes of reproducibility, it is incumbent upon us to clarify how the values of the free energy were normalized in order to produce the free-energy surface displayed in Figure 1. The normalization steps are explicitly detailed within the fortran program, below, that generated the data for plotting purposes; here we provide a brief summary. We evaluated the normalized free energy, <math>~\mathfrak{G}^*_{51}</math>, across a <math>~200 \times 200</math> zone grid of uniform spacing, covering the following <math>~(x,y) = (\ell_i,\Chi)</math> domain:

<math>~\frac{1}{\sqrt{3}}</math>	<math>~\le \ell_i \le</math>	<math>~\frac{3}{\sqrt{3}}</math>
<math>~0.469230769</math>	<math>~\le \Chi\le</math>	<math>~2.0</math>

(With this specific definition of the y-coordinate grid, <math>~\Chi = 1</math> is associated with zone 70.) After this evaluation, a constant, <math>~E_\mathrm{fudge} = -10</math>, was added to <math>~\mathfrak{G}^*</math> in order to ensure that the free energy was negative across the entire domain. Then (inorm = 1), for each specified interface location, <math>~x = \ell_i</math>, employing the equilibrium value of the free energy,

<math>~E_0 = \mathfrak{G}^*_{51}(\ell_i, \Chi = 1) + E_\mathrm{fudge} \, ,</math>

the free energy was normalized across all values of <math>~y = \Chi</math> via the expression,

<math>~\mathrm{fe} = \frac{(\mathfrak{G}^*_{51} + E_\mathrm{fudge}) - (E_0)_i}{|E_0|_i} \, .</math>

Finally, for plotting purposes, at each grid cell vertex ("vertex") — as well as at each grid cell center ("cell") — the value of the free energy, <math>~\mathrm{fe}</math>, was renormalized as follows,

<math>~\mathrm{vertex} = \frac{\mathrm{fe} - \mathrm{min}(\mathrm{fe})}{ \mathrm{max}(\mathrm{fe}) - \mathrm{min}(\mathrm{fe})} \, .</math>

Via this last step, the minimum "vertex" energy across the entire domain was 0.0 while the maximum "vertex" energy was 1.0.

FORTRAN Program Documentation			Example Evaluations (See also associated Table 1)
Coord. Axis	Coord. Name	Associated Physical Quantity	<math>~\frac{\mu_e}{\mu_c} = 1</math>	<math>~\frac{\mu_e}{\mu_c} = 0.305</math>
x-axis	bsize	<math>~\ell_i \equiv \frac{\xi_i}{\sqrt{3}}</math>	<math>~\frac{2.416}{\sqrt{3}} = 1.395</math>	<math>~\frac{8.1938}{\sqrt{3}} = 4.7307</math>	<math>~\frac{14.389}{\sqrt{3}} = 8.3076</math>
y-axis	csize	<math>~\Chi \equiv \frac{\chi}{\chi_\mathrm{eq}}</math>	<math>~1</math>	<math>~1</math>	<math>~1</math>
Relevant Lines of Code
eta = 3.0d0muratiobsize/(1.0d0+bsize2) Gami = 1.0d0/eta-bsize frakL = (bsize4-1.0d0)/bsize*2 + & & DATAN(bsize)((1.0d0+bsize2)/bsize)3 frakK = Gami/eta + ((1.0d0+Gami*2)/eta)(pii/2.0d0+DATAN(Gami)) E0 = ((5.0d0frakL) + (4.0d0frakK)& & - (3.0d0frakL+12.0d0frakK))/bsize2+Efudge fescalar(j,k) = (csize(-0.6d0)(5.0d0frakL)& & + csize*(-3.0d0)(4.0d0frakK)& & - (3.0d0frakL+12.0d0frakK)/csize)/bsize*2 + Efudge if(inorm.eq.1)fescalar(j,k)=fescalar(j,k)/DABS(E0) & & - E0/DABS(E0)
Variable	Represents	Value calculated via the expression …
eta	<math>~\eta_i</math>	<math>~3 \biggl(\frac{\mu_e}{\mu_c}\biggr)\biggl[ \frac{\ell_i}{(1+\ell_i^2)} \biggr]</math>	<math>~1.421</math>	<math>~0.1851</math>	<math>~0.1086</math>
Gami	<math>~\Lambda_i</math>	<math>~\frac{1}{\eta_i} - \ell_i</math>	<math>~-0.691</math>	<math>~0.6705</math>	<math>~0.9033</math>
frakL	<math>~\mathfrak{L}_i</math>	<math>~ \frac{(\ell_i^4 - 1)}{\ell_i^2} + \biggl[ \frac{1+\ell_i^2}{\ell_i} \biggr]^3 \tan^{-1}\ell_i </math>	<math>~10.37</math>	<math>~186.80</math>	<math>~937.64</math>
frakK	<math>~\mathfrak{K}_i</math>	<math>~ \frac{\Lambda_i}{\eta_i} + \frac{(1 + \Lambda_i^2)}{\eta_i} \biggl[ \frac{\pi}{2} + \tan^{-1}\Lambda_i \biggr] </math>	<math>~0.518</math>	<math>~20.544</math>	<math>~46.882</math>
	<math>~\frac{\mathfrak{L}_i}{\mathfrak{K}_i}</math>		<math>~20</math>	<math>~9.093</math>	<math>~20</math>
E0 - Efudge	<math>~\mathfrak{G}^*_{51}(\ell_i,\Chi=1)</math>	<math>~ \frac{1}{\ell_i^2} \biggl[ 5 \mathfrak{L}_i + 4\mathfrak{K}_i - (3\mathfrak{L}_i +12\mathfrak{K}_i ) \biggr] = \frac{2(\mathfrak{L}_i - 4\mathfrak{K}_i)}{\ell_i^2} </math>	<math>~8.525</math>	<math>~9.3496</math>	<math>~21.737</math>

Figure 2: Free-Energy Surface for <math>~(n_c,n_e) = (5,1)</math> and <math>~\frac{\mu_e}{\mu_c} = 0.305</math>

Left Panel: The free energy (vertical, blue axis) is plotted as a function of the radial interface location, <math>~\xi_i</math> (red axis), and the normalized configuration radius, <math>~\Chi \equiv \chi/\chi_\mathrm{eq}</math> (green axis). Right Panel: Same as the left panel, but animated in order to highlight undulations of the surface. The value of the free energy is indicated by color as well as by the height of the warped surface — red identifies the lowest depicted energies while blue identifies the highest depicted energies; these same colors have been projected down onto the <math>~z = 0</math> plane to present a two-dimensional, color-contour plot. A multi-colored line segment drawn parallel to the <math>~\xi_i</math> axis at the value, <math>~\Chi = 1</math>, identifies the configuration's equilibrium radius for each value of the interface location. Equilibrium configurations marked in white lie at the bottom of the principal free-energy "valley" and are stable, while configurations marked in blue lie at the top of a free-energy "hill," indicating that they are unstable; the red dot identifies the location along this equilibrium sequence where the transition from stable to unstable configurations occurs.

BiPolytrope00

Out-of-Equilibrium, Free-Energy Expression for BiPolytropes with Structural <math>~(n_c, n_e) = (0, 0)</math>

<math>~\mathfrak{G}^*_{00} \equiv 5 \biggl(\frac{q}{\nu^2}\biggr) \chi_\mathrm{eq} \biggl[\frac{\mathfrak{G}_{00}}{E_\mathrm{norm}} \biggr]</math>

<math>~ \frac{5}{2q^3} \biggl[ n_c A_2\Chi^{-3/n_c} + n_e B_2\Chi^{-3/n_e} - 3C_2\Chi^{-1} \biggr] </math>

where,

<math>~A_2 </math>	<math>~\equiv</math>	<math> \frac{2}{5}q^3 \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \, , </math>
<math>~B_2 </math>	<math>~\equiv</math>	<math> \frac{2}{5} q^3 f - A_2 \, , </math>
<math>~C_2 </math>	<math>~\equiv</math>	<math> \frac{2}{5} q^3 f \, , </math>
<math>~f</math>	<math>~\equiv</math>	<math> 1+ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \biggl(\frac{1}{q^2}-1 \biggr) +\biggl( \frac{\rho_e}{\rho_c} \biggr)^2 \biggl[ \frac{1}{q^5}-1 + \frac{5}{2} \biggl( 1-\frac{1}{q^2}\biggr)\biggr] \, , </math>
<math>~\mathfrak{F} </math>	<math>~\equiv</math>	<math>~ \frac{5}{2} \biggl( \frac{\rho_e}{\rho_c} \biggr) \frac{1}{q^5} \biggl[ (-2q^2 + 3q^3 - q^5) + \frac{3}{5} \biggl( \frac{\rho_e}{\rho_c}\biggr) (-1 +5q^2 - 5q^3 + q^5) \biggr] \, , </math>
<math>~\frac{\rho_e}{\rho_c} </math>	<math>~=</math>	<math>~ \frac{q^3(1-\nu)}{\nu(1-q^3)} \, . </math>

The associated equilibrium radius is,

<math>~\chi_\mathrm{eq}</math>

<math>~ \biggl\{ \biggl(\frac{\pi}{3}\biggr) 2^{2-n_c} \nu^{n_c-1} q^{3-n_c} \biggl[1 + \frac{2}{5} q^3(f-1-\mathfrak{F})\biggr]^{n_c} \biggr\}^{1/(n_c-3)} \, . </math>

We have deduced that the system is unstable if,

<math>~\frac{n_e}{3}\biggl[ \frac{3-n_e}{n_c-n_e} \biggr] </math>

<math>~ \frac{A_2}{C_2} = \frac{1}{f} \biggl[1 + q^3 (f - 1-\mathfrak{F} ) \biggr] \, . </math>

Fortran Code

This is the program that generated the free-energy data for the "five-one" bipolytrope that is displayed in the above, Figure 1 image/animation.

      PROGRAM BiPolytrope
      real*8 pii
      real*8 bmin,bmax,cmin,cmax,db,dc
      real*8 c(200),b(200),chalf(199),bhalf(199)
      real*8 bsize,csize,emin,emax
      real*8 fepoint(200,200),fescalar(199,199)
      real*8 ell(200),ellhalf(199)
      real*8 muratio,eta,Gami,frakK,frakL
      real*8 eshift,ediff
      real   xx(200),yy(200),cell(199,199),vertex(200,200)
      real   valuemin,minlog,valufudge
      real*8 q,nu,chiEq,Enorm,E0,Efudge
      integer j,k,n,nmax,inorm
  101 format(4x,'bsize',7x,'csize',8x,'xi',10x,'A',12x,'B',12x,&
     &'fM',13x,'fW',11x,'fA',/)
! 102 format(1p8d12.4)
  103 format(2i5,1p3d14.6)
  201 format(5x,'valuemin = ',1pe15.5,//)
  205 format(5x,'For Cell-center ... emin, emax = ',1p2d14.6,/)
  206 format(5x,'For Cell-vertex ... emin, emax = ',1p2d14.6,/)
  701 format(5x,1p10d10.2)  
  700 format(8x,'xi',9x,'ell',8x,'eta',8x,'Lambda',5x,'frakK',&
     &       5x,'frakL',8x,'q',5x,'nu',5x,'chiEq',8x,'E0',/)
!!!!!!!!
!!!!!!!!
      inorm=1
      pii = 4.0d0*datan(1.0d0) 
      muratio = 1.0d0
      bsize = 0.0d0
      csize = 0.0d0
      Efudge = -10.0d0
      write(*,101)
!     write(*,102)bsize,csize,xival,coefA,coefB,fM,fW,fA
!!!!!!!!!!!
!
!  In this free-energy routine, c = X = chi/chi_eq and b = xi_i
!
!!!!!!!!!!!
      nmax = 200
      bmin = 1.0d0
      bmax = 3.0d0
      db   = (bmax-bmin)/dfloat(nmax-1)
      b(1) = bmin
      ell(1) = b(1)/dsqrt(3.0d0)
!  These values of cmin and cmax ensure that X=1 occurs at zone 70
      cmin = 0.469230769d0
      cmax = 2.00d0
      dc   = (cmax-cmin)/dfloat(nmax-1)
      c(1) = cmin
      do n=2,nmax
        b(n) = b(n-1)+db
        c(n) = c(n-1)+dc
        ell(n) = b(n)/dsqrt(3.0d0)
      enddo
      do n=1,nmax-1
        bhalf(n) = 0.5d0*(b(n)+b(n+1))
        chalf(n) = 0.5d0*(c(n)+c(n+1))
        ellhalf(n) = bhalf(n)/dsqrt(3.0d0)
      enddo
!
!  BEGIN LOOP to evaluate free energy (cell center)
!
      emin = 0.0d0
      emax = 0.0d0
      write(*,700)
      do j=1,nmax-1
        bsize = ellhalf(j)
      eta = 3.0d0*muratio*bsize/(1.0d0+bsize**2)
      Gami = 1.0d0/eta-bsize
      frakL = (bsize**4-1.0d0)/bsize**2 + &
     &        DATAN(bsize)*((1.0d0+bsize**2)/bsize)**3
      frakK = Gami/eta + ((1.0d0+Gami**2)/eta)*(pii/2.0d0+DATAN(Gami))
      q = 1.0d0/(1.0d0 + (0.5d0*pii+DATAN(Gami))/eta)
      nu = bsize*q/dsqrt(1.0d0+Gami**2)
      chiEq = dsqrt(pii/8.0d0)*(nu**2/(q*bsize**2))&
     &        *((1.0d0+bsize**2)/(3.0d0*bsize))**3
      Enorm = 16.0d0*(q/nu**2)*chiEq
      E0    = ((5.0d0*frakL) + (4.0d0*frakK)&
     &        - (3.0d0*frakL+12.0d0*frakK))/bsize**2+Efudge
      write(*,701)b(j),bsize,eta,Gami,frakK,frakL,q,nu,chiEq,E0
        do k=1,nmax-1
          csize=chalf(k)
          fescalar(j,k) = (csize**(-0.6d0)*(5.0d0*frakL)&
     &        + csize**(-3.0d0)*(4.0d0*frakK)&
     &   - (3.0d0*frakL+12.0d0*frakK)/csize)/bsize**2 + Efudge
          if(inorm.eq.1)fescalar(j,k)=fescalar(j,k)/DABS(E0) &
     &                  - E0/DABS(E0)
        if(fescalar(j,k).gt.0.5d0)fescalar(j,k)=0.5d0
          if(fescalar(j,k).lt.emin)emin=fescalar(j,k)
          if(fescalar(j,k).gt.emax)emax=fescalar(j,k)
!         write(*,103)j,k,bsize,csize,fescalar(j,k)
        enddo
      enddo
      write(*,205)emin,emax
!
!  BEGIN LOOP to evaluate free energy (cell vertex)
!
      emin = 0.0d0
      emax = 0.0d0
      do j=1,nmax
        bsize = ell(j)
      eta = 3.0d0*muratio*bsize/(1.0d0+bsize**2)
      Gami = 1.0d0/eta-bsize
      frakL = (bsize**4-1.0d0)/bsize**2 + &
     &        DATAN(bsize)*((1.0d0+bsize**2)/bsize)**3
      frakK = Gami/eta + ((1.0d0+Gami**2)/eta)*(pii/2.0d0+DATAN(Gami))
      q = 1.0d0/(1.0d0 + (0.5d0*pii+DATAN(Gami))/eta)
      nu = bsize*q/dsqrt(1.0d0+Gami**2)
      chiEq = dsqrt(pii/8.0d0)*(nu**2/(q*bsize**2))&
     &        *((1.0d0+bsize**2)/(3.0d0*bsize))**3
      Enorm = 16.0d0*(q/nu**2)*chiEq
      E0    = ((5.0d0*frakL) + (4.0d0*frakK)&
     &        - (3.0d0*frakL+12.0d0*frakK))/bsize**2 + Efudge
        do k=1,nmax
          csize=c(k)
          fepoint(j,k) = (csize**(-0.6d0)*(5.0d0*frakL)&
     &        + csize**(-3.0d0)*(4.0d0*frakK)&
     &   - (3.0d0*frakL+12.0d0*frakK)/csize)/bsize**2 + Efudge
          if(inorm.eq.1)fepoint(j,k)=fepoint(j,k)/DABS(E0) &
     &                  - E0/DABS(E0)
        if(fepoint(j,k).gt.0.5d0)fepoint(j,k)=0.5d0
          if(fepoint(j,k).lt.emin)emin=fepoint(j,k)
          if(fepoint(j,k).gt.emax)emax=fepoint(j,k)
!         write(*,103)j,k,bsize,csize,fepoint(j,k)
        enddo
      enddo
      write(*,206)emin,emax
!
!  Now fill single-precision arrays for plotting.
!
      do n=1,nmax
!       xx(n)=b(n)/b(nmax)
!       yy(n)=c(n)/c(nmax)
        xx(n)=b(n)-bmin
        yy(n)=c(n)-cmin
!       xx(n)=b(n)
!       yy(n)=c(n)
      enddo
      valuemin= -emin
      valufudge = 1.0d0/(emax-emin)
      do k=1,nmax
        do j=1,nmax
          vertex(j,k)=fepoint(j,k)+valuemin
          vertex(j,k)=vertex(j,k)*valufudge
        enddo
      enddo
      do k=1,nmax-1
        do j=1,nmax-1
          cell(j,k)=fescalar(j,k)+valuemin
          cell(j,k)=cell(j,k)*valufudge
        enddo
      enddo
      call XMLwriter01(nmax,xx,yy,cell,vertex)
      stop
      END PROGRAM BiPolytrope
      Subroutine XMLwriter01(imax,x,y,cell_scalar,point_scalar)
      real x(200),y(200),z(1)
      real cell_scalar(199,199),point_scalar(200,200)
      integer imax
      integer extentX,extentY,extentZ
      integer ix0,iy0,iz0
      integer norm(200,3)
!     imax=200
      ix0=0
      iy0=0
      iz0=0
      extentX=imax-1
      extentY=imax-1
      extentZ=0
      z(1) = 0.0
! Set normal vector 1D array
      do i=1,imax
        norm(i,1)=0
        norm(i,2)=0
        norm(i,3)=1
      enddo
  201 format('<?xml version="1.0"?>')
  202 format('<VTKFile type="RectilinearGrid" version="0.1" byte_order="LittleEndian">')
  302 format('</VTKFile>')
  203 format(2x,'<RectilinearGrid WholeExtent="',6I4,'">')
  303 format(2x,'</RectilinearGrid>')
  204 format(4x,'<Piece Extent="',6I4,'">')
  304 format(4x,'</Piece>')
  205 format(6x,'<CellData Scalars="cell_scalars" Normals="magnify">')
  305 format(6x,'</CellData>')
  206 format(8x,'<DataArray type="Float32" Name="magnify" NumberOfComponents="3" format="ascii">')
  207 format(8x,'<DataArray type="Float32" Name="cell_scalars" format="ascii">')
  399 format(8x,'</DataArray>')
  208 format(6x,'<PointData Scalars="colorful" Normals="direction">')
  308 format(6x,'</PointData>')
  209 format(8x,'<DataArray type="Float32" Name="colorful" format="ascii">')
  210 format(6x,'<Coordinates>')
  310 format(6x,'</Coordinates>')
  211 format(8x,'<DataArray type="Float32" format="ascii" RangeMin="0" RangeMax="5">')
  212 format(8x,'<DataArray type="Float32" format="ascii">')
  213 format(8x,'<DataArray type="Float32" Name="direction" NumberOfComponents="3" format="ascii">')
  501 format(10f9.5)
  502 format(10f9.5)
  503 format(5x,9(1x,3I2))
  504 format(10f9.5)
  505 format(5x,10(1x,3I2))
!!!!!
!
!  Begin writing out XML tags.
!
!!!!!
      write(*,201)                              !<?xml
      write(*,202)                              !VTKFile
        write(*,203)ix0,extentX,iy0,extentY,iz0,extentZ        !  RectilinearGrid
          write(*,204)ix0,extentX,iy0,extentY,iz0,extentZ      !    Piece
            write(*,205)                        !      CellData
              write(*,207)                      !        DataArray(cell_scalars)
      do j=1,imax-1
      write(*,501)(cell_scalar(i,j),i=1,imax-1)
      enddo
              write(*,399)                      !        /DataArray
              write(*,206)                      !        DataArray(cell_scalars)
      do j=1,imax-1
      write(*,503)(norm(i,1),norm(i,2),norm(i,3),i=1,imax-1)
      enddo
              write(*,399)                      !        /DataArray
            write(*,305)                        !      /CellData
            write(*,208)                        !      PointData
              write(*,209)                      !        DataArray(points)
      write(*,502)((point_scalar(i,j),i=1,imax),j=1,imax)
              write(*,399)                      !        /DataArray
              write(*,213)                      !        DataArray(cell_scalars)
      do j=1,imax
      write(*,505)(norm(i,1),norm(i,2),norm(i,3),i=1,imax)
      enddo
              write(*,399)                      !        /DataArray
            write(*,308)                        !      /PointData
            write(*,210)                        !      Coordinates
              write(*,212)                      !        DataArray(x-direction)
        write(*,504)(x(i),i=1,imax)
              write(*,399)                      !        /DataArray
              write(*,212)                      !        DataArray(y-direction)
        write(*,504)(y(i),i=1,imax)
              write(*,399)                      !        /DataArray
              write(*,212)                      !        DataArray(z-direction)
        write(*,504)z(1)
              write(*,399)                      !        /DataArray
            write(*,310)                        !      /Coordinates
          write(*,304)                          !    /Piece
        write(*,303)                            !  /RectilinearGrid
      write(*,302)                              !/VTKFile
      return
      end

Nonstandard Examination

In our introductory remarks, above, we said the warped free-energy surface drapes across a five-dimensional parameter "plane" such that,

<math>~\mathfrak{G}_{51}</math>

<math>~\mathfrak{G}(R, K_c, M_\mathrm{tot}, q, \nu) \, .</math>

From a more pragmatic point of view, we should have said that the "five-one" free-energy surface drapes across a five-dimensional parameter "plane" such that,

<math>~\mathfrak{G}_{51}</math>

<math>~\mathfrak{G}(R, K_c, M_\mathrm{tot}, \ell_i, \tfrac{\mu_e}{\mu_c}) \, .</math>

In our initial, standard examination of the structure of this warped free-energy surface, we held three parameters fixed — namely, <math>~K_c, M_\mathrm{tot}, \tfrac{\mu_e}{\mu_c}</math> — and plotted <math>~\mathfrak{G}_{51}(\ell_i, \Chi\equiv R/R_\mathrm{eq})</math>. Now, let's fix <math>~\Chi = 1</math> and plot <math>~\mathfrak{G}_{51}(\ell_i, \tfrac{\mu_e}{\mu_c})</math>. The following plot shows how a portion of the <math>~(\ell_i, \mu_e/\mu_c)</math> grid maps onto the traditional <math>~(q, \nu)</math> plane. The numerical labels identify lines of constant <math>~\xi_i = \sqrt{3}\ell_i</math> — 7 (light green), 9 (pink), and 12 (orange) — and lines of constant <math>~\mu_e/\mu_c</math> — 0.330 (purple), 0.315 (dark green), and 0.305 (white/blue).

User:Tohline/SSC/FreeEnergy/PolytropesEmbedded

Free-Energy Synopsis

Equilibrium Radii and Critical Radii

Examples

Pressure-Truncated Polytropes

Case M

Case P

Reconcile

Five-One Bipolytropes

Zero-Zero Bipolytropes

General Form

Compare with Five-One

Free-Energy of Truncated Polytropes

Case M Free-Energy Surface

Case P Free-Energy Surface

Free-Energy of Bipolytropes

Order of Magnitude Derivation

Equilibrium Radius

Order of Magnitude Estimate

Reconcile With Known Analytic Expression

Focus on Five-One Free-Energy Expression

Approximate Expressions

From Detailed Force-Balance Models

Gravitational Potential Energy of the Core

Thermal Energy of the Core

Gravitational Potential Energy of the Envelope

Thermal Energy of the Envelope

Combined in Equilibrium

Out of Equilibrium

Summary51

Focus on Zero-Zero Free-Energy Expression

From Detailed Force-Balance Models

Equilibrium Radius

First View

Second View

Gravitational Potential Energy

Internal Energy Components

First View

Second View

Summary00

Overview

BiPolytrope51

Key Analytic Expressions

Behavior of Equilibrium Sequence

Graphical Depiction of Free-Energy Surface

BiPolytrope00

Fortran Code

Nonstandard Examination

See Also

Navigation menu

Search