Difference between revisions of "User:Tohline/SSC/Stability/BiPolytropes"
| (277 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
__FORCETOC__ | __FORCETOC__ | ||
<!-- __NOTOC__ will force TOC off --> | <!-- __NOTOC__ will force TOC off --> | ||
=Marginally Unstable | =Marginally Unstable (n<sub>c</sub>,n<sub>e</sub>) = (5,1) Bipolytropes= | ||
<!-- Our aim is to determine whether or not there is a relationship between (1) equilibrium models at turning points along bipolytrope sequences and (2) bipolytropic models that are marginally (dynamically) unstable toward collapse (or dynamical expansion).--> | |||
{{LSU_HBook_header}} | {{LSU_HBook_header}} | ||
| Line 42: | Line 41: | ||
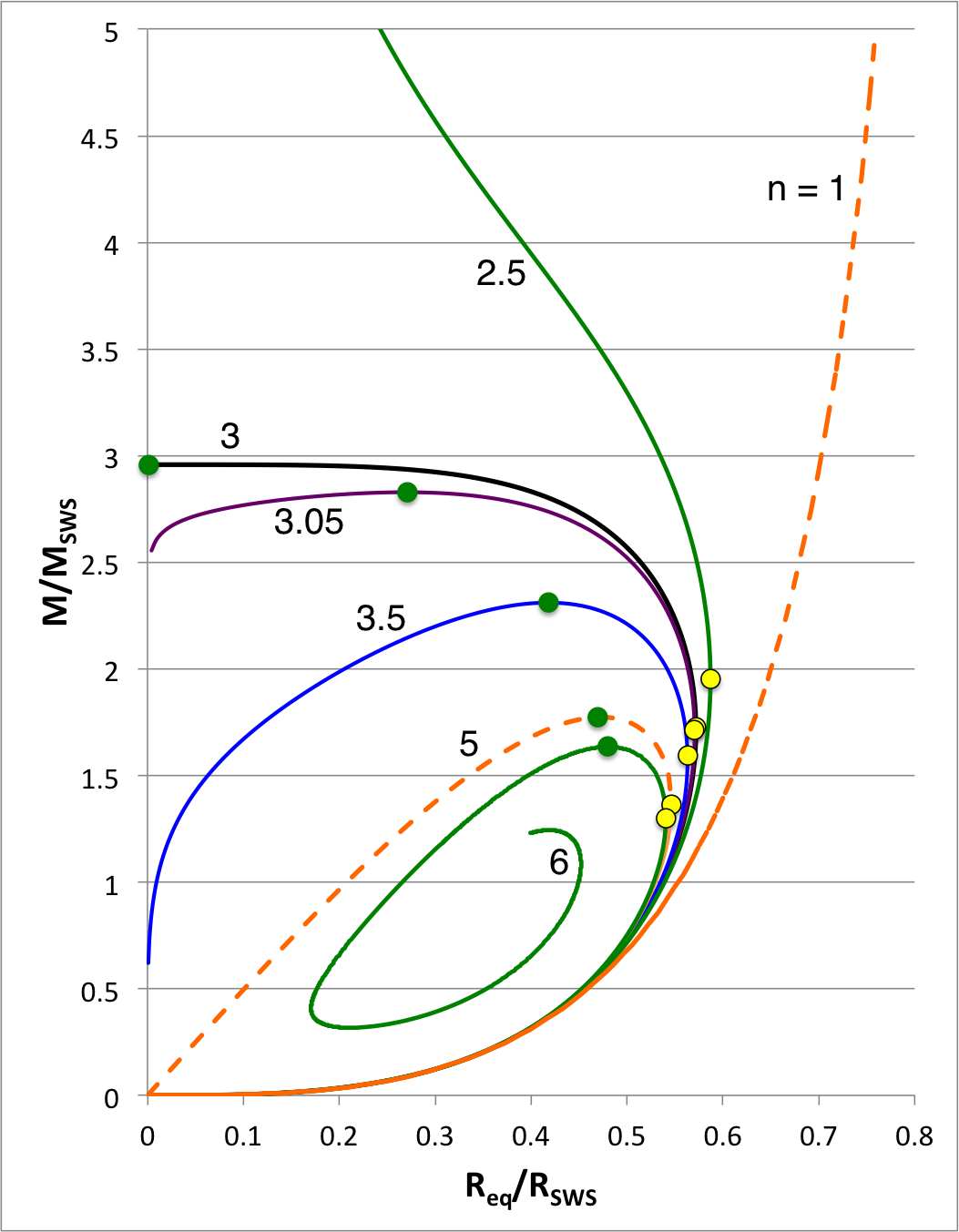
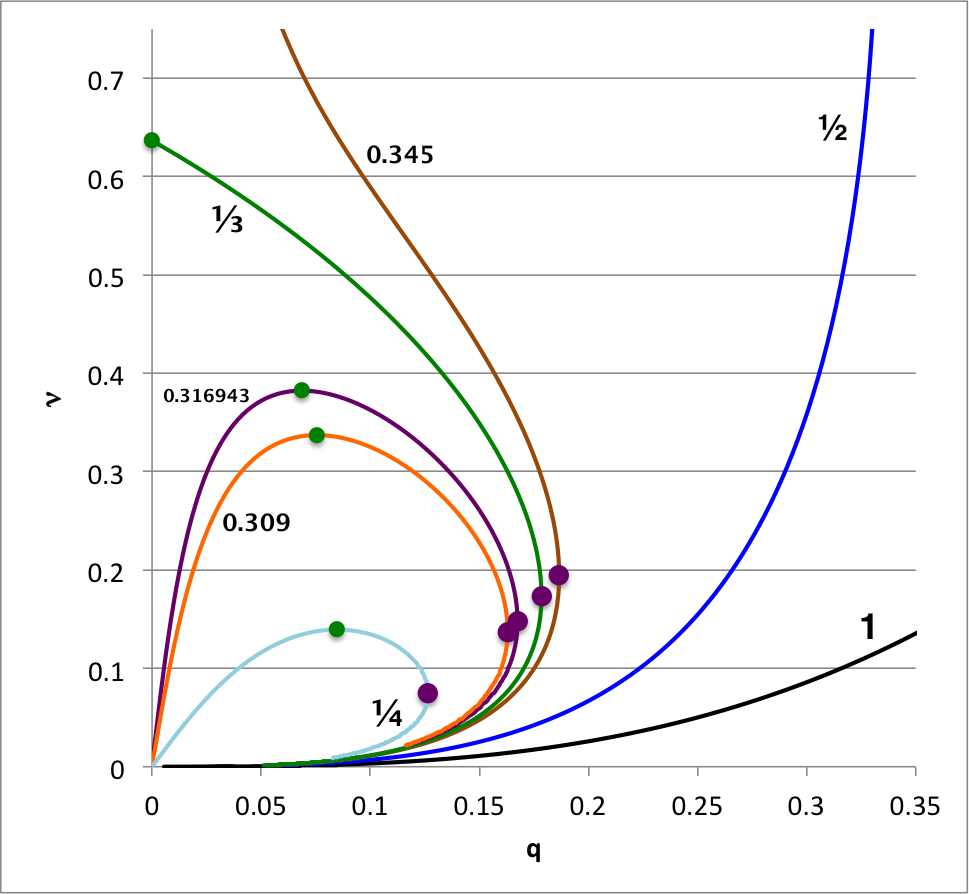
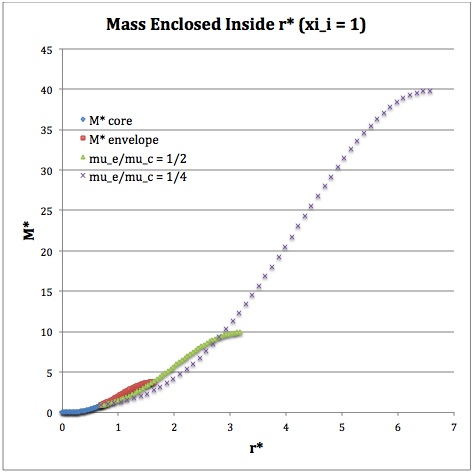
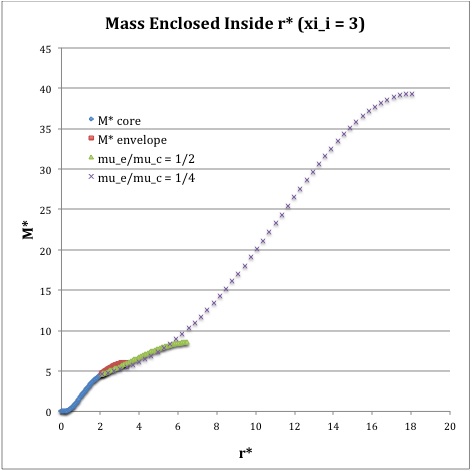
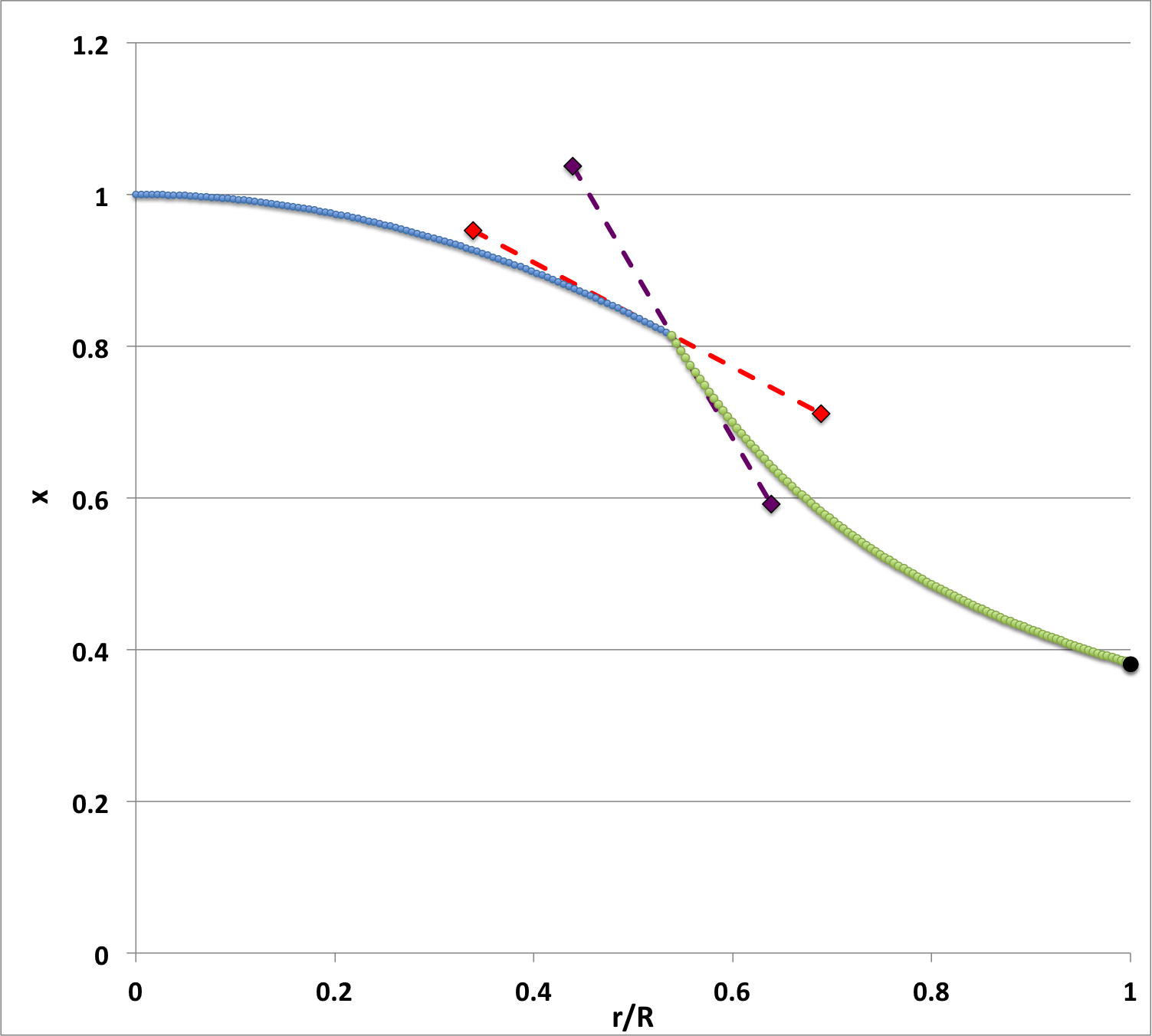
In another [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#BiPolytrope_with_nc_.3D_5_and_ne_.3D_1|accompanying chapter]], we have used purely analytic techniques to construct equilibrium sequences of spherically symmetric bipolytropes that have, <math>~(n_c,n_e) = (5,1)</math>. For a given choice of <math>~\mu_e/\mu_c</math> — the ratio of the mean-molecular weight of envelope material to the mean-molecular weight of material in the core — a physically relevant sequence of models can be constructed by steadily increasing the value of the dimensionless radius at the core/envelope interface, <math>~\xi_i</math>, from zero to infinity. Figure 2, | In another [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#BiPolytrope_with_nc_.3D_5_and_ne_.3D_1|accompanying chapter]], we have used purely analytic techniques to construct equilibrium sequences of spherically symmetric bipolytropes that have, <math>~(n_c,n_e) = (5,1)</math>. For a given choice of <math>~\mu_e/\mu_c</math> — the ratio of the mean-molecular weight of envelope material to the mean-molecular weight of material in the core — a physically relevant sequence of models can be constructed by steadily increasing the value of the dimensionless radius at the core/envelope interface, <math>~\xi_i</math>, from zero to infinity. Figure 2, whose content is essentially the same as [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Model_Sequences|Figure 1 of this separate chapter]], shows how the fractional core mass, <math>\nu \equiv M_\mathrm{core}/M_\mathrm{tot}</math>, varies with the fractional core radius, <math>q \equiv r_\mathrm{core}/R</math>, along sequences having seven different values of <math>~\mu_e/\mu_c</math>, as labeled: 1 (black), ½ (dark blue), 0.345 (brown), ⅓ (dark green), 0.316943 (purple), 0.309 (orange), and ¼ (light blue). | ||
When modeling bipolytropes, the default expectation is that an increase in <math>\xi_i</math> along a given sequence will correspond to an increase in the relative size — both the radius and the mass — of the core. | When modeling bipolytropes, the default expectation is that an increase in <math>~\xi_i</math> along a given sequence will correspond to an increase in the relative size — both the radius and the mass — of the core. This expectation is realized along the Figure 2 sequences that have the largest mean-molecular weight ratios: <math>~\mu_e/\mu_c</math> = 1 and ½. But the behavior is different along the other five illustrated sequences. For sufficiently large <math>~\xi_i</math>, the relative radius of the core begins to decrease; along each sequence, a solid purple circular marker identifies the location of this ''turning point'' in radius. Furthermore, along sequences for which <math>~\mu_e/\mu_c < \tfrac{1}{3}</math>, eventually the fractional mass of the core reaches a maximum and, thereafter, decreases even as the value of <math>~\xi_i</math> continues to increase; a solid green circular marker identifies the location of this ''maximum mass turning point'' along each of these sequences. (Additional properties of these equilibrium sequences are discussed in [[User:Tohline/SSC/FreeEnergy/PolytropesEmbedded#Behavior_of_Equilibrium_Sequence|yet another accompanying chapter]].) | ||
<font color="red">'''The principal question is:'''</font> ''Along bipolytropic sequences, are maximum-mass models associated with the onset of dynamical instabilities?'' | <font color="red">'''The principal question is:'''</font> ''Along bipolytropic sequences, are maximum-mass models associated with the onset of dynamical instabilities?'' | ||
| Line 50: | Line 49: | ||
==Planned Approach== | ==Planned Approach== | ||
<table border="0" cellpadding="5" width=" | <table border="0" cellpadding="5" width="30%" align="right"> | ||
<tr> | <tr> | ||
<th colspan="2" align="center">Figure 2: Equilibrium Sequences of Bipolytropes with <math>~(n_c,n_e) = (5,1)</math></th> | <th colspan="2" align="center">Figure 2: Equilibrium Sequences of Bipolytropes <br />with <math>~(n_c,n_e) = (5,1)</math> and Various <math>~\mu_e/\mu_c</math></th> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="center" colspan="2" bgcolor="white"> | <td align="center" colspan="2" bgcolor="white"> | ||
[[Image: | [[Image:TurningPoints51Bipolytropes.png|300px|center]] | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 77: | Line 76: | ||
** Solve the relevant eigenvalue problem for this specific model, initially for <math>~(\gamma_c, \gamma_e) = (6/5, 2)</math> and initially for the fundamental mode of oscillation. | ** Solve the relevant eigenvalue problem for this specific model, initially for <math>~(\gamma_c, \gamma_e) = (6/5, 2)</math> and initially for the fundamental mode of oscillation. | ||
= | =Virial Analysis= | ||
In an [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Stability_Condition|accompanying chapter]] we have examined the viral stability of <math>~(n_c, n_e) = (5, 1)</math> bipolytropes — see also an [[User:Tohline/SSC/Structure/BiPolytropes/FreeEnergy5_1#Free_Energy_of_BiPolytrope_with|associated discussion of the free-energy of these configurations]]. We present, here, an overview of this separate discussion in an effort to provide a broader perspective to the focus of this current chapter. | |||
==Various Energy Expressions== | |||
Drawing from our [[User:Tohline/SSC/Structure/BiPolytropes/FreeEnergy5_1#Free_Energy_of_BiPolytrope_with|accompanying detailed discussion]], we have, | |||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\mathfrak{G}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 115: | Line 93: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~W_\mathrm{grav}\biggr|_\mathrm{core} + \mathfrak{S}_A\biggr|_\mathrm{core} + W_\mathrm{grav}\biggr|_\mathrm{env} + \mathfrak{S}_A\biggr|_\mathrm{env} </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 132: | Line 105: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
~W_\mathrm{grav}\biggr|_\mathrm{core} + \biggl[ \frac{2}{3(\gamma_c - 1)} \biggr] S_\mathrm{core} + W_\mathrm{grav}\biggr|_\mathrm{env} + \biggl[ \frac{2}{3(\gamma_e - 1)} \biggr] S_\mathrm{env} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
Recognizing that, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~q</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 150: | Line 124: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{r_i}{R} = \frac{\eta_i}{\eta_s} \, ,</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 158: | Line 130: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\nu</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~\equiv</math> | ||
</td> | |||
<td align="left"> | |||
<math>~\frac{M_\mathrm{core}}{M_\mathrm{tot}} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\ell</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{\xi}{\sqrt{3}} \, ,</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 176: | Line 154: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\chi_\mathrm{eq} </math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 182: | Line 160: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl( \frac{\pi}{2^3 \cdot 3^6}\biggr)^{1 / 2} | ||
\ | \biggl( \frac{\nu}{q^3} \biggr)^{2} \biggl( 1 + \ell_i^2 \biggr)^{3} \biggl( \frac{q}{\ell_i}\biggr)^{5 } \, , | ||
\frac{ | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 191: | Line 167: | ||
</table> | </table> | ||
we have, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~- \chi \biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{core}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | |||
<math> | <math> | ||
\biggl[\biggl(\frac{3}{2^4}\biggr) \biggl( \frac{q}{\ell_i}\biggr)^{5}\biggl(\frac{\nu}{q^3} \biggr)^2 \biggl( 1 + \ell_i^2 \biggr)^{3} \biggr]_\mathrm{eq} | |||
\biggl[ | |||
\ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i | |||
\biggr] | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 208: | Line 189: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ \ | <math>~\Rightarrow ~~~ \biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{core}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | |||
<math> | <math>- \frac{\chi_\mathrm{eq}}{\chi} | ||
\biggl( \frac{ | \biggl( \frac{3^8}{2^5 \pi}\biggr)^{1 / 2} | ||
\biggl[ | |||
\frac{ | \ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i | ||
</math> | \biggr] \, . | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Next, given that, | |||
< | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~b_\eta = \eta_s</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| |||
and | |||
| |||
</td> | |||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~b_\eta q = \eta_i = 3\biggl( \frac{\mu_e}{\mu_c} \biggr) \ell_i (1 + \ell_i^2)^{-1} \, ,</math> | ||
- | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
we have, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | |||
<math> | <math> | ||
- \frac{\chi_\mathrm{eq}}{\chi} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 | |||
\biggl[6 b_\eta x - 3\sin[2(b_\eta x-B)] - 4b_\eta x \sin^2(b_\eta x - B) \biggr]_q^1 | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | |||
<math> | <math> | ||
- \frac{\chi_\mathrm{eq}}{\chi} | |||
\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 | |||
\biggl\{ | |||
\biggl[ 6 \eta_s - 3\sin[2(\eta_s -B)] - 4\eta_s \sin^2(\eta_s - B) \biggr] | |||
- | |||
\biggl[ 6 \eta_i - 3\sin[2(\eta_i -B)] - 4\eta_i \sin^2(\eta_i - B) \biggr] | |||
\biggr\} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
Also, given that, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~q a_\xi^{1 / 2}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 294: | Line 273: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{\xi_i}{\sqrt{3}} \, ,</math> | ||
\frac{ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
we have, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{core}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 318: | Line 288: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
- | \frac{2}{3({\gamma_c}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_c} | ||
\biggl\{\biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ | |||
\tan^{-1}[a_\xi^{1/2}q] - a_\xi^{1/2}q ~\frac{(1 - a_\xi q^2)}{(1 + a_\xi q^2)^2} \biggr] | |||
\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 332: | Line 305: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\frac{ 3\ | \frac{2}{3({\gamma_c}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_c} | ||
\biggl\{\biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ | |||
\tan^{-1}\biggl( \frac{\xi_i}{\sqrt{3}}\biggr) - \biggl( \frac{\xi_i}{\sqrt{3}}\biggr)\frac{(1 - \xi_i^2/3)}{(1 + \xi_i^2/3)^2} \biggr] | |||
\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 339: | Line 315: | ||
</table> | </table> | ||
Finally, then, we can write, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{env}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 350: | Line 328: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <td align="left"> | ||
\frac{ | <math> | ||
\frac{2}{3({\gamma_e}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_e} \biggl\{ | |||
\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl[ 2b_\eta x -\sin[2(b_\eta x - B)] \biggr]_q^1 \biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 366: | Line 344: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <td align="left"> | ||
\frac{ | <math> | ||
\frac{2}{3({\gamma_e}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_e} | |||
\bigg( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl\{ | |||
\biggl[ 2\eta_s -\sin[2(\eta_s - B)] \biggr] | |||
- | |||
\biggl[ 2\eta_i -\sin[2(\eta_i - B)] \biggr] | |||
\biggr\} \ . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
== | ==Free Energy and Its Derivatives== | ||
<table border="0" cellpadding="5" align="center"> | We can now rewrite the free-energy expression in the form, | ||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ \frac{ | <math>~\mathfrak{g} \equiv \frac{\mathfrak{G}}{E_\mathrm{norm}}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 389: | Line 373: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \biggl(\frac{\ | <math> | ||
~-\biggl(\frac{\chi_\mathrm{eq}}{\chi}\biggr)\mathfrak{w} | |||
+ \biggl[ \frac{2}{3(\gamma_c - 1)} \biggr] \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{3 - 3\gamma_c}\mathfrak{s}_\mathrm{core} | |||
+ \biggl[ \frac{2}{3(\gamma_e - 1)} \biggr] \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{3 - 3\gamma_e}\mathfrak{s}_\mathrm{env} | |||
\, , | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
where, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\mathfrak{s}_\mathrm{core}</math> | ||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ | ||
\tan^{-1}\biggl( \frac{\xi_i}{\sqrt{3}}\biggr) - \biggl( \frac{\xi_i}{\sqrt{3}}\biggr)\frac{(1 - \xi_i^2/3)}{(1 + \xi_i^2/3)^2} \biggr]\, , | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 410: | Line 403: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\mathfrak{s}_\mathrm{env}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\ | \bigg( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl\{ | ||
\biggl[ 2\eta_s -\sin[2(\eta_s - B)] \biggr] | |||
- | |||
\biggl[ 2\eta_i -\sin[2(\eta_i - B)] \biggr] | |||
\biggr\} \, , | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 424: | Line 421: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\mathfrak{w}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \biggl( \frac{3^8}{2^5 \pi}\biggr)^{1 / 2} | ||
\biggl[ | |||
\ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i | |||
\biggr] | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 441: | Line 441: | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\ | +~ | ||
\ | \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 | ||
\biggl\{ | |||
\biggl[ 6 \eta_s - 3\sin[2(\eta_s -B)] - 4\eta_s \sin^2(\eta_s - B) \biggr] | |||
- | |||
\biggl[ 6 \eta_i - 3\sin[2(\eta_i -B)] - 4\eta_i \sin^2(\eta_i - B) \biggr] | |||
\biggr\} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
The first derivative is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{d\mathfrak{g}}{d\chi} </math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 458: | Line 468: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
\frac{\ | +~\biggl(\frac{\chi_\mathrm{eq}}{\chi^2}\biggr)\mathfrak{w} | ||
~-~\frac{2}{\chi_\mathrm{eq}} \biggl[\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{2 - 3\gamma_c}\mathfrak{s}_\mathrm{core} | |||
~+~\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{2 - 3\gamma_e}\mathfrak{s}_\mathrm{env} \biggr] \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
And the second derivative is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{d^2\mathfrak{g}}{d\chi^2} </math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 471: | Line 487: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\biggl(\frac{\ | <math> | ||
\frac{\ | -~2\biggl(\frac{\chi_\mathrm{eq}}{\chi^3}\biggr)\mathfrak{w} | ||
~-~\frac{2}{\chi_\mathrm{eq}^2} \biggl[(2 - 3\gamma_c) \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{1 - 3\gamma_c}\mathfrak{s}_\mathrm{core} | |||
~+~(2 - 3\gamma_e)\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{1 - 3\gamma_e}\mathfrak{s}_\mathrm{env} \biggr] \ . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
==What to Expect for Equilibrium Configurations== | |||
In equilibrium we should set <math>~d\mathfrak{g}/d\chi = 0</math> and <math>~\chi = \chi_\mathrm{eq}</math>. In which case we should expect to find that, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | <math>~0 </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 491: | Line 508: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math> | <math> | ||
\frac{ | \frac{1}{\chi_\mathrm{eq}}\biggl[\mathfrak{w} | ||
~-~2(\mathfrak{s}_\mathrm{core} | |||
~+~\mathfrak{s}_\mathrm{env}) \biggr] \, . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
In addition, an evaluation of the second derivative should give, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{\chi_\mathrm{eq}^2}{2} \cdot \frac{d^2\mathfrak{g}}{d\chi^2} </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \ | <math> | ||
-~\mathfrak{w} | |||
~-~ \biggl[(2 - 3\gamma_c) \mathfrak{s}_\mathrm{core} | |||
~+~(2 - 3\gamma_e)\mathfrak{s}_\mathrm{env} \biggr] \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Then, the transition from stable to unstable configurations occurs when <math>~d^2\mathfrak{g}/d\chi^2 = 0</math>, that is, when, | |||
</math> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\mathfrak{w} </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 536: | Line 546: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
- \ | ~-~ \biggl[(2 - 3\gamma_c) \mathfrak{s}_\mathrm{core} | ||
~+~(2 - 3\gamma_e)\mathfrak{s}_\mathrm{env} \biggr] | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 544: | Line 555: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~0</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 550: | Line 561: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
~(4 - 3\gamma_c) \mathfrak{s}_\mathrm{core} | |||
~+~(4 - 3\gamma_e)\mathfrak{s}_\mathrm{env} | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow ~~~\frac{\mathfrak{s}_\mathrm{core} }{\mathfrak{s}_\mathrm{env}} </math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 569: | Line 576: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
\frac{ | ~-~\frac{(4 - 3\gamma_e) }{(4 - 3\gamma_c) } \, . | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
For example, if we set <math>~\gamma_c = 6/5</math> and <math>~\gamma_e = 2</math>, this implies, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\mathfrak{s}_\mathrm{core} }{\mathfrak{s}_\mathrm{env}} </math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 585: | Line 594: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math> | ||
~\frac{2 }{(4 - 18/5) } = 5 \, . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
==Free-Energy Stability Evaluation== | |||
Here we pull together excerpts from several different [[User:Tohline/H_BookTiledMenu#Tiled_Menu|H_Book Chapters]] in which we have presented, from several different perspectives, an analysis of the free-energy of bipolytropes. | |||
* In [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#BiPolytrope_with_nc_.3D_5_and_ne_.3D_1|one chapter]], using purely analytic techniques, we have derived expressions that detail the structural properties of bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>. Among these are analytic expressions for various terms that make up the free-energy expression: <math>~\mathfrak{s}_\mathrm{core}</math>, <math>~\mathfrak{s}_\mathrm{env}</math>, <math>~\mathfrak{w}_\mathrm{core}</math>, <math>~\mathfrak{w}_\mathrm{core}</math>, and <math>~P_iV_\mathrm{core}</math>. Equilibrium model ''sequences'' are defined by fixing the ratio, <math>~\mu_e/\mu_c</math>, then varying the radial location, <math>~r_i</math>, of the core-envelope interface; note that the volume of the core is, then, <math>~V_\mathrm{core} \equiv 4\pi r_i^3/3</math>. | |||
<div align="left"> | |||
* <font color="red">[Virial Equilibrium]</font> In a subsection of this same chapter titled, ''[[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Global|Equilibrium Condition: Global]]'', we have shown that a statement of virial equilibrium — obtained by setting the first derivative of the free-energy expression to zero — is,<table border="0" cellpadding="4" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~( 2S + W )_\mathrm{tot} ~=~ 2(S_\mathrm{core} + S_\mathrm{env}) + (W_\mathrm{core} + W_\mathrm{env})</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=~</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ 0 \, .</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table>In another subsection of this same chapter titled, ''[[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Global|Equilibrium Condition: In Parts]]'', we showed that for each bipolytropic equilibrium structure, the statements | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~2S_\mathrm{core} + W_\mathrm{core} = 3P_i V_\mathrm{core}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
and | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~2S_\mathrm{env} + W_\mathrm{env} = - 3P_i V_\mathrm{core} \, ,</math> | ||
\ | |||
+ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table>also hold separately. Therefore, for every equilibrium configuration we should expect the <b>CASE1</b> expression (see Table XXX) to precisely sum to unity. | ||
</div> | |||
<div | |||
<div align="left"> | |||
* <font color="red">[Marginally Unstable Model]</font> Near the bottom of this same chapter, in a subsection titled, ''[[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Stability_Condition|Stability Condition]]'', we point out that the model along each sequence that is marginally (dynamically) unstable — obtained setting the second derivative of the free-energy expression to zero — is identified by the configuration for which,<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~ | ||
2(\gamma_e - \gamma_c) \mathfrak{s}_\mathrm{core} + ( \mathfrak{w}_\mathrm{core} + \mathfrak{w}_\mathrm{env} )(\gamma_e - \tfrac{4}{3}) | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 644: | Line 647: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~0 \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table>Therefore, along each equilibrium sequence, the marginally unstable model can be identified by the configuration for which the <b>CASE2</b> expression (see Table XXX) precisely sums to zero. Immediately above, in a subsection titled, ''[[User:Tohline/SSC/Stability/BiPolytropes#What_to_Expect_for_Equilibrium_Configurations|What to Expect for Equilibrium Configurations]]'', we have shown that this same marginally unstable model can be identified by the configuration for which the <b>CASE3</b> energy ratio, <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env}</math>, has a value that is precisely 5. And, thirdly, as highlighted in our accompanying [[User:Tohline/SSC/Synopsis_StyleSheet#Bipolytropes|Tabular Overview]], this same marginally unstable model can be identified by the configuration for which the <b>CASE4</b> expression precisely sums to zero. | ||
</div> | </div> | ||
<div align="center"> | <div align="center"> | ||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<th align="center" colspan="16"> | |||
'''Table XXX:''' Properties of Marginally Unstable Bipolytropes Having<br /><br /><math>~(n_c, n_e) = (5, 1)</math> and <math>~(\gamma_c, \gamma_e) = (\tfrac{6}{5}, 2)</math><br /><br />Determined from Free-Energy Arguments | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\frac{\mu_e}{\mu_c}</math></td> | |||
<td align="center"><math>~\xi_i</math></td> | |||
<td align="center"><math>~R^*_\mathrm{surf}</math></td> | |||
<td align="center"><math>~q \equiv \frac{r_\mathrm{core}}{R_\mathrm{surf}}</math></td> | |||
<td align="center"><math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math></td> | |||
<td align="center"><math>~\frac{\rho_c}{\bar\rho}</math></td> | |||
<td align="center"><math>~P_i</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{env}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{env}</math></td> | |||
<td align="center"><math>~3P_i V_\mathrm{core}</math></td> | |||
<td align="center"><b>CASE1</b></td> | |||
<td align="center"><b>CASE2</b></td> | |||
<td align="center"><b>CASE3</b></td> | |||
<td align="center"><b>CASE4</b></td> | |||
</tr> | |||
<tr> | |||
<td align="center">1</td> | |||
<td align="left">2.41610822</td> | |||
<td align="right">2.8049</td> | |||
<td align="center">0.59520261</td> | |||
<td align="center">0.68306067</td> | |||
<td align="center">16.3788</td> | |||
<td align="center">0.039116848</td> | |||
<td align="center">4.446748782</td> | |||
<td align="center">- 6.606135366</td> | |||
<td align="center">0.889349762</td> | |||
<td align="center">- 4.066061722</td> | |||
<td align="center">2.287362198</td> | |||
<td align="center">1</td> | |||
<td align="center">0</td> | |||
<td align="center">5.0000000</td> | |||
<td align="center">2.1 × 10<sup>-8</sup></td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{2}</math></td> | |||
<td align="left">4.1853093</td> | |||
<td align="right">8.8058</td> | |||
<td align="center">0.328419479</td> | |||
<td align="center">0.70131896</td> | |||
<td align="center">354.089</td> | |||
<td align="center">0.003126324</td> | |||
<td align="center">5.76978580</td> | |||
<td align="center">- 10.58931853</td> | |||
<td align="center">1.153956968</td> | |||
<td align="center">- 3.258165567</td> | |||
<td align="center">0.95025163</td> | |||
<td align="center">1</td> | |||
<td align="center">6.3 × 10<sup>-8</sup></td> | |||
<td align="center">5.0000002</td> | |||
<td align="center">0</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.345</td> | |||
<td align="left">7.64325</td> | |||
<td align="right">44.116</td> | |||
<td align="center">0.119714454</td> | |||
<td align="center">0.52700045</td> | |||
<td align="center">2.85 × 10<sup>4</sup></td> | |||
<td align="center">0.000116533</td> | |||
<td align="center">6.230343527</td> | |||
<td align="center">- 12.24495934</td> | |||
<td align="center">1.1246068658</td> | |||
<td align="center">- 2.707865028</td> | |||
<td align="center">0.215727713</td> | |||
<td align="center">1</td> | |||
<td align="center">6.4 × 10<sup>-8</sup></td> | |||
<td align="center">5.0000002</td> | |||
<td align="center">0</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{3}</math></td> | |||
<td align="left">8.548103</td> | |||
<td align="right">59.643</td> | |||
<td align="center">0.099032423</td> | |||
<td align="center">0.47901529</td> | |||
<td align="center">6.30 × 10<sup>4</sup></td> | |||
<td align="center">6.1337 × 10<sup>-5</sup></td> | |||
<td align="center">6.261548334</td> | |||
<td align="center">- 12.36425897</td> | |||
<td align="center">1.252309682</td> | |||
<td align="center">- 2.663457063</td> | |||
<td align="center">0.158837699</td> | |||
<td align="center">1</td> | |||
<td align="center">0</td> | |||
<td align="center">4.9999999</td> | |||
<td align="center">6.0 × 10<sup>-8</sup></td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.316943</td> | |||
<td align="left">10.7441565</td> | |||
<td align="right">108.14</td> | |||
<td align="center">0.068655205</td> | |||
<td align="center">0.38238387</td> | |||
<td align="center">2.93 × 10<sup>5</sup></td> | |||
<td align="center">1.6252 × 10<sup>-5</sup></td> | |||
<td align="center">6.301810768</td> | |||
<td align="center">- 12.52005323</td> | |||
<td align="center">1.260362204</td> | |||
<td align="center">- 2.604292714</td> | |||
<td align="center">0.083568307</td> | |||
<td align="center">1</td> | |||
<td align="center">0</td> | |||
<td align="center">4.9999998</td> | |||
<td align="center">2.0 × 10<sup>-7</sup></td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.309</td> | |||
<td align="left">12.77156</td> | |||
<td align="right">166.06</td> | |||
<td align="center">0.053145011</td> | |||
<td align="center">0.31696879</td> | |||
<td align="center">8.70 × 10<sup>5</sup></td> | |||
<td align="center">5.8905 × 10<sup>-6</sup></td> | |||
<td align="center">6.318902171</td> | |||
<td align="center">- 12.58692884</td> | |||
<td align="center">1.26378042</td> | |||
<td align="center">- 2.57843634</td> | |||
<td align="center">0.050875500</td> | |||
<td align="center">1</td> | |||
<td align="center">1.9 × 10<sup>-8</sup></td> | |||
<td align="center">5.0000001</td> | |||
<td align="center">0</td> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="16"> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
< | <b>CASE1</b> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~ | ||
\frac{1}{6P_i V_\mathrm{core}}\biggl[ | |||
(2 \mathfrak{s}_\mathrm{core} + \mathfrak{w}_\mathrm{core}) | |||
-(2 \mathfrak{s}_\mathrm{core} + \mathfrak{w}_\mathrm{core}) | |||
\biggr] | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 668: | Line 810: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<b>CASE2</b> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\gamma_e \ | <math>~ | ||
2(\gamma_e - \gamma_c) \mathfrak{s}_\mathrm{core} + ( \mathfrak{w}_\mathrm{core} + \mathfrak{w}_\mathrm{env} )(\gamma_e - \tfrac{4}{3}) | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 680: | Line 824: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
< | <b>CASE3</b> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\mathfrak{s}_\mathrm{core}}{\mathfrak{s}_\mathrm{env}} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
< | <b>CASE4</b> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 726: | Line 845: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
4\ | (4 - 3\gamma_c)\mathfrak{w}_\mathrm{core} + (4 - 3\gamma_e)\mathfrak{w}_\mathrm{env} + 3^2(\gamma_c - \gamma_e)P_i V_\mathrm{core} | ||
\ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
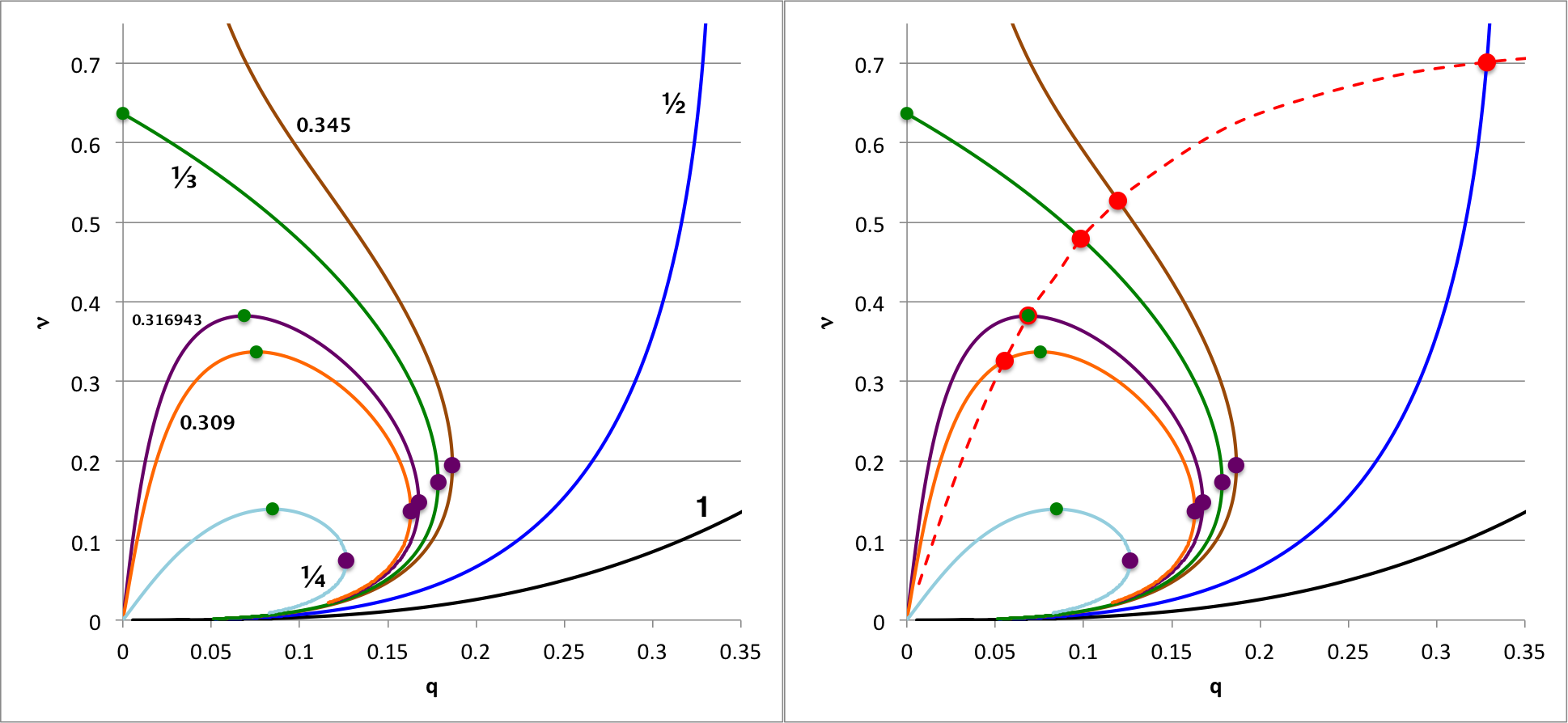
The left-hand panel of Figure 3 is identical to Figure 2, above. It displays in the <math>~q-\nu</math> parameter space, the behavior of <math>~(n_c, n_e) = (5, 1)</math> bipolytropic equilibrium sequences that have, as labeled, seven different values of the ratio of mean-molecular-weights, <math>~\mu_e/\mu_c</math>. Using a numerical root-finding technique, we have determined where the virial stability condition, <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env} = 5</math>, is satisfied along each of these sequences — as well as along a number of additional equilibrium sequences. Key properties of each of these identified models have been recorded in [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Stability_Condition|Table 1 of an accompanying discussion]]; see also an [[User:Tohline/SSC/Structure/BiPolytropes/FreeEnergy5_1#Free_Energy_of_BiPolytrope_with|associated discussion of the free-energy of these configurations]]. Pulling from this tabulated data, the solid-red circular markers that appear in the right-hand panel of Figure 3 identify where this virial stability condition is satisfied along the separate equilibrium sequences while the accompanying dashed red curve identifies more broadly how the <math>~q-\nu</math> parameter space is divided into stable (below and to the right) versus unstable (above and to the left) regions. | |||
<table border="0" align="center" cellpadding="8"> | |||
<tr> | |||
<th align="center">Figure 3</th> | |||
</tr> | |||
<tr> | <tr> | ||
<td align="center"> | <td align="center"> | ||
[[File:CompositeAlabeled.png|800px|dynamical stability in qNu plane]] | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
In what follows we use a complementary — and more quantitatively rigorous — approach to evaluating the stability of equilibrium models, and contrast the results of that analysis with the virial-analysis results presented graphically here in Figure 3. | |||
=Review of the Analysis by Murphy & Fiedler (1985b)= | |||
As we have [[User:Tohline/SSC/Stability/Polytropes#Boundary_Conditions|detailed separately]], the boundary condition at the center of a polytropic configuration is, | |||
<div align="center"> | |||
<math>~\frac{dx}{d\xi} \biggr|_{\xi=0} = 0 \, ;</math> | |||
</div> | |||
and the boundary condition at the surface of an isolated polytropic configuration is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{d\ln x}{d\ln\xi}</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~- \alpha + \frac{\omega^2}{\gamma_g } \biggl( \frac{1}{4\pi G \rho_c } \biggr) \frac{\xi}{(-\theta^')} </math> | ||
at <math>~\xi = \xi_s \, .</math> | |||
\ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
But this surface condition is not applicable to bipolytropes. Instead, let's return to the [[User:Tohline/SSC/Perturbations#Ensure_Finite-Amplitude_Fluctuations|original, more general expression of the surface boundary condition]]: | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ \frac{d\ln x}{d\ln\xi}\biggr|_s</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~- \alpha + \frac{\omega^2 R^3}{\gamma_g GM_\mathrm{tot}} \, .</math> | ||
\ | |||
\gamma_g | |||
\, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
<div id="SurfaceCondition"> | |||
< | <table border="1" align="center" width="85%" cellpadding="10"><tr><td align="left"> | ||
Utilizing an [[User:Tohline/SSC/Stability/Polytropes#Groundwork|accompanying discussion]], let's examine the frequency normalization used by [http://adsabs.harvard.edu/abs/1985PASAu...6..222M Murphy & Fiedler (1985b)] (see the top of the left-hand column on p. 223): | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Omega^2</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\omega^2 \biggl[ \frac{R^3}{GM_\mathrm{tot}} \biggr] | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{\ | <math>~ | ||
\omega^2 \biggl[ \frac{3}{4\pi G \bar\rho} \biggr] | |||
= | |||
\omega^2 \biggl[ \frac{3}{4\pi G \rho_c} \biggr] \frac{\rho_c}{\bar\rho} | |||
= | |||
\frac{3\omega^2}{(n_c+1)} \biggl[ \frac{(n_c+1)}{4\pi G \rho_c} \biggr] \frac{\rho_c}{\bar\rho} | |||
</math> | |||
</td> | </td> | ||
</tr> | |||
<tr> | |||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 831: | Line 956: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~( | <math>~ | ||
\frac{3\omega^2}{(n_c+1)} \biggl[ \frac{a_n^2\rho_c}{P_c} \cdot \theta_c \biggr] \frac{\rho_c}{\bar\rho} | |||
= | |||
\frac{3\gamma}{(n_c+1)} \frac{\rho_c}{\bar\rho} \biggl[ \frac{a_n^2\rho_c}{P_c} \cdot \frac{\omega^2 \theta_c}{\gamma} \biggr] \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
For a given radial quantum number, <math>~k</math>, the factor inside the square brackets in this last expression is what [http://adsabs.harvard.edu/abs/1985PASAu...6..222M Murphy & Fiedler (1985b)] refer to as <math>~\omega^2_k \theta_c</math>. Keep in mind, as well, that, in the notation we are using, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\sigma_c^2</math> | ||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{3\omega^2}{2\pi G \rho_c}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ \sigma_c^2</math> | |||
</td> | |||
<tr> | |||
<td align="right"> | |||
<math>~\ | |||
</td> | |||
<td align="center"> | <td align="center"> | ||
<math>~=</math> | <math>~=</math> | ||
| Line 881: | Line 989: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\ | \biggl( \frac{2\bar\rho}{\rho_c}\biggr) \Omega^2 | ||
= | |||
\frac{6\gamma}{(n_c+1)} \biggl[ \frac{a_n^2\rho_c}{P_c} \cdot \frac{\omega^2 \theta_c}{\gamma} \biggr] | |||
= | = | ||
\frac{ | \frac{6\gamma}{(n_c+1)} \biggl[ \omega_k^2 \theta_c \biggr] \, . | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
This also means that the surface boundary condition may be rewritten as, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~ \frac{d\ln x}{d\ln\xi}\biggr|_s</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 896: | Line 1,010: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{\Omega^2}{\gamma_g } - \alpha \, .</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</td></tr></table> | |||
</div> | |||
Let's apply these relations to the core and envelope, separately. | |||
==Interface Conditions== | |||
Here, we will simply copy the discussion already provided in the context of our attempt to analyze the stability of <math>~(n_c, n_e) = (0, 0)</math> bipolytropes; specifically, we will draw from [[User:Tohline/SSC/Stability/BiPolytrope0_0#Piecing_Together|<font color="red">'''STEP 4:'''</font> in the ''Piecing Together'' subsection]]. Following the discussion in §§57 & 58 of [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)], the proper treatment is to ensure that fractional perturbation in the gas pressure (see their equation 57.31), | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{\delta P}{P}</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~- \gamma x \biggl( 3 + \frac{d\ln x}{d\ln \xi} \biggr) \, ,</math> | ||
\biggl | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
is continuous across the interface. That is to say, at the interface <math>~(\xi = \xi_i)</math>, we need to enforce the relation, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 936: | Line 1,051: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\biggl[ \gamma_c x_\mathrm{core} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \gamma_e x_\mathrm{env} \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=\xi_i}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 942: | Line 1,057: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 950: | Line 1,063: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\gamma_e \biggl[ \frac{\gamma_c}{\gamma_e} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=\xi_i}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 957: | Line 1,069: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow~~~ \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=\xi_i}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 964: | Line 1,075: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr)_{\xi=\xi_i} \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
< | In the context of this interface-matching constraint (see their equation 62.1), [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)] state the following: <font color="darkgreen"><b>In the static</b></font> (''i.e.,'' unperturbed equilibrium) <font color="darkgreen"><b>model</b></font> … <font color="darkgreen"><b>discontinuities in <math>~\rho</math> or in <math>~\gamma</math> might occur at some [radius]</b></font>. <font color="darkgreen"><b>In the first case</b></font> — that is, a discontinuity only in density, while <math>~\gamma_e = \gamma_c</math> — the interface conditions <font color="darkgreen"><b>imply the continuity of <math>~\tfrac{1}{x} \cdot \tfrac{dx}{d\xi}</math> at that [radius]. In the second case</b></font> — that is, a discontinuity in the adiabatic exponent — <font color="darkgreen"><b>the dynamical condition may be written</b></font> as above. <font color="darkgreen"><b>This implies a discontinuity of the first derivative at any discontinuity of <math>~\gamma</math></b></font>. | ||
The algorithm that [http://adsabs.harvard.edu/abs/1985PASAu...6..222M Murphy & Fiedler (1985b)] used to "<font color="#007700">… [integrate] through each zone …</font>" was designed "<font color="#007700">… with continuity in <math>~x</math> and <math>~dx/d\xi</math> being imposed at the interface …</font>" Given that they set <math>~\gamma_c = \gamma_e = 5/3</math>, their interface matching condition is consistent with the one prescribed by [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)]. | |||
=Radial Oscillations of (n<sub>c</sub>, n<sub>e</sub>) = (5, 1) Models= | |||
==Foundation== | |||
In an [[User:Tohline/SSC/Perturbations#2ndOrderODE|accompanying discussion]], we derived the so-called, | |||
<div align="center" id="2ndOrderODE"> | |||
<font color="#770000">'''Adiabatic Wave''' (or ''Radial Pulsation'') '''Equation'''</font><br /> | |||
{{User:Tohline/Math/EQ_RadialPulsation01}} | |||
</div> | |||
whose solution gives eigenfunctions that describe various radial modes of oscillation in spherically symmetric, self-gravitating fluid configurations. Assuming that the underlying equilibrium structure is that of a bipolytrope having <math>~(n_c, n_e) = (5, 1)</math>, it makes sense to adopt the normalizations used when defining the equilibrium structure, namely, | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\ | <math>~\rho^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~\ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{\rho_0}{\rho_c}</math> | ||
\frac{\ | |||
</math> | |||
</td> | </td> | ||
<td align="center">; </td> | |||
<td align="right"> | <td align="right"> | ||
<math>~r^*</math> | <math>~r^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~\equiv</math> | |||
<math>~\ | |||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{r_0}{[K_c^{1/2}/(G^{1/2}\rho_c^{2/5})]}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,013: | Line 1,123: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~P^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{P_0}{K_c\rho_c^{6/5}}</math> | ||
</td> | </td> | ||
< | <td align="center">; </td> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~M_r^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{M_r}{[K_c^{3/2}/(G^{3/2}\rho_c^{1/5})]}</math> | ||
\frac{ | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
We | We note as well that, | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~g_0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,060: | Line 1,159: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{GM(r_0)}{r_0^2}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,076: | Line 1,171: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
G \biggl[ M_r^* \rho_c^{-1 / 5} \biggl( \frac{K_c}{G}\biggr)^{3 / 2} \biggr] \biggl[ r^* \rho_c^{-2 / 5}\biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr]^{-2} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,082: | Line 1,179: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,088: | Line 1,185: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Hence, multiplying the LAWE through by <math>~(K_c/G)\rho_c^{-4 / 5}</math> gives, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} | ||
+ \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,137: | Line 1,225: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{d^2x}{dr*^2} + \biggl[\frac{4}{r^*} ~-~ \rho_c^{-2 / 5} \biggl( \frac{K_c}{G} \biggr)^{1 / 2}\biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr*} | ||
~- | ~+~ \rho_c^{-4 / 5}\biggl( \frac{K_c}{G} \biggr)\biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 ~+~ (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x | ||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,145: | Line 1,233: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\ | \frac{d^2x}{dr*^2} ~+~ \biggl\{\frac{4}{r^*} ~-~ \rho_c^{-2 / 5} \biggl( \frac{K_c}{G} \biggr)^{1 / 2} \frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \biggl[ \frac{\rho_c \rho^*}{P^* K_c \rho_c^{6/5}}\biggr] \biggr\} \frac{dx}{dr*} | ||
~+~ \rho_c^{-4 / 5}\biggl( \frac{K_c}{G} \biggr)\biggl[ \frac{\rho_c \rho^*}{\gamma_\mathrm{g}P^* K_c \rho_c^{6/5}}\biggr] | |||
\biggl\{\omega^2 ~+~ (4 - 3\gamma_\mathrm{g}) \frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \frac{\rho_c^{2 / 5}}{r^*}\biggl( \frac{G}{K_c}\biggr)^{1 / 2} \biggr\} x | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,166: | Line 1,256: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{d^2x}{dr*^2} ~+~ \biggl\{\frac{4}{r^*} ~-~ \frac{M_r^*}{(r^*)^2} \biggl[ \frac{\rho^*}{P^* }\biggr] \biggr\} \frac{dx}{dr*} | ||
~+~ \biggl( \frac{1}{G\rho_c} \biggr)\biggl[ \frac{ \rho^*}{\gamma_\mathrm{g}P^* }\biggr] | |||
\biggl\{\omega^2 ~+~ (4 - 3\gamma_\mathrm{g}) \frac{G\rho_c M_r^*}{(r^*)^3} \biggr\} x | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,180: | Line 1,272: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{d^2x}{dr*^2} + \biggl\{ \frac{4}{r^*} -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)^2}\biggr\} \frac{dx}{dr*} | ||
\frac{ | + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{\omega^2}{\gamma_\mathrm{g} G\rho_c} + \biggl(\frac{4}{\gamma_\mathrm{g}} - 3\biggr)\frac{ M_r^*}{(r^*)^3}\biggr\} x | ||
\biggl | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,196: | Line 1,287: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | \frac{d^2x}{dr*^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{r^*} \frac{dx}{dr*} | ||
\biggl | + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . | ||
\, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,204: | Line 1,294: | ||
</table> | </table> | ||
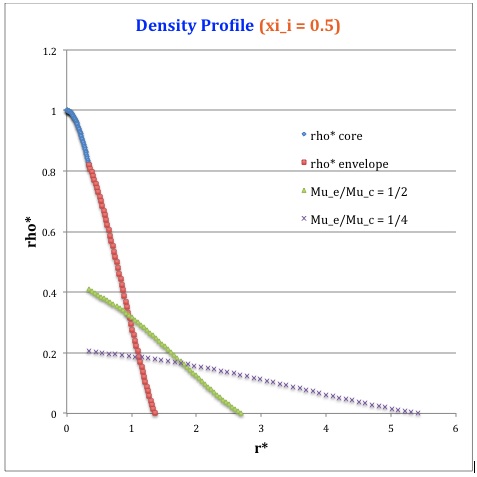
==Profile== | |||
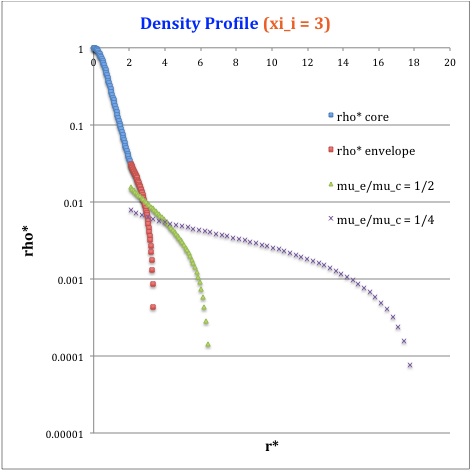
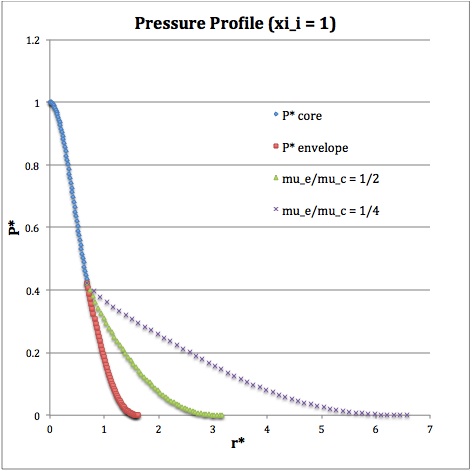
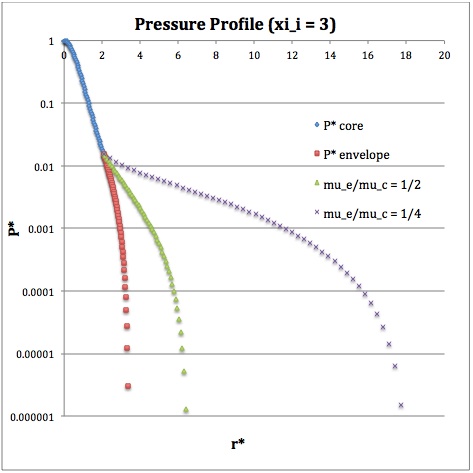
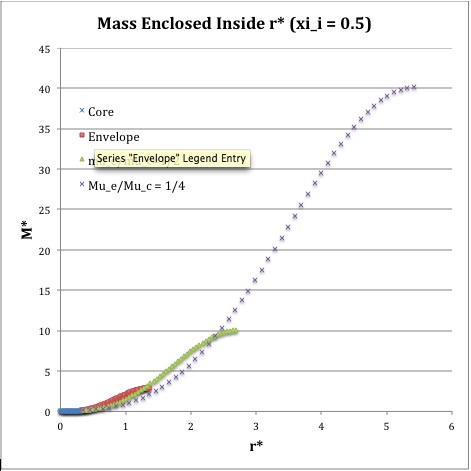
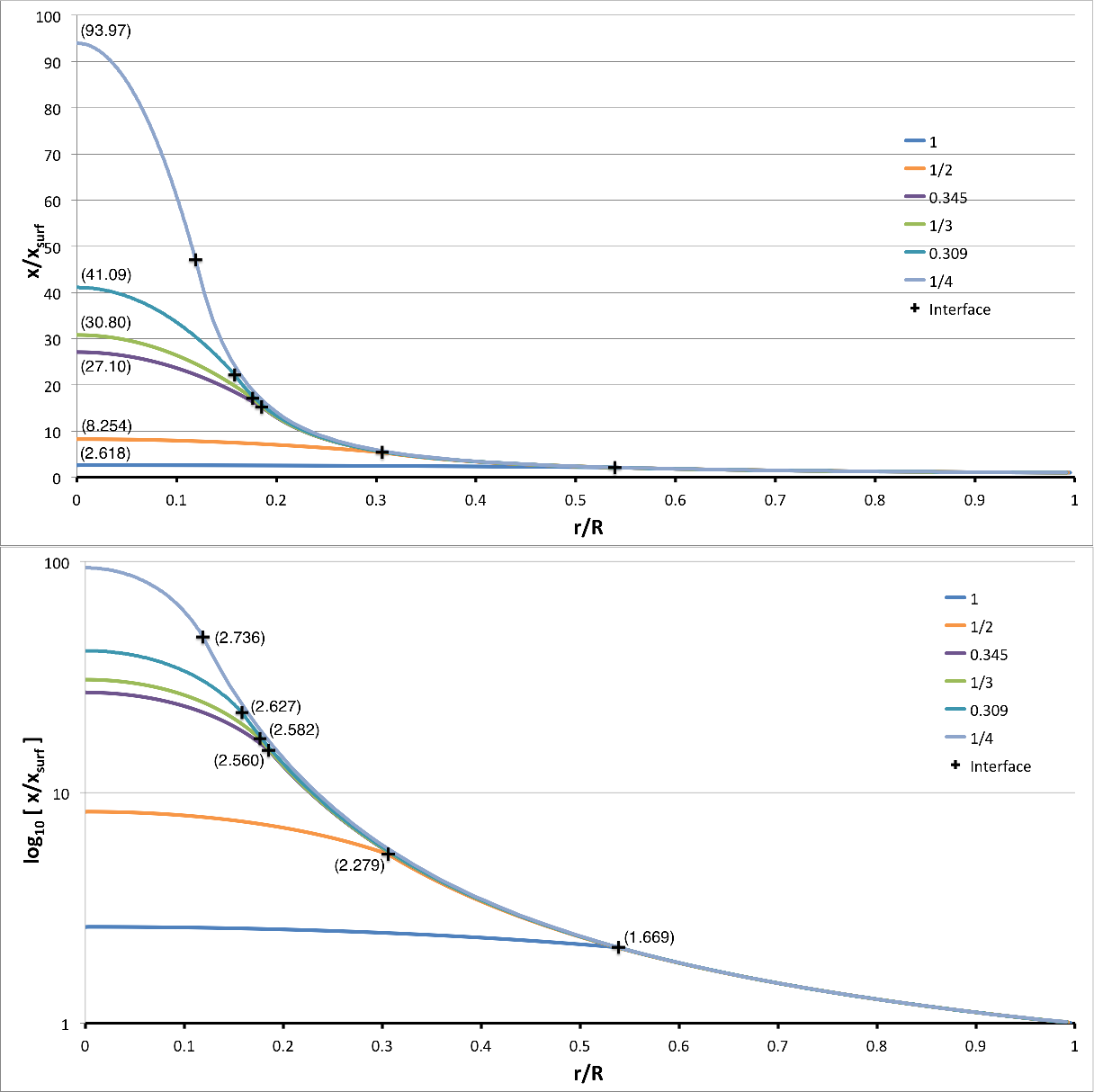
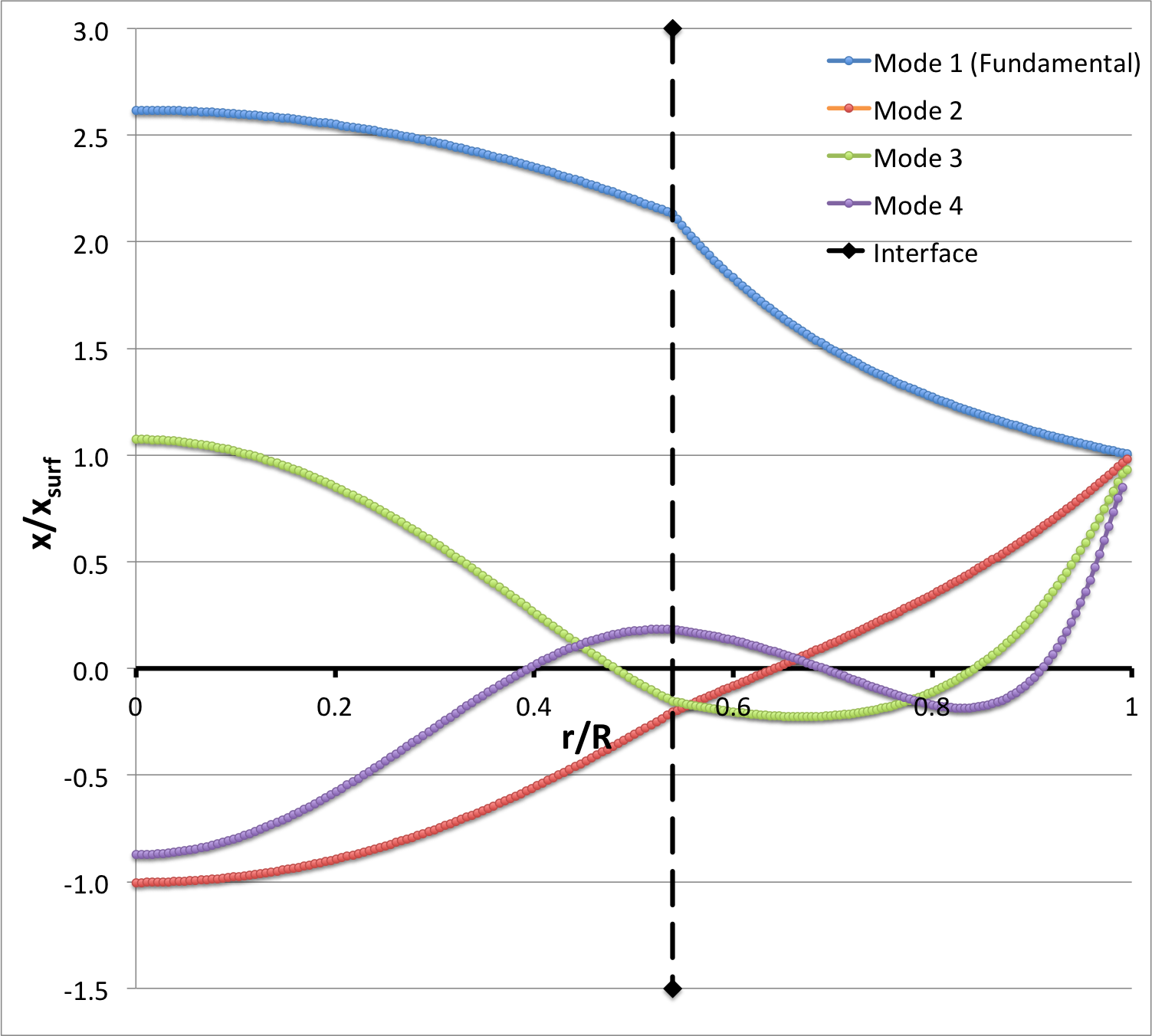
Now, referencing the [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Profile|derived bipolytropic model profile]], we should incorporate the following relations: | |||
<div align="center"> | |||
<b>Table 1: Radial Profile of Various Physical Variables</b> | |||
<table border="1" cellpadding="6"> | |||
<tr> | <tr> | ||
<td align=" | <td align="center" rowspan="2"> | ||
<math>~ | Variable | ||
</td> | |||
<td align="center" rowspan="2"> | |||
Throughout the Core<br> | |||
<math>~0 \le \xi \le \xi_i</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center" rowspan="2"> | ||
<math>~ | Throughout the Envelope<sup>†</sup><br> | ||
<math>~\eta_i \le \eta \le \eta_s</math> | |||
</td> | </td> | ||
<td align=" | |||
<td align="center" colspan="3"> | |||
Plotted Profiles | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td align="center"> | ||
<math>~\ | <math>~\xi_i = 0.5</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~\ | <math>~\xi_i = 1.0</math> | ||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
<math>~\ | <math>~\xi_i = 3.0</math> | ||
</td> | </td> | ||
</tr> | |||
<tr> | |||
<td align="center"> | <td align="center"> | ||
<math>~r^*</math> | |||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
<math> | <math>\biggl( \frac{3}{2\pi} \biggr)^{1/2} \xi</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math> | <math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta</math> | ||
</td> | </td> | ||
<td align=" | |||
<td align="center" colspan="3"> | |||
| |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td align="center"> | ||
<math>~ | <math>~\rho^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math> | <math>\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-5/2}</math> | ||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
<math> | <math>\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{5}_i \phi(\eta)</math> | ||
</td> | </td> | ||
<td align="center"> | |||
<td align=" | <!-- [[File:PlotDensity_xi_0.5.jpg|thumb|75px]] --> | ||
[[Image:DenXi05.jpg|thumb|75px]] | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
[[Image:DenXi10.jpg|thumb|75px]] | |||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
[[Image:DenXi30.jpg|thumb|75px]] | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td align="center"> | ||
<math>~ | <math>~P^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math> | <math>\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3}</math> | ||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
<math> | <math>\theta^{6}_i [\phi(\eta)]^{2}</math> | ||
</math> | |||
</td> | </td> | ||
= | <td align="center"> | ||
<!-- [[File:PlotPressure_xi_0.5.jpg|thumb|75px]] --> | |||
[[Image:PresXi05.jpg|thumb|75px]] | |||
</td> | |||
< | <td align="center"> | ||
[[Image:PresXi10.jpg|thumb|75px]] | |||
</ | </td> | ||
<td align="center"> | |||
[[Image:PresXi30.jpg|thumb|75px]] | |||
</td> | |||
< | </tr> | ||
<tr> | <tr> | ||
<td align=" | <td align="center"> | ||
<math>~ | <math>~M_r^*</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math> | <math>\biggl( \frac{2\cdot 3}{\pi } \biggr)^{1/2} \biggl[ \xi^3 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} \biggr]</math> | ||
</td> | </td> | ||
<td align=" | <td align="center"> | ||
<math> | <math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \theta^{-1}_i \biggl( \frac{2}{\pi} \biggr)^{1/2} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr)</math> | ||
\frac{ | |||
- \ | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | |||
<td align=" | <!-- [[File:PlotPressure_xi_0.5.jpg|thumb|75px]] --> | ||
< | [[Image:MassXi05.jpg|thumb|75px]] | ||
</td> | |||
<td align="center"> | |||
[[Image:MassXi10.jpg|thumb|75px]] | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
[[Image:MassXi30.jpg|thumb|75px]] | |||
</td> | </td> | ||
<td align="left"> | </tr> | ||
<math> | |||
<tr> | |||
<td align="left" colspan="6"> | |||
<sup>†</sup>In order to obtain the various envelope profiles, it is necessary to evaluate <math>\phi(\eta)</math> and its first derivative using the information presented in Step 6, above. | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</div> | |||
Therefore, throughout the core we have, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{\rho^*}{P^*}</math> | ||
\ | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,365: | Line 1,437: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\biggl | <math>~\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{1 / 2} \, ;</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,371: | Line 1,443: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{M_r^*}{r^*}</math> | ||
\frac{ | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,379: | Line 1,449: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \frac{\ | <math>~ | ||
\biggl( \frac{2\cdot 3}{\pi } \biggr)^{1/2} \biggl[ \xi^3 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} \biggr]\biggl( \frac{2\pi}{3}\biggr)^{1 / 2} \frac{1}{\xi} | |||
= 2 \xi^2 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} | |||
\, ; | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
and, throughout the envelope we have, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{\rho^*}{P^*}</math> | ||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,394: | Line 1,470: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl | \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{-1}_i \phi(\eta)^{-1} | ||
\, ; | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,401: | Line 1,478: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{M_r^*}{r^*}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,408: | Line 1,485: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{\ | \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \theta^{-1}_i \biggl( \frac{2}{\pi} \biggr)^{1/2} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) | ||
\biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta \biggr]^{-1} | |||
= | |||
2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \frac{\theta_i}{\eta} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) | |||
\, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,414: | Line 1,495: | ||
</table> | </table> | ||
<table border="1" align="center" width="80%" cellpadding="8"><tr><td align="left"> | |||
<font color="red">'''NOTE on 15 May 2019:'''</font> Prior to this date the last RHS expression had an incorrect exponent on <math>~\eta</math>. It previously (incorrectly) read, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{M_r^*}{r^*}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,426: | Line 1,508: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta_i \eta \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) | |||
\, . | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</td></tr></table> | |||
==Numerical Integration== | |||
===General Approach=== | |||
Here, we begin by recognizing that the 2<sup>nd</sup>-order ODE that must be integrated to obtain the desired eigenvectors has the generic form, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~0</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,442: | Line 1,532: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
x'' + \frac{\mathcal{H}}{r^*} x' + \mathcal{K}x \, , | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
where, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~x'</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,457: | Line 1,548: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{dx}{dr^*}</math> | ||
</math> | |||
</td> | </td> | ||
< | <td align="center"> and </td> | ||
< | |||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~x''</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,473: | Line 1,558: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~\frac{d^2x}{d(r^*)^2} \, .</math> | ||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
Adopting the same approach [[User:Tohline/SSC/Stability/Polytropes#Numerical_Integration_from_the_Center.2C_Outward|as before when we integrated the LAWE for pressure-truncated polytropes]], we will enlist the finite-difference approximations, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| <math>~x'</math> | ||
</td> | |||
<td align="center"> | |||
<math>~\approx</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{x_+ - x_-}{2\delta r^*} | |||
</math> | |||
</td> | |||
<td align="center"> and </td> | |||
<td align="right"> | |||
<math>~x''</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\approx</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{x_+ -2x_j + x_-}{(\delta r^*)^2} \, . | |||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
The finite-difference representation of the LAWE is, therefore, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{x_+ -2x_j + x_-}{(\delta r^*)^2}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,508: | Line 1,603: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
-~ \frac{\mathcal{H}}{r^*} \biggl[ \frac{x_+ - x_-}{2\delta r^*} \biggr] ~-~ \mathcal{K}x_j | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,514: | Line 1,611: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow~~~ | <math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,520: | Line 1,617: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \frac{ | <math>~ | ||
-~ \frac{\delta r^*}{2r^*} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta r^*)^2\mathcal{K}x_j | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,526: | Line 1,625: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow~~~ \frac{ | <math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,532: | Line 1,631: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \frac{ | <math>~ | ||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{j-1} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
In what follows we will also find it useful to rewrite <math>~\mathcal{K}</math> in the form, | |||
<div align="center"> | |||
<math>~\mathcal{K} ~\rightarrow ~\biggl(\frac{\sigma_c^2}{\gamma_\mathrm{g}}\biggr) \mathcal{K}_1 - \alpha_\mathrm{g} \mathcal{K}_2 \, .</math> | |||
</div> | |||
The relevant coefficient expressions for ''all'' regions of the configuration are, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\mathcal{H}</math> | ||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\} | |||
</math> | </math> | ||
</td> | </td> | ||
< | <td align="center"> , </td> | ||
<td align="right"> | |||
< | <math>~\mathcal{K}_1</math> | ||
<td align="right"> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{2\pi }{3}\biggl(\frac{\rho^*}{ P^* } \biggr) | |||
</math> | </math> | ||
</td> | </td> | ||
< | <td align="center"> and </td> | ||
< | |||
<td align="right"> | <td align="right"> | ||
<math>~\mathcal{K}_2</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\equiv</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl(\frac{\rho^*}{ P^* } \biggr)\frac{M_r^*}{(r^*)^3} \, . | |||
</math> | </math> | ||
</td> | </td> | ||
| Line 1,595: | Line 1,685: | ||
</table> | </table> | ||
===Special Handling at the Center=== | |||
In order to kick-start the integration, we set the displacement function value to <math>~x_1 = 1</math> at the center of the configuration <math>~(\xi_1 = 0)</math>, then draw on the [[User:Tohline/Appendix/Ramblings/PowerSeriesExpressions#PolytropicDisplacement|derived power-series expression]] to determine the value of the displacement function at the first radial grid line, <math>~\xi_2 = \delta\xi</math>, away from the center. Specifically, we set, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~ | ||
x_2 | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,607: | Line 1,700: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \frac{ | <math>~ | ||
x_1 \biggl[ 1 - \frac{(n+1) \mathfrak{F} (\delta\xi)^2}{60} \biggr]_{n=5} | |||
= | |||
x_1 \biggl[ 1 - \frac{\mathfrak{F} (\delta\xi)^2}{10} \biggr] | |||
\, .</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
</div> | |||
===Special Handling at the Interface=== | |||
Integrating outward from the center, the ''general approach'' will work up through the determination of <math>~x_{j+1}</math> when "j+1" refers to the interface location. In order to properly transition from the core to the envelope, we need to determine the value of the slope at this interface location. Let's do this by setting j = i, then projecting forward to what <math>~x_+</math> ''would be'' — that is, to what the amplitude just beyond the interface ''would be'' — if the core were to be extended one more zone. Then, the slope at the interface (as viewed from the perspective of the core) will be, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~x'_i\biggr|_\mathrm{core}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\approx</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{1}{2\delta r^*} \biggl\{ | |||
x_+ - x_{i-1} | |||
\biggr\} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,631: | Line 1,740: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- | <math>~ | ||
-\frac{x_{i-1}}{2\delta r^*} | |||
+ | |||
\frac{1}{2\delta r^*} \biggl\{ | |||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{i-1} | |||
\biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,643: | Line 1,758: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~2 \biggl[1 - \frac{ | <math>~ | ||
\frac{1}{2\delta r^*} \biggl\{ | |||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{i-1} ~-~\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]x_{i-1} | |||
\biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
| |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,659: | Line 1,774: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{1}{2\delta r^*} \biggl\{ | |||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~2x_{i-1} | |||
\biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
Conversely, as viewed from the ''envelope'', if we assume that we know <math>~x_i</math> and <math>~x'_i</math>, we can determine the amplitude, <math>~x_{i+1}</math>, at the first zone beyond the interface as follows: | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~x_-</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~\approx</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
x_{i+1} - 2\delta r^*\cdot x'_i\biggr|_\mathrm{env} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,677: | Line 1,802: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ x_{i+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,683: | Line 1,808: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~ | ||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] | |||
\biggl[ x_{i+1} - 2\delta r^*\cdot x'_i\biggr|_\mathrm{env} | |||
\biggr] | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,689: | Line 1,818: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ x_{i+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr] | |||
~+~ | |||
\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] | |||
x_{i+1} | |||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,695: | Line 1,828: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~ | ||
\biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i | |||
~+~ | |||
\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] | |||
2\delta r^*\cdot x'_i\biggr|_\mathrm{env} | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow ~~~ x_{i+1} | ||
</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,714: | Line 1,847: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl[ 1 - \tfrac{1}{2}(\delta r^*)^2\mathcal{K}\biggr] x_i | |||
+ | ~+~ | ||
\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] | |||
\delta r^*\cdot x'_i\biggr|_\mathrm{env} | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
==Splitting Analysis Into Separate Core and Envelope Components== | |||
===Core:=== | |||
Given that, <math>~\sqrt{2\pi/3}~r^* = \xi</math>, lets multiply the LAWE through by <math>~3/(2\pi)</math>. This gives, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
<math>~ | <math>~=</math> | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~- \frac{d\ | <math>~ | ||
\frac{d^2x}{d\xi^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{\xi} \cdot \frac{dx}{d\xi} | |||
+ \frac{3}{2\pi}\biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Specifically for the core, therefore, the finite-difference representation of the LAWE is, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{x_+ -2x_j + x_-}{(\delta \xi)^2}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,750: | Line 1,890: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | -~ \frac{\mathcal{H}}{\xi} \biggl[ \frac{x_+ - x_-}{2\delta \xi} \biggr] ~-~ \biggl[ \frac{3\mathcal{K}}{2\pi} \biggr]x_j | ||
+ | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,771: | Line 1,904: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | -~ \frac{\delta \xi}{2\xi} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta \xi)^2 \biggl[ \frac{3\mathcal{K}}{2\pi} \biggr] x_j | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta \xi}{2\xi}\biggr) \mathcal{H} \biggr]</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,791: | Line 1,917: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\biggl( \frac{3}{2\pi}\biggr) | <math>~ | ||
\biggl[ 2 - (\delta \xi)^2\biggl( \frac{3\mathcal{K}}{2\pi} \biggr) \biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta \xi}{2\xi} \biggr) \mathcal{H} \biggr]x_{j-1} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
This also means that, as viewed from the perspective of the core, the slope at the interface is | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\biggl[ \frac{dx}{d\xi}\biggr]_\mathrm{interface}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,809: | Line 1,935: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\biggl[ \biggl( \frac{\ | <math>~ | ||
\frac{1}{2\delta \xi} \biggl\{ | |||
\biggl[ 2 - (\delta \xi)^2 \biggl( \frac{3\mathcal{K}}{2\pi} \biggr)\biggr] x_i ~-~2x_{i-1} | |||
\biggr\}\biggl[1 + \biggl( \frac{\delta \xi}{2\xi}\biggr) \mathcal{H} \biggr]^{-1} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | |||
===Envelope:=== | |||
Given that, | |||
<div align="center"> | |||
<math>~\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta_i^2 (2\pi)^{1 / 2}~r^* = \eta \, ,</math> | |||
</div> | |||
let's multiply the LAWE through by <math>~(2\pi)^{-1} \theta_i^{-4}( \mu_e/\mu_c)^{-2} </math>. This gives, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~0</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,828: | Line 1,962: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | \frac{d^2x}{d\eta^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} | ||
\, . | + \frac{1}{2\pi \theta_i^4} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . | ||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Specifically for the envelope, therefore, the finite-difference representation of the LAWE is, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\frac{x_+ -2x_j + x_-}{(\delta \eta)^2}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,851: | Line 1,981: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
-~ \frac{\mathcal{H}}{\eta} \biggl[ \frac{x_+ - x_-}{2\delta \eta} \biggr] ~-~ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2}\biggl[ \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr]x_j | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,866: | Line 1,988: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~ | <math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,873: | Line 1,995: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\frac{ | -~ \frac{\delta \eta}{2\eta} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2}\biggl[ \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr] x_j | ||
- | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{ | <math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta \eta}{2\eta}\biggr) \mathcal{H} \biggr]</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,900: | Line 2,008: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\biggl[ 2 - (\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl( \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr) \biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta \eta}{2\eta} \biggr) \mathcal{H} \biggr]x_{j-1} \, . | |||
</math> | |||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
This also means that, once we know the slope at the interface (see immediately below), the amplitude at the first zone outside of the interface will be given by the expression, | |||
< | <table border="0" cellpadding="5" align="center"> | ||
<tr> | |||
<td align="right"> | |||
<math>~x_{i+1} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 1 - \tfrac{1}{2}(\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl( \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr)\biggr] x_i | |||
~+~ | |||
\biggl[ 1 - \biggl( \frac{\delta \eta}{2\eta} \biggr) \mathcal{H} \biggr] | |||
\delta \eta \cdot \biggl[ \frac{dx}{d\eta} \biggr]_\mathrm{interface} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
===Interface=== | |||
If we consider only cases where <math>~\gamma_e = \gamma_c</math>, then at the interface we expect, | |||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\frac{d\ln | <math>~\frac{d\ln x}{d\ln r^*}</math> | ||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,917: | Line 2,050: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{ | <math>~\frac{d\ln x}{d\ln \xi} = \frac{d\ln x}{d\ln \eta}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,923: | Line 2,056: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ r^*\frac{dx}{d r^*}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,929: | Line 2,062: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\ | <math>~\xi \frac{dx}{d \xi} = \eta \frac{d x}{d \eta}</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
| Line 1,935: | Line 2,068: | ||
<tr> | <tr> | ||
<td align="right"> | <td align="right"> | ||
<math>~\Rightarrow ~~~ \frac{dx}{dr^*}</math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,941: | Line 2,074: | ||
</td> | </td> | ||
<td align="left"> | <td align="left"> | ||
<math>~\frac{ | <math>~\biggl(\frac{2\pi}{3} \biggr)^{1 / 2}\frac{dx}{d\xi} = \biggl(\frac{\mu_e}{\mu_c}\biggr) \theta_i^2 (2\pi)^{1 / 2} \frac{dx}{d\eta} \, .</math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
</ | Switching at the interface from <math>~\xi</math> to <math>~\eta</math> therefore means that, | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
<tr> | <tr> | ||
<td align="right"> | |||
<math>~ \biggl[ \frac{dx}{d\eta}\biggr]_\mathrm{interface}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{\sqrt{3}} \biggl(\frac{\mu_e}{\mu_c}\biggr)^{-1} \theta_i^{-2} \biggl[ \frac{dx}{d\xi}\biggr]_\mathrm{interface} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
If, however, we want to consider values for the adiabatic index that are different in the two regions, we have to follow the [[#Interface_Conditions|above-outlined guidelines]], that is, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \frac{d\ln x}{d\ln \eta} \biggr|_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x}{d\ln \xi} \biggr)_i </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \Rightarrow ~~~ \biggl[ \frac{dx}{d\eta} \biggr]_i</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{x_i}{\eta_i} \biggl\{ | |||
3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \cdot \frac{\xi_i}{x_i}\biggl[ \frac{dx}{d\xi} \biggr]_i | |||
\biggr\}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{3x_i}{\eta_i} \biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \cdot \frac{1}{\sqrt{3}} \biggl(\frac{\mu_e}{\mu_c}\biggr)^{-1} \theta_i^{-2} \biggl[ \frac{dx}{d\xi} \biggr]_i \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
==Eigenvectors for Marginally Unstable Models with (γ<sub>c</sub>, γ<sub>e</sub>) = (6/5, 2)== | |||
We now have the tools in hand to identify the eigenvectors — that is, various radial eigenfunctions and the corresponding eigenfrequency for each — associated with various modes of oscillation in <math>~(n_c, n_e) = (5,1)</math> bipolytropes. Which models should we examine? | |||
In our [[User:Tohline/SSC/Stability/MurphyFiedler85#Review_of_the_BiPolytrope_Stability_Analysis_by_Murphy_.26_Fiedler_.281985b.29|accompanying review of the bipolytrope stability analysis presented by Murphy & Fiedler (1983b)]], our primary objective was to show that we were able to match their results quantitatively. We therefore set <math>~\mu_e/\mu_c</math> = 1 — the only <math>~\mu</math>-ratio that they considered — and picked values of the core-envelope interface radius, <math>~\xi_i</math>, that were listed among their set of chosen models. For a fixed value of <math>~\xi_i</math>, we integrated the relevant LAWE from the center toward the surface for many different eigenfrequency <math>~(\sigma_c^2)</math> ''guesses'' until an eigenfunction was found whose behavior at the surface matched with high precision the physically justified surface boundary condition. | |||
With the [[#Virial_Stability_Evaluation|above virial stability analysis]] in mind (see especially [[#Virial_Stability_Evaluation|Figure 3]]), here we have chosen to focus on models that reside along six of the equilibrium sequences that have already been analytically identified, above — specifically, the sequences for which <math>~\mu_e/\mu_c</math> = 1, ½, 0.345, ⅓, 0.309, and ¼ — and to examine oscillation modes under the assumption that, | |||
<div align="center"> | |||
<math>~\gamma_c = \frac{(n_c+1)}{n_c} = \frac{6}{5} \, ,</math> and <math>~\gamma_e = \frac{(n_e+1)}{n_e} =2 \, .</math> | |||
</div> | |||
===Fundamental Modes=== | |||
We decided to examine, first, whether any model along each sequence marks a transition from dynamically stable to dynamically unstable configurations. We accomplished this by setting <math>~\sigma_c^2</math> = 0, then integrating the relevant LAWE from the center toward the surface for many different ''guesses'' of the core-envelope interface radius until an eigenfunction with no radial nodes — ''i.e.,'' an eigenfunction associated with the fundamental mode of radial oscillation — was found whose behavior at the surface matched with high precision the physically desired surface boundary condition. We were successful in this endeavor. A marginally unstable model was identified on each of the six separate equilibrium sequences. | |||
====Equilibrium Properties of Marginally Unstable Models==== | |||
Table 2 summarizes some of the equilibrium properties of these six models. For example, the second column of the table gives the value of the core-envelope interface radius, <math>~\xi_i</math>, associated with each marginally unstable model. The table also lists: the value of the model's dimensionless radius, <math>~R^*_\mathrm{surf}</math>, the key structural parameters, <math>~q</math> & <math>~\nu</math>, and the central-to-mean density associated with each model; and in each case the dimensionless thermal energy <math>~(\mathfrak{s})</math> and dimensionless gravitational potential energy <math>~(\mathfrak{w})</math> associated, separately, with the core and the envelope. Note that, once the pair of parameters, <math>~(\mu_e/\mu_c, \xi_i)</math>, has been specified, we can legitimately assign high-precision values to all of the other model parameters because they are [[User:Tohline/SSC/Structure/BiPolytropes/Analytic5_1#Parameter_Values|analytically prescribed]]. | |||
<div align="center"> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<th align="center" colspan="10"> | |||
'''Table 2:''' Properties of Marginally Unstable Bipolytropes Having<br /><br /><math>~(n_c, n_e) = (5, 1)</math> and <math>~(\gamma_c, \gamma_e) = (\tfrac{6}{5}, 2)</math><br /><br />Determined from Integration of the LAWE | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\frac{\mu_e}{\mu_c}</math></td> | |||
<td align="center"><math>~\xi_i</math></td> | |||
<td align="center"><math>~R^*_\mathrm{surf}</math></td> | |||
<td align="center"><math>~q \equiv \frac{r_\mathrm{core}}{R_\mathrm{surf}}</math></td> | |||
<td align="center"><math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math></td> | |||
<td align="center"><math>~\frac{\rho_c}{\bar\rho}</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{env}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{env}</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">1</td> | |||
<td align="left">1.6686460157</td> | |||
<td align="right">2.139737</td> | |||
<td align="center">0.53885819</td> | |||
<td align="center">0.497747626</td> | |||
<td align="center">8.51704656</td> | |||
<td align="center">3.021916335</td> | |||
<td align="center">-3.356583022</td> | |||
<td align="center">1.47780476</td> | |||
<td align="center">-5.642859167</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{2}</math></td> | |||
<td align="left">2.27925811317</td> | |||
<td align="right">5.146499</td> | |||
<td align="center">0.306021732</td> | |||
<td align="center">0.401776274</td> | |||
<td align="center">63.29514949</td> | |||
<td align="center">4.241287117</td> | |||
<td align="center">-6.074241035</td> | |||
<td align="center">4.284931508</td> | |||
<td align="center">-10.97819621</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.345</td> | |||
<td align="left">2.560146865247</td> | |||
<td align="right">9.554041</td> | |||
<td align="center">0.185160563</td> | |||
<td align="center">0.234302525</td> | |||
<td align="center">209.7739052</td> | |||
<td align="center">4.639705843</td> | |||
<td align="center">-7.125754184</td> | |||
<td align="center">11.72861751</td> | |||
<td align="center">-25.61089252</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{3}</math></td> | |||
<td align="left">2.582007485476</td> | |||
<td align="right">10.120558</td> | |||
<td align="center">0.176288391</td> | |||
<td align="center">0.218241608</td> | |||
<td align="center">230.4125398</td> | |||
<td align="center">4.667042505</td> | |||
<td align="center">-7.200966267</td> | |||
<td align="center">13.15887139</td> | |||
<td align="center">-28.45086152</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.309</td> | |||
<td align="left">2.6274239687695</td> | |||
<td align="center">11.464303</td> | |||
<td align="center">0.158362807</td> | |||
<td align="center">0.184796947</td> | |||
<td align="center">279.0788798</td> | |||
<td align="center">4.722277318</td> | |||
<td align="center">-7.354156963</td> | |||
<td align="center">17.1374434</td> | |||
<td align="center">-36.36528446</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{4}</math></td> | |||
<td align="left">2.7357711469398</td> | |||
<td align="center">15.895632</td> | |||
<td align="center">0.118924863</td> | |||
<td align="center">0.11071211</td> | |||
<td align="center">430.0444648</td> | |||
<td align="center">4.84592201</td> | |||
<td align="center">-7.70305421</td> | |||
<td align="center">37.84289623</td> | |||
<td align="center">-77.67458196</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<!-- | |||
OLD VERSION; IGNORE! | |||
<div align="center"> | |||
<font color="red">'''OLD Table 2'''</font> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<th align="center" colspan="9"> | |||
Properties of Marginally Unstable Bipolytropes Having<br /><br /><math>~(n_c, n_e) = (5, 1)</math> and <math>~(\gamma_c, \gamma_e) = (\tfrac{6}{5}, 2)</math><br /><br />Determined from Integration of the LAWE | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\frac{\mu_e}{\mu_c}</math></td> | |||
<td align="center"><math>~\xi_i</math></td> | |||
<td align="center"><math>~q \equiv \frac{r_\mathrm{core}}{R_\mathrm{surf}}</math></td> | |||
<td align="center"><math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math></td> | |||
<td align="center"><math>~\frac{\rho_c}{\bar\rho}</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{env}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{env}</math></td> | |||
<td align="center"><math>~\mathfrak{s}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\mathfrak{w}_\mathrm{core}</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">1</td> | |||
<td align="left">2.467359668</td> | |||
<td align="center">0.597684036</td> | |||
<td align="center">0.692367564</td> | |||
<td align="center">17.09749847</td> | |||
<td align="center">4.518031091</td> | |||
<td align="center">-6.79580606</td> | |||
<td align="center">0.857904827</td> | |||
<td align="center">-3.956065776</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{2}</math></td> | |||
<td align="left">2.65925887</td> | |||
<td align="center">0.314759926</td> | |||
<td align="center">0.481811565</td> | |||
<td align="center">95.04044773</td> | |||
<td align="center">4.759771212</td> | |||
<td align="center">-7.459080087</td> | |||
<td align="center">3.20977926</td> | |||
<td align="center">-8.480020858</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.345</td> | |||
<td align="left">2.77457272408</td> | |||
<td align="center">0.183201946</td> | |||
<td align="center">0.25725514</td> | |||
<td align="center">281.7482802</td> | |||
<td align="center">4.887554727</td> | |||
<td align="center">-7.822410223</td> | |||
<td align="center">10.22665208</td> | |||
<td align="center">-22.40600339</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{3}</math></td> | |||
<td align="left">2.7843424754</td> | |||
<td align="center">0.17412463</td> | |||
<td align="center">0.237677946</td> | |||
<td align="center">305.4881577</td> | |||
<td align="center">4.897826446</td> | |||
<td align="center">-7.852004624</td> | |||
<td align="center">11.6114224</td> | |||
<td align="center">-25.16649306</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.309</td> | |||
<td align="left">2.8050375512</td> | |||
<td align="center">0.156021514</td> | |||
<td align="center">0.197918988</td> | |||
<td align="center">359.3276918</td> | |||
<td align="center">4.91930991</td> | |||
<td align="center">-7.914090174</td> | |||
<td align="center">15.49005222</td> | |||
<td align="center">-32.90463409</td> | |||
</tr> | |||
</table> | |||
</div> | |||
OLD VERSION; IGNORE! | |||
--> | |||
As was expected from our [[#What_to_Expect_for_Equilibrium_Configurations|above discussion of virial equilibrium conditions]], we found that to high precision for each of these equilibrium models, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math> | |||
(\mathfrak{w}_\mathrm{core} | |||
~+~\mathfrak{w}_\mathrm{env}) | |||
~+~2(\mathfrak{s}_\mathrm{core} | |||
~+~\mathfrak{s}_\mathrm{env}) | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~0 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<span id="Figure4">However, contrary to expectations,</span> in no case did we find that <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env} = 5</math>. That is to say, we found that ''none'' of the models lies on the (red-dashed) curve in the <math>~q-\nu</math> parameter space that separates stable from unstable models as defined by our [[#What_to_Expect_for_Equilibrium_Configurations|above free-energy-based stability analysis]]. The left-hand panel of Figure 4 shows this (red-dashed) demarcation curve; for all intents and purposes, it is a reproduction of the right-hand panel of [[#Virial_Stability_Evaluation|Figure 3, above]] — turning-point markers have been removed to minimize clutter, the equilibrium sequences have been labeled, and the horizontal axis has been extended to unity in order to include a longer portion of the <math>~\mu_e/\mu_c = 1</math> sequence. The orange triangular markers that appear in the right-hand panel of Figure 4 pinpoint where each of the Table 2 "marginally unstable" models resides in this <math>~q-\nu</math> plane. Clearly, all six of the orange triangles lie well off of — and to the ''stable'' side of — the red-dashed demarcation curve. This discrepancy, which has resulted from our use of two separate approaches to stability analysis, will be discussed further and gratifyingly resolved, below. | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<th align="center">Figure 4</th> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="10">[[File:NEWCompositeDlabeled.png|800px|Marginally unstable models]]</td> | |||
</tr> | |||
</table> | |||
====Eigenfunction Details==== | |||
Here we examine some of the properties of the fundamental-mode eigenfunctions that we have found are associated with marginally unstable, <math>~(n_c, n_e) = (5,1)</math> bipolytropes. | |||
<table border="0" align="right" width="40%"> | |||
<tr> | |||
<th align="center">Figure 5</th> | |||
</tr> | |||
<tr><td align="center"> | |||
[[File:Mod0MuRatio100.png|450px|Example eigenvector]] | |||
</td></tr> | |||
</table> | |||
Consider the model on the <math>~\mu_e/\mu_c = 1</math> sequence for which <math>~\sigma_c^2=0~</math>; key properties of this specific equilibrium model are enumerated in the first row of numbers provided in [[#Equilibrium_Properties_of_Marginally_Unstable_Models|Table 2, above]]. Figure 5 shows how our numerically derived, fundamental-mode eigenfunction, <math>~x = \delta r/r_0</math>, varies with the fractional radius over the entire range, <math>~0 \le r/R \le 1</math>. By prescription, the eigenfunction has a value of unity and a slope of zero at the center <math>~(r/R = 0)</math>. Integrating the LAWE outward from the center, through the model's core (blue curve segment), <math>~x</math> drops smoothly to the value <math>~x_i = 0.81437</math> at the interface <math>~(\xi_i = 1.6686460157 ~\Rightarrow~ q = r_\mathrm{core}/R_\mathrm{surf} = 0.53885819)</math>. Our numerical integration of the LAWE showed that, at the interface, the logarithmic slope of the ''core'' (blue) segment of the eigenfunction is, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i \biggr\}_\mathrm{core} | |||
= | |||
\biggl\{ \frac{d\ln x}{d\ln \xi}\biggr|_i \biggr\}_\mathrm{core} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- 0.455872 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Next, following the [[#Interface|above discussion of matching conditions at the interface]], we determined that, from the perspective of the envelope, the slope of the eigenfunction at the interface must therefore be, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i \biggr\}_\mathrm{env} | |||
= | |||
\biggl\{ \frac{d\ln x}{d\ln \eta}\biggr|_i \biggr\}_\mathrm{env} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl\{ \frac{d\ln x}{d\ln \xi}\biggr|_i \biggr\}_\mathrm{core} = -1.47352 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Adopting this "env" slope along with the amplitude, <math>~x_i = 0.81437</math>, as the appropriate ''interface'' boundary conditions, we integrated the LAWE from the interface to the surface, obtaining the green-colored segment of the eigenfunction that is shown in Figure 5. The amplitude continued to steadily decrease, reaching a value of <math>~x_s = 0.38203</math>, at the model's surface <math>~(r/R = 1)</math>. At the surface, this ''envelope'' (green) segment of the eigenfunction exhibits a logarithmic slope that matches to eight significant digits the value that is [[#SurfaceCondition|expected from astrophysical arguments]] for this marginally unstable <math>~(\sigma_c^2=0)</math> model, namely, | |||
<div align="center"> | |||
<math>~ | |||
\frac{d\ln x}{d\ln \eta}\biggr|_s = \biggl[ \biggl( \frac{\rho_c}{\bar\rho} \biggr)\frac{\cancelto{0}{\sigma_c^2}}{2\gamma_e} - \biggl(3 - \frac{4}{\gamma_e}\biggr)\biggr] = -1 \, . | |||
</math> | |||
</div> | |||
<table border="1" cellpadding="10" width="80%" align="center"><tr><td align="left"> | |||
<font color="red">'''Key Reminder:'''</font> We were able to find an eigenfunction whose surface boundary condition matched the desired value — in this particular case, a logarithmic slope of negative one — to this high level of precision only by iterating many times and, at each step, fine-tuning our choice of the equilibrium model's radial interface location, <math>~\xi_i</math> before performing a numerical integration of the LAWE. | |||
</td></tr></table> | |||
The discontinuous jump that occurs in the ''slope'' of the eigenfunction at the interface results from our assumption that the effective adiabatic index of material in the core <math>~(\gamma_c = 6/5)</math> is different from the effective adiabatic index of the envelope material <math>~(\gamma_e = 2)</math>. In an effort to emphasize and more clearly illustrate the behavior of this fundamental-mode eigenfunction as it crosses the core/envelope interface, we have added a pair of dashed line segments to the Figure 5 plot. The red-dashed line segment touches, and is tangent to, the blue segment of the eigenfunction at the location of the core/envelope interface; it has a slope, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\frac{dx}{d(r/R)}\biggr|_i | |||
= | |||
\frac{x_i}{(r_i/R)}\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i\biggr\}_\mathrm{core} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- 0.455872 \biggl(\frac{ 0.81437 }{ 0.53885819 }\biggr) = - 0.68895\, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
On the other hand, the purple-dashed line segment touches, and is tangent to, the green segment of the eigenfunction at the location of the core/envelope interface; it has a slope, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ | |||
\frac{dx}{d(r/R)}\biggr|_i | |||
= | |||
\frac{x_i}{(r_i/R)}\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i\biggr\}_\mathrm{env} | |||
</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- 1.47352 \biggl(\frac{ 0.81437 }{ 0.53885819 }\biggr) = - 2.22691\, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
For comparison purposes, the eigenfunction shown in Figure 5 has been presented again in Figure 6, along with several other of our numerically derived eigenfunctions, but in Figure 6 the plotted amplitude has been renormalized to give a surface value — rather than a central value — of unity. | |||
In Figure 6 we show the behavior of the fundamental-mode eigenfunction for each of the marginally unstable models identified in Table 2. In the top figure panel, each curve shows — on a linear-linear plot — how the amplitude varies with radius; in the bottom figure panel, the amplitude is plotted on a logarithmic scale. On each curve, the black plus sign marks the radial location of the core-envelope interface; in the bottom panel, these markers are accompanied by the values of <math>~\xi_i</math> that are associated with each corresponding model (see also the second column of Table 2). Each eigenfunction has been normalized such that the surface amplitude is unity. In the top panel, the value of the central amplitude of the eigenfunction that results from this normalization is recorded near the point where each eigenfunction touches the vertical axis. (In each case, the value provided on the plot is simply the inverse of the value of <math>~x_s</math> given in Table 3, below.) | |||
<table border="0" align="center" cellpadding="8"> | |||
<tr> | |||
<th align="center"> | |||
'''Figure 6: Eigenfunctions Associated with the Fundamental-Mode of Radial Oscillation'''<br /> | |||
'''in Marginally Unstable Models having Various''' <math>~\mu_e/\mu_c</math> | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"> | |||
[[File:Mode0EigenfunctionsCombinedSmall.png|800px|Eigenfunctions for Marginally Unstable Models]] | |||
</td> | |||
</tr> | |||
</table> | |||
Notice that, especially as they approach the surface, the "envelope" segments of these six marginally unstable eigenfunction appear to merge into the same curve, irrespective of their value of the ratio of mean molecular weights. Note as well that the discontinuous jump that occurs in the ''slope'' of each eigenfunction at the radial location of the core/envelope interface — resulting from our choice to adopt a different adiabatic index, <math>~\gamma_g</math>, in the core from the one in the envelope — becomes less and less noticeable for smaller and smaller values of the ratio of mean molecular weights. | |||
====Is There an Analytic Expression for the Eigenfunction?==== | |||
After noticing that, in Figure 6, the ''envelope'' segments of all of the marginally unstable eigenfunctions merge into the same curve, we began to wonder whether a single expression — and, even better, an ''analytically defined'' expression — would perfectly describe the eigenfunction. We had reason to believe that this might actually be possible because, in [[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Analyses_of_Radial_Oscillations|pressure-truncated polytropic configurations, we have derived analytic expressions for the marginally unstable, fundamental-mode eigenfunctions]] of both <math>~n = 5</math> and <math>~n=1</math> systems. | |||
Very quickly, we convinced ourselves that a parabolic function does indeed perfectly match the "core" segment of each displayed eigenfunction. Specifically, throughout the core <math>~(0 \le \xi \le \xi_i)</math>, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~x_P\biggr|_\mathrm{core}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~1 - \frac{\xi^2}{15}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~\frac{dx_P}{d\xi}\biggr|_\mathrm{core}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- \frac{2\xi}{15} </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~\frac{d\ln x_P}{d\ln \xi}\biggr|_\mathrm{core}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- \frac{2\xi^2}{15} \biggl[ \frac{(15 - \xi^2)}{15} \biggr]^{-1} = - \frac{2\xi^2}{(15 - \xi^2)} \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
The envelope segment posed a much greater challenge. In the context of our [[User:Tohline/SSC/Stability/n1PolytropeLAWE#Radial_Oscillations_of_n_.3D_1_Polytropic_Spheres|discussion of ''Radial Oscillations of n = 1 Polytropic Spheres'']], and in an [[User:Tohline/Appendix/Ramblings/BiPolytrope51AnalyticStability#Is_There_an_Analytic_Expression_for_the_Eigenfunction.3F|accompanying ''Ramblings Appendix'' chapter]] we have detailed some trial derivations that are mostly blind alleyways. Twice — once in [[User:Tohline/SSC/Stability/n1PolytropeLAWE#tagJanuary2019|January, 2019]] and again (independently) in [[User:Tohline/Appendix/Ramblings/BiPolytrope51AnalyticStability#Attempt_4B|April 2019]] — we have analytically demonstrated that the following appears to work for the envelope: | |||
Given that the ''Structural Properties'' of the envelope are described by the Lane-Emden function, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\phi</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~a_0 \biggl[ \frac{\sin(\eta - b_0)}{\eta} \biggr] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~ Q \equiv - \frac{d\ln \phi}{d\ln\eta}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[1 - \eta \cot(\eta - b_0) \biggr] \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
the relevant LAWE is satisfied by the fractional displacement function, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~x_P</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3c_0 Q}{\eta^2} \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
where, <math>~c_0</math> is an arbitrary scale factor. | |||
<table border="1" align="center" cellpadding="8" width="60%"><tr><td align="left"> | |||
Note that, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{dx_P}{d\eta}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{3c_0}{\eta^2}\biggl[\eta -\cot(\eta - b_0) | |||
+\eta\cot^2(\eta - b_0) | |||
\biggr] | |||
- \frac{6c_0 Q}{\eta^3} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~\frac{d\ln x_P}{d\ln\eta} = \frac{\eta}{x_P}\cdot \frac{dx_P}{d\eta}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{\eta^3}{3c_0 Q} \biggl\{ | |||
\frac{3c_0}{\eta^2}\biggl[\eta -\cot(\eta - b_0) | |||
+\eta\cot^2(\eta - b_0) | |||
\biggr] | |||
- \frac{6c_0 Q}{\eta^3} | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{Q} \biggl\{ | |||
\biggl[\eta^2 -\eta \cot(\eta - b_0) | |||
+\eta^2\cot^2(\eta - b_0) | |||
\biggr] | |||
- 2 Q | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{Q} | |||
\biggl[\eta^2 -(1-Q) | |||
+(1-Q)^2 | |||
\biggr] | |||
- 2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{Q} | |||
\biggl[\eta^2 - Q + Q^2\biggr] | |||
- 2 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{\eta^2}{Q} + Q - 3 \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</td></tr></table> | |||
But, as far as we have been able to determine (as of 16 April 2019), this analytic displacement function does not match the displacement function that has been generated through numerical integration of the LAWE (see the light-green segment of the eigenfunction displayed [[#Eigenfunction_Details|above in Figure 5]]). It remains unclear whether (a) the numerical integration is at fault, (b) we are imposing an incorrect slope at the core-envelope interface, or ( c) we are misinterpreting how to compare the two separately derived (one, numerical, and the other, analytic) envelope eigenfunctions. | |||
===Other Modes=== | |||
<!-- | |||
Keep in mind that, for all models, we ''expect'' that, at the surface, the logarithmic derivative of each proper eigenfunction will be, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{d\ln x}{d\ln r^*}\biggr|_\mathrm{surf}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{\Omega^2}{\gamma} - \alpha \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Also, keep in mind that, for Model 10 <math>~(\xi_i = 2.5646)</math>: | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{r_i}{R}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~0.39302482</math> | |||
</td> | |||
<td align="center"> , </td> | |||
<td align="right"> | <td align="right"> | ||
<math>~\frac{\rho_c}{\bar\rho}</math> | <math>~\frac{\rho_c}{\bar\rho}</math> | ||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~34.3460405</math> | |||
</td> | |||
</tr> | |||
</table> | |||
--> | |||
<table border="1" align="center" cellpadding="8"> | |||
<tr> | |||
<td align="center" colspan="15"> | |||
'''Our Determinations for Marginally Unstable Model Having <math>~\mu_e/\mu_c = 1</math>'''<br /> <br /> | |||
<math>~[ ~\xi_i = 1.6686460157 ~]</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="center" rowspan="2">Mode</td> | |||
<td align="center" rowspan="2"><math>~\sigma_c^2</math></td> | |||
<td align="center" rowspan="2"><math>~\Omega^2 \equiv \frac{\sigma_c^2}{2} \biggl( \frac{\rho_c}{\bar\rho}\biggr)</math></td> | |||
<td align="center" rowspan="2"><math>~x_i</math></td> | |||
<td align="center" colspan="2"><math>~\frac{d\ln x}{d\ln r^*}\biggr|_i</math></td> | |||
<td align="center" rowspan="2"><math>~x_\mathrm{surf}</math></td> | |||
<td align="center" colspan="2"><math>~\frac{d\ln x}{d\ln r^*}\biggr|_\mathrm{surf}</math></td> | |||
<td align="center" rowspan="2"><math>~\frac{r}{R}\biggr|_1</math></td> | |||
<td align="center" rowspan="2"><math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_1</math></td> | |||
<td align="center" rowspan="2"><math>~\frac{r}{R}\biggr|_2</math></td> | |||
<td align="center" rowspan="2"><math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_2</math></td> | |||
<td align="center" rowspan="2"><math>~\frac{r}{R}\biggr|_3</math></td> | |||
<td align="center" rowspan="2"><math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_3</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">core</td> | |||
<td align="center">env</td> | |||
<td align="center">''expected''</td> | |||
<td align="center">measured</td> | |||
</tr> | |||
<tr> | |||
<td align="center">1<br /><font size="-1">(Fundamental)</font></td> | |||
<td align="right">0.00</td> | |||
<td align="right">0.00</td> | |||
<td align="right">+0.81437470</td> | |||
<td align="right">-0.455872</td> | |||
<td align="right">-1.473523</td> | |||
<td align="right">+0.3820</td> | |||
<td align="right">-1</td> | |||
<td align="right">-0.999999992</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
</tr> | |||
<tr> | |||
<td align="center">2</td> | |||
<td align="right">2.51513333</td> | |||
<td align="right">10.7107538</td> | |||
<td align="right">0.20482050</td> | |||
<td align="right">-7.09124</td> | |||
<td align="right">-5.4547441</td> | |||
<td align="right">- 0.9962</td> | |||
<td align="right">4.355376917</td> | |||
<td align="right">4.35537692</td> | |||
<td align="right">0.64133</td> | |||
<td align="right">0.3502</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
</tr> | |||
<tr> | |||
<td align="center">3</td> | |||
<td align="right">5.72371888</td> | |||
<td align="right">24.3745901</td> | |||
<td align="right">-0.14269277</td> | |||
<td align="right">+8.046019</td> | |||
<td align="right">+3.627611</td> | |||
<td align="right">+0.9308</td> | |||
<td align="right">11.18729505</td> | |||
<td align="right">11.18729506</td> | |||
<td align="right">0.4837</td> | |||
<td align="right">0.5864</td> | |||
<td align="right">0.842</td> | |||
<td align="right">0.0854</td> | |||
<td align="center">n/a</td> | |||
<td align="center">n/a</td> | |||
</tr> | |||
<tr> | |||
<td align="center">4</td> | |||
<td align="right">10.3458476</td> | |||
<td align="right">44.0622916</td> | |||
<td align="right">-0.20845197</td> | |||
<td align="right">-0.6949966</td> | |||
<td align="right">-1.61699793</td> | |||
<td align="right">-1.1443</td> | |||
<td align="right">21.03114578</td> | |||
<td align="right">21.03114577</td> | |||
<td align="right">0.3939</td> | |||
<td align="right">0.7154</td> | |||
<td align="right">0.6902</td> | |||
<td align="right">0.2777</td> | |||
<td align="center">0.9115</td> | |||
<td align="center">0.0284</td> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="15"> | |||
[[File:Mod0MuRatio100.png|550px|Our determination of eigenvector for mu_ratio = 1]] [[File:FourModesMuRatio100.png|550px|Our determination of multiple eigenvectors for mu_ratio = 1]] | |||
</td> | |||
</tr> | |||
</table> | |||
=Reconciliation= | |||
<table border="0" cellpadding="8" align="right"> | |||
<tr> | |||
<th align="center">Figure 7: Conflicting Instability Regions</th> | |||
</tr> | |||
<tr> | |||
<td align="center" colspan="10">[[File:EigenvectorStability.png|400px|Marginally unstable models]]</td> | |||
</tr> | |||
</table> | |||
Figure 7, shown here on the right, is identical to the right-hand panel of [[#Figure4|Figure 4, as displayed above]]. From a standard global, free-energy analysis — such as the one [[#Virial_Stability_Evaluation|summarized above]] — we have determined that the red-dashed curve shown in the right panel of Figure 6 divides the <math>~q-\nu</math> plane into dynamically stable (below and to the right) and unstable (above and to the left) regions. | |||
==Variational Principle== | |||
===Setup=== | |||
Let's follow the guidelines of the [[User:Tohline/SSC/Synopsis_StyleSheet|variational principle]]. Instead of starting with the form of the LAWE given above, namely, | |||
<div align="center" id="2ndOrderODE"> | |||
<font color="#770000">'''Adiabatic Wave''' (or ''Radial Pulsation'') '''Equation'''</font><br /> | |||
{{User:Tohline/Math/EQ_RadialPulsation01}} | |||
</div> | |||
we will start with a form that is more amenable to the variational principle, namely, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{d}{dr_0}\biggl[ r_0^4 \gamma P_0 ~\frac{dx}{dr_0} \biggr] | |||
+\biggl[ \omega^2 \rho_0 r_0^4 + (3\gamma - 4) r_0^3 \frac{dP_0}{dr_0} \biggr] x \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<table border="1" cellpadding="8" align="center" width="85%"><tr><td align="left"> | |||
<font color="red">'''ASIDE:'''</font> Let's show that these two expressions are equivalent. Remembering that, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{dP_0}{dr_0}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~- g_0 \rho_0 = - \biggl(\frac{GM_r}{r_0^2}\biggr)\rho_0 \, ,</math> | |||
</td> | |||
</tr> | |||
</table> | |||
the second expression becomes, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
r_0^4 \gamma P_0 ~\frac{d^2x}{dr_0^2} | |||
+ ~\gamma \frac{dx}{dr_0}\biggl[ 4r_0^3 P_0 - r_0^4 g_0 \rho_0\biggr] | |||
+\biggl[ \omega^2 \rho_0 r_0^4 - (3\gamma - 4) r_0^3 g_0 \rho_0\biggr] x | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
r_0^4 \gamma P_0 \biggl\{\frac{d^2x}{dr_0^2} | |||
+ \biggl[ \frac{4}{r_0} - \frac{g_0 \rho_0}{P_0} \biggr] \frac{dx}{dr_0} | |||
+\frac{\rho_0}{\gamma P_0 }\biggl[ \omega^2 - (3\gamma - 4) \frac{g_0}{r_0} \biggr] x | |||
\biggr\}\, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Hence, we must multiply the first expression through by <math>~r_0^4 \gamma P_0</math> in order to obtain the second expression. | |||
</td></tr></table> | |||
From [[#Foundation|above]], we realize that multiplying the second expression through by <math>~(K_c/G)\rho_c^{-4 / 5}</math> gives, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
r_0^4\gamma P_0 \biggl\{ | |||
\frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} | |||
+ \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
(r^*)^4 [K_c^{1 / 2}/(G^{1 / 2} \rho_c^{2/5}) ]^4 \gamma P^* [K_c\rho_c^{6/5}] \biggl\{ | |||
\frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} | |||
+ \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{K_c^{3}}{ G^{2} \rho_c^{2/5}} \biggr] (r^*)^4 \gamma P^* \biggl\{ | |||
\frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} | |||
+ \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x | |||
\biggr\} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
That is, multiplying the second expression through by, <math>~(K_c/G)\rho_c^{-4 / 5} \cdot G^2 \rho_c^{2 / 5}/K_c^3 = G/(K_c^2 \rho_c^{2 / 5})</math> , should give a desirable, totally dimensionless version of the LAWE. Remembering that, | |||
<div align="center"> | |||
<table border="0" cellpadding="3"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\rho^*</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{\rho_0}{\rho_c}</math> | |||
</td> | |||
<td align="center">; </td> | |||
<td align="right"> | |||
<math>~r^*</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{r_0}{[K_c^{1/2}/(G^{1/2}\rho_c^{2/5})]}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~P^*</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{P_0}{K_c\rho_c^{6/5}}</math> | |||
</td> | |||
<td align="center">; </td> | |||
<td align="right"> | |||
<math>~M_r^*</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{M_r}{[K_c^{3/2}/(G^{3/2}\rho_c^{1/5})]}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\frac{dP^*}{dr^*}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~-\frac{M^*_r \rho^*}{(r^*)^2} </math> | |||
</td> | |||
<td align="center">; </td> | |||
<td align="right"> | |||
<math>~E_\mathrm{norm}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\biggl[ \frac{K_c^5}{G^3}\biggr]^{1 / 2}</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
let's try it. | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~G K_c^{-2} \rho_c^{-2 / 5} \biggl\{ | |||
\frac{d}{dr_0}\biggl[ r_0^4 \gamma P_0 ~\frac{dx}{dr_0} \biggr] | |||
+\biggl[ \omega^2 \rho_0 r_0^4 + (3\gamma - 4) r_0^3 \frac{dP_0}{dr_0} \biggr] x | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~G K_c^{-2} \rho_c^{-2 / 5} \biggl\{ \biggl( \frac{K_c^2 \rho_c^{2/5}}{G} \biggr) | |||
\frac{d}{dr^*}\biggl[ (r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr] | |||
+\biggl[ \omega^2 \biggl(\frac{K_c^2}{G^2 \rho_c^{8/5}}\biggr) \rho_c \rho^* (r^*)^4 + (3\gamma - 4)\biggl(\frac{K_c }{G \rho_c^{4/5}}\biggr)K_c\rho_c^{6/5} (r^*)^3 \frac{dP^*}{dr^*} \biggr] x | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{d}{dr^*}\biggl[ (r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr] | |||
+\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] x \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Now, guided by the [[User:Tohline/SSC/Synopsis_StyleSheet#Stability|accompanying summary]], if we multiply through by <math>~4\pi x dr^*</math> and integrate over the entire volume, we obtain the ''governing variational relation'', namely, | |||
<!-- OLD VERSION; IGNORE | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{R^*}4\pi x\cdot | |||
d\biggl[ (r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr] | |||
+ \int_0^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] 4\pi x^2 dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 4\pi x(r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr]_0^{R^*} | |||
- \int_0^{R^*}4\pi \biggl[ (r^*)^4 \gamma P^* ~\biggl( \frac{dx}{dr^*} \biggr)^2\biggr] dr^* | |||
+ \int_0^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 \biggr] 4\pi x^2 dr^* | |||
- \int_0^{R^*}\biggl[ (3\gamma - 4)M^*_r \rho^* \biggr] 4\pi x^2 r^* dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \int_0^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 \gamma 4\pi (r^*)^2 P^* dr^* | |||
+ \int_0^{R^*} (3\gamma - 4) x^2\biggl( -\frac{M^*_r}{r^*}\biggr) 4\pi \rho^* (r^*)^2 dr^* | |||
-\biggl[ 4\pi x^2 (r^*)^3 \gamma P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_0^{R^*} | |||
+ \int_0^{R^*} 4\pi \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 x^2 dr^* \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
OLD VERSION; IGNORE--> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{r^*_\mathrm{core}}4\pi x\cdot | |||
d\biggl[ (r^*)^4 \gamma_c P^* ~\frac{dx}{dr^*} \biggr] | |||
+ \int_0^{r^*_\mathrm{core}}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma_c - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] 4\pi x^2 dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+\int_{r^*_\mathrm{core}}^{R^*}4\pi x\cdot | |||
d\biggl[ (r^*)^4 \gamma_e P^* ~\frac{dx}{dr^*} \biggr] | |||
+ \int_{r^*_\mathrm{core}}^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma_e - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] 4\pi x^2 dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ 4\pi x(r^*)^4 \gamma_c P^* ~\frac{dx}{dr^*} \biggr]_0^{r^*_\mathrm{core}} | |||
- \int_0^{r^*_\mathrm{core}}4\pi \biggl[ (r^*)^4 \gamma_c P^* ~\biggl( \frac{dx}{dr^*} \biggr)^2\biggr] dr^* | |||
- \int_0^{r^*_\mathrm{core}}\biggl[ (3\gamma_c - 4)M^*_r \rho^* \biggr] 4\pi x^2 r^* dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+\biggl[ 4\pi x(r^*)^4 \gamma_e P^* ~\frac{dx}{dr^*} \biggr]_{r^*_\mathrm{core}}^{R^*} | |||
- \int_{r^*_\mathrm{core}}^{R^*}4\pi \biggl[ (r^*)^4 \gamma_e P^* ~\biggl( \frac{dx}{dr^*} \biggr)^2\biggr] dr^* | |||
- \int_{r^*_\mathrm{core}}^{R^*}\biggl[ (3\gamma_e - 4)M^*_r \rho^* \biggr] 4\pi x^2 r^* dr^* | |||
+ \int_{0}^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 \biggr] 4\pi x^2 dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 \gamma_c 4\pi (r^*)^2 P^* dr^* | |||
+ \int_0^{r^*_\mathrm{core}} (3\gamma_c - 4) x^2\biggl( -\frac{M^*_r}{r^*}\biggr) 4\pi \rho^* (r^*)^2 dr^* | |||
-\biggl[ 4\pi x^2 (r^*)^3 \gamma_c P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_0^{r^*_\mathrm{core}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- \int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 \gamma_e 4\pi (r^*)^2 P^* dr^* | |||
+ \int_{r^*_\mathrm{core}}^{R^*} (3\gamma_e - 4) x^2\biggl( -\frac{M^*_r}{r^*}\biggr) 4\pi \rho^* (r^*)^2 dr^* | |||
-\biggl[ 4\pi x^2 (r^*)^3 \gamma_e P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_{r^*_\mathrm{core}}^{R^*} | |||
+ \int_{0}^{R^*} 4\pi \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 x^2 dr^* \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Energy Normalization: | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~(\gamma - 1)dU_\mathrm{int}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
4\pi r^2 P dr | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
4\pi \biggl[ \frac{K_c^{1 / 2}}{G^{1 / 2} \rho_c^{2 / 5}} \biggr]^3 K_c \rho_c^{6 / 5} (r^*)^2 P^* dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
4\pi \biggl[ \frac{K_c^{5}}{G^{3}} \biggr]^{1 / 2} (r^*)^2 P^* dr^* | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow ~~~ E_\mathrm{norm}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\biggl[ \frac{K_c^{5}}{G^{3}} \biggr]^{1 / 2} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~dW_\mathrm{grav}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\biggl( \frac{GM_r}{r} \biggr) 4\pi r^2 \rho dr | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\biggl( \frac{GM^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* \biggl[ \frac{K_c}{G\rho_c^{4 / 5}} \biggr]\rho_c \biggl[ \frac{ K_c^{3 / 2} }{G^{3 / 2}\rho_c^{1 / 5} } \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
-\biggl( \frac{M^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* E_\mathrm{norm} | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<span id="VariationPrincipleRelation">Hence, the ''dimensionless'' governing variational relation becomes,</span> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\int_0^{R^*} \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2(r^*)^2 x^2 dM_r^* </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\int_0^{r^*_\mathrm{core}} \gamma_c(\gamma_c - 1) x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} | |||
- \int_0^{r^*_\mathrm{core}} (3\gamma_c - 4) x^2 dW^*_\mathrm{grav} | |||
+ \biggl[ 4\pi x^2 (r^*)^3 \gamma_c P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_0^{r^*_\mathrm{core}} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ \int_{r^*_\mathrm{core}}^{R^*} \gamma_e(\gamma_e - 1) x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} | |||
- \int_{r^*_\mathrm{core}}^{R^*} (3\gamma_e - 4) x^2 dW^*_\mathrm{grav} | |||
+ \biggl[ 4\pi x^2 (r^*)^3 \gamma_e P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_{r^*_\mathrm{core}}^{R^*} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} | |||
+ 4\pi x_i^2 (r_\mathrm{core}^*)^3 \gamma_c P_i^* \biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} | |||
- 4\pi x_i^2 (r_\mathrm{core}^*)^3 \gamma_e P_i^* \biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} | |||
- 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^*\biggl[ \gamma_c \biggl\{ \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core} - \gamma_e \biggl\{ \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env} \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} | |||
- (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} | |||
- 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^*\biggl[ 3(\gamma_e - \gamma_c) \biggr] \, , | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
where, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~dM^*_r</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~4\pi (r^*)^2 \rho^* dr^* \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~dU^*_\mathrm{int}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{1}{(\gamma-1)} \biggl[4\pi (r^*)^2 P^* dr^* \biggr] = \biggl[ \frac{2}{3(\gamma - 1)} \biggr]dS^*_\mathrm{therm} \, ,</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~dW^*_\mathrm{grav}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~-\biggl( \frac{M^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
Or, for inclusion in our accompanying ''[[User:Tohline/SSC/Synopsis_StyleSheet#Bipolytropes|Tabular Overview]]'', | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 \int_0^{R^*} (x r^*)^2 dM_r^* </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
\gamma_c (\gamma_c-1) \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} | |||
- (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ ~\gamma_e (\gamma_e-1) \int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} | |||
- (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
| |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
+ ~3^2(\gamma_c - \gamma_e) x_i^2 P_i^* V_\mathrm{core}^* \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
===Implementation=== | |||
<div align="center"> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<th align="center" colspan="15"> | |||
Table 3 | |||
</th> | |||
</tr> | |||
<tr> | |||
<td rowspan="3" align="center"><math>~\frac{\mu_e}{\mu_c}</math></td> | |||
<td rowspan="3" align="center"><math>~\xi_i</math></td> | |||
<td colspan="6" align="center" bgcolor="lightblue">Core</td> | |||
<td colspan="6" align="center" bgcolor="lightgreen">Envelope</td> | |||
<td align="center">Virial</td> | |||
</tr> | |||
<tr> | |||
<td colspan="3" align="center"><font size="-1">Integrals over</font><p></p><math>~dS^*_\mathrm{therm}</math></td> | |||
<td colspan="3" align="center"><font size="-1">Integrals over</font><p></p><math>~dW^*_\mathrm{grav}</math></td> | |||
<td colspan="3" align="center"><font size="-1">Integrals over</font><p></p><math>~dS^*_\mathrm{therm}</math></td> | |||
<td colspan="3" align="center"><font size="-1">Integrals over</font><p></p><math>~dW^*_\mathrm{grav}</math></td> | |||
<td rowspan="2" align="center">Numerical<p></p><math>~\biggl[\frac{2\mathfrak{s}_\mathrm{tot}}{|\mathfrak{w}_\mathrm{tot}|}-1\biggr] </math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">Analytic<br /><math>~\mathfrak{s}_\mathrm{core}</math></td> | |||
<td align="center">Numerical<br /><math>~\mathfrak{s}_\mathrm{core}</math></td> | |||
<td align="center">'''TERM1'''</td> | |||
<td align="center">Analytic<br /><math>~\mathfrak{w}_\mathrm{core}</math></td> | |||
<td align="center">Numerical<br /><math>~\mathfrak{w}_\mathrm{core}</math></td> | |||
<td align="center">'''TERM2'''</td> | |||
<td align="center">Analytic<br /><math>~\mathfrak{s}_\mathrm{env}</math></td> | |||
<td align="center">Numerical<br /><math>~\mathfrak{s}_\mathrm{env}</math></td> | |||
<td align="center">'''TERM3'''</td> | |||
<td align="center">Analytic<br /><math>~\mathfrak{w}_\mathrm{env}</math></td> | |||
<td align="center">Numerical<br /><math>~\mathfrak{w}_\mathrm{env}</math></td> | |||
<td align="center">'''TERM4'''</td> | |||
</tr> | |||
<tr> | |||
<td align="center">1</td> | |||
<td align="left">1.6686460157</td> | |||
<td align="right">3.021916335</td> | |||
<td align="center">3.021921</td> | |||
<td align="center">0.116389175</td> | |||
<td align="center">-3.356583022</td> | |||
<td align="center">-3.35666</td> | |||
<td align="center">-2.649752079</td> | |||
<td align="center">1.47780476</td> | |||
<td align="center">1.47791</td> | |||
<td align="center">1.0720821</td> | |||
<td align="center">-5.642859167</td> | |||
<td align="center">-5.642820</td> | |||
<td align="center">-1.91142893</td> | |||
<td align="center">0.000020</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{2}</math></td> | |||
<td align="left">2.27925811317</td> | |||
<td align="right">4.241287117</td> | |||
<td align="center">4.241410819</td> | |||
<td align="center">0.440878529</td> | |||
<td align="center">-6.074241035</td> | |||
<td align="center">-6.074317546</td> | |||
<td align="center">-4.150731169</td> | |||
<td align="center">4.284931508</td> | |||
<td align="center">4.28547195</td> | |||
<td align="center">1.44651932</td> | |||
<td align="center">-10.97819621</td> | |||
<td align="center">-10.97847622</td> | |||
<td align="center">-0.92598634</td> | |||
<td align="center">0.000057</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.345</td> | |||
<td align="left">2.560146865247</td> | |||
<td align="right">4.639705843</td> | |||
<td align="center">4.6399114</td> | |||
<td align="center">0.6794857</td> | |||
<td align="center">-7.125754184</td> | |||
<td align="center">-7.125854025</td> | |||
<td align="center">-4.5487829</td> | |||
<td align="center">11.72861751</td> | |||
<td align="center">11.730381</td> | |||
<td align="center">1.51410084</td> | |||
<td align="center">-25.61089252</td> | |||
<td align="center">-25.6115597</td> | |||
<td align="center">-0.4496513</td> | |||
<td align="center">0.000097</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{3}</math></td> | |||
<td align="left">2.582007485476</td> | |||
<td align="right">4.667042505</td> | |||
<td align="center">4.667254935</td> | |||
<td align="center">0.700414598</td> | |||
<td align="center">-7.200966267</td> | |||
<td align="center">-7.201068684</td> | |||
<td align="center">-4.57274936</td> | |||
<td align="center">13.15887139</td> | |||
<td align="center">13.1608467</td> | |||
<td align="center">1.51408246</td> | |||
<td align="center">-28.45086152</td> | |||
<td align="center">-28.45153761</td> | |||
<td align="center">-0.4170461</td> | |||
<td align="center">0.000101</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.309</td> | |||
<td align="left">2.6274239687695</td> | |||
<td align="right">4.722277318</td> | |||
<td align="center">4.722504339</td> | |||
<td align="center">0.744964507</td> | |||
<td align="center">-7.354156963</td> | |||
<td align="center">-7.3542507</td> | |||
<td align="center">-4.61961058</td> | |||
<td align="center">17.1374434</td> | |||
<td align="center">17.1399773</td> | |||
<td align="center">1.51055838</td> | |||
<td align="center">-36.36528446</td> | |||
<td align="center">-36.36591543</td> | |||
<td align="center">-0.3524855</td> | |||
<td align="center">0.000110</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{4}</math></td> | |||
<td align="left">2.7357711469398</td> | |||
<td align="right">4.84592201</td> | |||
<td align="center">4.846185027</td> | |||
<td align="center">0.857001395</td> | |||
<td align="center">-7.70305421</td> | |||
<td align="center">-7.703178009</td> | |||
<td align="center">-4.7163542</td> | |||
<td align="center">37.84289623</td> | |||
<td align="center">37.8479208</td> | |||
<td align="center">1.47966673</td> | |||
<td align="center">-77.67458196</td> | |||
<td align="center">-77.67408155</td> | |||
<td align="center">-0.2194152</td> | |||
<td align="center">0.000128</td> | |||
</tr> | |||
<tr> | |||
<td colspan="15" align="left"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{s}_\mathrm{core}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{r^*_\mathrm{core}} dS^*_\mathrm{therm}</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
'''TERM1''' | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{w}_\mathrm{core}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \int_0^{r^*_\mathrm{core}} dW^*_\mathrm{grav}</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
'''TERM2''' | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{s}_\mathrm{env}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_{r^*_\mathrm{core}}^{R^*} dS^*_\mathrm{therm}</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
'''TERM3''' | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{w}_\mathrm{env}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \int_{r^*_\mathrm{core}}^{R^*} dW^*_\mathrm{grav}</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
'''TERM4''' | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav}</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td colspan="7" align="center">NOTE: In all integrals, the fractional radial-displacement function, <math>~x</math>, has been normalized<br />to unity at the center of the spherical model.</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\frac{3}{2\pi \cdot \mathbf{TERM5} } \biggl\{ | |||
\frac{2\gamma_c}{3} [\mathbf{TERM1}] | |||
- (3\gamma_c - 4) [\mathbf{TERM2}] | |||
+ \frac{2 \gamma_e}{3} [\mathbf{TERM3}] | |||
- (3\gamma_e - 4) [\mathbf{TERM4}] | |||
- 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^* [3(\gamma_e - \gamma_c)] | |||
\biggr\} | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.036312577\times \biggl[0.093111340 - 1.059900832 + 1.4294428 - (-3.82285786) - 1.782200484\times (2.4) \biggr] = 0.036312577\times [0.00819] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.0002973 \, .</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 2}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.020704186\times \biggl[0.352702823 - 1.660292468 + 1.928692426 - (-1.85197268) - 1.029029184\times (2.4) \biggr] = 0.020704186\times [0.003405] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.0000705 \, .</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 0.345}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.019377703\times \biggl[0.54358856 - 1.81951316 + 2.01880112 - (-0.8993026) - 0.68747441\times (2.4) \biggr] = 0.019377703\times [0.003585] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.0000695 \, .</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 3}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.019467503\times \biggl[0.560331678 - 1.81099744 + 2.018776613 - (-0.8340922) - 0.658354814\times (2.4) \biggr] = 0.019467503\times [1.602203052 - 1.580051555] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.00043123 \, .</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 0.309}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.019781992\times \biggl[0.595971606 - 1.847844232 + 2.01407784 - (-0.704971) - 0.60905486\times (2.4) \biggr] = 0.019781992\times [1.467176214 - 1.461731665] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.0001077 \, .</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 4}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.021344909\times \biggl[0.685601116 - 1.88654168 + 1.972888973 - (-0.4388304) - 0.499262049\times (2.4) \biggr] = 0.021344909\times [1.210778809 - 1.198228918] </math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
0.000267876 \, .</math> | |||
</td> | |||
</tr> | |||
</table> | |||
<table border="1" cellpadding="8" align="center"> | |||
<tr> | |||
<th align="center" colspan="11"> | |||
Table 4 | |||
</th> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\frac{\mu_e}{\mu_c}</math></td> | |||
<td align="center"><math>~\xi_i</math></td> | |||
<td align="center">Analytic<br /><math>~\mathfrak{m}_\mathrm{tot}</math></td> | |||
<td align="center">Numerical<br /><math>~\mathfrak{m}_\mathrm{tot}</math></td> | |||
<td align="center">'''TERM5'''</td> | |||
<td align="center"><math>~x_i</math></td> | |||
<td align="center"><math>~r^*_\mathrm{core}</math></td> | |||
<td align="center"><math>~P^*_i</math></td> | |||
<td align="center"><math>~\biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core}</math></td> | |||
<td align="center"><math>~\biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env}</math></td> | |||
<td align="center"><math>~\biggl[\sigma_c^2\biggr]^\mathrm{VP}</math></td> | |||
</tr> | |||
<tr> | |||
<td align="center">1</td> | |||
<td align="left">1.6686460157</td> | |||
<td align="right">4.818155928</td> | |||
<td align="center">4.818145</td> | |||
<td align="center">13.14874521</td> | |||
<td align="center">0.814374698</td> | |||
<td align="center">1.153014872</td> | |||
<td align="center">0.139506172</td> | |||
<td align="center">+0.455871977</td> | |||
<td align="center">+1.47352</td> | |||
<td align="center">0.0002973</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{2}</math></td> | |||
<td align="left">2.27925811317</td> | |||
<td align="right">9.020985415</td> | |||
<td align="center">9.021084268</td> | |||
<td align="center">23.0612702</td> | |||
<td align="center">0.653665497</td> | |||
<td align="center">1.574940686</td> | |||
<td align="center">0.049058481</td> | |||
<td align="center">+1.059668912</td> | |||
<td align="center">+1.835801347</td> | |||
<td align="center">0.0000705</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.345</td> | |||
<td align="left">2.560146865247</td> | |||
<td align="right">17.41399388</td> | |||
<td align="center">17.4141672</td> | |||
<td align="center">24.63990825</td> | |||
<td align="center">0.563043202</td> | |||
<td align="center">1.769031527</td> | |||
<td align="center">0.030957085</td> | |||
<td align="center">+1.552125296</td> | |||
<td align="center">+2.131275177</td> | |||
<td align="center">0.0000695</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{3}</math></td> | |||
<td align="left">2.582007485476</td> | |||
<td align="right">18.8449906</td> | |||
<td align="center">18.8451614</td> | |||
<td align="center">24.52624951</td> | |||
<td align="center">0.555549156</td> | |||
<td align="center">1.78413696</td> | |||
<td align="center">0.029889634</td> | |||
<td align="center">+1.600041467</td> | |||
<td align="center">+2.16002488</td> | |||
<td align="center">0.0004312</td> | |||
</tr> | |||
<tr> | |||
<td align="center">0.309</td> | |||
<td align="left">2.6274239687695</td> | |||
<td align="right">22.61541791</td> | |||
<td align="center">22.6155686</td> | |||
<td align="center">24.13633675</td> | |||
<td align="center">0.539776219</td> | |||
<td align="center">1.815519219</td> | |||
<td align="center">0.027798189</td> | |||
<td align="center">+1.705239188</td> | |||
<td align="center">+2.223143513</td> | |||
<td align="center">0.0001077</td> | |||
</tr> | |||
<tr> | |||
<td align="center"><math>~\tfrac{1}{4}</math></td> | |||
<td align="left">2.7357711469398</td> | |||
<td align="right">39.12088278</td> | |||
<td align="center">39.1208075</td> | |||
<td align="center">22.36902623</td> | |||
<td align="center">0.501037082</td> | |||
<td align="center">1.890385851</td> | |||
<td align="center">0.023427588</td> | |||
<td align="center">+1.991720516</td> | |||
<td align="center">+2.39503231</td> | |||
<td align="center">0.0002679</td> | |||
</tr> | |||
<tr> | |||
<td colspan="11" align="left"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~\mathfrak{m}_\mathrm{tot}</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{R^*}dM_r^*</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
<math>~I_\mathrm{sphere}^*</math> | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{R^*}(r^*)^2 dM_r^*</math> | |||
</td> | |||
<td align="center"> ; </td> | |||
<td align="right"> | |||
'''TERM5''' | |||
</td> | |||
<td align="center"> | |||
<math>~\equiv</math> | |||
</td> | |||
<td align="left"> | |||
<math>~\int_0^{R^*}(r^*)^2 x^2 dM_r^*</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td colspan="11" align="center">NOTE: In the '''TERM5''' integral (as elsewhere), the fractional radial-displacement function, <math>~x</math>, <br />has been normalized to unity at the center of the spherical model.</td> | |||
</tr> | |||
</table> | |||
</td> | |||
</tr> | |||
</table> | |||
==Revised Free-Energy Analysis== | |||
If we set <math>~x </math> = constant in the [[#VariationPrincipleRelation|variational principle relation]], we have, | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~ \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 \int_0^{R^*}(r^*)^2dM_r^* </math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ | |||
- (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} dW^*_\mathrm{grav} | |||
- (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} dW^*_\mathrm{grav} | |||
- 4\pi (r_\mathrm{core}^*)^3 P_i^*\biggl[ 3(\gamma_e - \gamma_c) \biggr] | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
<math>~\Rightarrow~~~~~~ \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 I_\mathrm{sphere}^* </math> | |||
</td> | </td> | ||
<td align="center"> | <td align="center"> | ||
| Line 1,958: | Line 4,190: | ||
<td align="left"> | <td align="left"> | ||
<math>~ | <math>~ | ||
\ | (4 - 3\gamma_c )\mathfrak{w}_\mathrm{core} | ||
+ (4 - 3\gamma_e ) \mathfrak{w}_\mathrm{env} | |||
+ P_i^*V^*_\mathrm{core}\biggl[ 3^2(\gamma_c - \gamma_e) \biggr] \, , | |||
</math> | </math> | ||
</td> | </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
Notice the similarity between this last expression and the pair of expressions — numbered <b><font color="maroon" size="+1">⑥</font></b> and <b><font color="maroon" size="+1">⑦</font></b> — that arise in the context of [[User:Tohline/SSC/Synopsis_StyleSheet#Stability|pressure-truncated polytropes]]. | |||
=See Also= | =See Also= | ||
Latest revision as of 22:35, 15 May 2019
Marginally Unstable (nc,ne) = (5,1) Bipolytropes

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Overview
| Figure 1: Equilibrium Sequences of Pressure-Truncated Polytropes |
|---|
We expect the content of this chapter — which examines the relative stability of bipolytropes — to parallel in many ways the content of an accompanying chapter in which we have successfully analyzed the relative stability of pressure-truncated polytopes. Figure 1, shown here on the right, has been copied from a closely related discussion. The curves show the mass-radius relationship for pressure-truncated model sequences having a variety of polytropic indexes, as labeled, over the range <math>1 \le n \le 6</math>. (Another version of this figure includes the isothermal sequence.) On each sequence for which <math>~n \ge 3</math>, the green filled circle identifies the model with the largest mass. We have shown analytically that the oscillation frequency of the fundamental-mode of radial oscillation is precisely zero† for each one of these maximum-mass models. As a consequence, we know that each green circular marker identifies the point along its associated sequence that separates dynamically stable (larger radii) from dynamically unstable (smaller radii) models.
†In each case, the fundamental-mode oscillation frequency is precisely zero if, and only if, the adiabatic index governing expansions/contractions is related to the underlying structural polytropic index via the relation, <math>~\gamma_g = (n + 1)/n</math>, and if a constant surface-pressure boundary condition is imposed.
In another accompanying chapter, we have used purely analytic techniques to construct equilibrium sequences of spherically symmetric bipolytropes that have, <math>~(n_c,n_e) = (5,1)</math>. For a given choice of <math>~\mu_e/\mu_c</math> — the ratio of the mean-molecular weight of envelope material to the mean-molecular weight of material in the core — a physically relevant sequence of models can be constructed by steadily increasing the value of the dimensionless radius at the core/envelope interface, <math>~\xi_i</math>, from zero to infinity. Figure 2, whose content is essentially the same as Figure 1 of this separate chapter, shows how the fractional core mass, <math>\nu \equiv M_\mathrm{core}/M_\mathrm{tot}</math>, varies with the fractional core radius, <math>q \equiv r_\mathrm{core}/R</math>, along sequences having seven different values of <math>~\mu_e/\mu_c</math>, as labeled: 1 (black), ½ (dark blue), 0.345 (brown), ⅓ (dark green), 0.316943 (purple), 0.309 (orange), and ¼ (light blue).
When modeling bipolytropes, the default expectation is that an increase in <math>~\xi_i</math> along a given sequence will correspond to an increase in the relative size — both the radius and the mass — of the core. This expectation is realized along the Figure 2 sequences that have the largest mean-molecular weight ratios: <math>~\mu_e/\mu_c</math> = 1 and ½. But the behavior is different along the other five illustrated sequences. For sufficiently large <math>~\xi_i</math>, the relative radius of the core begins to decrease; along each sequence, a solid purple circular marker identifies the location of this turning point in radius. Furthermore, along sequences for which <math>~\mu_e/\mu_c < \tfrac{1}{3}</math>, eventually the fractional mass of the core reaches a maximum and, thereafter, decreases even as the value of <math>~\xi_i</math> continues to increase; a solid green circular marker identifies the location of this maximum mass turning point along each of these sequences. (Additional properties of these equilibrium sequences are discussed in yet another accompanying chapter.)
The principal question is: Along bipolytropic sequences, are maximum-mass models associated with the onset of dynamical instabilities?
Planned Approach
| Figure 2: Equilibrium Sequences of Bipolytropes with <math>~(n_c,n_e) = (5,1)</math> and Various <math>~\mu_e/\mu_c</math> |
|
|---|---|
Ideally we would like to answer the just-stated "principal question" using purely analytic techniques. But, to date, we have been unable to fully address the relevant issues analytically, even in what would be expected to be the simplest case: bipolytropic models that have <math>~(n_c,n_e) = (0, 0)</math>. Instead, we will streamline the investigation a bit and proceed — at least initially — using a blend of techniques. We will investigate the relative stability of bipolytropic models having <math>~(n_c,n_e) = (5,1) </math> whose equilibrium structures are completely defined analytically; then the eigenvectors describing radial modes of oscillation will be determined, one at a time, by solving the relevant LAWE(s) numerically. We are optimistic that this can be successfully accomplished because we have had experience numerically integrating the LAWE that governs the oscillation of:
- Isolated n = 3 polytropes — including a quantitative comparison against Schwarzschild's (1941) published work;
- Pressure-truncated isothermal spheres — including a quantitative comparison against the published analysis of Taff & Van Horn (1974); and
- Pressure-truncated n = 5 polytropes.
A key reference throughout this investigation will be the paper by J. O. Murphy & R. Fiedler (1985b, Proc. Astr. Soc. of Australia, 6, 222). They studied Radial Pulsations and Vibrational Stability of a Sequence of Two Zone Polytropic Stellar Models. Specifically, their underlying equilibrium models were bipolytropes that have <math>~(n_c,n_e) = (1, 5)</math>. In an accompanying chapter, we describe in detail how Murphy & Fiedler obtained these equilibrium bipolytropic structures and detail some of their equilibrium properties.
Here are the steps we initially plan to take:
- Governing LAWEs:
- Identify the relevant LAWEs that govern the behavior of radial oscillations in the <math>~n_c = 5</math> core and, separately, in the <math>~n_e = 1</math> envelope. Check these LAWE specifications against the published work of Murphy & Fiedler (1985b).
- Determine the matching conditions that must be satisfied across the core/envelope interface. Be sure to take into account the critical interface jump conditions spelled out by P. Ledoux & Th. Walraven (1958), as we have already discussed in the context of an analysis of radial oscillations in zero-zero bipolytropes.
- Determine what surface boundary condition should be imposed on physically relevant LAWE solutions, i.e., on the physically relevant radial-oscillation eigenvectors.
- Initial Analysis:
- Choose a maximum-mass model along the bipolytropic sequence that has, for example, <math>~\mu_e/\mu_c = 1/4</math>. Hopefully, we will be able to identify precisely (analytically) where this maximum-mass model lies along the sequence. Yes! Our earlier analysis does provide an analytic prescription of the model that sits at the maximum-mass location along the chosen sequence.
- Solve the relevant eigenvalue problem for this specific model, initially for <math>~(\gamma_c, \gamma_e) = (6/5, 2)</math> and initially for the fundamental mode of oscillation.
Virial Analysis
In an accompanying chapter we have examined the viral stability of <math>~(n_c, n_e) = (5, 1)</math> bipolytropes — see also an associated discussion of the free-energy of these configurations. We present, here, an overview of this separate discussion in an effort to provide a broader perspective to the focus of this current chapter.
Various Energy Expressions
Drawing from our accompanying detailed discussion, we have,
|
<math>~\mathfrak{G}</math> |
<math>~=</math> |
<math>~W_\mathrm{grav}\biggr|_\mathrm{core} + \mathfrak{S}_A\biggr|_\mathrm{core} + W_\mathrm{grav}\biggr|_\mathrm{env} + \mathfrak{S}_A\biggr|_\mathrm{env} </math> |
|
|
<math>~=</math> |
<math> ~W_\mathrm{grav}\biggr|_\mathrm{core} + \biggl[ \frac{2}{3(\gamma_c - 1)} \biggr] S_\mathrm{core} + W_\mathrm{grav}\biggr|_\mathrm{env} + \biggl[ \frac{2}{3(\gamma_e - 1)} \biggr] S_\mathrm{env} \, . </math> |
Recognizing that,
|
<math>~q</math> |
<math>~\equiv</math> |
<math>~\frac{r_i}{R} = \frac{\eta_i}{\eta_s} \, ,</math> |
|
<math>~\nu</math> |
<math>~\equiv</math> |
<math>~\frac{M_\mathrm{core}}{M_\mathrm{tot}} \, ,</math> |
|
<math>~\ell</math> |
<math>~\equiv</math> |
<math>~\frac{\xi}{\sqrt{3}} \, ,</math> |
|
<math>~\chi_\mathrm{eq} </math> |
<math>~=</math> |
<math>~\biggl( \frac{\pi}{2^3 \cdot 3^6}\biggr)^{1 / 2} \biggl( \frac{\nu}{q^3} \biggr)^{2} \biggl( 1 + \ell_i^2 \biggr)^{3} \biggl( \frac{q}{\ell_i}\biggr)^{5 } \, , </math> |
we have,
|
<math>~- \chi \biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{core}</math> |
<math>~=</math> |
<math> \biggl[\biggl(\frac{3}{2^4}\biggr) \biggl( \frac{q}{\ell_i}\biggr)^{5}\biggl(\frac{\nu}{q^3} \biggr)^2 \biggl( 1 + \ell_i^2 \biggr)^{3} \biggr]_\mathrm{eq} \biggl[ \ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i \biggr] </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{core}</math> |
<math>~=</math> |
<math>- \frac{\chi_\mathrm{eq}}{\chi} \biggl( \frac{3^8}{2^5 \pi}\biggr)^{1 / 2} \biggl[ \ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i \biggr] \, . </math> |
Next, given that,
|
<math>~b_\eta = \eta_s</math> |
and |
<math>~b_\eta q = \eta_i = 3\biggl( \frac{\mu_e}{\mu_c} \biggr) \ell_i (1 + \ell_i^2)^{-1} \, ,</math> |
we have,
|
<math>~\biggl[ \frac{W_\mathrm{grav}}{E_\mathrm{norm}} \biggr]_\mathrm{env}</math> |
<math>~=</math> |
<math> - \frac{\chi_\mathrm{eq}}{\chi} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 \biggl[6 b_\eta x - 3\sin[2(b_\eta x-B)] - 4b_\eta x \sin^2(b_\eta x - B) \biggr]_q^1 </math> |
|
|
<math>~=</math> |
<math> - \frac{\chi_\mathrm{eq}}{\chi} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 \biggl\{ \biggl[ 6 \eta_s - 3\sin[2(\eta_s -B)] - 4\eta_s \sin^2(\eta_s - B) \biggr] - \biggl[ 6 \eta_i - 3\sin[2(\eta_i -B)] - 4\eta_i \sin^2(\eta_i - B) \biggr] \biggr\} \, . </math> |
Also, given that,
|
<math>~q a_\xi^{1 / 2}</math> |
<math>~=</math> |
<math>~\frac{\xi_i}{\sqrt{3}} \, ,</math> |
we have,
|
<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{core}</math> |
<math>~=</math> |
<math> \frac{2}{3({\gamma_c}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_c} \biggl\{\biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ \tan^{-1}[a_\xi^{1/2}q] - a_\xi^{1/2}q ~\frac{(1 - a_\xi q^2)}{(1 + a_\xi q^2)^2} \biggr] \biggr\} </math> |
|
|
<math>~=</math> |
<math> \frac{2}{3({\gamma_c}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_c} \biggl\{\biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ \tan^{-1}\biggl( \frac{\xi_i}{\sqrt{3}}\biggr) - \biggl( \frac{\xi_i}{\sqrt{3}}\biggr)\frac{(1 - \xi_i^2/3)}{(1 + \xi_i^2/3)^2} \biggr] \biggr\} </math> |
Finally, then, we can write,
|
<math>~\biggl( \frac{\mathfrak{S}_A}{E_\mathrm{norm}} \biggr)_\mathrm{env}</math> |
<math>~=</math> |
<math> \frac{2}{3({\gamma_e}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_e} \biggl\{ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl[ 2b_\eta x -\sin[2(b_\eta x - B)] \biggr]_q^1 \biggr\} </math> |
|
|
|
<math>~=</math> |
<math> \frac{2}{3({\gamma_e}-1)} \biggl( \frac{\chi}{\chi_\mathrm{eq}} \biggr)^{3-3\gamma_e} \bigg( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl\{ \biggl[ 2\eta_s -\sin[2(\eta_s - B)] \biggr] - \biggl[ 2\eta_i -\sin[2(\eta_i - B)] \biggr] \biggr\} \ . </math> |
Free Energy and Its Derivatives
We can now rewrite the free-energy expression in the form,
|
<math>~\mathfrak{g} \equiv \frac{\mathfrak{G}}{E_\mathrm{norm}}</math> |
<math>~=</math> |
<math> ~-\biggl(\frac{\chi_\mathrm{eq}}{\chi}\biggr)\mathfrak{w} + \biggl[ \frac{2}{3(\gamma_c - 1)} \biggr] \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{3 - 3\gamma_c}\mathfrak{s}_\mathrm{core} + \biggl[ \frac{2}{3(\gamma_e - 1)} \biggr] \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{3 - 3\gamma_e}\mathfrak{s}_\mathrm{env} \, , </math> |
where,
|
<math>~\mathfrak{s}_\mathrm{core}</math> |
<math>~\equiv</math> |
<math>~ \biggl( \frac{3^8}{2^7\pi} \biggr)^{1/2} \biggl[ \tan^{-1}\biggl( \frac{\xi_i}{\sqrt{3}}\biggr) - \biggl( \frac{\xi_i}{\sqrt{3}}\biggr)\frac{(1 - \xi_i^2/3)}{(1 + \xi_i^2/3)^2} \biggr]\, , </math> |
|
<math>~\mathfrak{s}_\mathrm{env}</math> |
<math>~\equiv</math> |
<math>~ \bigg( \frac{\mu_e}{\mu_c} \biggr)^{-3} A^2 \biggl( \frac{3^2}{2^5\pi} \biggr)^{1/2} \biggl\{ \biggl[ 2\eta_s -\sin[2(\eta_s - B)] \biggr] - \biggl[ 2\eta_i -\sin[2(\eta_i - B)] \biggr] \biggr\} \, , </math> |
|
<math>~\mathfrak{w}</math> |
<math>~\equiv</math> |
<math>~ \biggl( \frac{3^8}{2^5 \pi}\biggr)^{1 / 2} \biggl[ \ell_i (\ell_i^4 - \frac{8}{3}\ell_i^2 - 1) (\ell_i^2 +1)^{-3} + \tan^{-1}\ell_i \biggr] </math> |
|
|
|
<math>~ +~ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-3} \biggl( \frac{1}{2^3\pi} \biggr)^{1/2} A^2 \biggl\{ \biggl[ 6 \eta_s - 3\sin[2(\eta_s -B)] - 4\eta_s \sin^2(\eta_s - B) \biggr] - \biggl[ 6 \eta_i - 3\sin[2(\eta_i -B)] - 4\eta_i \sin^2(\eta_i - B) \biggr] \biggr\} \, . </math> |
The first derivative is,
|
<math>~\frac{d\mathfrak{g}}{d\chi} </math> |
<math>~=</math> |
<math> +~\biggl(\frac{\chi_\mathrm{eq}}{\chi^2}\biggr)\mathfrak{w} ~-~\frac{2}{\chi_\mathrm{eq}} \biggl[\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{2 - 3\gamma_c}\mathfrak{s}_\mathrm{core} ~+~\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{2 - 3\gamma_e}\mathfrak{s}_\mathrm{env} \biggr] \, . </math> |
And the second derivative is,
|
<math>~\frac{d^2\mathfrak{g}}{d\chi^2} </math> |
<math>~=</math> |
<math> -~2\biggl(\frac{\chi_\mathrm{eq}}{\chi^3}\biggr)\mathfrak{w} ~-~\frac{2}{\chi_\mathrm{eq}^2} \biggl[(2 - 3\gamma_c) \biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{1 - 3\gamma_c}\mathfrak{s}_\mathrm{core} ~+~(2 - 3\gamma_e)\biggl(\frac{\chi}{\chi_\mathrm{eq}}\biggr)^{1 - 3\gamma_e}\mathfrak{s}_\mathrm{env} \biggr] \ . </math> |
What to Expect for Equilibrium Configurations
In equilibrium we should set <math>~d\mathfrak{g}/d\chi = 0</math> and <math>~\chi = \chi_\mathrm{eq}</math>. In which case we should expect to find that,
|
<math>~0 </math> |
<math>~=</math> |
<math> \frac{1}{\chi_\mathrm{eq}}\biggl[\mathfrak{w} ~-~2(\mathfrak{s}_\mathrm{core} ~+~\mathfrak{s}_\mathrm{env}) \biggr] \, . </math> |
In addition, an evaluation of the second derivative should give,
|
<math>~\frac{\chi_\mathrm{eq}^2}{2} \cdot \frac{d^2\mathfrak{g}}{d\chi^2} </math> |
<math>~=</math> |
<math> -~\mathfrak{w} ~-~ \biggl[(2 - 3\gamma_c) \mathfrak{s}_\mathrm{core} ~+~(2 - 3\gamma_e)\mathfrak{s}_\mathrm{env} \biggr] \, . </math> |
Then, the transition from stable to unstable configurations occurs when <math>~d^2\mathfrak{g}/d\chi^2 = 0</math>, that is, when,
|
<math>~\mathfrak{w} </math> |
<math>~=</math> |
<math> ~-~ \biggl[(2 - 3\gamma_c) \mathfrak{s}_\mathrm{core} ~+~(2 - 3\gamma_e)\mathfrak{s}_\mathrm{env} \biggr] </math> |
|
<math>~\Rightarrow ~~~0</math> |
<math>~=</math> |
<math> ~(4 - 3\gamma_c) \mathfrak{s}_\mathrm{core} ~+~(4 - 3\gamma_e)\mathfrak{s}_\mathrm{env} </math> |
|
<math>~\Rightarrow ~~~\frac{\mathfrak{s}_\mathrm{core} }{\mathfrak{s}_\mathrm{env}} </math> |
<math>~=</math> |
<math> ~-~\frac{(4 - 3\gamma_e) }{(4 - 3\gamma_c) } \, . </math> |
For example, if we set <math>~\gamma_c = 6/5</math> and <math>~\gamma_e = 2</math>, this implies,
|
<math>~\frac{\mathfrak{s}_\mathrm{core} }{\mathfrak{s}_\mathrm{env}} </math> |
<math>~=</math> |
<math> ~\frac{2 }{(4 - 18/5) } = 5 \, . </math> |
Free-Energy Stability Evaluation
Here we pull together excerpts from several different H_Book Chapters in which we have presented, from several different perspectives, an analysis of the free-energy of bipolytropes.
- In one chapter, using purely analytic techniques, we have derived expressions that detail the structural properties of bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>. Among these are analytic expressions for various terms that make up the free-energy expression: <math>~\mathfrak{s}_\mathrm{core}</math>, <math>~\mathfrak{s}_\mathrm{env}</math>, <math>~\mathfrak{w}_\mathrm{core}</math>, <math>~\mathfrak{w}_\mathrm{core}</math>, and <math>~P_iV_\mathrm{core}</math>. Equilibrium model sequences are defined by fixing the ratio, <math>~\mu_e/\mu_c</math>, then varying the radial location, <math>~r_i</math>, of the core-envelope interface; note that the volume of the core is, then, <math>~V_\mathrm{core} \equiv 4\pi r_i^3/3</math>.
- [Virial Equilibrium] In a subsection of this same chapter titled, Equilibrium Condition: Global, we have shown that a statement of virial equilibrium — obtained by setting the first derivative of the free-energy expression to zero — is,
In another subsection of this same chapter titled, Equilibrium Condition: In Parts, we showed that for each bipolytropic equilibrium structure, the statements<math>~( 2S + W )_\mathrm{tot} ~=~ 2(S_\mathrm{core} + S_\mathrm{env}) + (W_\mathrm{core} + W_\mathrm{env})</math>
<math>~=~</math>
<math>~ 0 \, .</math>
also hold separately. Therefore, for every equilibrium configuration we should expect the CASE1 expression (see Table XXX) to precisely sum to unity.<math>~2S_\mathrm{core} + W_\mathrm{core} = 3P_i V_\mathrm{core}</math>
and
<math>~2S_\mathrm{env} + W_\mathrm{env} = - 3P_i V_\mathrm{core} \, ,</math>
- [Marginally Unstable Model] Near the bottom of this same chapter, in a subsection titled, Stability Condition, we point out that the model along each sequence that is marginally (dynamically) unstable — obtained setting the second derivative of the free-energy expression to zero — is identified by the configuration for which,
Therefore, along each equilibrium sequence, the marginally unstable model can be identified by the configuration for which the CASE2 expression (see Table XXX) precisely sums to zero. Immediately above, in a subsection titled, What to Expect for Equilibrium Configurations, we have shown that this same marginally unstable model can be identified by the configuration for which the CASE3 energy ratio, <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env}</math>, has a value that is precisely 5. And, thirdly, as highlighted in our accompanying Tabular Overview, this same marginally unstable model can be identified by the configuration for which the CASE4 expression precisely sums to zero.<math>~ 2(\gamma_e - \gamma_c) \mathfrak{s}_\mathrm{core} + ( \mathfrak{w}_\mathrm{core} + \mathfrak{w}_\mathrm{env} )(\gamma_e - \tfrac{4}{3}) </math>
<math>~=</math>
<math>~0 \, .</math>
|
Table XXX: Properties of Marginally Unstable Bipolytropes Having |
|||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| <math>~\frac{\mu_e}{\mu_c}</math> | <math>~\xi_i</math> | <math>~R^*_\mathrm{surf}</math> | <math>~q \equiv \frac{r_\mathrm{core}}{R_\mathrm{surf}}</math> | <math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math> | <math>~\frac{\rho_c}{\bar\rho}</math> | <math>~P_i</math> | <math>~\mathfrak{s}_\mathrm{core}</math> | <math>~\mathfrak{w}_\mathrm{core}</math> | <math>~\mathfrak{s}_\mathrm{env}</math> | <math>~\mathfrak{w}_\mathrm{env}</math> | <math>~3P_i V_\mathrm{core}</math> | CASE1 | CASE2 | CASE3 | CASE4 | ||||||||||||
| 1 | 2.41610822 | 2.8049 | 0.59520261 | 0.68306067 | 16.3788 | 0.039116848 | 4.446748782 | - 6.606135366 | 0.889349762 | - 4.066061722 | 2.287362198 | 1 | 0 | 5.0000000 | 2.1 × 10-8 | ||||||||||||
| <math>~\tfrac{1}{2}</math> | 4.1853093 | 8.8058 | 0.328419479 | 0.70131896 | 354.089 | 0.003126324 | 5.76978580 | - 10.58931853 | 1.153956968 | - 3.258165567 | 0.95025163 | 1 | 6.3 × 10-8 | 5.0000002 | 0 | ||||||||||||
| 0.345 | 7.64325 | 44.116 | 0.119714454 | 0.52700045 | 2.85 × 104 | 0.000116533 | 6.230343527 | - 12.24495934 | 1.1246068658 | - 2.707865028 | 0.215727713 | 1 | 6.4 × 10-8 | 5.0000002 | 0 | ||||||||||||
| <math>~\tfrac{1}{3}</math> | 8.548103 | 59.643 | 0.099032423 | 0.47901529 | 6.30 × 104 | 6.1337 × 10-5 | 6.261548334 | - 12.36425897 | 1.252309682 | - 2.663457063 | 0.158837699 | 1 | 0 | 4.9999999 | 6.0 × 10-8 | ||||||||||||
| 0.316943 | 10.7441565 | 108.14 | 0.068655205 | 0.38238387 | 2.93 × 105 | 1.6252 × 10-5 | 6.301810768 | - 12.52005323 | 1.260362204 | - 2.604292714 | 0.083568307 | 1 | 0 | 4.9999998 | 2.0 × 10-7 | ||||||||||||
| 0.309 | 12.77156 | 166.06 | 0.053145011 | 0.31696879 | 8.70 × 105 | 5.8905 × 10-6 | 6.318902171 | - 12.58692884 | 1.26378042 | - 2.57843634 | 0.050875500 | 1 | 1.9 × 10-8 | 5.0000001 | 0 | ||||||||||||
|
|||||||||||||||||||||||||||
The left-hand panel of Figure 3 is identical to Figure 2, above. It displays in the <math>~q-\nu</math> parameter space, the behavior of <math>~(n_c, n_e) = (5, 1)</math> bipolytropic equilibrium sequences that have, as labeled, seven different values of the ratio of mean-molecular-weights, <math>~\mu_e/\mu_c</math>. Using a numerical root-finding technique, we have determined where the virial stability condition, <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env} = 5</math>, is satisfied along each of these sequences — as well as along a number of additional equilibrium sequences. Key properties of each of these identified models have been recorded in Table 1 of an accompanying discussion; see also an associated discussion of the free-energy of these configurations. Pulling from this tabulated data, the solid-red circular markers that appear in the right-hand panel of Figure 3 identify where this virial stability condition is satisfied along the separate equilibrium sequences while the accompanying dashed red curve identifies more broadly how the <math>~q-\nu</math> parameter space is divided into stable (below and to the right) versus unstable (above and to the left) regions.
| Figure 3 |
|---|
In what follows we use a complementary — and more quantitatively rigorous — approach to evaluating the stability of equilibrium models, and contrast the results of that analysis with the virial-analysis results presented graphically here in Figure 3.
Review of the Analysis by Murphy & Fiedler (1985b)
As we have detailed separately, the boundary condition at the center of a polytropic configuration is,
<math>~\frac{dx}{d\xi} \biggr|_{\xi=0} = 0 \, ;</math>
and the boundary condition at the surface of an isolated polytropic configuration is,
|
<math>~\frac{d\ln x}{d\ln\xi}</math> |
<math>~=</math> |
<math>~- \alpha + \frac{\omega^2}{\gamma_g } \biggl( \frac{1}{4\pi G \rho_c } \biggr) \frac{\xi}{(-\theta^')} </math> at <math>~\xi = \xi_s \, .</math> |
But this surface condition is not applicable to bipolytropes. Instead, let's return to the original, more general expression of the surface boundary condition:
|
<math>~ \frac{d\ln x}{d\ln\xi}\biggr|_s</math> |
<math>~=</math> |
<math>~- \alpha + \frac{\omega^2 R^3}{\gamma_g GM_\mathrm{tot}} \, .</math> |
|
Utilizing an accompanying discussion, let's examine the frequency normalization used by Murphy & Fiedler (1985b) (see the top of the left-hand column on p. 223):
For a given radial quantum number, <math>~k</math>, the factor inside the square brackets in this last expression is what Murphy & Fiedler (1985b) refer to as <math>~\omega^2_k \theta_c</math>. Keep in mind, as well, that, in the notation we are using,
This also means that the surface boundary condition may be rewritten as,
|
Let's apply these relations to the core and envelope, separately.
Interface Conditions
Here, we will simply copy the discussion already provided in the context of our attempt to analyze the stability of <math>~(n_c, n_e) = (0, 0)</math> bipolytropes; specifically, we will draw from STEP 4: in the Piecing Together subsection. Following the discussion in §§57 & 58 of P. Ledoux & Th. Walraven (1958), the proper treatment is to ensure that fractional perturbation in the gas pressure (see their equation 57.31),
|
<math>~\frac{\delta P}{P}</math> |
<math>~=</math> |
<math>~- \gamma x \biggl( 3 + \frac{d\ln x}{d\ln \xi} \biggr) \, ,</math> |
is continuous across the interface. That is to say, at the interface <math>~(\xi = \xi_i)</math>, we need to enforce the relation,
|
<math>~0</math> |
<math>~=</math> |
<math>~\biggl[ \gamma_c x_\mathrm{core} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \gamma_e x_\mathrm{env} \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=\xi_i}</math> |
|
|
<math>~=</math> |
<math>~\gamma_e \biggl[ \frac{\gamma_c}{\gamma_e} \biggl( 3 + \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr) - \biggl( 3 + \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr)\biggr]_{\xi=\xi_i}</math> |
|
<math>~\Rightarrow~~~ \frac{d\ln x_\mathrm{env}}{d\ln \xi} \biggr|_{\xi=\xi_i}</math> |
<math>~=</math> |
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x_\mathrm{core}}{d\ln \xi} \biggr)_{\xi=\xi_i} \, .</math> |
In the context of this interface-matching constraint (see their equation 62.1), P. Ledoux & Th. Walraven (1958) state the following: In the static (i.e., unperturbed equilibrium) model … discontinuities in <math>~\rho</math> or in <math>~\gamma</math> might occur at some [radius]. In the first case — that is, a discontinuity only in density, while <math>~\gamma_e = \gamma_c</math> — the interface conditions imply the continuity of <math>~\tfrac{1}{x} \cdot \tfrac{dx}{d\xi}</math> at that [radius]. In the second case — that is, a discontinuity in the adiabatic exponent — the dynamical condition may be written as above. This implies a discontinuity of the first derivative at any discontinuity of <math>~\gamma</math>.
The algorithm that Murphy & Fiedler (1985b) used to "… [integrate] through each zone …" was designed "… with continuity in <math>~x</math> and <math>~dx/d\xi</math> being imposed at the interface …" Given that they set <math>~\gamma_c = \gamma_e = 5/3</math>, their interface matching condition is consistent with the one prescribed by P. Ledoux & Th. Walraven (1958).
Radial Oscillations of (nc, ne) = (5, 1) Models
Foundation
In an accompanying discussion, we derived the so-called,
Adiabatic Wave (or Radial Pulsation) Equation
|
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math> |
whose solution gives eigenfunctions that describe various radial modes of oscillation in spherically symmetric, self-gravitating fluid configurations. Assuming that the underlying equilibrium structure is that of a bipolytrope having <math>~(n_c, n_e) = (5, 1)</math>, it makes sense to adopt the normalizations used when defining the equilibrium structure, namely,
|
<math>~\rho^*</math> |
<math>~\equiv</math> |
<math>~\frac{\rho_0}{\rho_c}</math> |
; |
<math>~r^*</math> |
<math>~\equiv</math> |
<math>~\frac{r_0}{[K_c^{1/2}/(G^{1/2}\rho_c^{2/5})]}</math> |
|
<math>~P^*</math> |
<math>~\equiv</math> |
<math>~\frac{P_0}{K_c\rho_c^{6/5}}</math> |
; |
<math>~M_r^*</math> |
<math>~\equiv</math> |
<math>~\frac{M_r}{[K_c^{3/2}/(G^{3/2}\rho_c^{1/5})]}</math> |
We note as well that,
|
<math>~g_0</math> |
<math>~=</math> |
<math>~\frac{GM(r_0)}{r_0^2}</math> |
|
|
<math>~=</math> |
<math>~ G \biggl[ M_r^* \rho_c^{-1 / 5} \biggl( \frac{K_c}{G}\biggr)^{3 / 2} \biggr] \biggl[ r^* \rho_c^{-2 / 5}\biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr]^{-2} </math> |
|
|
<math>~=</math> |
<math>~ \frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \, . </math> |
Hence, multiplying the LAWE through by <math>~(K_c/G)\rho_c^{-4 / 5}</math> gives,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x </math> |
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d^2x}{dr*^2} + \biggl[\frac{4}{r^*} ~-~ \rho_c^{-2 / 5} \biggl( \frac{K_c}{G} \biggr)^{1 / 2}\biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr*} ~+~ \rho_c^{-4 / 5}\biggl( \frac{K_c}{G} \biggr)\biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 ~+~ (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x </math> |
|
|
<math>~=</math> |
<math>~ \frac{d^2x}{dr*^2} ~+~ \biggl\{\frac{4}{r^*} ~-~ \rho_c^{-2 / 5} \biggl( \frac{K_c}{G} \biggr)^{1 / 2} \frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \biggl[ \frac{\rho_c \rho^*}{P^* K_c \rho_c^{6/5}}\biggr] \biggr\} \frac{dx}{dr*} ~+~ \rho_c^{-4 / 5}\biggl( \frac{K_c}{G} \biggr)\biggl[ \frac{\rho_c \rho^*}{\gamma_\mathrm{g}P^* K_c \rho_c^{6/5}}\biggr] \biggl\{\omega^2 ~+~ (4 - 3\gamma_\mathrm{g}) \frac{G M_r^*}{(r^*)^2} \biggl[ \rho_c^{3 / 5} \biggl( \frac{K_c}{G}\biggr)^{1 / 2} \biggr] \frac{\rho_c^{2 / 5}}{r^*}\biggl( \frac{G}{K_c}\biggr)^{1 / 2} \biggr\} x
</math> |
|
|
<math>~=</math> |
<math>~ \frac{d^2x}{dr*^2} ~+~ \biggl\{\frac{4}{r^*} ~-~ \frac{M_r^*}{(r^*)^2} \biggl[ \frac{\rho^*}{P^* }\biggr] \biggr\} \frac{dx}{dr*} ~+~ \biggl( \frac{1}{G\rho_c} \biggr)\biggl[ \frac{ \rho^*}{\gamma_\mathrm{g}P^* }\biggr] \biggl\{\omega^2 ~+~ (4 - 3\gamma_\mathrm{g}) \frac{G\rho_c M_r^*}{(r^*)^3} \biggr\} x
</math> |
|
|
<math>~=</math> |
<math>~ \frac{d^2x}{dr*^2} + \biggl\{ \frac{4}{r^*} -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)^2}\biggr\} \frac{dx}{dr*} + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{\omega^2}{\gamma_\mathrm{g} G\rho_c} + \biggl(\frac{4}{\gamma_\mathrm{g}} - 3\biggr)\frac{ M_r^*}{(r^*)^3}\biggr\} x </math> |
|
|
<math>~=</math> |
<math>~ \frac{d^2x}{dr*^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{r^*} \frac{dx}{dr*} + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . </math> |
Profile
Now, referencing the derived bipolytropic model profile, we should incorporate the following relations:
Table 1: Radial Profile of Various Physical Variables
|
Variable |
Throughout the Core |
Throughout the Envelope† |
Plotted Profiles |
||
|
<math>~\xi_i = 0.5</math> |
<math>~\xi_i = 1.0</math> |
<math>~\xi_i = 3.0</math> |
|||
|
<math>~r^*</math> |
<math>\biggl( \frac{3}{2\pi} \biggr)^{1/2} \xi</math> |
<math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta</math> |
|
||
|
<math>~\rho^*</math> |
<math>\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-5/2}</math> |
<math>\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{5}_i \phi(\eta)</math> |
|||
|
<math>~P^*</math> |
<math>\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3}</math> |
<math>\theta^{6}_i [\phi(\eta)]^{2}</math> |
|||
|
<math>~M_r^*</math> |
<math>\biggl( \frac{2\cdot 3}{\pi } \biggr)^{1/2} \biggl[ \xi^3 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} \biggr]</math> |
<math>\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \theta^{-1}_i \biggl( \frac{2}{\pi} \biggr)^{1/2} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr)</math> |
|||
|
†In order to obtain the various envelope profiles, it is necessary to evaluate <math>\phi(\eta)</math> and its first derivative using the information presented in Step 6, above. |
|||||
Therefore, throughout the core we have,
|
<math>~\frac{\rho^*}{P^*}</math> |
<math>~=</math> |
<math>~\biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{1 / 2} \, ;</math> |
|
<math>~\frac{M_r^*}{r^*}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{2\cdot 3}{\pi } \biggr)^{1/2} \biggl[ \xi^3 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} \biggr]\biggl( \frac{2\pi}{3}\biggr)^{1 / 2} \frac{1}{\xi} = 2 \xi^2 \biggl( 1 + \frac{1}{3}\xi^2 \biggr)^{-3/2} \, ; </math> |
and, throughout the envelope we have,
|
<math>~\frac{\rho^*}{P^*}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{-1}_i \phi(\eta)^{-1} \, ; </math> |
|
<math>~\frac{M_r^*}{r^*}</math> |
<math>~=</math> |
<math>~ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \theta^{-1}_i \biggl( \frac{2}{\pi} \biggr)^{1/2} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) \biggl[ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \theta^{-2}_i (2\pi)^{-1/2}\eta \biggr]^{-1} = 2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \frac{\theta_i}{\eta} \biggl(-\eta^2 \frac{d\phi}{d\eta} \biggr) \, . </math> |
|
NOTE on 15 May 2019: Prior to this date the last RHS expression had an incorrect exponent on <math>~\eta</math>. It previously (incorrectly) read,
|
Numerical Integration
General Approach
Here, we begin by recognizing that the 2nd-order ODE that must be integrated to obtain the desired eigenvectors has the generic form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ x + \frac{\mathcal{H}}{r^*} x' + \mathcal{K}x \, , </math> |
where,
|
<math>~x'</math> |
<math>~=</math> |
<math>~\frac{dx}{dr^*}</math> |
and |
<math>~x</math> |
<math>~=</math> |
<math>~\frac{d^2x}{d(r^*)^2} \, .</math> |
Adopting the same approach as before when we integrated the LAWE for pressure-truncated polytropes, we will enlist the finite-difference approximations,
|
<math>~x'</math> |
<math>~\approx</math> |
<math>~ \frac{x_+ - x_-}{2\delta r^*} </math> |
and |
<math>~x</math> |
<math>~\approx</math> |
<math>~ \frac{x_+ -2x_j + x_-}{(\delta r^*)^2} \, . </math> |
The finite-difference representation of the LAWE is, therefore,
|
<math>~\frac{x_+ -2x_j + x_-}{(\delta r^*)^2}</math> |
<math>~=</math> |
<math>~ -~ \frac{\mathcal{H}}{r^*} \biggl[ \frac{x_+ - x_-}{2\delta r^*} \biggr] ~-~ \mathcal{K}x_j </math> |
|
<math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> |
<math>~=</math> |
<math>~ -~ \frac{\delta r^*}{2r^*} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta r^*)^2\mathcal{K}x_j </math> |
|
<math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]</math> |
<math>~=</math> |
<math>~ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{j-1} \, . </math> |
In what follows we will also find it useful to rewrite <math>~\mathcal{K}</math> in the form,
<math>~\mathcal{K} ~\rightarrow ~\biggl(\frac{\sigma_c^2}{\gamma_\mathrm{g}}\biggr) \mathcal{K}_1 - \alpha_\mathrm{g} \mathcal{K}_2 \, .</math>
The relevant coefficient expressions for all regions of the configuration are,
|
<math>~\mathcal{H}</math> |
<math>~\equiv</math> |
<math>~ \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\} </math> |
, |
<math>~\mathcal{K}_1</math> |
<math>~\equiv</math> |
<math>~ \frac{2\pi }{3}\biggl(\frac{\rho^*}{ P^* } \biggr) </math> |
and |
<math>~\mathcal{K}_2</math> |
<math>~\equiv</math> |
<math>~ \biggl(\frac{\rho^*}{ P^* } \biggr)\frac{M_r^*}{(r^*)^3} \, . </math> |
Special Handling at the Center
In order to kick-start the integration, we set the displacement function value to <math>~x_1 = 1</math> at the center of the configuration <math>~(\xi_1 = 0)</math>, then draw on the derived power-series expression to determine the value of the displacement function at the first radial grid line, <math>~\xi_2 = \delta\xi</math>, away from the center. Specifically, we set,
|
<math>~ x_2 </math> |
<math>~=</math> |
<math>~ x_1 \biggl[ 1 - \frac{(n+1) \mathfrak{F} (\delta\xi)^2}{60} \biggr]_{n=5} = x_1 \biggl[ 1 - \frac{\mathfrak{F} (\delta\xi)^2}{10} \biggr] \, .</math> |
Special Handling at the Interface
Integrating outward from the center, the general approach will work up through the determination of <math>~x_{j+1}</math> when "j+1" refers to the interface location. In order to properly transition from the core to the envelope, we need to determine the value of the slope at this interface location. Let's do this by setting j = i, then projecting forward to what <math>~x_+</math> would be — that is, to what the amplitude just beyond the interface would be — if the core were to be extended one more zone. Then, the slope at the interface (as viewed from the perspective of the core) will be,
|
<math>~x'_i\biggr|_\mathrm{core}</math> |
<math>~\approx</math> |
<math>~ \frac{1}{2\delta r^*} \biggl\{ x_+ - x_{i-1} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ -\frac{x_{i-1}}{2\delta r^*} + \frac{1}{2\delta r^*} \biggl\{ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{i-1} \biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2\delta r^*} \biggl\{ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr]x_{i-1} ~-~\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]x_{i-1} \biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{2\delta r^*} \biggl\{ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~2x_{i-1} \biggr\}\biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]^{-1} </math> |
Conversely, as viewed from the envelope, if we assume that we know <math>~x_i</math> and <math>~x'_i</math>, we can determine the amplitude, <math>~x_{i+1}</math>, at the first zone beyond the interface as follows:
|
<math>~x_-</math> |
<math>~\approx</math> |
<math>~ x_{i+1} - 2\delta r^*\cdot x'_i\biggr|_\mathrm{env} </math> |
|
<math>~\Rightarrow ~~~ x_{i+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr]</math> |
<math>~=</math> |
<math>~ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~-~\biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] \biggl[ x_{i+1} - 2\delta r^*\cdot x'_i\biggr|_\mathrm{env} \biggr] </math> |
|
<math>~\Rightarrow ~~~ x_{i+1} \biggl[1 + \biggl( \frac{\delta r^*}{2r^*}\biggr) \mathcal{H} \biggr] ~+~ \biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] x_{i+1} </math> |
<math>~=</math> |
<math>~ \biggl[ 2 - (\delta r^*)^2\mathcal{K}\biggr] x_i ~+~ \biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] 2\delta r^*\cdot x'_i\biggr|_\mathrm{env} </math> |
|
<math>~\Rightarrow ~~~ x_{i+1} </math> |
<math>~=</math> |
<math>~ \biggl[ 1 - \tfrac{1}{2}(\delta r^*)^2\mathcal{K}\biggr] x_i ~+~ \biggl[ 1 - \biggl( \frac{\delta r^*}{2r^*} \biggr) \mathcal{H} \biggr] \delta r^*\cdot x'_i\biggr|_\mathrm{env} </math> |
Splitting Analysis Into Separate Core and Envelope Components
Core:
Given that, <math>~\sqrt{2\pi/3}~r^* = \xi</math>, lets multiply the LAWE through by <math>~3/(2\pi)</math>. This gives,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d^2x}{d\xi^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{\xi} \cdot \frac{dx}{d\xi} + \frac{3}{2\pi}\biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . </math> |
Specifically for the core, therefore, the finite-difference representation of the LAWE is,
|
<math>~\frac{x_+ -2x_j + x_-}{(\delta \xi)^2}</math> |
<math>~=</math> |
<math>~ -~ \frac{\mathcal{H}}{\xi} \biggl[ \frac{x_+ - x_-}{2\delta \xi} \biggr] ~-~ \biggl[ \frac{3\mathcal{K}}{2\pi} \biggr]x_j </math> |
|
<math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> |
<math>~=</math> |
<math>~ -~ \frac{\delta \xi}{2\xi} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta \xi)^2 \biggl[ \frac{3\mathcal{K}}{2\pi} \biggr] x_j </math> |
|
<math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta \xi}{2\xi}\biggr) \mathcal{H} \biggr]</math> |
<math>~=</math> |
<math>~ \biggl[ 2 - (\delta \xi)^2\biggl( \frac{3\mathcal{K}}{2\pi} \biggr) \biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta \xi}{2\xi} \biggr) \mathcal{H} \biggr]x_{j-1} \, . </math> |
This also means that, as viewed from the perspective of the core, the slope at the interface is
|
<math>~\biggl[ \frac{dx}{d\xi}\biggr]_\mathrm{interface}</math> |
<math>~=</math> |
<math>~ \frac{1}{2\delta \xi} \biggl\{ \biggl[ 2 - (\delta \xi)^2 \biggl( \frac{3\mathcal{K}}{2\pi} \biggr)\biggr] x_i ~-~2x_{i-1} \biggr\}\biggl[1 + \biggl( \frac{\delta \xi}{2\xi}\biggr) \mathcal{H} \biggr]^{-1} \, . </math> |
Envelope:
Given that,
<math>~\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta_i^2 (2\pi)^{1 / 2}~r^* = \eta \, ,</math>
let's multiply the LAWE through by <math>~(2\pi)^{-1} \theta_i^{-4}( \mu_e/\mu_c)^{-2} </math>. This gives,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d^2x}{d\eta^2} + \biggl\{ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr\}\frac{1}{\eta} \cdot \frac{dx}{d\eta} + \frac{1}{2\pi \theta_i^4} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl\{ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr\} x \, . </math> |
Specifically for the envelope, therefore, the finite-difference representation of the LAWE is,
|
<math>~\frac{x_+ -2x_j + x_-}{(\delta \eta)^2}</math> |
<math>~=</math> |
<math>~ -~ \frac{\mathcal{H}}{\eta} \biggl[ \frac{x_+ - x_-}{2\delta \eta} \biggr] ~-~ \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2}\biggl[ \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr]x_j </math> |
|
<math>~\Rightarrow ~~~ x_+ -2x_j + x_-</math> |
<math>~=</math> |
<math>~ -~ \frac{\delta \eta}{2\eta} \biggl[ x_+ - x_- \biggr]\mathcal{H} ~-~ (\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2}\biggl[ \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr] x_j </math> |
|
<math>~\Rightarrow ~~~ x_{j+1} \biggl[1 + \biggl( \frac{\delta \eta}{2\eta}\biggr) \mathcal{H} \biggr]</math> |
<math>~=</math> |
<math>~ \biggl[ 2 - (\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl( \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr) \biggr] x_j ~-~\biggl[ 1 - \biggl( \frac{\delta \eta}{2\eta} \biggr) \mathcal{H} \biggr]x_{j-1} \, . </math> |
This also means that, once we know the slope at the interface (see immediately below), the amplitude at the first zone outside of the interface will be given by the expression,
|
<math>~x_{i+1} </math> |
<math>~=</math> |
<math>~ \biggl[ 1 - \tfrac{1}{2}(\delta \eta)^2 \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-2} \biggl( \frac{\mathcal{K}}{2\pi \theta_i^4} \biggr)\biggr] x_i ~+~ \biggl[ 1 - \biggl( \frac{\delta \eta}{2\eta} \biggr) \mathcal{H} \biggr] \delta \eta \cdot \biggl[ \frac{dx}{d\eta} \biggr]_\mathrm{interface} \, . </math> |
Interface
If we consider only cases where <math>~\gamma_e = \gamma_c</math>, then at the interface we expect,
|
<math>~\frac{d\ln x}{d\ln r^*}</math> |
<math>~=</math> |
<math>~\frac{d\ln x}{d\ln \xi} = \frac{d\ln x}{d\ln \eta}</math> |
|
<math>~\Rightarrow ~~~ r^*\frac{dx}{d r^*}</math> |
<math>~=</math> |
<math>~\xi \frac{dx}{d \xi} = \eta \frac{d x}{d \eta}</math> |
|
<math>~\Rightarrow ~~~ \frac{dx}{dr^*}</math> |
<math>~=</math> |
<math>~\biggl(\frac{2\pi}{3} \biggr)^{1 / 2}\frac{dx}{d\xi} = \biggl(\frac{\mu_e}{\mu_c}\biggr) \theta_i^2 (2\pi)^{1 / 2} \frac{dx}{d\eta} \, .</math> |
Switching at the interface from <math>~\xi</math> to <math>~\eta</math> therefore means that,
|
<math>~ \biggl[ \frac{dx}{d\eta}\biggr]_\mathrm{interface}</math> |
<math>~=</math> |
<math>~\frac{1}{\sqrt{3}} \biggl(\frac{\mu_e}{\mu_c}\biggr)^{-1} \theta_i^{-2} \biggl[ \frac{dx}{d\xi}\biggr]_\mathrm{interface} \, .</math> |
If, however, we want to consider values for the adiabatic index that are different in the two regions, we have to follow the above-outlined guidelines, that is,
|
<math>~ \frac{d\ln x}{d\ln \eta} \biggr|_i</math> |
<math>~=</math> |
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl( \frac{d\ln x}{d\ln \xi} \biggr)_i </math> |
|
<math>~ \Rightarrow ~~~ \biggl[ \frac{dx}{d\eta} \biggr]_i</math> |
<math>~=</math> |
<math>~\frac{x_i}{\eta_i} \biggl\{ 3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \cdot \frac{\xi_i}{x_i}\biggl[ \frac{dx}{d\xi} \biggr]_i \biggr\}</math> |
|
|
<math>~=</math> |
<math>~ \frac{3x_i}{\eta_i} \biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \cdot \frac{1}{\sqrt{3}} \biggl(\frac{\mu_e}{\mu_c}\biggr)^{-1} \theta_i^{-2} \biggl[ \frac{dx}{d\xi} \biggr]_i \, . </math> |
Eigenvectors for Marginally Unstable Models with (γc, γe) = (6/5, 2)
We now have the tools in hand to identify the eigenvectors — that is, various radial eigenfunctions and the corresponding eigenfrequency for each — associated with various modes of oscillation in <math>~(n_c, n_e) = (5,1)</math> bipolytropes. Which models should we examine?
In our accompanying review of the bipolytrope stability analysis presented by Murphy & Fiedler (1983b), our primary objective was to show that we were able to match their results quantitatively. We therefore set <math>~\mu_e/\mu_c</math> = 1 — the only <math>~\mu</math>-ratio that they considered — and picked values of the core-envelope interface radius, <math>~\xi_i</math>, that were listed among their set of chosen models. For a fixed value of <math>~\xi_i</math>, we integrated the relevant LAWE from the center toward the surface for many different eigenfrequency <math>~(\sigma_c^2)</math> guesses until an eigenfunction was found whose behavior at the surface matched with high precision the physically justified surface boundary condition.
With the above virial stability analysis in mind (see especially Figure 3), here we have chosen to focus on models that reside along six of the equilibrium sequences that have already been analytically identified, above — specifically, the sequences for which <math>~\mu_e/\mu_c</math> = 1, ½, 0.345, ⅓, 0.309, and ¼ — and to examine oscillation modes under the assumption that,
<math>~\gamma_c = \frac{(n_c+1)}{n_c} = \frac{6}{5} \, ,</math> and <math>~\gamma_e = \frac{(n_e+1)}{n_e} =2 \, .</math>
Fundamental Modes
We decided to examine, first, whether any model along each sequence marks a transition from dynamically stable to dynamically unstable configurations. We accomplished this by setting <math>~\sigma_c^2</math> = 0, then integrating the relevant LAWE from the center toward the surface for many different guesses of the core-envelope interface radius until an eigenfunction with no radial nodes — i.e., an eigenfunction associated with the fundamental mode of radial oscillation — was found whose behavior at the surface matched with high precision the physically desired surface boundary condition. We were successful in this endeavor. A marginally unstable model was identified on each of the six separate equilibrium sequences.
Equilibrium Properties of Marginally Unstable Models
Table 2 summarizes some of the equilibrium properties of these six models. For example, the second column of the table gives the value of the core-envelope interface radius, <math>~\xi_i</math>, associated with each marginally unstable model. The table also lists: the value of the model's dimensionless radius, <math>~R^*_\mathrm{surf}</math>, the key structural parameters, <math>~q</math> & <math>~\nu</math>, and the central-to-mean density associated with each model; and in each case the dimensionless thermal energy <math>~(\mathfrak{s})</math> and dimensionless gravitational potential energy <math>~(\mathfrak{w})</math> associated, separately, with the core and the envelope. Note that, once the pair of parameters, <math>~(\mu_e/\mu_c, \xi_i)</math>, has been specified, we can legitimately assign high-precision values to all of the other model parameters because they are analytically prescribed.
|
Table 2: Properties of Marginally Unstable Bipolytropes Having |
|||||||||
|---|---|---|---|---|---|---|---|---|---|
| <math>~\frac{\mu_e}{\mu_c}</math> | <math>~\xi_i</math> | <math>~R^*_\mathrm{surf}</math> | <math>~q \equiv \frac{r_\mathrm{core}}{R_\mathrm{surf}}</math> | <math>~\nu \equiv \frac{M_\mathrm{core}}{M_\mathrm{tot}}</math> | <math>~\frac{\rho_c}{\bar\rho}</math> | <math>~\mathfrak{s}_\mathrm{core}</math> | <math>~\mathfrak{w}_\mathrm{core}</math> | <math>~\mathfrak{s}_\mathrm{env}</math> | <math>~\mathfrak{w}_\mathrm{env}</math> |
| 1 | 1.6686460157 | 2.139737 | 0.53885819 | 0.497747626 | 8.51704656 | 3.021916335 | -3.356583022 | 1.47780476 | -5.642859167 |
| <math>~\tfrac{1}{2}</math> | 2.27925811317 | 5.146499 | 0.306021732 | 0.401776274 | 63.29514949 | 4.241287117 | -6.074241035 | 4.284931508 | -10.97819621 |
| 0.345 | 2.560146865247 | 9.554041 | 0.185160563 | 0.234302525 | 209.7739052 | 4.639705843 | -7.125754184 | 11.72861751 | -25.61089252 |
| <math>~\tfrac{1}{3}</math> | 2.582007485476 | 10.120558 | 0.176288391 | 0.218241608 | 230.4125398 | 4.667042505 | -7.200966267 | 13.15887139 | -28.45086152 |
| 0.309 | 2.6274239687695 | 11.464303 | 0.158362807 | 0.184796947 | 279.0788798 | 4.722277318 | -7.354156963 | 17.1374434 | -36.36528446 |
| <math>~\tfrac{1}{4}</math> | 2.7357711469398 | 15.895632 | 0.118924863 | 0.11071211 | 430.0444648 | 4.84592201 | -7.70305421 | 37.84289623 | -77.67458196 |
As was expected from our above discussion of virial equilibrium conditions, we found that to high precision for each of these equilibrium models,
|
<math> (\mathfrak{w}_\mathrm{core} ~+~\mathfrak{w}_\mathrm{env}) ~+~2(\mathfrak{s}_\mathrm{core} ~+~\mathfrak{s}_\mathrm{env}) </math> |
<math>~=</math> |
<math>~0 \, .</math> |
However, contrary to expectations, in no case did we find that <math>~\mathfrak{s}_\mathrm{core}/\mathfrak{s}_\mathrm{env} = 5</math>. That is to say, we found that none of the models lies on the (red-dashed) curve in the <math>~q-\nu</math> parameter space that separates stable from unstable models as defined by our above free-energy-based stability analysis. The left-hand panel of Figure 4 shows this (red-dashed) demarcation curve; for all intents and purposes, it is a reproduction of the right-hand panel of Figure 3, above — turning-point markers have been removed to minimize clutter, the equilibrium sequences have been labeled, and the horizontal axis has been extended to unity in order to include a longer portion of the <math>~\mu_e/\mu_c = 1</math> sequence. The orange triangular markers that appear in the right-hand panel of Figure 4 pinpoint where each of the Table 2 "marginally unstable" models resides in this <math>~q-\nu</math> plane. Clearly, all six of the orange triangles lie well off of — and to the stable side of — the red-dashed demarcation curve. This discrepancy, which has resulted from our use of two separate approaches to stability analysis, will be discussed further and gratifyingly resolved, below.
| Figure 4 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
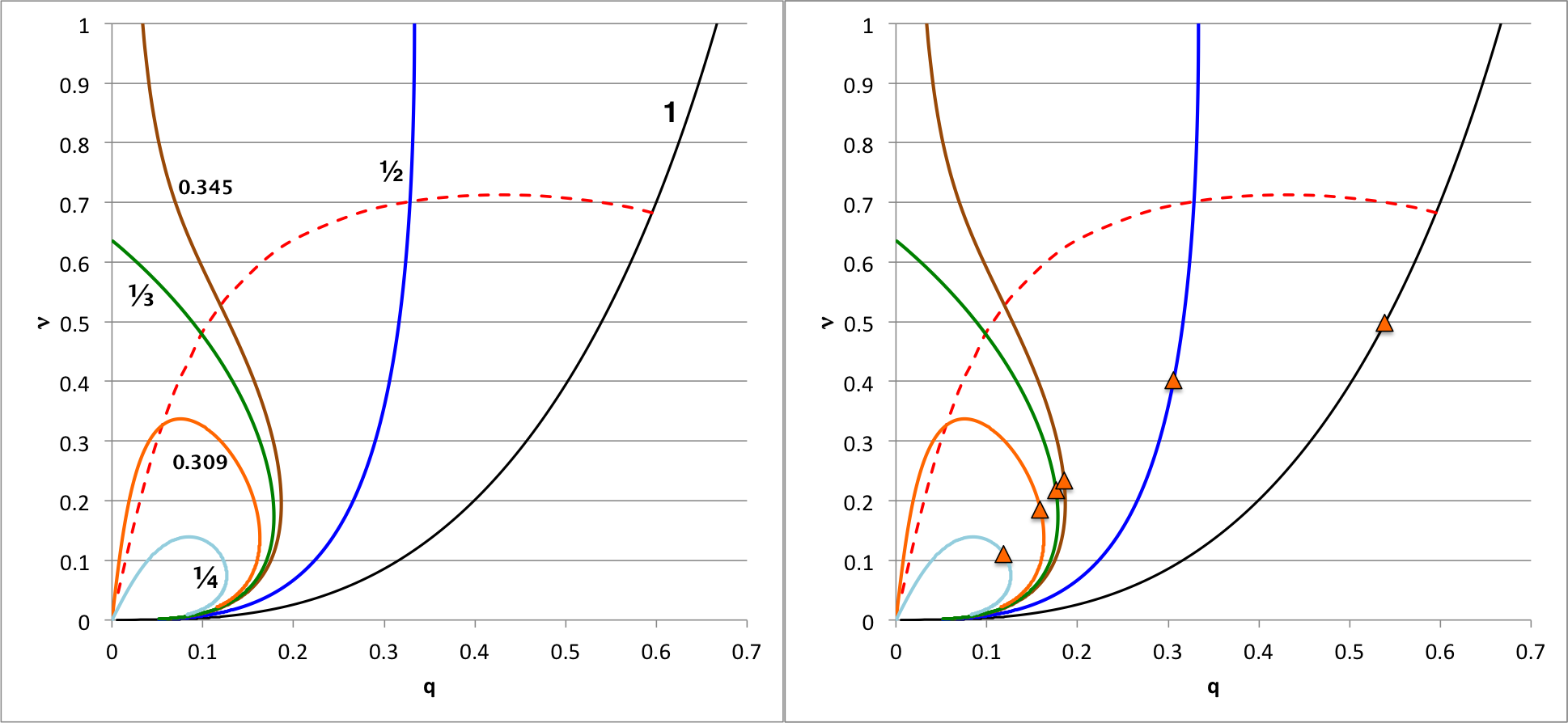 |
|||||||||
Eigenfunction Details
Here we examine some of the properties of the fundamental-mode eigenfunctions that we have found are associated with marginally unstable, <math>~(n_c, n_e) = (5,1)</math> bipolytropes.
| Figure 5 |
|---|
Consider the model on the <math>~\mu_e/\mu_c = 1</math> sequence for which <math>~\sigma_c^2=0~</math>; key properties of this specific equilibrium model are enumerated in the first row of numbers provided in Table 2, above. Figure 5 shows how our numerically derived, fundamental-mode eigenfunction, <math>~x = \delta r/r_0</math>, varies with the fractional radius over the entire range, <math>~0 \le r/R \le 1</math>. By prescription, the eigenfunction has a value of unity and a slope of zero at the center <math>~(r/R = 0)</math>. Integrating the LAWE outward from the center, through the model's core (blue curve segment), <math>~x</math> drops smoothly to the value <math>~x_i = 0.81437</math> at the interface <math>~(\xi_i = 1.6686460157 ~\Rightarrow~ q = r_\mathrm{core}/R_\mathrm{surf} = 0.53885819)</math>. Our numerical integration of the LAWE showed that, at the interface, the logarithmic slope of the core (blue) segment of the eigenfunction is,
|
<math>~ \biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i \biggr\}_\mathrm{core} = \biggl\{ \frac{d\ln x}{d\ln \xi}\biggr|_i \biggr\}_\mathrm{core} </math> |
<math>~=</math> |
<math>~- 0.455872 \, .</math> |
Next, following the above discussion of matching conditions at the interface, we determined that, from the perspective of the envelope, the slope of the eigenfunction at the interface must therefore be,
|
<math>~ \biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i \biggr\}_\mathrm{env} = \biggl\{ \frac{d\ln x}{d\ln \eta}\biggr|_i \biggr\}_\mathrm{env} </math> |
<math>~=</math> |
<math>~3\biggl(\frac{\gamma_c}{\gamma_e} -1\biggr) + \frac{\gamma_c}{\gamma_e} \biggl\{ \frac{d\ln x}{d\ln \xi}\biggr|_i \biggr\}_\mathrm{core} = -1.47352 \, .</math> |
Adopting this "env" slope along with the amplitude, <math>~x_i = 0.81437</math>, as the appropriate interface boundary conditions, we integrated the LAWE from the interface to the surface, obtaining the green-colored segment of the eigenfunction that is shown in Figure 5. The amplitude continued to steadily decrease, reaching a value of <math>~x_s = 0.38203</math>, at the model's surface <math>~(r/R = 1)</math>. At the surface, this envelope (green) segment of the eigenfunction exhibits a logarithmic slope that matches to eight significant digits the value that is expected from astrophysical arguments for this marginally unstable <math>~(\sigma_c^2=0)</math> model, namely,
<math>~ \frac{d\ln x}{d\ln \eta}\biggr|_s = \biggl[ \biggl( \frac{\rho_c}{\bar\rho} \biggr)\frac{\cancelto{0}{\sigma_c^2}}{2\gamma_e} - \biggl(3 - \frac{4}{\gamma_e}\biggr)\biggr] = -1 \, . </math>
|
Key Reminder: We were able to find an eigenfunction whose surface boundary condition matched the desired value — in this particular case, a logarithmic slope of negative one — to this high level of precision only by iterating many times and, at each step, fine-tuning our choice of the equilibrium model's radial interface location, <math>~\xi_i</math> before performing a numerical integration of the LAWE. |
The discontinuous jump that occurs in the slope of the eigenfunction at the interface results from our assumption that the effective adiabatic index of material in the core <math>~(\gamma_c = 6/5)</math> is different from the effective adiabatic index of the envelope material <math>~(\gamma_e = 2)</math>. In an effort to emphasize and more clearly illustrate the behavior of this fundamental-mode eigenfunction as it crosses the core/envelope interface, we have added a pair of dashed line segments to the Figure 5 plot. The red-dashed line segment touches, and is tangent to, the blue segment of the eigenfunction at the location of the core/envelope interface; it has a slope,
|
<math>~ \frac{dx}{d(r/R)}\biggr|_i = \frac{x_i}{(r_i/R)}\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i\biggr\}_\mathrm{core} </math> |
<math>~=</math> |
<math>~- 0.455872 \biggl(\frac{ 0.81437 }{ 0.53885819 }\biggr) = - 0.68895\, .</math> |
On the other hand, the purple-dashed line segment touches, and is tangent to, the green segment of the eigenfunction at the location of the core/envelope interface; it has a slope,
|
<math>~ \frac{dx}{d(r/R)}\biggr|_i = \frac{x_i}{(r_i/R)}\biggl\{ \frac{d\ln x}{d\ln r}\biggr|_i\biggr\}_\mathrm{env} </math> |
<math>~=</math> |
<math>~- 1.47352 \biggl(\frac{ 0.81437 }{ 0.53885819 }\biggr) = - 2.22691\, .</math> |
For comparison purposes, the eigenfunction shown in Figure 5 has been presented again in Figure 6, along with several other of our numerically derived eigenfunctions, but in Figure 6 the plotted amplitude has been renormalized to give a surface value — rather than a central value — of unity.
In Figure 6 we show the behavior of the fundamental-mode eigenfunction for each of the marginally unstable models identified in Table 2. In the top figure panel, each curve shows — on a linear-linear plot — how the amplitude varies with radius; in the bottom figure panel, the amplitude is plotted on a logarithmic scale. On each curve, the black plus sign marks the radial location of the core-envelope interface; in the bottom panel, these markers are accompanied by the values of <math>~\xi_i</math> that are associated with each corresponding model (see also the second column of Table 2). Each eigenfunction has been normalized such that the surface amplitude is unity. In the top panel, the value of the central amplitude of the eigenfunction that results from this normalization is recorded near the point where each eigenfunction touches the vertical axis. (In each case, the value provided on the plot is simply the inverse of the value of <math>~x_s</math> given in Table 3, below.)
|
Figure 6: Eigenfunctions Associated with the Fundamental-Mode of Radial Oscillation |
|---|
Notice that, especially as they approach the surface, the "envelope" segments of these six marginally unstable eigenfunction appear to merge into the same curve, irrespective of their value of the ratio of mean molecular weights. Note as well that the discontinuous jump that occurs in the slope of each eigenfunction at the radial location of the core/envelope interface — resulting from our choice to adopt a different adiabatic index, <math>~\gamma_g</math>, in the core from the one in the envelope — becomes less and less noticeable for smaller and smaller values of the ratio of mean molecular weights.
Is There an Analytic Expression for the Eigenfunction?
After noticing that, in Figure 6, the envelope segments of all of the marginally unstable eigenfunctions merge into the same curve, we began to wonder whether a single expression — and, even better, an analytically defined expression — would perfectly describe the eigenfunction. We had reason to believe that this might actually be possible because, in pressure-truncated polytropic configurations, we have derived analytic expressions for the marginally unstable, fundamental-mode eigenfunctions of both <math>~n = 5</math> and <math>~n=1</math> systems.
Very quickly, we convinced ourselves that a parabolic function does indeed perfectly match the "core" segment of each displayed eigenfunction. Specifically, throughout the core <math>~(0 \le \xi \le \xi_i)</math>,
|
<math>~x_P\biggr|_\mathrm{core}</math> |
<math>~\equiv</math> |
<math>~1 - \frac{\xi^2}{15}</math> |
|
<math>~\Rightarrow~~~\frac{dx_P}{d\xi}\biggr|_\mathrm{core}</math> |
<math>~\equiv</math> |
<math>~- \frac{2\xi}{15} </math> |
|
<math>~\Rightarrow~~~\frac{d\ln x_P}{d\ln \xi}\biggr|_\mathrm{core}</math> |
<math>~\equiv</math> |
<math>~- \frac{2\xi^2}{15} \biggl[ \frac{(15 - \xi^2)}{15} \biggr]^{-1} = - \frac{2\xi^2}{(15 - \xi^2)} \, .</math> |
The envelope segment posed a much greater challenge. In the context of our discussion of Radial Oscillations of n = 1 Polytropic Spheres, and in an accompanying Ramblings Appendix chapter we have detailed some trial derivations that are mostly blind alleyways. Twice — once in January, 2019 and again (independently) in April 2019 — we have analytically demonstrated that the following appears to work for the envelope:
Given that the Structural Properties of the envelope are described by the Lane-Emden function,
|
<math>~\phi</math> |
<math>~=</math> |
<math>~a_0 \biggl[ \frac{\sin(\eta - b_0)}{\eta} \biggr] </math> |
|
<math>~\Rightarrow~~~ Q \equiv - \frac{d\ln \phi}{d\ln\eta}</math> |
<math>~=</math> |
<math>~\biggl[1 - \eta \cot(\eta - b_0) \biggr] \, ,</math> |
the relevant LAWE is satisfied by the fractional displacement function,
|
<math>~x_P</math> |
<math>~=</math> |
<math>~\frac{3c_0 Q}{\eta^2} \, ,</math> |
where, <math>~c_0</math> is an arbitrary scale factor.
|
Note that,
|
But, as far as we have been able to determine (as of 16 April 2019), this analytic displacement function does not match the displacement function that has been generated through numerical integration of the LAWE (see the light-green segment of the eigenfunction displayed above in Figure 5). It remains unclear whether (a) the numerical integration is at fault, (b) we are imposing an incorrect slope at the core-envelope interface, or ( c) we are misinterpreting how to compare the two separately derived (one, numerical, and the other, analytic) envelope eigenfunctions.
Other Modes
|
Our Determinations for Marginally Unstable Model Having <math>~\mu_e/\mu_c = 1</math> |
||||||||||||||
| Mode | <math>~\sigma_c^2</math> | <math>~\Omega^2 \equiv \frac{\sigma_c^2}{2} \biggl( \frac{\rho_c}{\bar\rho}\biggr)</math> | <math>~x_i</math> | <math>~\frac{d\ln x}{d\ln r^*}\biggr|_i</math> | <math>~x_\mathrm{surf}</math> | <math>~\frac{d\ln x}{d\ln r^*}\biggr|_\mathrm{surf}</math> | <math>~\frac{r}{R}\biggr|_1</math> | <math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_1</math> | <math>~\frac{r}{R}\biggr|_2</math> | <math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_2</math> | <math>~\frac{r}{R}\biggr|_3</math> | <math>~1 - \frac{M_r}{M_\mathrm{tot}}\biggr|_3</math> | ||
| core | env | expected | measured | |||||||||||
| 1 (Fundamental) |
0.00 | 0.00 | +0.81437470 | -0.455872 | -1.473523 | +0.3820 | -1 | -0.999999992 | n/a | n/a | n/a | n/a | n/a | n/a |
| 2 | 2.51513333 | 10.7107538 | 0.20482050 | -7.09124 | -5.4547441 | - 0.9962 | 4.355376917 | 4.35537692 | 0.64133 | 0.3502 | n/a | n/a | n/a | n/a |
| 3 | 5.72371888 | 24.3745901 | -0.14269277 | +8.046019 | +3.627611 | +0.9308 | 11.18729505 | 11.18729506 | 0.4837 | 0.5864 | 0.842 | 0.0854 | n/a | n/a |
| 4 | 10.3458476 | 44.0622916 | -0.20845197 | -0.6949966 | -1.61699793 | -1.1443 | 21.03114578 | 21.03114577 | 0.3939 | 0.7154 | 0.6902 | 0.2777 | 0.9115 | 0.0284 |
Reconciliation
| Figure 7: Conflicting Instability Regions | |||||||||
|---|---|---|---|---|---|---|---|---|---|
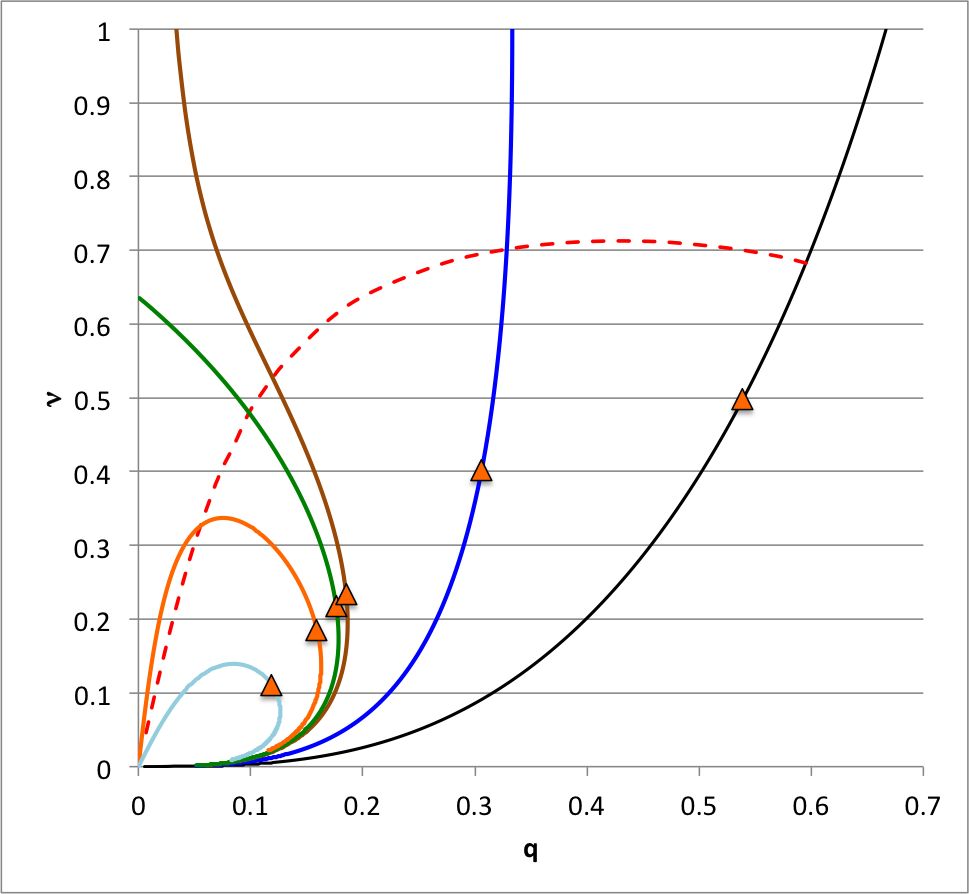 |
|||||||||
Figure 7, shown here on the right, is identical to the right-hand panel of Figure 4, as displayed above. From a standard global, free-energy analysis — such as the one summarized above — we have determined that the red-dashed curve shown in the right panel of Figure 6 divides the <math>~q-\nu</math> plane into dynamically stable (below and to the right) and unstable (above and to the left) regions.
Variational Principle
Setup
Let's follow the guidelines of the variational principle. Instead of starting with the form of the LAWE given above, namely,
Adiabatic Wave (or Radial Pulsation) Equation
|
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math> |
we will start with a form that is more amenable to the variational principle, namely,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d}{dr_0}\biggl[ r_0^4 \gamma P_0 ~\frac{dx}{dr_0} \biggr] +\biggl[ \omega^2 \rho_0 r_0^4 + (3\gamma - 4) r_0^3 \frac{dP_0}{dr_0} \biggr] x \, . </math> |
|
ASIDE: Let's show that these two expressions are equivalent. Remembering that,
the second expression becomes,
Hence, we must multiply the first expression through by <math>~r_0^4 \gamma P_0</math> in order to obtain the second expression. |
From above, we realize that multiplying the second expression through by <math>~(K_c/G)\rho_c^{-4 / 5}</math> gives,
|
<math>~0</math> |
<math>~=</math> |
<math>~ r_0^4\gamma P_0 \biggl\{ \frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ (r^*)^4 [K_c^{1 / 2}/(G^{1 / 2} \rho_c^{2/5}) ]^4 \gamma P^* [K_c\rho_c^{6/5}] \biggl\{ \frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{K_c^{3}}{ G^{2} \rho_c^{2/5}} \biggr] (r^*)^4 \gamma P^* \biggl\{ \frac{d^2x}{dr*^2} + \biggl[ 4 -\biggl(\frac{\rho^*}{P^*}\biggr)\frac{ M_r^*}{(r^*)}\biggr] \frac{1}{r^*} \frac{dx}{dr*} + \biggl(\frac{\rho^*}{ P^* } \biggr)\biggl[ \frac{2\pi \sigma_c^2}{3\gamma_\mathrm{g}} ~-~\frac{\alpha_\mathrm{g} M_r^*}{(r^*)^3}\biggr] x \biggr\} \, . </math> |
That is, multiplying the second expression through by, <math>~(K_c/G)\rho_c^{-4 / 5} \cdot G^2 \rho_c^{2 / 5}/K_c^3 = G/(K_c^2 \rho_c^{2 / 5})</math> , should give a desirable, totally dimensionless version of the LAWE. Remembering that,
|
<math>~\rho^*</math> |
<math>~\equiv</math> |
<math>~\frac{\rho_0}{\rho_c}</math> |
; |
<math>~r^*</math> |
<math>~\equiv</math> |
<math>~\frac{r_0}{[K_c^{1/2}/(G^{1/2}\rho_c^{2/5})]}</math> |
|
<math>~P^*</math> |
<math>~\equiv</math> |
<math>~\frac{P_0}{K_c\rho_c^{6/5}}</math> |
; |
<math>~M_r^*</math> |
<math>~\equiv</math> |
<math>~\frac{M_r}{[K_c^{3/2}/(G^{3/2}\rho_c^{1/5})]}</math> |
|
<math>~\frac{dP^*}{dr^*}</math> |
<math>~=</math> |
<math>~-\frac{M^*_r \rho^*}{(r^*)^2} </math> |
; |
<math>~E_\mathrm{norm}</math> |
<math>~=</math> |
<math>~\biggl[ \frac{K_c^5}{G^3}\biggr]^{1 / 2}</math> |
let's try it.
|
<math>~0</math> |
<math>~=</math> |
<math>~G K_c^{-2} \rho_c^{-2 / 5} \biggl\{ \frac{d}{dr_0}\biggl[ r_0^4 \gamma P_0 ~\frac{dx}{dr_0} \biggr] +\biggl[ \omega^2 \rho_0 r_0^4 + (3\gamma - 4) r_0^3 \frac{dP_0}{dr_0} \biggr] x \biggr\} </math> |
|
|
<math>~=</math> |
<math>~G K_c^{-2} \rho_c^{-2 / 5} \biggl\{ \biggl( \frac{K_c^2 \rho_c^{2/5}}{G} \biggr) \frac{d}{dr^*}\biggl[ (r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr] +\biggl[ \omega^2 \biggl(\frac{K_c^2}{G^2 \rho_c^{8/5}}\biggr) \rho_c \rho^* (r^*)^4 + (3\gamma - 4)\biggl(\frac{K_c }{G \rho_c^{4/5}}\biggr)K_c\rho_c^{6/5} (r^*)^3 \frac{dP^*}{dr^*} \biggr] x \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \frac{d}{dr^*}\biggl[ (r^*)^4 \gamma P^* ~\frac{dx}{dr^*} \biggr] +\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] x \, . </math> |
Now, guided by the accompanying summary, if we multiply through by <math>~4\pi x dr^*</math> and integrate over the entire volume, we obtain the governing variational relation, namely,
|
<math>~0</math> |
<math>~=</math> |
<math>~\int_0^{r^*_\mathrm{core}}4\pi x\cdot d\biggl[ (r^*)^4 \gamma_c P^* ~\frac{dx}{dr^*} \biggr] + \int_0^{r^*_\mathrm{core}}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma_c - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] 4\pi x^2 dr^* </math> |
|
|
|
<math>~ +\int_{r^*_\mathrm{core}}^{R^*}4\pi x\cdot d\biggl[ (r^*)^4 \gamma_e P^* ~\frac{dx}{dr^*} \biggr] + \int_{r^*_\mathrm{core}}^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 + (3\gamma_e - 4)(r^*)^3 \frac{dP^*}{dr^*} \biggr] 4\pi x^2 dr^* </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ 4\pi x(r^*)^4 \gamma_c P^* ~\frac{dx}{dr^*} \biggr]_0^{r^*_\mathrm{core}} - \int_0^{r^*_\mathrm{core}}4\pi \biggl[ (r^*)^4 \gamma_c P^* ~\biggl( \frac{dx}{dr^*} \biggr)^2\biggr] dr^* - \int_0^{r^*_\mathrm{core}}\biggl[ (3\gamma_c - 4)M^*_r \rho^* \biggr] 4\pi x^2 r^* dr^* </math> |
|
|
|
<math>~ +\biggl[ 4\pi x(r^*)^4 \gamma_e P^* ~\frac{dx}{dr^*} \biggr]_{r^*_\mathrm{core}}^{R^*} - \int_{r^*_\mathrm{core}}^{R^*}4\pi \biggl[ (r^*)^4 \gamma_e P^* ~\biggl( \frac{dx}{dr^*} \biggr)^2\biggr] dr^* - \int_{r^*_\mathrm{core}}^{R^*}\biggl[ (3\gamma_e - 4)M^*_r \rho^* \biggr] 4\pi x^2 r^* dr^* + \int_{0}^{R^*}\biggl[ \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 \biggr] 4\pi x^2 dr^* </math> |
|
|
<math>~=</math> |
<math>~ - \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 \gamma_c 4\pi (r^*)^2 P^* dr^* + \int_0^{r^*_\mathrm{core}} (3\gamma_c - 4) x^2\biggl( -\frac{M^*_r}{r^*}\biggr) 4\pi \rho^* (r^*)^2 dr^* -\biggl[ 4\pi x^2 (r^*)^3 \gamma_c P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_0^{r^*_\mathrm{core}} </math> |
|
|
|
<math>~ - \int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 \gamma_e 4\pi (r^*)^2 P^* dr^* + \int_{r^*_\mathrm{core}}^{R^*} (3\gamma_e - 4) x^2\biggl( -\frac{M^*_r}{r^*}\biggr) 4\pi \rho^* (r^*)^2 dr^* -\biggl[ 4\pi x^2 (r^*)^3 \gamma_e P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_{r^*_\mathrm{core}}^{R^*} + \int_{0}^{R^*} 4\pi \biggl( \frac{\omega^2}{G\rho_c}\biggr) \rho^* (r^*)^4 x^2 dr^* \, . </math> |
Energy Normalization:
|
<math>~(\gamma - 1)dU_\mathrm{int}</math> |
<math>~=</math> |
<math>~ 4\pi r^2 P dr </math> |
|
|
<math>~=</math> |
<math>~ 4\pi \biggl[ \frac{K_c^{1 / 2}}{G^{1 / 2} \rho_c^{2 / 5}} \biggr]^3 K_c \rho_c^{6 / 5} (r^*)^2 P^* dr^* </math> |
|
|
<math>~=</math> |
<math>~ 4\pi \biggl[ \frac{K_c^{5}}{G^{3}} \biggr]^{1 / 2} (r^*)^2 P^* dr^* </math> |
|
<math>~\Rightarrow ~~~ E_\mathrm{norm}</math> |
<math>~\equiv</math> |
<math>~ \biggl[ \frac{K_c^{5}}{G^{3}} \biggr]^{1 / 2} </math> |
|
<math>~dW_\mathrm{grav}</math> |
<math>~=</math> |
<math>~ -\biggl( \frac{GM_r}{r} \biggr) 4\pi r^2 \rho dr </math> |
|
|
<math>~=</math> |
<math>~ -\biggl( \frac{GM^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* \biggl[ \frac{K_c}{G\rho_c^{4 / 5}} \biggr]\rho_c \biggl[ \frac{ K_c^{3 / 2} }{G^{3 / 2}\rho_c^{1 / 5} } \biggr] </math> |
|
|
<math>~=</math> |
<math>~ -\biggl( \frac{M^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* E_\mathrm{norm} </math> |
Hence, the dimensionless governing variational relation becomes,
|
<math>~\int_0^{R^*} \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2(r^*)^2 x^2 dM_r^* </math> |
<math>~=</math> |
<math>~ \int_0^{r^*_\mathrm{core}} \gamma_c(\gamma_c - 1) x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int}
- \int_0^{r^*_\mathrm{core}} (3\gamma_c - 4) x^2 dW^*_\mathrm{grav} + \biggl[ 4\pi x^2 (r^*)^3 \gamma_c P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_0^{r^*_\mathrm{core}} </math> |
|
|
|
<math>~ + \int_{r^*_\mathrm{core}}^{R^*} \gamma_e(\gamma_e - 1) x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} - \int_{r^*_\mathrm{core}}^{R^*} (3\gamma_e - 4) x^2 dW^*_\mathrm{grav} + \biggl[ 4\pi x^2 (r^*)^3 \gamma_e P^* \biggl( - \frac{d\ln x}{d\ln r^*} \biggr) \biggr]_{r^*_\mathrm{core}}^{R^*} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} + 4\pi x_i^2 (r_\mathrm{core}^*)^3 \gamma_c P_i^* \biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core} </math> |
|
|
|
<math>~ + \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} - 4\pi x_i^2 (r_\mathrm{core}^*)^3 \gamma_e P_i^* \biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env} </math> |
|
|
<math>~=</math> |
<math>~ \frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} </math> |
|
|
|
<math>~ + \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} - 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^*\biggl[ \gamma_c \biggl\{ \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core} - \gamma_e \biggl\{ \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env} \biggr] </math> |
|
|
<math>~=</math> |
<math>~ \frac{2\gamma_c}{3} \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} </math> |
|
|
|
<math>~ + \frac{2 \gamma_e}{3}\int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dS^*_\mathrm{therm} - (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} - 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^*\biggl[ 3(\gamma_e - \gamma_c) \biggr] \, , </math> |
where,
|
<math>~dM^*_r</math> |
<math>~=</math> |
<math>~4\pi (r^*)^2 \rho^* dr^* \, ,</math> |
|
<math>~dU^*_\mathrm{int}</math> |
<math>~=</math> |
<math>~\frac{1}{(\gamma-1)} \biggl[4\pi (r^*)^2 P^* dr^* \biggr] = \biggl[ \frac{2}{3(\gamma - 1)} \biggr]dS^*_\mathrm{therm} \, ,</math> |
|
<math>~dW^*_\mathrm{grav}</math> |
<math>~=</math> |
<math>~-\biggl( \frac{M^*_r}{r^*} \biggr) 4\pi (r^*)^2 \rho^* dr^* \, .</math> |
Or, for inclusion in our accompanying Tabular Overview,
|
<math>~\biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 \int_0^{R^*} (x r^*)^2 dM_r^* </math> |
<math>~=</math> |
<math>~ \gamma_c (\gamma_c-1) \int_0^{r^*_\mathrm{core}} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} - (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} x^2 dW^*_\mathrm{grav} </math> |
|
|
|
<math>~ + ~\gamma_e (\gamma_e-1) \int_{r^*_\mathrm{core}}^{R^*} x^2~\biggl( \frac{d\ln x}{d\ln r^*} \biggr)^2 dU^*_\mathrm{int} - (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} x^2 dW^*_\mathrm{grav} </math> |
|
|
|
<math>~ + ~3^2(\gamma_c - \gamma_e) x_i^2 P_i^* V_\mathrm{core}^* \, . </math> |
Implementation
|
Table 3 |
|||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| <math>~\frac{\mu_e}{\mu_c}</math> | <math>~\xi_i</math> | Core | Envelope | Virial | |||||||||||||||||||||||||||||||||||||||||||||
| Integrals over<math>~dS^*_\mathrm{therm}</math> | Integrals over<math>~dW^*_\mathrm{grav}</math> | Integrals over<math>~dS^*_\mathrm{therm}</math> | Integrals over<math>~dW^*_\mathrm{grav}</math> | Numerical<math>~\biggl[\frac{2\mathfrak{s}_\mathrm{tot}}{|\mathfrak{w}_\mathrm{tot}|}-1\biggr] </math> | |||||||||||||||||||||||||||||||||||||||||||||
| Analytic <math>~\mathfrak{s}_\mathrm{core}</math> |
Numerical <math>~\mathfrak{s}_\mathrm{core}</math> |
TERM1 | Analytic <math>~\mathfrak{w}_\mathrm{core}</math> |
Numerical <math>~\mathfrak{w}_\mathrm{core}</math> |
TERM2 | Analytic <math>~\mathfrak{s}_\mathrm{env}</math> |
Numerical <math>~\mathfrak{s}_\mathrm{env}</math> |
TERM3 | Analytic <math>~\mathfrak{w}_\mathrm{env}</math> |
Numerical <math>~\mathfrak{w}_\mathrm{env}</math> |
TERM4 | ||||||||||||||||||||||||||||||||||||||
| 1 | 1.6686460157 | 3.021916335 | 3.021921 | 0.116389175 | -3.356583022 | -3.35666 | -2.649752079 | 1.47780476 | 1.47791 | 1.0720821 | -5.642859167 | -5.642820 | -1.91142893 | 0.000020 | |||||||||||||||||||||||||||||||||||
| <math>~\tfrac{1}{2}</math> | 2.27925811317 | 4.241287117 | 4.241410819 | 0.440878529 | -6.074241035 | -6.074317546 | -4.150731169 | 4.284931508 | 4.28547195 | 1.44651932 | -10.97819621 | -10.97847622 | -0.92598634 | 0.000057 | |||||||||||||||||||||||||||||||||||
| 0.345 | 2.560146865247 | 4.639705843 | 4.6399114 | 0.6794857 | -7.125754184 | -7.125854025 | -4.5487829 | 11.72861751 | 11.730381 | 1.51410084 | -25.61089252 | -25.6115597 | -0.4496513 | 0.000097 | |||||||||||||||||||||||||||||||||||
| <math>~\tfrac{1}{3}</math> | 2.582007485476 | 4.667042505 | 4.667254935 | 0.700414598 | -7.200966267 | -7.201068684 | -4.57274936 | 13.15887139 | 13.1608467 | 1.51408246 | -28.45086152 | -28.45153761 | -0.4170461 | 0.000101 | |||||||||||||||||||||||||||||||||||
| 0.309 | 2.6274239687695 | 4.722277318 | 4.722504339 | 0.744964507 | -7.354156963 | -7.3542507 | -4.61961058 | 17.1374434 | 17.1399773 | 1.51055838 | -36.36528446 | -36.36591543 | -0.3524855 | 0.000110 | |||||||||||||||||||||||||||||||||||
| <math>~\tfrac{1}{4}</math> | 2.7357711469398 | 4.84592201 | 4.846185027 | 0.857001395 | -7.70305421 | -7.703178009 | -4.7163542 | 37.84289623 | 37.8479208 | 1.47966673 | -77.67458196 | -77.67408155 | -0.2194152 | 0.000128 | |||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1}</math> |
<math>~=</math> |
<math>~\frac{3}{2\pi \cdot \mathbf{TERM5} } \biggl\{ \frac{2\gamma_c}{3} [\mathbf{TERM1}] - (3\gamma_c - 4) [\mathbf{TERM2}] + \frac{2 \gamma_e}{3} [\mathbf{TERM3}] - (3\gamma_e - 4) [\mathbf{TERM4}] - 4\pi x_i^2 (r_\mathrm{core}^*)^3 P_i^* [3(\gamma_e - \gamma_c)] \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ 0.036312577\times \biggl[0.093111340 - 1.059900832 + 1.4294428 - (-3.82285786) - 1.782200484\times (2.4) \biggr] = 0.036312577\times [0.00819] </math> |
|
|
<math>~=</math> |
<math>~ 0.0002973 \, .</math> |
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 2}</math> |
<math>~=</math> |
<math>~ 0.020704186\times \biggl[0.352702823 - 1.660292468 + 1.928692426 - (-1.85197268) - 1.029029184\times (2.4) \biggr] = 0.020704186\times [0.003405] </math> |
|
|
<math>~=</math> |
<math>~ 0.0000705 \, .</math> |
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 0.345}</math> |
<math>~=</math> |
<math>~ 0.019377703\times \biggl[0.54358856 - 1.81951316 + 2.01880112 - (-0.8993026) - 0.68747441\times (2.4) \biggr] = 0.019377703\times [0.003585] </math> |
|
|
<math>~=</math> |
<math>~ 0.0000695 \, .</math> |
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 3}</math> |
<math>~=</math> |
<math>~ 0.019467503\times \biggl[0.560331678 - 1.81099744 + 2.018776613 - (-0.8340922) - 0.658354814\times (2.4) \biggr] = 0.019467503\times [1.602203052 - 1.580051555] </math> |
|
|
<math>~=</math> |
<math>~ 0.00043123 \, .</math> |
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 0.309}</math> |
<math>~=</math> |
<math>~ 0.019781992\times \biggl[0.595971606 - 1.847844232 + 2.01407784 - (-0.704971) - 0.60905486\times (2.4) \biggr] = 0.019781992\times [1.467176214 - 1.461731665] </math> |
|
|
<math>~=</math> |
<math>~ 0.0001077 \, .</math> |
|
<math>~\biggl[ \sigma_c^2 \biggr]^\mathrm{VP}_{\mu_e/\mu_c = 1 / 4}</math> |
<math>~=</math> |
<math>~ 0.021344909\times \biggl[0.685601116 - 1.88654168 + 1.972888973 - (-0.4388304) - 0.499262049\times (2.4) \biggr] = 0.021344909\times [1.210778809 - 1.198228918] </math> |
|
|
<math>~=</math> |
<math>~ 0.000267876 \, .</math> |
|
Table 4 |
||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| <math>~\frac{\mu_e}{\mu_c}</math> | <math>~\xi_i</math> | Analytic <math>~\mathfrak{m}_\mathrm{tot}</math> |
Numerical <math>~\mathfrak{m}_\mathrm{tot}</math> |
TERM5 | <math>~x_i</math> | <math>~r^*_\mathrm{core}</math> | <math>~P^*_i</math> | <math>~\biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{core}</math> | <math>~\biggl\{ - \frac{d\ln x}{d\ln r^*}\biggr|_i \biggr\}_\mathrm{env}</math> | <math>~\biggl[\sigma_c^2\biggr]^\mathrm{VP}</math> | ||||||||||||||||||||||
| 1 | 1.6686460157 | 4.818155928 | 4.818145 | 13.14874521 | 0.814374698 | 1.153014872 | 0.139506172 | +0.455871977 | +1.47352 | 0.0002973 | ||||||||||||||||||||||
| <math>~\tfrac{1}{2}</math> | 2.27925811317 | 9.020985415 | 9.021084268 | 23.0612702 | 0.653665497 | 1.574940686 | 0.049058481 | +1.059668912 | +1.835801347 | 0.0000705 | ||||||||||||||||||||||
| 0.345 | 2.560146865247 | 17.41399388 | 17.4141672 | 24.63990825 | 0.563043202 | 1.769031527 | 0.030957085 | +1.552125296 | +2.131275177 | 0.0000695 | ||||||||||||||||||||||
| <math>~\tfrac{1}{3}</math> | 2.582007485476 | 18.8449906 | 18.8451614 | 24.52624951 | 0.555549156 | 1.78413696 | 0.029889634 | +1.600041467 | +2.16002488 | 0.0004312 | ||||||||||||||||||||||
| 0.309 | 2.6274239687695 | 22.61541791 | 22.6155686 | 24.13633675 | 0.539776219 | 1.815519219 | 0.027798189 | +1.705239188 | +2.223143513 | 0.0001077 | ||||||||||||||||||||||
| <math>~\tfrac{1}{4}</math> | 2.7357711469398 | 39.12088278 | 39.1208075 | 22.36902623 | 0.501037082 | 1.890385851 | 0.023427588 | +1.991720516 | +2.39503231 | 0.0002679 | ||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||
Revised Free-Energy Analysis
If we set <math>~x </math> = constant in the variational principle relation, we have,
|
<math>~ \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 \int_0^{R^*}(r^*)^2dM_r^* </math> |
<math>~=</math> |
<math>~ - (3\gamma_c - 4) \int_0^{r^*_\mathrm{core}} dW^*_\mathrm{grav} - (3\gamma_e - 4) \int_{r^*_\mathrm{core}}^{R^*} dW^*_\mathrm{grav} - 4\pi (r_\mathrm{core}^*)^3 P_i^*\biggl[ 3(\gamma_e - \gamma_c) \biggr] </math> |
|
<math>~\Rightarrow~~~~~~ \biggl( \frac{2\pi}{3}\biggr)\sigma_c^2 I_\mathrm{sphere}^* </math> |
<math>~=</math> |
<math>~ (4 - 3\gamma_c )\mathfrak{w}_\mathrm{core} + (4 - 3\gamma_e ) \mathfrak{w}_\mathrm{env} + P_i^*V^*_\mathrm{core}\biggl[ 3^2(\gamma_c - \gamma_e) \biggr] \, , </math> |
Notice the similarity between this last expression and the pair of expressions — numbered ⑥ and ⑦ — that arise in the context of pressure-truncated polytropes.
See Also
- K. De et al. (12 October 2018, Science, Vol. 362, No. 6411, pp. 201 - 206), A Hot and Fast Ultra-stripped Supernova that likely formed a Compact Neutron Star Binary.

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |