|
|
| Line 13: |
Line 13: |
| </div> | | </div> |
|
| |
|
| We will draw heavily from the paper published by [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)], and from pp. 458-474 of the review by [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)] in explaining how the ''variational principle'' can be used to identify the eigenvector of the fundamental mode of radial oscillation in spherically symmetric configurations. | | We will draw heavily from the papers published by [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)] and by [http://adsabs.harvard.edu/abs/1964ApJ...139..664C S. Chandrasekhar (1964)], as well as from pp. 458-474 of the review by [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)] in explaining how the ''variational principle'' can be used to identify the eigenvector of the fundamental mode of radial oscillation in spherically symmetric configurations. In an associated "Ramblings" appendix, we provide [[User:Tohline/Appendix/Ramblings/LedouxVariationalPrinciple#Ledoux.27s_Variational_Principle_.28Supporting_Derivations.29|various derivations that support]] this chapter's relatively abbreviated presentation. |
|
| |
|
| ==Ledoux and Pekeris (1941)== | | ==Ledoux and Pekeris (1941)== |
| Line 742: |
Line 742: |
| This also make sense in that the equilibrium configuration should be stable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} > 0</math> — in which case, <math>~\sigma^2</math> is positive; whereas the equilibrium configuration should be ''unstable'' if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} < 0</math> — in which case, <math>~\sigma^2</math> is negative. | | This also make sense in that the equilibrium configuration should be stable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} > 0</math> — in which case, <math>~\sigma^2</math> is positive; whereas the equilibrium configuration should be ''unstable'' if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} < 0</math> — in which case, <math>~\sigma^2</math> is negative. |
|
| |
|
| | =See Also= |
|
| |
|
| {{ LSU_WorkInProgress }}
| | * [[User:Tohline/Appendix/Ramblings/LedouxVariationalPrinciple#Ledoux.27s_Variational_Principle_.28Supporting_Derivations.29|Derivations that support this chapter's discussion of the Ledoux Variational Principle]] |
| | |
| =Our Initial Explorations=
| |
| ==Review by Ledoux and Walraven (1958)==
| |
| Here we are especially interested in understanding the origin of equation (59.10) of [http://adsabs.harvard.edu/abs/1958HDP....51..353L P. Ledoux & Th. Walraven (1958)], which appears in §59 (pp. 464 - 466) of their ''Handbuch der Physik'' article.
| |
| | |
| From our [[User:Tohline/SSC/Perturbations#Summary_Set_of_Nonlinear_Governing_Relations|accompanying summary of the set of nonlinear governing relations]], we highlight the
| |
| | |
| <div align="center">
| |
| <span id="PGE:Euler"><font color="#770000">'''Euler + Poisson Equations'''</font></span><br />
| |
| <math>\frac{d^2 r}{dt^2} = - 4\pi r^2 \frac{dP}{dm} - \frac{Gm}{r^2} </math><br />
| |
| </div>
| |
| | |
| Repeating a result from our [[User:Tohline/SSC/Perturbations#Euler_.2B_Poisson_Equations|separate derivation]], linearization of the two terms on the righthand side of this equation gives,
| |
| | |
| <table align="center" border="0" cellpadding="5">
| |
| <tr>
| |
| <td align="right">
| |
| <math>
| |
| r^2 \frac{dP}{dm}
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| \rightarrow
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>
| |
| r_0^2 \biggl[1 + x~ e^{i\omega t} \biggr]^2 \biggl\{\frac{dP_0}{dm} \biggl[1 + p~ e^{i\omega t} \biggr] + P_0~e^{i\omega t} \frac{dp}{dm} \biggr\} \approx r_0^2 \frac{dP_0}{dm} \biggl[1 + (2x+p)~ e^{i\omega t} \biggr] + P_0 r_0^2~e^{i\omega t} \frac{dp}{dm}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| <tr>
| |
| <td align="right">
| |
| <math>
| |
| \frac{Gm}{r^2}
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| \rightarrow
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>
| |
| \frac{Gm}{ r_0^2} \biggl[1 + x~ e^{i\omega t} \biggr]^{-2} \approx \frac{Gm}{ r_0^2}
| |
| \biggl[1 -2 x~ e^{i\omega t} \biggr] \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| | |
| Adopting the terminology of [http://adsabs.harvard.edu/abs/1958HDP....51..353L Ledoux & Walraven (1958)], the "variation" of each of these terms is obtained by subtracting off the leading order pieces — which presumably cancel in equilibrium. In particular, drawing a parallel with their equation (59.1), we can write,
| |
| <div align="center">
| |
| <table align="center" border="0" cellpadding="5">
| |
| <tr>
| |
| <td align="right">
| |
| <math>
| |
| ~\delta \biggl( - \frac{Gm}{r^2} \biggr)
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| ~\approx
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>
| |
| \frac{Gm}{ r_0^2}
| |
| \biggl[2 x~ e^{i\omega t} \biggr] \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| And, drawing a parallel with their equation (59.2), we have,
| |
| <div align="center">
| |
| <table align="center" border="0" cellpadding="5">
| |
| <tr>
| |
| <td align="right">
| |
| <math>
| |
| ~\delta \biggl( - \frac{1}{\rho} \cdot \frac{dP}{dr} \biggr) = \delta \biggl( - 4\pi r^2 \frac{dP}{dm} \biggr)
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| ~\approx
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>
| |
| -4\pi r_0^2 \frac{dP_0}{dm} \biggl[(2x+p)~ e^{i\omega t} \biggr] - 4\pi P_0 r_0^2~e^{i\omega t} \frac{dp}{dm}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| ~=
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl\{
| |
| \frac{Gm}{r_0^2}\biggl[(2x)\biggr]
| |
| -4\pi r_0^2 \frac{dP_0}{dm} \biggl[(p) \biggr]
| |
| - 4\pi P_0 r_0^2 \frac{dp}{dm} \biggr\} e^{i\omega t}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| ~=
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl\{
| |
| \biggl( \frac{2 Gm}{r_0^2}\biggr) x
| |
| -\frac{1}{\rho_0} \cdot \frac{d}{dr_0} \biggl[ P_0 p \biggr]
| |
| \biggr\} e^{i\omega t} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| Now, if we combined the linearized continuity equation and the linearized (adiabatic form of the) first law of thermodynamics, as [[User:Tohline/SSC/Perturbations#Summary_Set_of_Linearized_Equations|derived elsewhere]], we can write,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~p = \gamma_\mathrm{g} d</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~- \gamma_\mathrm{g} \biggl[
| |
| 3x + r_0 \frac{dx}{dr_0}
| |
| \biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~- \frac{\gamma_\mathrm{g}}{r_0^2} \frac{d}{dr_0} \biggl( r_0^3 x \biggr) \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| Hence,
| |
| <div align="center">
| |
| <table align="center" border="0" cellpadding="5">
| |
| <tr>
| |
| <td align="right">
| |
| <math>
| |
| ~\delta \biggl( - \frac{1}{\rho} \cdot \frac{dP}{dr} \biggr)
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>
| |
| ~\approx
| |
| </math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl\{
| |
| \biggl( \frac{2 Gm}{r_0^2}\biggr) x
| |
| +\frac{1}{\rho_0} \cdot \frac{d}{dr_0} \biggl[ \frac{\gamma_\mathrm{g} P_0}{r_0^2} \frac{d}{dr_0} \biggl( r_0^3 x \biggr) \biggr]
| |
| \biggr\} e^{i\omega t} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| So, given that a mapping from our notation to that used by Ledoux & Walraven (1958) requires <math>~xe^{i\omega t} \rightarrow \zeta/r_0</math>, I understand the origins of their equations (59.1) and (59.2). But I do not yet understand how … <font color="darkgreen">"Accordingly, the acting forces per unit volume can be considered as deriving from a potential density"</font>
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\rho_0 \mathcal{V}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 + \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| It is clear that, once I understand the origin of this expression for the potential density, I will understand how the "Lagrangian density" as defined by their equation (47.8), viz.,
| |
| <div align="center">
| |
| <math>~\mathcal{L} = \rho_0 [\mathcal{K} - \mathcal{V}] \, ,</math>
| |
| </div>
| |
| becomes (see their equation 59.5),
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\mathcal{L}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{\rho_0}{2} {\dot\zeta}^2
| |
| + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| <span id="LDefinition">Noting that,</span> <math>~\dot\zeta = i\omega r_0 x e^{i\omega t}</math>, this in turn gives,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~L \equiv \int_0^R 4\pi r_0^2 \mathcal{L} dr_0</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 4\pi \int_0^R \biggl\{ \frac{\rho_0}{2} {\dot\zeta}^2
| |
| + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 4\pi e^{2i\omega t} \int_0^R \biggl\{ \frac{\rho_0}{2} (i \omega r_0 x)^2
| |
| + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 (r_0 x)^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^3 x)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^2 x^2
| |
| - 4 r_0 x^2 \frac{dP_0}{dr_0} - \frac{\gamma_\mathrm{g} P_0}{r_0^4} \biggl[ 3r_0^2 x + r_0^3 \frac{\partial x}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2
| |
| - \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2
| |
| - 4 r_0^3 x^2 \frac{dP_0}{dr_0}
| |
| - 3\gamma_\mathrm{g} P_0 \biggl[ 3r_0^2 x^2 + 2r_0^3 x \frac{\partial x}{\partial r_0} \biggr] \biggr\}dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2
| |
| - \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2
| |
| - 4 r_0^3 x^2 \frac{dP_0}{dr_0}
| |
| +r_0^3 x^2 \frac{d}{dr_0}\biggl(3\gamma_\mathrm{g}P_0\biggr)
| |
| -\frac{d}{dr_0}\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr]
| |
| \biggr\}dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 2\pi e^{2i\omega t} \biggl\{
| |
| - \int_0^R \rho_0 \omega^2 r_0^4 x^2 dr_0
| |
| - \int_0^R \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0
| |
| + \int_0^R r_0^3 x^2 \frac{d}{dr_0}\biggl[ (3\gamma_\mathrm{g} - 4)P_0\biggr]dr_0
| |
| -\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr]_0^{R}
| |
| \biggr\} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| The group of terms inside the curly braces, here, matches the group of terms inside the curly braces of Ledoux & Walraven's equation (59.8) if we acknowledge that:
| |
| # Our <math>~\omega^2</math> has the same meaning as, but the opposite sign of, their <math>~\sigma^2</math>.
| |
| # Our last term goes to zero because, <math>~r_0 = 0</math> at the center, while <math>~P_0 = 0</math> at the surface.
| |
| | |
| ==LP41 Again==
| |
| After setting the last term to zero, this last expression can be rewritten as,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~2 e^{-2i\omega t} L </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \omega^2 \int_0^R 4\pi\rho_0 r_0^4 x^2 dr_0
| |
| - \int_0^R 4\pi \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0
| |
| - (3\gamma_\mathrm{g} - 4)\int_0^R 4\pi\rho_0 r_0^3 x^2 \biggl( -\frac{1}{\rho_0}\frac{dP_0}{dr_0} \biggr)dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \omega^2 \int_0^R x^2 r_0^2 dm
| |
| - \gamma_\mathrm{g} \int_0^R \biggl[ r_0 \biggl( \frac{\partial x}{\partial r_0}\biggr) \biggr]^2 P_0 dV
| |
| - (3\gamma_\mathrm{g} - 4) \int_0^R r_0 x^2 \biggl( \frac{Gm}{r_0^2} \biggr)dm
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~- \biggl[ \frac{2 e^{-2i\omega t}}{\int_0^R x^2 r_0^2 dm } \biggr] L </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \omega^2
| |
| + \biggl\{ \frac{\gamma_\mathrm{g} \int_0^R \bigl[ r_0 \bigl( \frac{\partial x}{\partial r_0}\bigr) \bigr]^2 P_0 dV
| |
| + (3\gamma_\mathrm{g} - 4) \int_0^R x^2 \bigl( \frac{Gm}{r_0} \bigr)dm}{\int_0^R x^2 r_0^2 dm}
| |
| \biggr\} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| As is explained in detail in §59 (pp. 464 - 465) of [http://adsabs.harvard.edu/abs/1958HDP....51..353L Ledoux & Walraven (1958)], and summarized in §1 of [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)], the function inside the curly braces of this last expression will be minimized if the radially dependent displacement function, <math>~x</math>, is set equal to the eigenfunction of the fundamental mode of radial oscillation, <math>~x_0</math>; and, after evaluation, the minimum value of this expression will be equal to (the negative of) the square of the fundamental-mode oscillation frequency, <math>~\omega^2</math>. This explicit mathematical statement is contained within equation (8) of Ledoux & Pekeris and within equation (59.10) of Ledoux & Walraven.
| |
| | |
| <span id="EnergiesDefined">Now,</span> as we have [[User:Tohline/SphericallySymmetricConfigurations/Virial#Wgrav|discussed separately]] — see, also, p. 64, Equation (12) of [<b>[[User:Tohline/Appendix/References#C67|<font color="red">C67</font>]]</b>] — the gravitational potential energy of the unperturbed configuration is given by the integral,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| <tr>
| |
| <td align="right">
| |
| <math>~W_\mathrm{grav}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ - \int_0^{M} \biggl( \frac{Gm}{r_0} \biggr) dm \, ;</math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| for adiabatic systems, the [[User:Tohline/SphericallySymmetricConfigurations/Virial#Reservoir|internal energy]] is,
| |
| <div align="center">
| |
| <math>
| |
| U_\mathrm{int}
| |
| = \frac{1}{({\gamma_g}-1)} \int_0^R P_0 dV
| |
| \, ;</math>
| |
| </div>
| |
| and — see the text at the top of p. 126 of [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)] — the moment of inertia of the configuration about its center is,
| |
| <div align="center">
| |
| <math>
| |
| I = \int_0^M r_0^2 dm
| |
| \, .</math>
| |
| </div>
| |
| Hence, the function to be minimized may be written as,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \biggl\{ \frac{\gamma_\mathrm{g} (\gamma_\mathrm{g}-1) \int_0^R \bigl[ r_0 \bigl( \frac{\partial x}{\partial r_0}\bigr) \bigr]^2 dU_\mathrm{int}
| |
| - (3\gamma_\mathrm{g} - 4) \int_0^R x^2 dW_\mathrm{grav}}{\int_0^R x^2 dI}
| |
| \biggr\} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| This expression appears in equation (9) of [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)].
| |
| | |
| ==Chandrasekhar (1964)==
| |
| In a paper titled, ''A General Variational Principle Governing the Radial and the Non-Radial Oscillations of Gaseous Masses'', [http://adsabs.harvard.edu/abs/1964ApJ...139..664C S. Chandrasekhar (1964, ApJ, 139, 664)] independently derived the Ledoux-Pekeris Lagrangian.
| |
| | |
| ===The Lagrangian Expression using Chandrasekhar's Notation===
| |
| First, let's show that the Lagrangian expression derived by Chandrasekhar is, indeed, equivalent to the one presented by Ledoux & Pekeris. Returning to the second line of our effort to simplify the [[#LDefinition|above definition of the Lagrangian]], and making the substitution, <math>~\psi \equiv r_0^3 x</math>, we have,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~2L </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 4\pi e^{2i\omega t} \int_0^R \biggl\{ \rho_0 \biggl( \frac{i \omega \psi}{r_0^2} \biggr)^2
| |
| + \biggl( \frac{4Gm}{r_0^3}\biggr) \rho_0 \biggl( \frac{\psi}{r_0^2} \biggr)^2
| |
| - \gamma_\mathrm{g} P_0 \biggl[ \frac{1}{r_0^2} \frac{\partial(\psi)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 4\pi e^{2i\omega t} \int_0^R \biggl\{ -\omega^2 \rho_0 \biggl( \frac{\psi}{r_0} \biggr)^2
| |
| - 4\biggl( \frac{dP_0}{dr_0}\biggr) \biggl( \frac{\psi^2}{r_0^3} \biggr)
| |
| - \gamma_\mathrm{g} P_0 \biggl[ \frac{1}{r_0} \frac{\partial(\psi)}{\partial r_0} \biggr]^2 \biggr\}dr_0
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~- 4\pi e^{2i\omega t} \int_0^R \biggl\{ \omega^2 \rho_0 \psi^2
| |
| + 4\biggl( \frac{dP_0}{dr_0}\biggr) \biggl( \frac{\psi^2}{r_0} \biggr)
| |
| + \gamma_\mathrm{g} P_0 \biggl[ \frac{\partial\psi}{\partial r_0} \biggr]^2 \biggr\} \frac{dr_0}{r_0^2} .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| This integral expression matches the integral expression that appears in equation (49) of [http://adsabs.harvard.edu/abs/1964ApJ...139..664C Chandrasekhar (1964)], if we accept that our squared frequency, <math>~\omega^2</math>, has the opposite sign to Chandrasekhar's <math>~\sigma^2</math>. Chandrasekhar acknowledged that, for radial modes of oscillation, his result was the same as that derived earlier by Ledoux and his collaborators.
| |
| | |
| ===Chandrasekhar's Independent Derivation===
| |
| Now, let's follow Chandrasekhar's lead and derive the Lagrangian directly from the governing LAWE. We begin with a version of the LAWE that [[#RewrittenLAWE|appears above]] in our review of the paper by [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)], namely,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~0</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr]
| |
| +\biggl[ \sigma^2 \rho r^4 + (3\Gamma_1 - 4) r^3 \frac{dP}{dr} \biggr] \xi \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| We will develop the Lagrangian expression by following the guidance provided at the top of p. 666 of [http://adsabs.harvard.edu/abs/1964ApJ...139..664C S. Chandrasekhar (1964, ApJ, 139, 664)]. First, we multiply the LAWE through by the fractional displacement, <math>~\xi</math>; second, we make the substitution, <math>~\xi \rightarrow \psi/r^3</math>, in order to shift to Chandrasekhar's variable notation; then we multiply through by <math>~dr</math> and integrate from the center <math>~(r = 0)</math> to the surface <math>~(r = R)</math> of the configuration.
| |
| | |
| Multiplying through by the fractional displacement gives,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\sigma^2 \rho r^4 \xi^2</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| -\xi \cdot \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr]
| |
| - (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| Next, making the stated variable substitution gives,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\frac{\sigma^2 \rho \psi^2}{r^2}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d}{dr} \biggl( \frac{\psi}{r^3} \biggr) \biggr]
| |
| - (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} -3 \Gamma_1 P \psi ~\biggr]
| |
| - (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| (4-3\Gamma_1 ) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| - \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} \biggr]
| |
| + 3 \Gamma_1 \biggl( \frac{\psi^2}{r^3}\biggr) \frac{dP}{dr}
| |
| +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
| |
| - \biggl\{
| |
| \frac{d}{dr}\biggl[ r \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}\biggr] -r\Gamma_1 P ~\frac{d\psi}{dr} \cdot \frac{d}{dr}\biggl( \frac{\psi}{r^3}\biggr)
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| +3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
| |
| + \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2
| |
| - \biggl[\frac{3\Gamma_1 P\psi}{r^3}\biggr]\frac{d\psi}{dr}
| |
| - \frac{d}{dr}\biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
| |
| + \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2
| |
| - \frac{d}{dr}\biggl[ \frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr] \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| Finally, integrating over the volume gives,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\int_0^R (\sigma^2 \rho \psi^2)\frac{dr}{r^2}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \int_0^R \biggl[ \Gamma_1 P \biggl(\frac{d\psi}{dr} \biggr)^2
| |
| + \frac{4\psi^2}{r} \biggl( \frac{dP}{dr} \biggr) \biggr]\frac{dr}{r^2}
| |
| - \biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr]_0^R \, ,
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| which is identical to equation (49) of [http://adsabs.harvard.edu/abs/1964ApJ...139..664C Chandrasekhar (1964)], if the last term — the difference of the central and surface boundary conditions — is set to zero.
| |
| | |
| =Examples=
| |
| | |
| ==Ledoux's Expression==
| |
| Returning to the last line of our [[#LDefinition|above definition of the Lagrangian]], that is,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~L </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 2\pi e^{2i\omega t} \biggl\{
| |
| - \int_0^R \rho_0 \omega^2 r_0^4 x^2 dr_0
| |
| - \int_0^R \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0
| |
| + \int_0^R r_0^3 x^2 \frac{d}{dr_0}\biggl[ (3\gamma_\mathrm{g} - 4)P_0\biggr]dr_0
| |
| -\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr]_0^{R}
| |
| \biggr\} \, ,
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| let's attempt to evaluate the terms inside the curly braces for the case of pressure-truncated polytropic configurations because, as has been discussed separately, we have an analytic expression for the eigenvector of the fundamental-mode of radial oscillation. Dividing through by <math>~P_c R_\mathrm{eq}^3</math> and making the substitution, <math>~r_0/R_\mathrm{eq} \rightarrow \xi/\tilde\xi</math>, gives,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\frac{L_{\{\}} }{P_c R_\mathrm{eq}^3}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \int_0^R \frac{\rho_0 \omega^2}{P_c R_\mathrm{eq}^3} r_0^4 x^2 dr_0
| |
| - \int_0^R \gamma_\mathrm{g} \frac{P_0}{P_c R_\mathrm{eq}^3} r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0
| |
| + \int_0^R r_0^3 x^2 \frac{d}{dr_0}\biggl[ (3\gamma_\mathrm{g} - 4)\frac{P_0}{P_c R_\mathrm{eq}^3}\biggr]dr_0
| |
| - 3 \gamma_\mathrm{g} x_\mathrm{surf}^2 \frac{P_e}{P_c}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \int_0^{\tilde\xi} \omega^2 \biggl[\frac{\rho_c R_\mathrm{eq}^2}{P_c } \biggr] \biggl( \frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{\xi}{\tilde\xi}\biggr)^4 x^2 \frac{d\xi}{\tilde\xi}
| |
| - \int_0^{\tilde\xi} \gamma_\mathrm{g} \biggl(\frac{P_0}{P_c }\biggr) \biggl(\frac{\xi}{\tilde\xi}\biggr)^4\biggl[ \frac{\partial x}{\partial (\xi/\tilde\xi)}\biggr]^2 \frac{d\xi}{\tilde\xi}
| |
| + \int_0^{\tilde\xi} \biggl(\frac{\xi}{\tilde\xi}\biggr) x^2 \frac{d}{d(\xi/\tilde\xi)}\biggl[ (3\gamma_\mathrm{g} - 4)\frac{P_0}{P_c }\biggr] \frac{d\xi}{\tilde\xi}
| |
| - 3 \gamma_\mathrm{g} x_\mathrm{surf}^2 \frac{P_e}{P_c}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \omega^2 \biggl[\frac{\rho_c R_\mathrm{eq}^2}{P_c ~{\tilde\xi}^5} \biggr] \int_0^{\tilde\xi} \theta^n \xi^4 x^2 d\xi
| |
| - \frac{\gamma_\mathrm{g}}{ {\tilde\xi}^3} \int_0^{\tilde\xi} \theta^{n+1} \xi^4\biggl[ \frac{\partial x}{\partial \xi}\biggr]^2 d\xi
| |
| + \frac{(3\gamma_\mathrm{g} - 4)}{\tilde\xi}\int_0^{\tilde\xi} \xi x^2 \frac{d}{d\xi}\biggl[ \theta^{n+1}\biggr] d\xi
| |
| - \biggl[\frac{3 \gamma_\mathrm{g}P_e}{P_c} \biggr]x_\mathrm{surf}^2
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \omega^2 \biggl[\frac{\rho_c R_\mathrm{eq}^2}{P_c ~{\tilde\xi}^5} \biggr] \int_0^{\tilde\xi} \theta^n \xi^4 x^2 d\xi
| |
| - \biggl[\frac{3 \gamma_\mathrm{g}P_e}{P_c} \biggr]x_\mathrm{surf}^2
| |
| + \frac{1}{ {\tilde\xi}^3} \int_0^{\tilde\xi} \biggl[ (3\gamma_\mathrm{g} - 4) {\tilde\xi}^2 \xi x^2 \frac{d\theta^{n+1}}{d\xi}
| |
| - \gamma_\mathrm{g} \theta^{n+1} \xi^4\biggl( \frac{\partial x}{\partial \xi}\biggr)^2 \biggr]d\xi
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| where, we have set the pressure at the (truncated) surface to the value, <math>~P_0|_\mathrm{surface} = P_e</math>.
| |
| | |
| ==Chandra's Expression==
| |
| ===Normalization===
| |
| | |
| Alternatively, starting from Chandrasekhar's expression,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~2L_{\{\}} </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\int_0^R \biggl\{ \omega^2 \rho_0 (r_0^3 x)^2
| |
| + 4\biggl( \frac{dP_0}{dr_0}\biggr) \frac{(r_0^3 x)^2}{r_0}
| |
| + \gamma_\mathrm{g} P_0 \biggl[ \frac{\partial (r_0^3 x)}{\partial r_0} \biggr]^2 \biggr\} \frac{dr_0}{r_0^2}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\Rightarrow ~~~ \frac{2L_{\{\}} }{P_c R_\mathrm{eq}^3}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\int_0^R \biggl\{ \frac{\omega^2 \rho_c}{P_c} \biggl( \frac{\rho_0}{\rho_c}\biggr) r_0^4 x^2
| |
| + 4 r_0^3 x^2 \cdot \frac{d}{dr_0}\biggl(\frac{P_0}{P_c}\biggr)
| |
| + \frac{\gamma_\mathrm{g} P_0}{r_0^2 P_c} \biggl[ \frac{\partial (r_0^3 x)}{\partial r_0} \biggr]^2 \biggr\} \frac{dr_0 }{R_\mathrm{eq}^3}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\int_0^{\tilde\xi} \biggl\{ \frac{\omega^2 \rho_c R_\mathrm{eq}^2}{P_c} \biggl( \frac{\rho_0}{\rho_c}\biggr) \biggl(\frac{\xi}{ {\tilde\xi}} \biggr)^4 x^2
| |
| + 4 \tilde\xi ~x^2 \biggl(\frac{\xi}{ {\tilde\xi}} \biggr)^3 \frac{d}{d\xi}\biggl(\frac{P_0}{P_c}\biggr)
| |
| + \frac{\gamma_\mathrm{g} P_0}{ P_c} \biggl(\frac{\xi}{ {\tilde\xi}} \biggr)^{-2}\biggl[ \frac{\partial }{\partial (\xi/\tilde\xi)}\biggl(\frac{\xi^3 x}{ {\tilde\xi}^3} \biggr) \biggr]^2 \biggr\} \frac{d\xi }{ {\tilde\xi}}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\int_0^{\tilde\xi} \biggl\{ \frac{\omega^2 \rho_c R_\mathrm{eq}^2}{{\tilde\xi}^4 P_c} \biggl( \theta^n \xi^4 \biggr) x^2
| |
| + \biggl(\frac{4 }{{\tilde\xi}^2}\biggr) ~x^2 \xi^3 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr]
| |
| + \frac{\gamma_\mathrm{g} }{ {\tilde\xi}^2} \biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \biggl[ \frac{\partial (\xi^3 x)}{\partial \xi} \biggr]^2 \biggr\} \frac{d\xi }{ {\tilde\xi}}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{1}{ {\tilde\xi}^3}\int_0^{\tilde\xi} \biggl\{ \frac{\omega^2 \rho_c R_\mathrm{eq}^2}{{\tilde\xi}^2 P_c} \biggl( \theta^n \xi^4 \biggr) x^2
| |
| + 4x^2 \xi^3 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr]
| |
| + \gamma_\mathrm{g} \biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \biggl[ \frac{\partial (\xi^3 x)}{\partial \xi} \biggr]^2 \biggr\} d\xi \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| ===Known Analytic Eigenfunction===
| |
| Now, let's plug in the [[User:Tohline/SSC/Stability/InstabilityOnsetOverview#Analyses_of_Radial_Oscillations|known eigenfunction for the marginally unstable configuration]], namely,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| <tr>
| |
| <td align="center" colspan="3"><font color="maroon"><b>Exact Solution to the <math>~(3 \le n < \infty)</math> Polytropic LAWE</b></font></td>
| |
| </tr>
| |
| <tr>
| |
| <td align="right">
| |
| <math>~\sigma_c^2 = 0</math>
| |
| </td>
| |
| <td align="center">
| |
| and
| |
| </td>
| |
| <td align="left">
| |
| <math>~x_P \equiv \frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{1}{\xi \theta^{n}}\biggr) \frac{d\theta}{d\xi}\biggr] \, .</math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| Given that, from the Lane-Emden equation,
| |
| <div align="center">
| |
| <math>~\frac{\theta^{''}}{\theta^n} = - 1 -\frac{2\theta^'}{\xi \theta^n} \, ,</math>
| |
| </div>
| |
| | |
| we recognize that,
| |
| | |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\frac{d(\xi^3 x_P)}{d\xi}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{3(n-1)}{2n} \frac{d\xi^3}{d\xi}
| |
| +\frac{3(n-3)}{2n} \frac{d}{d\xi} \biggl( \frac{\xi^2 \theta^'}{\theta^{n}}\biggr)
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{3^2(n-1)\xi^2}{2n}
| |
| +\frac{3(n-3)\xi^2}{2n} \biggl[ \frac{\theta^{''}}{\theta^{n}} + \frac{2\theta^'}{\xi \theta^{n}} - \frac{n (\theta^')^2}{\theta^{n+1}}\biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{3\xi^2}{2n} \biggl\{ 3(n-1)
| |
| -(n-3)\biggl[ 1 + \frac{n (\theta^')^2}{\theta^{n+1}}\biggr] \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{3\xi^2}{2} \biggl\{ 2 + (3-n)\biggl[ \frac{(\theta^')^2}{\theta^{n+1}}\biggr]\biggr\} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| ===General Evaluation===
| |
| Therefore, returning to Chandrasekhar's expression for the Lagrangian and evaluating the sum of the last two terms inside the curly braces gives,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\biggl\{\sum^2\biggr\}_{x_P}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~\equiv</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl\{4x^2 \xi^3 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr]
| |
| + \gamma_\mathrm{g} \biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \biggl[ \frac{d (\xi^3 x)}{d \xi} \biggr]^2\biggr\}_{x_P} </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4(n+1) \biggl\{ \frac{3(n-1)}{2n}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{\theta^'}{\xi \theta^{n}}\biggr) \biggr] \biggr\}^2 \xi^3 \theta^n \theta^'
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| + \frac{(n+1)}{n} \biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \frac{3^2\xi^4}{2^2} \biggl\{ 2 + (3-n)\biggl[ \frac{(\theta^')^2}{\theta^{n+1}}\biggr]\biggr\}^2
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4(n+1) \frac{3^2(n-1)^2}{2^2n^2}\biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{\theta^'}{\xi \theta^{n}}\biggr) \biggr]^2 \xi^3 \theta^n \theta^'
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| + \frac{(n+1)}{n} \biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \frac{3^2\xi^4}{2^2} \biggl[ 2 + \frac{(3-n)(\theta^')^2}{\theta^{n+1}}\biggr]^2
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi^2 }{2^2n^2}\biggl\{
| |
| 2^2(n-1)^2 \biggl[1 + \biggl(\frac{n-3}{n-1}\biggr) \biggl( \frac{\theta^'}{\xi \theta^{n}}\biggr) \biggr]^2 \xi \theta^n \theta^'
| |
| + n \theta^{n+1} \biggl[ 2 + \frac{(3-n)(\theta^')^2}{\theta^{n+1}}\biggr]^2 \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi^2 }{2^2n^2}\biggl\{
| |
| 2^2 \biggl[(n-1)\xi \theta^{n} + (n-3)\theta^' \biggr]^2 \frac{ \theta^'}{\xi \theta^{n}}
| |
| + \frac{n}{\theta^{n+1} } \biggl[ 2\theta^{n+1} + (3-n)(\theta^')^2\biggr]^2 \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi }{2^2n^2 \theta^{n+1}}\biggl\{
| |
| n\xi \biggl[ 2\theta^{n+1} + (3-n)(\theta^')^2\biggr]^2
| |
| +2^2 \theta\theta^'\biggl[(n-1)\xi \theta^{n} + (n-3)\theta^' \biggr]^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi }{2^2n^2 \theta^{n+1}}\biggl\{
| |
| n\xi \biggl[ 2^2\theta^{2(n+1)} + 4(3-n) \theta^{n+1}(\theta^')^2 + (3-n)^2 (\theta^')^4\biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| +2^2 \theta\theta^'\biggl[(n-1)^2\xi^2 \theta^{2n} + 2(n-1)(n-3) \xi \theta^{n}\theta^' + (n-3)^2 (\theta^')^2 \biggr]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi }{2^2n^2 \theta^{n+1}}\biggl\{
| |
| n\xi \biggl[ 2^2\theta^{2(n+1)} + (3-n)^2 (\theta^')^4\biggr]
| |
| +2^2 \theta\theta^'\biggl[ (n-1)^2\xi^2 \theta^{2n} + (n-3)^2 (\theta^')^2 \biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| + \biggl[ 2^3(n-1)(n-3) - 4n(n-3)\biggr] \xi \theta^{n+1} (\theta^')^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| | |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{3^2(n+1) \xi }{2^2n^2 \theta^{n+1}}\biggl\{
| |
| n\xi \biggl[ 2^2\theta^{2(n+1)} + (3-n)^2 (\theta^')^4\biggr]
| |
| +2^2 \theta\theta^'\biggl[ (n-1)^2\xi^2 \theta^{2n} + (n-3)^2 (\theta^')^2 \biggr]
| |
| + 2^2 (n^2- 5n +6) \xi \theta^{n+1} (\theta^')^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| | |
| ===Application to n = 5 Polytropic Configuration===
| |
| | |
| Let's try plugging in expressions for n = 5 configurations, for which the [[User:Tohline/SSC/Structure/PolytropesEmbedded#Tabular_Summary_.28n.3D5.29|Lane-Emden function is known analytically]]. Specifically,
| |
| <div align="center">
| |
| <math>~\theta_{n=5} = \biggl(1 + \frac{\xi^2}{3}\biggr)^{-1 / 2}</math> and <math>~\theta_{n=5}^' = - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2} \, .</math>
| |
| </div>
| |
| | |
| ====First Attempt====
| |
| We have,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| | |
| <tr>
| |
| <td align="right">
| |
| <math>~\biggl\{\sum^2\biggr\}_{x_P}^{n=5}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^3 \xi }{5^2 \theta^{6}}\biggl\{
| |
| 5\xi \biggl[ \theta^{12} + (\theta^')^4\biggr]
| |
| + 2^4 \theta\theta^'\biggl[ \xi^2 \theta^{10} + (\theta^')^2 \biggr]
| |
| + 2\cdot 3 \xi \theta^{6} (\theta^')^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
|
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^3 \xi }{5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{3}\biggl\{
| |
| 5\xi \biggl[ \biggl(1 + \frac{\xi^2}{3}\biggr)^{-6} + \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2}\biggr]^4\biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| + 2^4 \biggl(1 + \frac{\xi^2}{3}\biggr)^{-1 / 2} \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2}\biggr] \biggl[ \xi^2 \biggl(1 + \frac{\xi^2}{3}\biggr)^{-5} + \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2}\biggr]^2 \biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| + 2\cdot 3 \xi \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3} \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2}\biggr]^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^3 \xi }{5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{3}\biggl\{
| |
| 5\xi \biggl[ \biggl(1 + \frac{\xi^2}{3}\biggr)^{-6} + \frac{\xi^4}{3^4} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-6} \biggr]
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
|
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \frac{2^4\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-2} \biggl[ \xi^2 \biggl(1 + \frac{\xi^2}{3}\biggr)^{-5} + \frac{\xi^2}{3^2} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 } \biggr]
| |
| + \frac{2\xi^3}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-6 }
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^3 \xi^2 }{5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4}\biggl\{
| |
| 5\biggl(1 + \frac{\xi^2}{3}\biggr) \biggl[ 1 + \frac{\xi^4}{3^4} \biggr]
| |
| + \frac{2\xi^2}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)
| |
| - \frac{2^4\xi^2}{3} \biggl[ 1 + \frac{1}{3^2} \biggl(1 + \frac{\xi^2}{3}\biggr)^{2 } \biggr]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^3 \xi^2 }{5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4}\biggl\{
| |
| \frac{5}{3^5}\biggl[3^5 + 3^4\xi^2 + 3\xi^4 + \xi^6 \biggr]
| |
| + \frac{2}{3^2} \biggl(3\xi^2 + \xi^4\biggr)
| |
| - \frac{2^4\xi^2}{3^5} \biggl[ 3^4 + 3^2 + 2\cdot 3\xi^2 + \xi^4 \biggr]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2 \xi^2 }{3^2\cdot 5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4}\biggl\{
| |
| 5 \biggl[3^5 + 3^4\xi^2 + 3\xi^4 + \xi^6 \biggr]
| |
| + 2\cdot 3^3 \biggl(3\xi^2 + \xi^4\biggr)
| |
| - 2^4 \biggl[ 2\cdot 3^2\cdot 5 \xi^2 + 2\cdot 3\xi^4 + \xi^6 \biggr]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2 \xi^2 }{3^2\cdot 5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4}\biggl\{
| |
| 3^5\cdot 5 + 3^2\xi^2 [3^2\cdot 5 + 2\cdot 3^2 - 2^5 \cdot 5 ] + 3\xi^4 [ 5 + 2\cdot 3^2 - 2^5] + \xi^6 [5 -2^4]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2 \xi^2 }{3^2\cdot 5^2 } \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4}\biggl[
| |
| 3^5\cdot 5 - 3^2 \cdot 97\xi^2 - 3^3 \xi^4 - 11 \xi^6
| |
| \biggr] \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
|
| |
| ====Second Attempt====
| |
|
| |
| First, we evaluate,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
| <tr>
| |
| <td align="right">
| |
| <math>~\biggl\{ x_P \biggr\}^{n=5}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3}{5}\biggl[1 - \frac{1}{2\xi} \biggl(1 + \frac{\xi^2}{3}\biggr)^{5 / 2} \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2} \biggr] </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{1}{5}\biggl[6 - \biggl(1 + \frac{\xi^2}{3}\biggr) \biggr] </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~1 - \frac{\xi^2}{3\cdot 5} \, ; </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
|
| |
| and,
| |
|
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~\biggl\{ \frac{d(\xi^3 x_P)}{d\xi} \biggr\}^{n=5}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 3\xi^2 \biggl\{ 1 -\biggl[ \frac{(\theta^')^2}{\theta^{6}}\biggr]\biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 3\xi^2 \biggl\{ 1 - \biggl[ \biggl(1 + \frac{\xi^2}{3}\biggr)^{-1 / 2} \biggr]^{-6} \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2} \biggr]^2 \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 3\xi^2 \biggl( 1 - \frac{\xi^2}{3^2} \biggr) \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
|
| |
| Then, returning to Chandrasekhar's expression for the Lagrangian and evaluating the sum of the last two terms inside the curly braces gives,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~\biggl\{\sum^2\biggr\}^{n=5}_{x_P}</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl\{4x^2 \xi^3 \biggl[ \frac{d\theta^{n+1}}{d\xi} \biggr]
| |
| + \frac{n+1}{n}\biggl( \frac{\theta^{n+1}}{\xi^2}\biggr) \biggl[ \frac{d (\xi^3 x)}{d \xi} \biggr]^2\biggr\}^{n=5}_{x_P} </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl[\theta_{n=5}\biggr]^5\biggl\{ 2^3\cdot 3 \xi^3 \biggl[x_P \biggr]^2 \biggl[ \frac{d\theta}{d\xi} \biggr]
| |
| + \frac{2\cdot 3}{5\xi^2}\biggl[ \theta \biggr] \biggl[ \frac{d (\xi^3 x_P)}{d \xi} \biggr]^2 \biggr\}^{n=5}</math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl[ \biggl(1 + \frac{\xi^2}{3}\biggr)^{-1 / 2}\biggr]^5\biggl\{
| |
| \frac{2\cdot 3}{5\xi^2}\biggl[ \biggl(1 + \frac{\xi^2}{3}\biggr)^{-1 / 2}\biggr] \biggl[ 3\xi^2 \biggl( 1 - \frac{\xi^2}{3^2} \biggr) \biggr]^2
| |
| + 2^3\cdot 3 \xi^3 \biggl[3\xi^2 \biggl( 1 - \frac{\xi^2}{3^2} \biggr) \biggr]^2 \biggl[ - \frac{\xi}{3} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-3 / 2} \biggr]
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\biggl(1 + \frac{\xi^2}{3}\biggr)^{-4} \biggl\{
| |
| \frac{2\cdot 3^3 \xi^2}{5} \biggl( 1 - \frac{\xi^2}{3^2} \biggr)^2 \biggl(1 + \frac{\xi^2}{3}\biggr)
| |
| - 2^3\cdot 3^2 \xi^8 \biggl( 1 - \frac{\xi^2}{3^2} \biggr)^2
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{1}{3\cdot 5} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4} \biggl( 1 - \frac{\xi^2}{3^2} \biggr)^2 \biggl\{
| |
| 2\cdot 3^3 \xi^2 (3 + \xi^2 )
| |
| - 2^3\cdot 3^3\cdot 5 \xi^8
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~\frac{2\cdot 3^2 \xi^2}{5} \biggl(1 + \frac{\xi^2}{3}\biggr)^{-4} \biggl( 1 - \frac{\xi^2}{3^2} \biggr)^2 \biggl\{
| |
| 3 + \xi^2 - 2^2\cdot 5 \xi^6
| |
| \biggr\} \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
|
| |
| =Normalizations=
| |
| Returning to the last line of our derivation of the [[#LDefinition|Ledoux & Walraven Lagrangian]], we can write,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~
| |
| \biggl[\frac{2}{\gamma_\mathrm{g}} \biggr]e^{-2i\omega t} L + 4\pi \int_0^R \rho_0 \biggl(\frac{\omega^2}{\gamma_\mathrm{g}}\biggr) r_0^4 x^2 dr_0
| |
| </math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~ 4\pi \biggl\{
| |
| - \int_0^R P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0
| |
| + \int_0^R r_0^3 x^2 \frac{d}{dr_0}\biggl[ \biggl(3 - \frac{4}{\gamma_\mathrm{g}} \biggr)P_0\biggr]dr_0
| |
| -\biggl[3 r_0^3 x^2 P_0\biggr]_0^{R}
| |
| \biggr\}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \int_0^R \biggl[r_0\biggl( \frac{\partial x}{\partial r_0}\biggr) \biggr]^2 4\pi P_0 r_0^2 dr_0
| |
| - \biggl(3 - \frac{4}{\gamma_\mathrm{g}} \biggr) \int_0^R \biggl[ x^2 \biggr] \biggl(- \frac{r_0}{\rho_0} ~\frac{dP_0}{dr_0}\biggr) 4\pi r_0^2 \rho_0 dr_0
| |
| - 4\pi \biggl[3 r_0^3 x^2 P_0\biggr]_0^{R}
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \int_0^R \biggl[r_0\biggl( \frac{\partial x}{\partial r_0}\biggr) \biggr]^2 4\pi P_0 r_0^2 dr_0
| |
| - \biggl(3 - \frac{4}{\gamma_\mathrm{g}} \biggr) \int_0^R \biggl[ x^2 \biggr] \biggl(\frac{GM_r}{r_0}\biggr) 4\pi r_0^2 \rho_0 dr_0
| |
| - \biggl[ 3x \biggr]^2 \frac{4\pi R^3}{3} P_e \, .
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
|
| |
| =Alternate Approach: Integrate Over LAWE=
| |
|
| |
| As we have demonstrated, [[#Ledoux_and_Pekeris_.281941.29|above]], if we assume that <math>~\Gamma_1</math> is constant throughout the configuration, our version of the LAWE can be straightforwardly rearranged to give equation (58.1) of [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)], that is,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~0</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{dx}{dr} \biggr]
| |
| +\biggl[ \sigma^2 \rho r^4 + (3\Gamma_1 - 4) r^3 \frac{dP}{dr} \biggr] x \, ,
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| where, we are using <math>~x</math> in place of <math>~\xi</math> to represent the fractional Lagrangian displacement, <math>~\delta r/r</math>. If we multiply this expression through by <math>~4 \pi dr</math> and integrate over the entire volume of the configuration, we have,
| |
| <div align="center">
| |
| <table border="0" cellpadding="5" align="center">
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~0</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| 4\pi \int_0^R \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{dx}{dr} \biggr] dr
| |
| + \int_0^R\biggl[ \sigma^2 x r^2 + (3\Gamma_1 - 4) x \biggl( \frac{r}{\rho} \frac{dP}{dr}\biggr) \biggr] 4\pi r^2 \rho dr
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \biggl[4\pi r^4 \Gamma_1 P \biggl(\frac{dx}{dr}\biggr) \biggr]_0^R
| |
| + \int_0^R\biggl[ \sigma^2 x r^2 + (3\Gamma_1 - 4) x \biggl( -\frac{Gm}{r}\biggr) \biggr] dm
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
|
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| \biggl[4\pi r^4 \Gamma_1 P \biggl(\frac{dx}{dr}\biggr) \biggr]_0^R
| |
| + \sigma^2 \int_0^R x ~dI
| |
| + \int_0^R (3\Gamma_1 - 4) x ~dW_\mathrm{grav} \, ,
| |
| </math>
| |
| </td>
| |
| </tr>
| |
|
| |
| <tr>
| |
| <td align="right">
| |
| <math>~\Rightarrow ~~~ \sigma^2</math>
| |
| </td>
| |
| <td align="center">
| |
| <math>~=</math>
| |
| </td>
| |
| <td align="left">
| |
| <math>~
| |
| - \biggl\{
| |
| \int_0^R (3\Gamma_1 - 4) x ~dW_\mathrm{grav} + \biggl[4\pi r^4 \Gamma_1 P \biggl(\frac{dx}{dr}\biggr) \biggr]_0^R \biggr\} \biggl[\int_0^R x ~dI
| |
| \biggr]^{-1} \, ,
| |
| </math>
| |
| </td>
| |
| </tr>
| |
| </table>
| |
| </div>
| |
| where the definitions of <math>~dW_\mathrm{grav}</math> and <math>~dI</math> are as [[#EnergiesDefined|provided, above]]. This last expression is the same as equation (59.17) of [http://adsabs.harvard.edu/abs/1941ApJ....94..124L Ledoux & Pekeris (1941)], except that: these authors have retained a term allowing for radial variation of <math>~\Gamma_1</math>, whereas we have not; and we have retained a ''boundary'' term that can accommodate a nonzero surface pressure, whereas Ledoux & Pekeris have not.
| |
|
| |
| =See Also=
| |
|
| |
|
| {{LSU_HBook_footer}} | | {{LSU_HBook_footer}} |
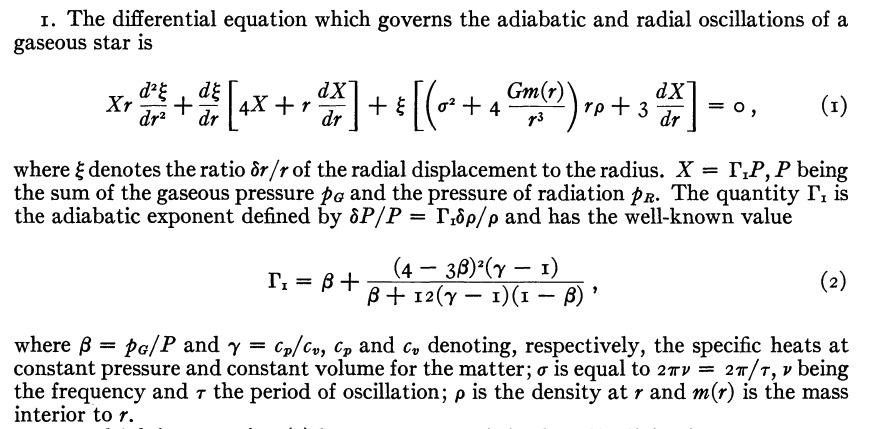
Ledoux's Variational Principle
All of the discussion in this chapter will build upon our derivation elsewhere of the,
LAWE: Linear Adiabatic Wave (or Radial Pulsation) Equation
|

|
<math>~
\frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0}
+ \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0
</math>
|
We will draw heavily from the papers published by Ledoux & Pekeris (1941) and by S. Chandrasekhar (1964), as well as from pp. 458-474 of the review by P. Ledoux & Th. Walraven (1958) in explaining how the variational principle can be used to identify the eigenvector of the fundamental mode of radial oscillation in spherically symmetric configurations. In an associated "Ramblings" appendix, we provide various derivations that support this chapter's relatively abbreviated presentation.
Ledoux and Pekeris (1941)
Historically, by the 1940s, the LAWE was a relatively familiar one to astrophysicists. For example, the opening paragraph of a 1941 paper by Ledoux & Pekeris (1941, ApJ, 94, 124), reads:
If we divide their equation (1) through by <math>~Xr = \Gamma_1 P r</math> and recognize that,
<math>
\frac{dX}{dr} = \frac{dX}{dm}\frac{dm}{dr} = - \Gamma_1 g_0 \rho \, ,
</math>
we obtain,
<math>
\frac{d^2\xi}{dr^2} + \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\frac{\rho}{\Gamma_1 P} \biggl[ \sigma^2 + (4 - 3\Gamma_1) \frac{g_0}{r} \biggr] \xi = 0 \, .
</math>
Clearly, this 2nd-order, ordinary differential equation is the same as our derived LAWE, but with a more general definition of the adiabatic exponent that allows consideration of a situation where the total pressure is a sum of both gas and radiation pressure.
Multiplying this last equation through by <math>~\Gamma_1 P r^4</math>, and recognizing that,
|
<math>~(r^4 \Gamma_1 P)\frac{d^2\xi}{dr^2} </math>
|
<math>~=</math>
|
<math>~
\frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr]
</math>
|
we can write,
|
<math>~0</math>
|
<math>~=</math>
|
<math>~
\frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr]
+ ( \Gamma_1 P r^4 ) \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr}
+\rho \biggl[ \sigma^2 r^4 + (4 - 3\Gamma_1) g_0 r^3\biggr] \xi
</math>
|
|
|
<math>~=</math>
|
<math>~
\frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \biggl[4r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr} \biggr] \frac{d\xi}{dr}
+ \biggl[ 4 r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr}\biggr] \frac{d\xi}{dr}
+\biggl[ \sigma^2 \rho r^4 - (4 - 3\Gamma_1) r^3 \frac{dP}{dr} \biggr] \xi
</math>
|
|
|
<math>~=</math>
|
<math>~
\frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr]
+\biggl[ \sigma^2 \rho r^4 + (3\Gamma_1 - 4) r^3 \frac{dP}{dr} \biggr] \xi
</math>
|
|
|
<math>~=</math>
|
<math>~
\frac{d}{dr}\biggl[ \Gamma_1 P r^4 ~\frac{d\xi}{dr} \biggr]
+\biggl[ \sigma^2 r^4 \rho + 4 Gm (r ) r \rho + 3\Gamma_1 r^3 \frac{dP}{dr} \biggr] \xi \, .
</math>
|
Assuming that <math>~\Gamma_1</math> is uniform throughout the configuration, this last expression is the same as equation (3) of Ledoux & Pekeris (1941), while the next-to-last expression is identical to equation (58.1) of Ledoux & Walraven (1958).
Stability Based on Variational Principle
Here we derive the Lagrangian directly from the governing LAWE. We begin with the next-to-last derived form of the LAWE that appears above in our review of the paper by Ledoux & Pekeris (1941) and, following the guidance provided at the top of p. 666 of S. Chandrasekhar (1964, ApJ, 139, 664), we multiply the LAWE through by the fractional displacement, <math>~\xi</math>. This gives, what we will henceforth refer to as, the,
| Foundational Variational Relation |
|
<math>~\sigma^2 \rho r^4 \xi^2</math>
|
<math>~=</math>
|
<math>~
-\xi \cdot \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr]
- (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) \, .
</math>
|
Chandrasekhar's Approach
Next, in an effort to adopt the notation used by Chandrasekhar (1964), we make the substitution, <math>~\xi \rightarrow \psi/r^3</math>, and regroup terms to obtain,
|
<math>~\frac{\sigma^2 \rho \psi^2}{r^2}</math>
|
<math>~=</math>
|
<math>~
- \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d}{dr} \biggl( \frac{\psi}{r^3} \biggr) \biggr]
- (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
</math>
|
|
|
<math>~=</math>
|
<math>~
- \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} -3 \Gamma_1 P \psi ~\biggr]
- (3\Gamma_1 - 4) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
</math>
|
|
|
<math>~=</math>
|
<math>~
(4-3\Gamma_1 ) \biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
- \biggl( \frac{\psi}{r^3}\biggr) \frac{d}{dr}\biggl[ r \Gamma_1 P ~\frac{d\psi}{dr} \biggr]
+ 3 \Gamma_1 \biggl( \frac{\psi^2}{r^3}\biggr) \frac{dP}{dr}
+3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
</math>
|
|
|
<math>~=</math>
|
<math>~
4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
+3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
- \biggl\{
\frac{d}{dr}\biggl[ r \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}\biggr] -r\Gamma_1 P ~\frac{d\psi}{dr} \cdot \frac{d}{dr}\biggl( \frac{\psi}{r^3}\biggr)
\biggr\}
</math>
|
|
|
<math>~=</math>
|
<math>~
4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
+3 \Gamma_1 P \biggl( \frac{\psi}{r^3}\biggr) \frac{d\psi}{dr}
+ \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2
- \biggl[\frac{3\Gamma_1 P\psi}{r^3}\biggr]\frac{d\psi}{dr}
- \frac{d}{dr}\biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr]
</math>
|
|
|
<math>~=</math>
|
<math>~
4\biggl( \frac{\psi^2}{r^3} \biggr) \biggl( \frac{dP}{dr} \biggr)
+ \frac{\Gamma_1 P}{r^2} \biggl[\frac{d\psi}{dr} \biggr]^2
- \frac{d}{dr}\biggl[ \frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr] \, .
</math>
|
Multiplying through by <math>~dr</math>, and integrating over the volume gives,
|
<math>~\int_0^R (\sigma^2 \rho \psi^2)\frac{dr}{r^2}</math>
|
<math>~=</math>
|
<math>~
\int_0^R \biggl[ \Gamma_1 P \biggl(\frac{d\psi}{dr} \biggr)^2
+ \frac{4\psi^2}{r} \biggl( \frac{dP}{dr} \biggr) \biggr]\frac{dr}{r^2}
- \biggl[\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr) \biggr]_0^R \, ,
</math>
|
which is identical to equation (49) of Chandrasekhar (1964), if the last term — the difference of the central and surface boundary conditions — is set to zero.
Note that if we shift from the variable, <math>~\psi</math>, back to the fractional displacement function, <math>~\xi</math>, the last term in this expression may be written as,
|
<math>~\frac{\Gamma_1 P \psi}{r^2} \biggl( \frac{d\psi}{dr} \biggr)</math>
|
<math>~=</math>
|
<math>~
\Gamma_1 P r \xi \frac{d}{dr} \biggl[r^3 \xi\biggr]
</math>
|
|
|
<math>~=</math>
|
<math>~
\Gamma_1 P r \xi \biggl[3r^2 \xi + r^3 \frac{d\xi}{dr}\biggr]
</math>
|
|
|
<math>~=</math>
|
<math>~
\Gamma_1 P r^3 \xi^2 \biggl[3 + \frac{d\ln\xi}{d\ln r}\biggr] \, .
</math>
|
So, as is pointed out by Ledoux & Walraven (1958) in connection with their equation (57.31), setting this expression to zero at the surface of the configuration is equivalent to setting the variation of the pressure to zero at the surface. Quite generally, this can be accomplished by demanding that,
<math>~\frac{d\ln\xi}{d\ln r}\biggr|_\mathrm{surface} = -3 \, .</math>
Ledoux & Walraven Approach
Returning to the above Foundational Variational Relation, we can also write,
|
<math>~\sigma^2 \rho r^4 \xi^2</math>
|
<math>~=</math>
|
<math>~
-\xi \cdot \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr]
- (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr)
</math>
|
|
|
<math>~=</math>
|
<math>~
r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2
- (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr)
- \frac{d}{dr}\biggr[r^4 \Gamma_1 P\xi \biggl(\frac{d\xi}{dr}\biggr) \biggr]
</math>
|
|
<math>~\Rightarrow ~~~ \int_0^R\sigma^2 \rho r^4 \xi^2 dr</math>
|
<math>~=</math>
|
<math>~
\int_0^R r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 dr
- \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) dr
- \biggr[r^4 \Gamma_1 P\xi \biggl(\frac{d\xi}{dr}\biggr) \biggr]_0^R
</math>
|
If the last term (boundary conditions) is set to zero, then we may also write,
|
<math>~\sigma^2 </math>
|
<math>~=</math>
|
<math>~
\frac{\int_0^R r^4 \Gamma_1 P \bigl(\frac{d\xi}{dr}\bigr)^2 dr
- \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \bigl( \frac{dP}{dr} \bigr) dr}{\int_0^R \rho r^4 \xi^2 dr} \, .
</math>
|
This means that, if the radial profile of the pressure and the density is known throughout a spherically symmetric, equilibrium configuration, and if, furthermore, the eigenfunction, <math>~\xi(r)</math>, of a radial oscillation mode is specified precisely, then this expression will give the (square of the) eigenfrequency of that oscillation mode.
By using formal variational principle techniques to derive this same expression, Ledoux & Walraven (1958) are able to offer a broader interpretation, which is encapsulated by their equation (59.10), viz.,
|
<math>~\sigma_0^2 </math>
|
<math>~=</math>
|
<math>~\mathrm{min}~
\frac{\int_0^R r^4 \Gamma_1 P \bigl(\frac{d\xi}{dr}\bigr)^2 dr
- \int_0^R (3\Gamma_1 - 4) r^3 \xi^2 \bigl( \frac{dP}{dr} \bigr) dr}{\int_0^R \rho r^4 \xi^2 dr} \, .
</math>
|
This means that, if the exact radial eigenfunction, <math>~\xi(r)</math>, is not known, various approximate eigenfunctions can be tried. The trial eigenfunction that minimizes the righthand-side of this expression will give the (square of the) eigenfrequency of the fundamental mode of oscillation (subscript zero). Furthermore, via an evaluation of this righthand-side expression, any reasonable trial eigenfunction — for example, <math>~\xi</math> = constant — can provide an upper limit to <math>~\sigma_0^2</math>.
Ledoux & Pekeris Approach
Here we follow the lead of Ledoux & Pekeris (1941). Returning to the integral expression just derived in our discussion of the Ledoux & Walraven approach, and multiplying through by <math>~4\pi</math>, we have,
|
<math>~\int_0^R 4\pi \sigma^2 \rho r^4 \xi^2 dr</math>
|
<math>~=</math>
|
<math>~
\int_0^R 4\pi r^4 \Gamma_1 P \biggl(\frac{d\xi}{dr}\biggr)^2 dr
- \int_0^R (3\Gamma_1 - 4) 4\pi r^3 \xi^2 \biggl( \frac{dP}{dr} \biggr) dr
- \biggr[4\pi r^3 \Gamma_1 P\xi^2 \biggl(\frac{d\ln \xi}{d\ln r}\biggr) \biggr]_0^R \, .
</math>
|
If we acknowledge that:
- at the center of the configuration, <math>~r^3 = 0</math>;
- as above, the boundary condition at the surface is <math>~P = P_e</math> while <math>~(d\ln \xi/d\ln r) = -3</math>;
- the differential mass element is, <math>~dm = 4\pi r^2 \rho dr</math> and the corresponding differential volume element is, <math>~dV = 4\pi r^2 dr</math>; and
- a statement of detailed force balance is, <math>~dP/dr = - Gm\rho/r^2</math>,
this integral relation becomes,
|
<math>~ \sigma^2 \int_0^R r^2 \xi^2 dm</math>
|
<math>~=</math>
|
<math>~
\Gamma_1 \int_0^R \biggl[ r \biggl(\frac{d\xi}{dr}\biggr)\biggr]^2 P dV
+ (3\Gamma_1 - 4) \int_0^R \xi^2 \biggl( \frac{Gm}{r} \biggr) dm
- \biggr[\Gamma_1 \xi_\mathrm{surface}^2 (3P_e V) \biggl(-3\biggr) \biggr] \, .
</math>
|
Now, as we have discussed separately — see, also, p. 64, Equation (12) of [C67] — the gravitational potential energy of the unperturbed configuration is given by the integral,
|
<math>~W_\mathrm{grav}</math>
|
<math>~=</math>
|
<math>~ - \int_0^{M} \biggl( \frac{Gm}{r_0} \biggr) dm \, ;</math>
|
for adiabatic systems, the internal energy is,
<math>
U_\mathrm{int}
= \frac{1}{(\Gamma_1-1)} \int_0^R P_0 dV
\, ;</math>
and — see the text at the top of p. 126 of Ledoux & Pekeris (1941) — the moment of inertia of the configuration about its center is,
<math>
I = \int_0^M r_0^2 dm
\, .</math>
Hence, the integral relation may be written in the form,
|
<math>~ \sigma^2 \int_0^R \xi^2 dI</math>
|
<math>~=</math>
|
<math>~
\Gamma_1 (\Gamma_1 - 1) \int_0^R \xi^2 \biggl[ \frac{d\ln\xi}{d\ln r}\biggr]^2 dU_\mathrm{int}
- (3\Gamma_1 - 4) \int_0^R \xi^2 dW_\mathrm{grav}
+ 3^2 \Gamma_1 P_e V \xi_\mathrm{surface}^2 \, .
</math>
|
Free-Energy Analysis
If we assume the simplest approximation for the fundamental-mode eigenfunction, namely, <math>~\xi = \xi_0</math> = constant, then this last integral expression gives,
|
<math>~ \sigma^2 I</math>
|
<math>~=</math>
|
<math>~
(4 - 3\Gamma_1) W_\mathrm{grav}
+ 3^2 \Gamma_1 P_e V \, .
</math>
|
Contrast this result with the following free-energy analysis:
|
<math>~\mathfrak{G}</math>
|
<math>~=</math>
|
<math>~W_\mathrm{grav} + U_\mathrm{int} + P_eV \, ,</math>
|
where,
|
<math>~W_\mathrm{grav}</math>
|
<math>~=</math>
|
<math>~-a\chi^{-1}</math>
|
|
<math>~U_\mathrm{int}</math>
|
<math>~=</math>
|
<math>~b\chi^{3-3\Gamma_1}</math>
|
|
<math>~V</math>
|
<math>~=</math>
|
<math>~\frac{4\pi}{3} \chi^3 \, .</math>
|
Then,
|
<math>~\frac{\partial \mathfrak{G}}{\partial \chi}</math>
|
<math>~=</math>
|
<math>~+a \chi^{-2} + 3(1-\Gamma_1) b \chi^{2-3\Gamma_1} + 4\pi P_e \chi^{2} </math>
|
|
|
<math>~=</math>
|
<math>~\chi^{-1} \biggl[- W_\mathrm{grav} + 3(1-\Gamma_1) U_\mathrm{int} + 3 P_e V \biggr] \, ,</math>
|
and,
|
<math>~\frac{\partial^2 \mathfrak{G}}{\partial \chi^2}</math>
|
<math>~=</math>
|
<math>~-2a \chi^{-3} + 3(1-\Gamma_1)(2-3\Gamma_1) b \chi^{1-3\Gamma_1} + 8\pi P_e \chi </math>
|
|
|
<math>~=</math>
|
<math>~\chi^{-2} \biggl[ 2W_\mathrm{grav} + 3(1-\Gamma_1)(2-3\Gamma_1) U_\mathrm{int}+ 6 P_e V \biggr] \, .</math>
|
The equilibrium condition occurs when <math>~\partial \mathfrak{G}/\partial \chi = 0</math>, that is, when,
|
<math>~3(1-\Gamma_1) U_\mathrm{int}</math>
|
<math>~=</math>
|
<math>~W_\mathrm{grav} - 3 P_e V \, ,</math>
|
in which case,
|
<math>~\chi^2 \cdot \frac{\partial^2 \mathfrak{G}}{\partial \chi^2}</math>
|
<math>~=</math>
|
<math>~2W_\mathrm{grav} + (2-3\Gamma_1) (W_\mathrm{grav} - 3P_eV) + 6 P_e V </math>
|
|
|
<math>~=</math>
|
<math>~(4-3\Gamma_1)W_\mathrm{grav} + 3^2 \Gamma_1 P_e V \, .</math>
|
Amazing! The righthand-sides of the two expressions are exactly the same, so we can make the association,
<math>~\sigma^2 I = \chi^2 \cdot \frac{\partial^2 \mathfrak{G}}{\partial \chi^2} \, .</math>
This also make sense in that the equilibrium configuration should be stable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} > 0</math> — in which case, <math>~\sigma^2</math> is positive; whereas the equilibrium configuration should be unstable if <math>~\tfrac{\partial^2 \mathfrak{G}}{\partial \chi^2} < 0</math> — in which case, <math>~\sigma^2</math> is negative.
See Also