User:Tohline/ThreeDimensionalConfigurations/RiemannStype
Riemann S-type Ellipsoids

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
General Coefficient Expressions
As has been detailed in an accompanying chapter, the gravitational potential anywhere inside or on the surface, <math>~(a_1,a_2,a_3) ~\leftrightarrow~(a,b,c)</math>, of an homogeneous ellipsoid may be given analytically in terms of the following three coefficient expressions:
|
<math> ~A_1 </math> |
<math> ~= </math> |
<math>~2\biggl(\frac{b}{a}\biggr)\biggl(\frac{c}{a}\biggr) \biggl[ \frac{F(\theta,k) - E(\theta,k)}{k^2 \sin^3\theta} \biggr] \, , </math> |
|
<math> ~A_3 </math> |
<math> ~= </math> |
<math> ~2\biggl(\frac{b}{a}\biggr) \biggl[ \frac{(b/a) \sin\theta - (c/a)E(\theta,k)}{(1-k^2) \sin^3\theta} \biggr] \, , </math> |
|
<math> ~A_2 </math> |
<math> ~= </math> |
<math>~2 - (A_1+A_3) \, ,</math> |
where, <math>~F(\theta,k)</math> and <math>~E(\theta,k)</math> are incomplete elliptic integrals of the first and second kind, respectively, with arguments,
|
<math>~\theta = \cos^{-1} \biggl(\frac{c}{a} \biggr)</math> |
and |
<math>~k = \biggl[\frac{1 - (b/a)^2}{1 - (c/a)^2} \biggr]^{1/2} \, .</math> |
| [ EFE, Chapter 3, §17, Eq. (32) ] | ||
| TEST (part 1) Notation: Use <math>~\phi</math> in place of <math>~\theta</math>. |
||||||||||
| <math>~\frac{b}{a}</math> | <math>~\frac{c}{a}</math> | <math>~\phi</math> | <math>~k</math> | Numerical Recipes | <math>~A_1</math> | <math>~A_2</math> | <math>~A_3</math> | |||
| (deg) | (rad) | (deg) | (rad) | <math>~F(\phi,k) </math> | <math>~E(\phi,k) </math> | |||||
| 0.9 | 0.641 | 50.13357253 | 0.874995907 | 32.53852919 | 0.567904468 | 0.909025949 | 0.843118048 | 0.521450273 | 0.595131012 | 0.883418715 |
Equilibrium Conditions for Riemann S-type Ellipsoids
Pulling from Chapter 7 — specifically, §48 — of Chandrasekhar's EFE, we understand that the semi-axis ratios, <math>~(\tfrac{b}{a}, \tfrac{c}{a})</math> associated with Riemann S-type ellipsoids are given by the roots of the equation,
|
<math>~ \biggl[ \frac{a^2 b^2}{a^2 + b^2} \biggr] f \biggl( \frac{\Omega^2}{\pi G \rho} \biggr) </math> |
<math>~=</math> |
<math>~a^2 b^2 A_{12} - c^2 A_3 \, ,</math> |
| [ EFE, §48, Eq. (34) ] | ||
and the associated value of the square of the equilibrium configuration's angular velocity is,
|
<math>~\biggl[ 1 + \frac{a^2 b^2 \cdot f^2}{(a^2 + b^2)^2} \biggr] \frac{\Omega^2}{\pi G \rho}</math> |
<math>~=</math> |
<math>~2B_{12} \, ,</math> |
| [ EFE, §48, Eq. (33) ] | ||
where,
|
<math>~A_{12}</math> |
<math>~\equiv</math> |
<math>~-\frac{A_1-A_2}{(a^2 - b^2)} \, ,</math> |
| [ EFE, §21, Eq. (107) ] | ||
|
<math>~B_{12}</math> |
<math>~\equiv</math> |
<math>~A_2 - a^2A_{12} \, .</math> |
| [ EFE, §21, Eq. (105) ] | ||
(Notice that if we set <math>~f \rightarrow 0</math>, this pair of expressions simplifies to the pair we have provided in a separate discussion of the equilibrium conditions for Jacobi ellipsoids.) Following Chandrasekhar's lead and eliminating <math>~\Omega^2</math> between these two expressions, we obtain,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{a^2 b^2}{(a^2 + b^2)^2} \biggr] f^2 + \biggl[ \frac{2a^2 b^2 B_{12}}{c^2 A_3 - a^2 b^2 A_{12}} \biggr]\frac{f}{a^2 + b^2} + 1 \, . </math> |
| [ EFE, §48, Eq. (35) ] | ||
For a given <math>~f</math>, this last expression determines the ratios of the axes of the ellipsoids that are compatible with equilibrium; and the value of <math>~\Omega^2</math>, that is to be associated with a particular solution of this last expression, then follows from either one of the first two expressions. For convenience of evaluation and for greater clarity, let's rewrite this last (quadratic) equation in the form,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \alpha f^2 + \beta f + 1 \, , </math> |
in which case the pair of solutions is,
|
<math>~f</math> |
<math>~=</math> |
<math>~ \frac{1}{2\alpha}\biggr\{ - \beta \pm \biggl[ \beta^2 - 4\alpha \biggr]^{1 / 2} \biggr\} \, ; </math> |
and the corresponding values of the angular velocity (in units of [G ρ]½) are provided by the expression,
|
<math>~\omega \equiv \frac{\Omega}{\sqrt{G\rho}}</math> |
<math>~=</math> |
<math>~ \pm \biggl\{ 2 \pi B_{12} \biggl[ 1 + \alpha f^2 \biggr]^{-1} \biggr\}^{1 / 2} </math> |
| TEST (part 2) | |||||||||
| <math>~\frac{b}{a}</math> | <math>~\frac{c}{a}</math> | <math>~a^2 A_{12}</math> | <math>~ B_{12}</math> | <math>~\alpha \equiv \frac{(b/a)^2}{[ 1 + (b/a)^2]^2} </math> | <math>~\beta \equiv \biggl[ \frac{2 B_{12}}{(c/b)^2 A_3 - a^2 A_{12}} \biggr]\frac{1}{1 + (b/a)^2} </math> | Direct | Adjoint | ||
| <math>~f </math> | <math>~\omega = \frac{\Omega}{\sqrt{G\rho}} </math> | <math>~f^\dagger </math> | <math>~\omega^\dagger = \frac{\Omega^\dagger}{\sqrt{G\rho}} </math> | ||||||
| 0.9 | 0.641 | 0.387793362 | 0.207337649 | 0.247245200 | 3.797483556 | - 0.268008879 | ± 1.131374734 | -15.09117122 | ± 0.150771618 |
Now, according to Ou (2006), at any coordinate position inside or on the surface of the ellipsoid, <math>~(x, y)</math>, the three components of the velocity as viewed from a frame of rotation that is spinning at the equilibrium configuration's frequency, <math>~\Omega</math>, are,
|
<math>~\vec{v}</math> |
<math>~=</math> |
<math>~\lambda \biggl( \frac{ay}{b} , - \frac{bx}{a} , 0 \biggr) \, ,</math> |
where, <math>~\lambda</math> is an overall scale factor. But, according to §48 of EFE, we see that,
|
<math>~\vec{u}</math> |
<math>~=</math> |
<math>~\biggl( Q_1 y , Q_2 x , 0 \biggr) \, ,</math> |
where,
|
<math>~Q_1</math> |
<math>~\equiv</math> |
<math>~- \biggl[ \frac{a^2}{a^2 + b^2} \biggr]\zeta</math> |
and, |
<math>~Q_2</math> |
<math>~\equiv</math> |
<math>~+ \biggl[ \frac{b^2}{a^2 + b^2} \biggr]\zeta \, ,</math> |
and <math>~\zeta</math> is the scalar magnitude of the vorticity vector, <math>~\vec\zeta</math>. The transformation from EFE's notation to the one used by Ou is, then,
|
<math>~\lambda \biggl( \frac{a}{b} \biggr) </math> |
<math>~=</math> |
<math>~- \biggl[ \frac{a^2}{a^2 + b^2} \biggr]\zeta</math> |
and, |
<math>~- \lambda \biggl( \frac{b}{a} \biggr) </math> |
<math>~=</math> |
<math>~+ \biggl[ \frac{b^2}{a^2 + b^2} \biggr]\zeta </math> |
|
<math>~\Rightarrow ~~~ \lambda </math> |
<math>~=</math> |
<math>~- \biggl[ \frac{a b}{a^2 + b^2} \biggr]\zeta = - \biggl[ \frac{b}{a} + \frac{a}{b} \biggr]^{-1} \zeta\, ,</math> |
which, gratifyingly agrees with Ou's equation (17).
Models Examined by Ou (2006)
His Tabulated Model Parameters
Table 1 (see below) lists a subset of the Riemann S-type ellipsoids that were studied by Ou (2006); properties of various so-called Direct configurations can be found in Ou's Table 1, while properties of various Adjoint configurations can be found in his Table 5. Each row of our Table 1 was constructed as follows:
- The pair of axis ratios <math>~(\tfrac{b}{a}, \tfrac{c}{a} )</math> associated with one of Ou's (2006) uniform-density, incompressible <math>~(n=0)</math> ellipsoid models (columns 1 and 2 from Ou's Table 1) has been copied into columns 1 and 2 of our table.
- Properties of Direct Configurations …
- The pair of parameter values <math>~(\omega_\mathrm{analytic}, \lambda_\mathrm{analytic})</math> that is required in order for this to be an equilibrium configuration — as specified by the above set of analytical expressions from EFE — is copied from, respectively, columns 11 and 13 of Ou's Table 1 into columns 3 and 4 of our table; in our table, the "analytic" subscript has been dropped from the column headings.
- The value of the equilibrium configuration's vorticity, <math>~\zeta</math> — see column 5 of our table — has been determined from the expression,
<math>~\zeta = - \biggl[ \frac{1 + (b/a)^2}{b/a} \biggr] \lambda \, .</math> - Column 6 of our table lists the value of the frequency ratio, <math>~f \equiv \zeta/\omega</math>.
- Properties of Adjoint Configurations [in order to distinguish from Direct configuration properties, a superscript † has been attached to each parameter name] …
- As listed in column 7 of our Table, the "spin" angular velocity of the adjoint equilibrium configuration has been determined from the vorticity of the direct configuration via the relation,
<math>~\omega^\dagger = \zeta \biggl[\frac{b/a}{1 + (b/a)^2}\biggr] \, .</math> - As listed in column 10 of our Table, the ratio <math>~(f^\dagger)</math> of the vorticity to the angular velocity in the adjoint equilibrium configuration has been determined from the same ratio <math>~(f)</math> in the direct configuration via the relation,
<math>~f^\dagger = \frac{1}{f} \biggl\{ \frac{[1 + (b/a)^2]^2}{(b/a)^2} \biggr\} \, .</math> - As indicated, the value of the vorticity in the adjoint equilibrium configuration (column 9 of our table) has been determined from a product of <math>~\omega^\dagger</math> and <math>~f^\dagger</math>.
- As listed in column 8 of our table, the value of the parameter, <math>~\lambda^\dagger</math>, has been determined from the vorticity in the adjoint equilibrium configuration via the relation,
<math>~\lambda^\dagger = -~ \zeta^\dagger \biggl[ \frac{b}{a} + \frac{a}{b}\biggr]^{-1} \, .</math>
|
Table 1: Example Riemann S-type Ellipsoids |
|||||||||
| <math>~\frac{b}{a}</math> | <math>~\frac{c}{a}</math> |
Properties of |
Properties of |
||||||
| <math>~\omega = \frac{\Omega}{\sqrt{G \rho}}</math> | <math>~\lambda</math> | <math>~\zeta </math> | <math>~f \equiv \frac{\zeta}{\omega}</math> | <math>~\omega^\dagger </math> | <math>~\lambda^\dagger </math> | <math>~\zeta^\dagger = \omega^\dagger f^\dagger</math> | <math>~f^\dagger </math> | ||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) |
| 0.90 | 0.795 | 1.14704 | 0.43181 | -0.86842 | -0.75709 | -0.43181 | -1.14704 | +2.30682 | -5.3422 |
| 0.641 | 1.13137 | 0.15077 | - 0.30322 | - 0.26801 | - 0.15077 | -1.13137 | 2.27531 | - 15.0913 | |
| 0.590 | 1.10661 | 0.06406 | -0.12883 | -0.11642 | -0.06406 | -1.10661 | +2.22552 | -34.7411 | |
| 0.564 | 1.09034 | 0.02033 | -0.04089 | -0.03750 | -0.02033 | -1.09034 | +2.19279 | -107.86 | |
| 0.538 | 1.07148 | - 0.02324 | +0.04674 | +0.04362 | +0.02324 | - 1.07148 | +2.15487 | +92.722 | |
| 0.487 | 1.02639 | - 0.10880 | +0.21881 | +0.21318 | +0.10880 | -1.02639 | +2.06418 | +18.972 | |
| 0.333 | 0.79257 | - 0.39224 | +0.78884 | +0.99529 | +0.39224 | -0.79257 | +1.59395 | +4.06370 | |
| 0.28 | 0.256 | 0.80944 | 0.03668 | -0.14127 | -0.17453 | -0.03668 | -0.80944 | +3.11750 | -84.992 |
| 0.245083 | 0.796512a | 0.0 | 0.0 | 0.0 | 0.0 | … | … | <math>~\infty</math> | |
| 0.231 | 0.77651 | - 0.04714 | +0.18156 | +0.23381 | +0.04714 | -0.77651 | +2.99067 | +63.442 | |
| 0.205 | 0.72853 | - 0.13511 | +0.52037 | +0.71427 | +0.13511 | -0.72853 | +2.80588 | +20.7674 | |
|
aAccording to Table IV (p. 103) of EFE, the square of the angular velocity of this Jacobi ellipsoid is, <math>~\Omega^2/(\pi G\rho) = 0.201946</math>; from this value, we find that, <math>~\omega = \sqrt{\pi} \cdot \sqrt{0.201946} = 0.796512</math>. |
|||||||||
Our Parameter Determinations
The parameter values that have been posted above in our Table 1 are typically given with five digits of precision. This is because, as explained, the values were determined from the analytically determined values, <math>~\omega_\mathrm{analytic}</math> and <math>~\lambda_\mathrm{analytic}</math>, that were provided by Ou (2006) with only five digit accuracy. Our Table 2 (shown immediately below) provides values of this same set of model parameters to better than eleven digits accuracy. We calculated these parameter values by following the steps detailed in earlier subsections of this chapter and, as a foundation, using double-precision versions of Numerical Recipes algorithms to evaluate the special functions, <math>~F(\phi,k)</math> and <math>~E(\phi,k)</math>. As an example, the above pair of brief tables titled, TEST (part 1) and TEST (part 2) detail all of the intermediate steps that were used in order to determine the high-precision parameter values specifically for the model having the axis-ratio pair <math>~(0.9,0.641)</math>. This table of higher precision parameter values was primarily generated in order to convince ourselves that we understood from first principles how to accurately determine the properties of Riemann S-type ellipsoids; the lower-precision parameter values that we derived from Ou's work provided a handy means of cross-checking these "first principles" determinations.
In generating our Table 2, we wondered what the approriate signs were of the various model parameters — especially when part of our objective is to distinguish between direct and adjunct configurations. We took the following approach: First we decided that the spin frequency of every direct configuration should be positive. (Evidently, Ou made this same choice.)
|
Table 2: Example Riemann S-type Ellipsoids (double-precision evaluation) |
|||||||||
| <math>~\frac{b}{a}</math> | <math>~\frac{c}{a}</math> |
Properties of |
Properties of |
||||||
| <math>~\omega = \frac{\Omega}{\sqrt{G \rho}}</math> | <math>~\lambda</math> | <math>~\zeta </math> | <math>~f \equiv \frac{\zeta}{\Omega}</math> | <math>~\omega^\dagger </math> | <math>~\lambda^\dagger </math> | <math>~\zeta^\dagger = \omega^\dagger f^\dagger</math> | <math>~f^\dagger </math> | ||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) |
| 0.90 | 0.795 | +1.147036091720 | +0.431809451699 | -0.868416786194 | -0.757096320116 | -0.431809460593 | -1.147036104571 | +2.306817054749 | -5.342210487323 |
| 0.641 | +1.131374738327 | +0.150771621841 | -0.303218483925 | -0.268008886644 | -0.150771621877 | -1.131374730590 | +2.275320291519 | -15.091170863305 | |
| 0.590 | +1.106612583610 | +0.064060198174 | -0.128832176328 | -0.116420305902 | -0.064060197762 | -1.106612576964 | +2.225520849228 | -34.741086358509 | |
| 0.564 | +1.090339840378 | +0.020334563779 | -0.040895067155 | -0.037506716440 | -0.020334563809 | -1.090339837153 | +2.192794561386 | -107.8358300897 | |
| 0.538 | +1.071485625744 | -0.023236834336 | +0.046731855720 | +0.043614077664 | +0.023236835120 | -1.071485656401 | +2.154876708984 | +92.735376233270 | |
| 0.487 | +1.026387311947 | -0.108799837242 | +0.218808561563 | +0.213183225210 | +0.108799835209 | -1.026387320039 | +2.064178943634 | +18.972261524065 | |
| 0.333 | +0.792566980901 | -0.392440787995 | +0.789242029190 | +0.995804846843 | +0.392440793882 | -0.792566979129 | +1.593940258026 | +4.061606964516 | |
| 0.74 | 0.692 | +1.132149 | +0.385992 | -0.385992 | -1.132149 | ||||
| 0.41 | 0.385 | +0.971082 | +0.141594 | -0.141594 | -0.971082 | ||||
| 0.333 | +0.929630138695 | +0.003311666790 | -0.009435019456 | -0.010149218281 | -0.003311666699 | -0.929630099681 | +2.648538827896 | -799.7601146950 | |
| 0.28 | 0.256 | +0.809436834686 | +0.036676037913 | -0.141255140305 | -0.174510396110 | -0.036676038521 | -0.809436833116 | +3.117488145828 | -85.000678306244 |
| 0.245083 | 0.796512a | 0.0 | 0.0 | 0.0 | 0.0 | … | … | <math>~\infty</math> | |
| 0.231 | +0.776514825339 | -0.047142035397 | +0.181564182043 | +0.233819345828 | +0.047142037070 | -0.776514835457 | +2.990691423416 | +63.440011724689 | |
| 0.205 | +0.728526018042 | -0.135108121071 | +0.520359277725 | +0.714263156392 | +0.135108125079 | -0.728526039364 | +2.805866003036 | +20.767558718483 | |
|
aAccording to Table IV (p. 103) of EFE, the square of the angular velocity of this Jacobi ellipsoid is, <math>~\Omega^2/(\pi G\rho) = 0.201946</math>; from this value, we find that, <math>~\omega = \sqrt{\pi} \cdot \sqrt{0.201946} = 0.796512</math>. |
|||||||||
Feeding a 3D Animation
Initial Thoughts
Let's examine the elliptical trajectory of a Lagrangian particle that is moving in the equatorial plane of a Riemann S-Type ellipsoid. As viewed in a frame that is spinning about the Z-axis at angular frequency, <math>~\Omega</math>, the trajectory is defined by,
|
<math> r^2 </math> |
<math> ~= </math> |
<math>~ \biggl(\frac{x}{a} \biggr)^2 + \biggl(\frac{y}{b}\biggr)^2 \, , </math> |
where <math>~0 < r \le 1</math>. (The surface of the relevant ellipsoid is associated with the value, <math>~r=1</math>.)
Let's choose a pair of axis ratios — for example, <math>~b/a = 0.28</math> and <math>~c/a = 0.231</math> — then, from Table 1 of our above discussion, draw the associated value of either <math>~\lambda</math> or <math>~\zeta</math> that corresponds to the Jacobi-like equilibrium configuration — in this example, <math>~\lambda = -0.04714</math> and <math>~\zeta = +0.18156</math>. Then, for any point <math>~(x,y)</math> inside of the ellipsoid, the fluid's velocity components (as viewed from the rotating frame of reference) are,
|
<math> v_x = \frac{dx}{dt} = \lambda \biggl( \frac{ay}{b} \biggr) = -0.16836 ~y </math> |
and, |
<math>~ v_y = \frac{dy}{dt} = - \lambda \biggl( \frac{bx}{a} \biggr) = + 0.01320~x \, . </math> |
Alternatively, we have,
|
<math> u_x = \frac{dx}{dt} = Q_1 y = - \biggl[ 1 + \frac{b^2}{a^2} \biggr]^{-1}\zeta ~y = -0.16836 ~y </math> |
and, |
<math>~ u_y = \frac{dy}{dt}= Q_2 x = + \biggl[ 1 + \frac{a^2}{b^2} \biggr]^{-1}\zeta ~x = + 0.01320~x \, . </math> |
Now, each Lagrangian fluid element's motion is oscillatory in both the <math>~x</math> and <math>~y</math> coordinate directions. So let's see how this plays out. Suppose,
|
<math> x = x_\mathrm{max} \cos(\varphi t) </math> |
and, |
<math>~ y = y_\mathrm{max} \sin(\varphi t) \, . </math> |
Then,
|
<math> \frac{dx}{dt} = - x_\mathrm{max}\varphi \sin(\varphi t) = - \biggl( \frac{x_\mathrm{max}}{y_\mathrm{max}}\biggr) \varphi y = - \varphi \biggl(\frac{ay}{b}\biggr) </math> |
and, |
<math>~ \frac{dy}{dt} = y_\mathrm{max} \varphi \cos(\varphi t) = + \biggl( \frac{y_\mathrm{max}}{x_\mathrm{max}}\biggr) \varphi x = + \varphi \biggl(\frac{bx}{a}\biggr) \, . </math> |
Hence our functional representation of the time-dependent behavior of both <math>~x</math> and <math>~y</math> works perfectly if, for each orbit inside of or on the surface of the configuration, we set <math>~\varphi = - \lambda</math> and if the ratio <math>~y_\mathrm{max}/x_\mathrm{max} = (b/a)</math>. Hooray!
Preferred Normalizations
Let's do this again, assuming that <math>~x</math> and <math>~y</math> both have units of length and that <math>~t</math> has the unit of time. Then, let's use <math>~a</math> to normalize lengths and use <math>~(\pi G \rho)^{-1 / 2}</math> to normalize time. We therefore have,
|
<math> \frac{x}{a} = \biggl(\frac{ x_\mathrm{max} }{a}\biggr) \cos\biggl[ \frac{\varphi}{(\pi G \rho)^{1 / 2}} \cdot \frac{t}{(\pi G \rho)^{-1 / 2}} \biggr] </math> |
and, |
<math>~ \frac{y}{a} = \biggl(\frac{ y_\mathrm{max} }{a}\biggr) \sin\biggl[ \frac{\varphi}{(\pi G \rho)^{1 / 2}} \cdot \frac{t}{(\pi G \rho)^{-1 / 2}} \biggr] \, . </math> |
|
NOTE: When implementing in an xml-based COLLADA (3D animation) file, we associate <math>~\mathrm{TIME} = 4</math> with <math>~t \cdot (\pi G \rho)^{1 / 2} = 2\pi</math>. Hence we can everywhere replace <math>~t \cdot (\pi G \rho)^{1 / 2}</math> with (in radians) <math>~(\pi/2)\cdot \mathrm{TIME}</math> or (in degrees) <math>~90 \cdot \mathrm{TIME}</math>.
|
Next, let's normalize the velocities such that <math>~\rho</math> and the total mass, <math>~M</math>, are both assumed to be the same in every examined Riemann ellipsoid. In particular, we will normalize to,
|
<math>~v_0</math> |
<math>~\equiv</math> |
<math>~(abc)^{1 / 3}(\pi G \rho)^{1 / 2}</math> |
<math>~=</math> |
<math>~a(\pi G \rho)^{1 / 2} \biggl( \frac{b}{a}\cdot \frac{c}{a} \biggr)^{1 / 3} \, ,</math> |
in which case we have,
|
<math> \frac{1}{v_0} \cdot \frac{dx}{dt} = - \frac{\varphi}{(\pi G \rho)^{1 / 2} } \biggl(\frac{a}{b}\biggr)\biggl( \frac{b}{a}\cdot \frac{c}{a} \biggr)^{-1 / 3} \cdot \biggl(\frac{y}{a}\biggr) </math> |
and, |
<math>~ \frac{1}{v_0} \cdot \frac{dy}{dt} = + \frac{\varphi}{(\pi G \rho)^{1 / 2} } \biggl(\frac{b}{a}\biggr) \biggl( \frac{b}{a}\cdot \frac{c}{a} \biggr)^{-1 / 3} \cdot \biggl(\frac{x}{a}\biggr) \, . </math> |
Finally, setting, <math>~\varphi/(\pi G\rho)^{1 / 2} \rightarrow -\lambda_\mathrm{EFE}</math> means,
|
<math> V_x \equiv \frac{1}{v_0} \cdot \frac{dx}{dt} = \lambda_\mathrm{EFE} \biggl(\frac{a}{b}\biggr)\biggl( \frac{b}{a}\cdot \frac{c}{a} \biggr)^{-1 / 3} \cdot \biggl(\frac{y}{a}\biggr) </math> |
and, |
<math>~ V_y \equiv \frac{1}{v_0} \cdot \frac{dy}{dt} = - \lambda_\mathrm{EFE} \biggl(\frac{b}{a}\biggr) \biggl( \frac{b}{a}\cdot \frac{c}{a} \biggr)^{-1 / 3} \cdot \biggl(\frac{x}{a}\biggr) \, . </math> |
Example Mach Surface
Let's try to plot the "Mach surface" for the example model, b41c385, referenced below. Its relevant parameter values are,
- <math>~b/a = 0.41</math>
- <math>~c/a = 0.385</math>
- <math>~\lambda_\mathrm{EFE} = 0.079886</math>
Hence, if we set a = 1 then we have,
| <math>~V_x</math> | <math>=</math> | <math>~(0.079886) \biggl(\frac{1}{0.41}\biggr)\biggl( 1.85034 \biggr) \cdot y</math> | and | <math>~V_y</math> | <math>=</math> | <math>~- (0.079886) \biggl(0.41\biggr) \biggl( 1.85034 \biggr) \cdot x</math> |
| <math>=</math> | <math>~0.3605 y</math> | <math>=</math> | <math>~- 0.0606 x</math> |
Borrowing from an accompanying discussion, we have the following example data set.
| Direct | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| n | Axisymmetric | b41c385 | Surface | |V| = 0.1 | ||||||
| x0 | y0 | y = 0.41 × y0 | Vx | Vy | |V| | factor | x | y | ||
| 1 | 1.0000 | 0.0000 | 0.0000 | 0.0000 | -0.0606 | 0.0606 | --- | --- | --- | |
| 2 | 0.9921 | -0.1253 | -0.0514 | -0.0185 | -0.0601 | 0.0629 | --- | --- | --- | |
| 3 | 0.9686 | -0.2487 | -0.1020 | -0.0368 | -0.0587 | 0.0693 | --- | --- | --- | |
| 4 | 0.9298 | -0.3681 | -0.1509 | -0.0544 | -0.0563 | 0.0783 | --- | --- | --- | |
| 5 | 0.8763 | -0.4818 | -0.1975 | -0.0712 | -0.0531 | 0.0888 | --- | --- | --- | |
| 6 | 0.8090 | -0.5878 | -0.2410 | -0.0869 | -0.0490 | 0.0998 | 1.002 | 0.8090 | -0.2410 | |
| 7 | 0.7290 | -0.6845 | -0.2807 | -0.1012 | -0.0442 | 0.1104 | 0.9058 | 0.6603 | -0.2543 | |
| 8 | 0.6374 | -0.7705 | -0.3159 | -0.1139 | -0.0386 | 0.1203 | 0.8313 | 0.5298 | -0.2626 | |
| 9 | 0.5358 | -0.8443 | -0.3462 | -0.1248 | -0.0325 | 0.1290 | 0.7752 | 0.4153 | -0.2684 | |
| 10 | 0.4258 | -0.9048 | -0.3710 | -0.1337 | -0.0258 | 0.1362 | 0.7342 | 0.3126 | -0.2724 | |
| 11 | 0.3090 | -0.9511 | -0.3899 | -0.1406 | -0.0187 | 0.1418 | 0.7052 | 0.2179 | -0.2750 | |
| 12 | 0.1874 | -0.9823 | -0.4027 | -0.1452 | -0.0114 | 0.1456 | 0.6868 | 0.1287 | -0.2766 | |
| 13 | 0.0628 | -0.9980 | -0.4092 | -0.1475 | -0.0038 | 0.1476 | 0.6775 | 0.0425 | -0.2772 | |
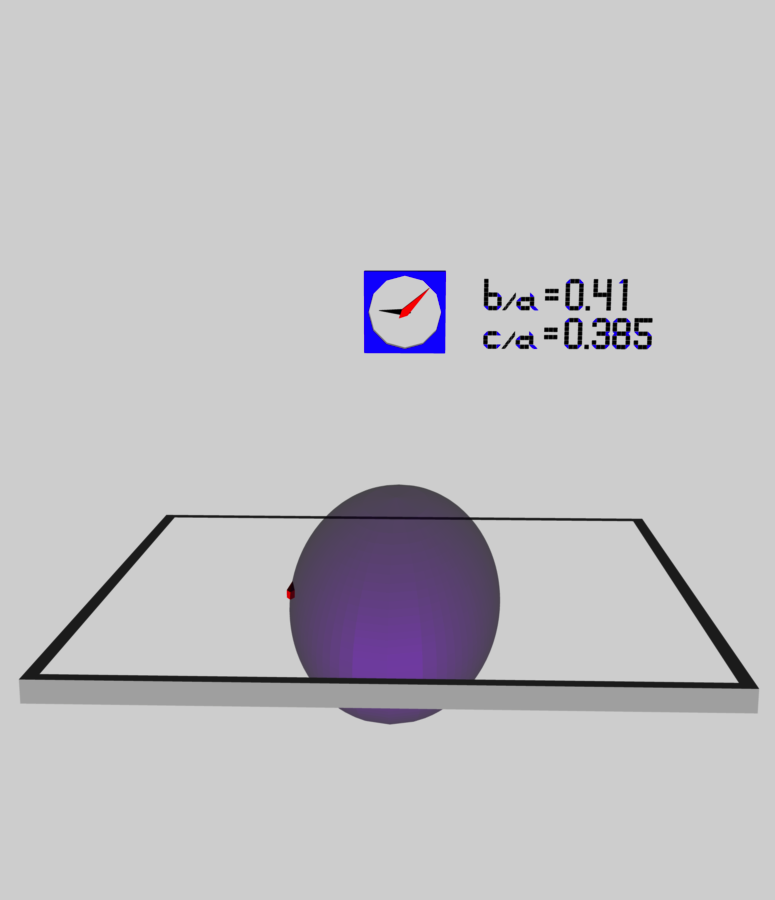
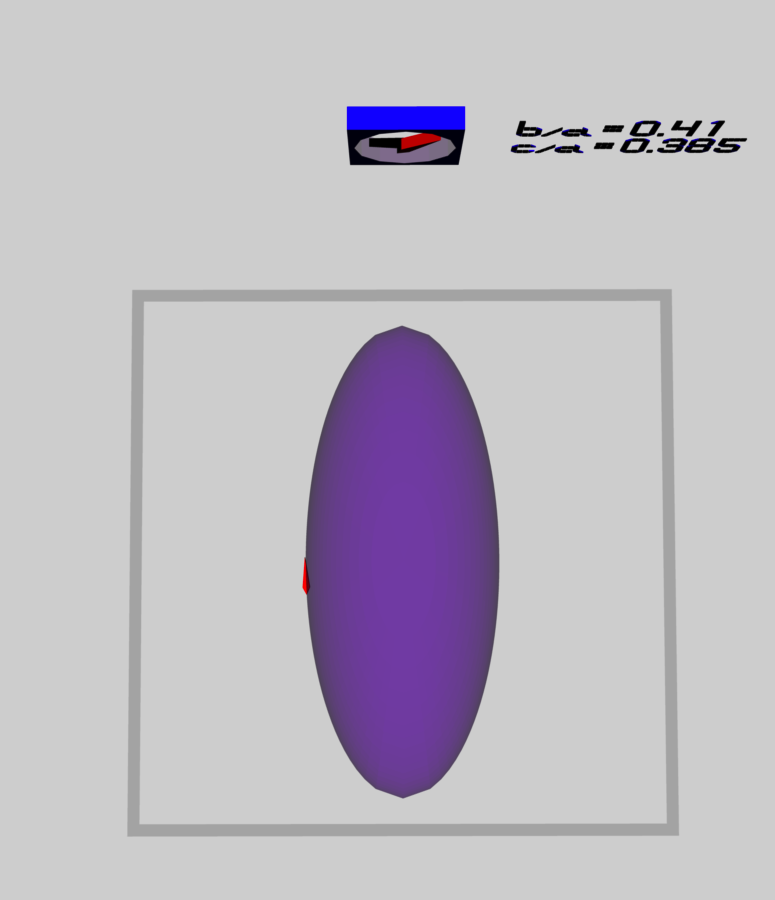
S-Type Ellipsoid Example b41c385
The EFE model that we chose to use in our first successful construction of a COLLADA-based, 3D and interactive animation had the following properties (model selected from the above table):
- <math>~b/a = 0.41</math>
- <math>~c/a = 0.385</math>
- <math>~\Omega_\mathrm{EFE} = \omega/\sqrt\pi = 0.971082/\sqrt\pi = 0.547874</math>
- <math>~\lambda_\mathrm{EFE} = 0.141594/\sqrt\pi = 0.079886</math>
Figure 1 displays a pair of still-frame images of this (purple) ellipsoidal configuration after the ellipsoid has completed precisely five (counter-clockwise) spin cycles. (The snapshots have been taken at the same point in time, but from two different "camera" viewing angles.) The cycle of the "wall mounted" clock is based on the fundamental, EFE-adopted frequency of [π G ρ]½. In the left-hand image (labeled Figure 1a), the time on the clock appears to be about 9:08. This means that as the ellipsoid has completed five spin cycles, the clock has completed approximately [9 + 8/60] ≈ 9.13 cycles. In other words, the ratio (ellipsoid-to-clock) of these two frequencies is,
|
<math>~\frac{\Omega_\mathrm{EFE}}{[\pi G \rho]^{1 / 2}}</math> |
<math>~\approx</math> |
<math>~\frac{5}{9.13} = 0.548 \, .</math> |
This matches the tabulated value of <math>~\Omega_\mathrm{EFE}</math> presented above. Now, let's examine the motion of an example Lagrangian fluid element, which has been marked in the 3D scene by a red "arrow" riding in the equatorial plane and along the surface of the (purple) ellipsoidal figure. At time zero, the fluid marker was placed at the end of the longest axis of the ellipsoid that was nearest the "wall clock"; then, as time progressed and the ellipsoidal figure turned counter-clockwise, the fluid marker moved clockwise and completed less than one full "orbit" in the same time that the ellipsoidal figure completed five full spin cycles. In the right-hand image (labeled Figure 1b), we can see that relative to the ellipsoidal figure, the fluid marker has moved through approximately three-quarters of its assigned elliptical "orbit"; let's say, 73% of one full cycle. This means that the ratio of the Lagrangian fluid element's orbital frequency to the frequency of the wall-clock is,
|
<math>~\frac{\lambda_\mathrm{EFE}}{[\pi G \rho]^{1 / 2}}</math> |
<math>~\approx</math> |
<math>~\frac{0.73}{9.13} = 0.080 \, .</math> |
This matches the tabulated value of <math>~\lambda_\mathrm{EFE}</math> presented above.
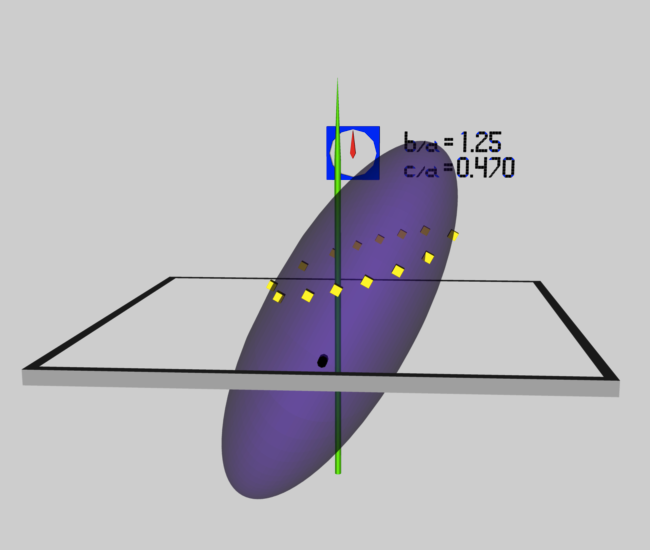
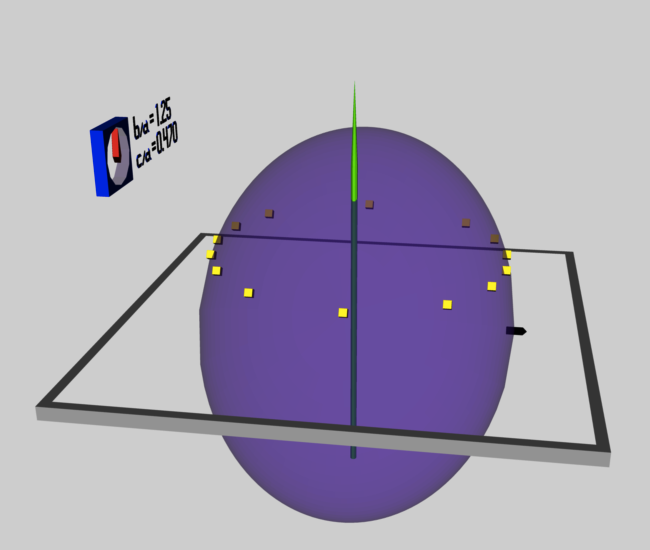
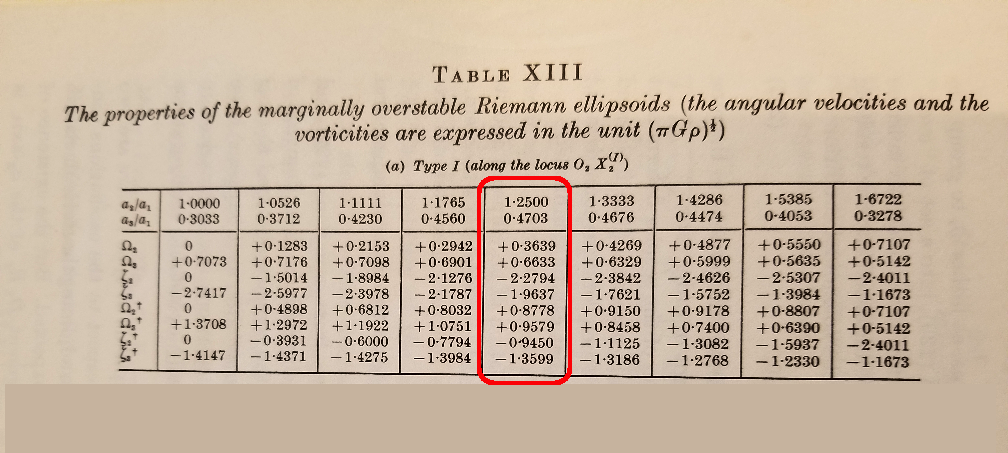
Type I Ellipsoid Example b1.25c0.470
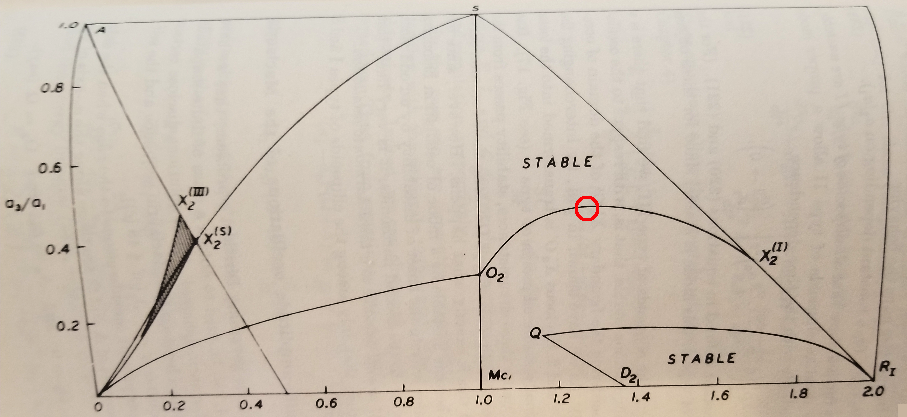
We have pulled the parameters for this example Type I Ellipsoid from Table XIII(a) on p. 170 of EFE; in addition, the superior values of β+ and γ+ have been obtained from eqs. (16) and (17), respectively, as found on p. 131 of Chapter 7, §47. This model sits along the locus O2X2(I) shown in Figure 17 (p. 171) of EFE.
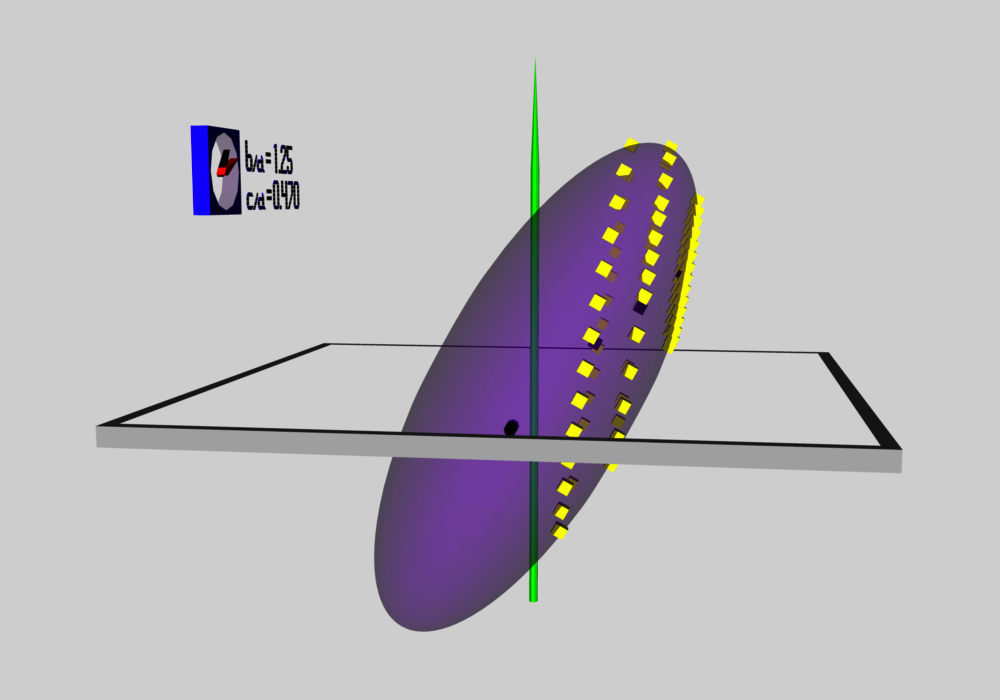
In explaining the elements depicted in Figure 2 we will reference two separate cartesian frames: The (inertial) "lab" frame and a "body" frame affixed to the ellipsoid. The x-y plane of the inertial frame is identified by the square, grey/black frame and its z-axis is identified by a thin, green, pointed vertical stick. As depicted in both panels of Figure 2, the x-axis of the ellipsoid's body frame aligns with the x-axis of the inertial frame; in panel 2a, we're generally looking in the plus-x direction toward the clock, and in both panels a black arrow "tip" is affixed to the ellipsoid's negative x-axis. In this "Type I" ellipsoid — as opposed to the "S-Type" ellipsoids discussed earlier — the x-axis of the body frame aligns permanently with the intermediate axis of the (purple) ellipsoidal configuration; following EFE, we assign the value, a = 1, to this intermediate length. The body frame's y-axis is permanently aligned with the configurations's longest axis; in the particular model displayed in Figure 2, b/a = 1.25. And the body frame's z-axis is permanently aligned with the configuration's shortest axis; in this case, c/a = 0.470.
In S-Type ellipsoids (discussed previously), the ellipsoidal configuration spins about its shortest axis — this means that the ellipsoidal configuration's angular velocity vector has no component along either the x-axis or the y-axis (Ω2 = Ω1 = 0). But for the Type I ellipsoid shown in Figure 2, Ω2 = 0.3639 while Ω3 = 0.6633. This means that the configuration's spin axis — associated with the inertial frame's z-axis (the green pointed stick in both panels of Figure 2) — is not aligned with the z-axis of the (purple) ellipsoidal configuration. As is illustrated best in panel 2a, the ellipsoidal configuration is tipped about the x-axis (Ω1 = 0) by an angle, 61.25° = tan-1(Ω3/Ω2) to the spin axis. When this COLLADA-based 3D scene is animated, the purple ellipsoid spins about the z-axis (green pointed stick) of the inertial frame at a frequency, |Ω| = [Ω22 + Ω32]½ = 0.7566, in units of [π G ρ]½.
|
In our COLLADA-based animation, we associate TIME=4 with one clock cycle. Given that <math>~|\Omega| = 0.7566 [\pi G \rho]^{1 / 2}</math> for this particular example Type I Riemann ellipsoid, we recognize that the number of clock cycles required to complete one spin about the green axis is <math>~1.0/0.7566 = 1.3217</math> cycles. Hence spinning through 360° is equivalent to TIME = 5.2868; or, TIME = 4 is equivalent to a spin angle of 272.38°. |
Now … how do we determine the motion of Lagrangian fluid elements due to the nonzero internal vorticity of the ellipsoidal structure? Evidently the vorticity vector is tipped at an angle of 40.74° = tan-1(ζ3/ζ2) to the inertial frame's z-axis. [The preceding sentence is incorrect! This is a misinterpretation of how the "tipping angle" is determined. The correct expression for this "tipping angle" is presented below.] This presumably means that, as viewed from the frame of reference of the spinning ellipsoidal figure, each Lagrangian fluid element moves along a closed (approximately elliptical?) path in a plane that is perpendicular to this vorticity vector. How we determine these various trajectories precisely?
- First, we acknowledge that EFE provides an expression for the velocity vector of each fluid element, given its instantaneous body-coordinate position (x, y, z) = (x1, x2, x3). Namely — see eq. 154 of Chapter 7, §51 (p. 156) —
<math>~u_1</math>
<math>~=</math>
<math>~- \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 y + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 z</math>
<math>~=</math>
<math>~+\biggl[ \frac{a^2}{ b^2} \biggr]\Omega_3 \gamma y - \biggl[ \frac{a^2}{c^2} \biggr] \Omega_2 \beta z \, ,</math>
<math>~u_2</math>
<math>~=</math>
<math>~+\biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 x</math>
<math>~=</math>
<math>~- \Omega_3 \gamma x \, ,</math>
<math>~u_3</math>
<math>~=</math>
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 x</math>
<math>~=</math>
<math>~-\Omega_2 \beta x \, .</math>
But we cannot immediately deduce, from these expressions alone, what the time-dependent behavior is of the three Lagrangian coordinates (x, y, z).
- Second, we need to determine how to mathematically specify, then display, the coordinates of a plane that is tipped about the x-axis at an arbitrary angle to the equatorial plane of the (purple) ellipsoidal figure.
Regarding the second issue, let's identify the coordinates of a closed curve (an ellipse?) that identifies the the intersection between a "tipped" plane and the surface of the underlying ellipsoid. The surface of the ellipsoid is defined by the equation,
|
<math>~1</math> |
<math>~=</math> |
<math>~\biggl( \frac{x}{a} \biggr)^2 + \biggl( \frac{y}{b} \biggr)^2 + \biggl( \frac{z}{c} \biggr)^2 \, .</math> |
And the "tipped" plane is defined such that, for any/all values of x,
|
<math>~z </math> |
<math>~=</math> |
<math>~my + z_0 \, .</math> |
This means,
|
<math>~1</math> |
<math>~=</math> |
<math>~\biggl( \frac{x}{a} \biggr)^2 + \frac{1}{b^2}\biggl( \frac{z-z_0}{m} \biggr)^2 + \biggl( \frac{z}{c} \biggr)^2 </math> |
|
<math>~\Rightarrow ~~~\biggl( \frac{x}{a} \biggr)^2</math> |
<math>~=</math> |
<math>~1 - \biggl(\frac{z_0}{mb}\biggr)^2 \biggl( \frac{z}{z_0} - 1 \biggr)^2 - \biggl( \frac{z}{c} \biggr)^2 \, .</math> |
If we specifically set m = 1 — that is, choose a slope of 45° — and set z0 = c — that is, choose a plane that intersects the north pole of the (purple) ellipsoid — we then have,
|
<math>~\frac{x}{a} </math> |
<math>~=</math> |
<math>~\pm \biggl[ 1 - \biggl(\frac{c}{b}\biggr)^2 \biggl( \frac{z}{c} - 1 \biggr)^2 - \biggl( \frac{z}{c} \biggr)^2 \biggr]^{1 / 2} \, .</math> |
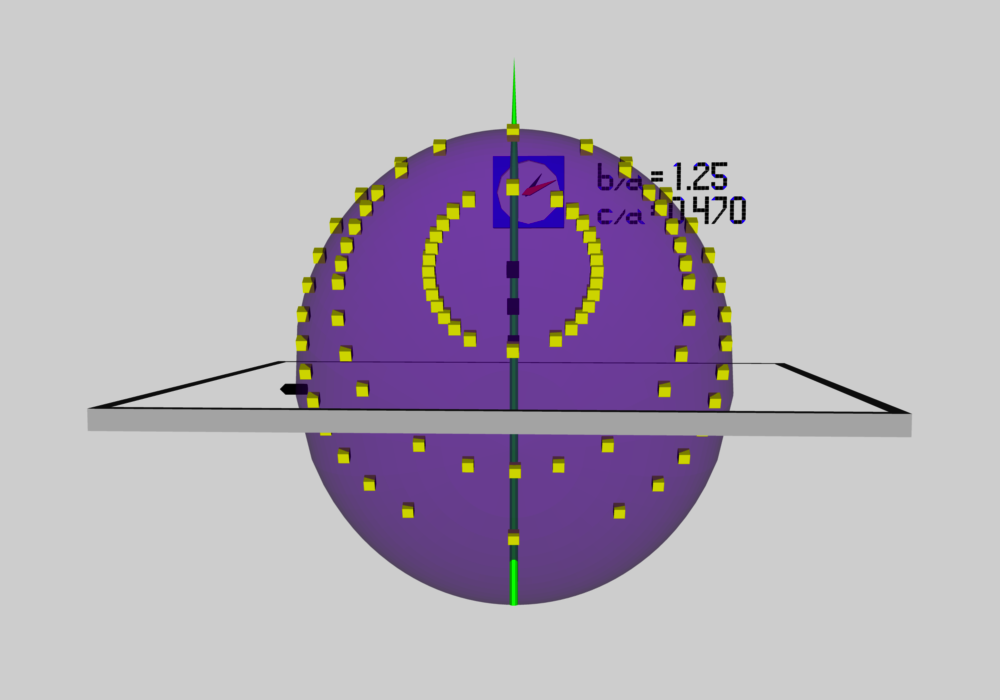
[In retrospect, we acknowledge that this was a useful way to explore how to determine the shape of each Lagrangian-fluid-element orbit. It is important to emphasize, however, that our choice of a 45° tip angle was only meant to provide an example test calculation. Eventually (see below) we determined that, for this particular Type I example, the correct tipping angle is -19.02°.] Remembering that for the chosen ellipsoidal model (a, b, c) = (1.000, 1.250, 0.4703), the following table identifies the coordinates of a collection of points that simultaneously lie on the surface of the ellipsoid and within the chosen 45° "tipped" plane. These points are each represented by a small yellow cube in both panels of Figure 2.
| m = 1 and z_0 = c | ||
|---|---|---|
| z | y | |x| |
| c | 0 | 0 |
| 0.8c | -0.2c | 0.5953 |
| 0.5c | -0.5c | 0.8454 |
| 0.25c | -0.75c | 0.9262 |
| 0 | -c | 0.9265 |
| -0.25c | -1.25c | 0.8464 |
| -0.5c | -1.5c | 0.6569 |
| -0.75227c | -1.75227c | 0 |
Here are a few thoughts/possible lines-of-attack to consider when addressing the first issue stated above.
- It is likely that the angle at which the plane of motion is tilted about the x-axis of the "body" frame is directly related to the ratio, <math>~\zeta_3/\zeta_2</math>.
- It is likely that the angle at which the plane of motion is tilted with respect to the z-axis of the "body" frame can be deduced by considering the ratio of the velocity components, vy and vz, when the x-component of the velocity vector is known to be zero; that is, when the fluid element is "crossing the x-axis."
- Does the closed-orbit motion in the tipped plane always form a (off-center) ellipse?
- Once you know the exact angle at which the orbital plane is tipped about the x-axis, an Euler-angle projection of every fluid particle's velocity vector should reduce to in-the-plane motion that has only two components. Once this transformation is made — especially if the resulting orbit is always a closed ellipse — we should be able to determine analytic expressions for the time-dependent behavior of each fluid element's x and y coordinate positions in the orbit plane and, subsequently, the (x, y, z) coordinate positions in the "body" frame.
Regarding thought "A"
For the example model depicted in Figure 2, we have,
|
<math>~\theta_\mathrm{tip} \equiv \tan^{-1}\biggl( \frac{\zeta_3}{\zeta_2} \biggr)</math> |
<math>~=</math> |
<math>~\tan^{-1}\biggl( \frac{1.9637}{2.2794} \biggr) = 40.74^{\circ}</math> |
Regarding thought "B"
When a fluid element crosses the x-axis we should expect z = 0. This needs to be rethought because the center of the closed elliptical trajectory of most fluid elements will be offset from the body's inherent z-axis.
Regarding thought "D"
|
<math>~v_x\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~v_x\biggr|_\mathrm{body}</math> |
|
<math>~v_y\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~v_y|_\mathrm{body}\cdot \cos\theta + v_z|_\mathrm{body}\cdot \sin\theta</math> |
|
<math>~v_z\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~-v_y|_\mathrm{body}\cdot \sin\theta + v_z|_\mathrm{body}\cdot \cos\theta</math> |
Setting <math>~v_z\biggr|_\mathrm{tip} = 0</math> implies,
|
<math>~v_y|_\mathrm{body}\cdot \sin\theta </math> |
<math>~=</math> |
<math>~v_z|_\mathrm{body}\cdot \cos\theta</math> |
|
<math>~\Rightarrow ~~~ \tan\theta </math> |
<math>~=</math> |
<math>~\biggl(\frac{v_z}{v_y}\biggr)_\mathrm{body}</math> |
|
|
<math>~=</math> |
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \biggl[ \frac{b^2}{a^2 + b^2} \biggr]^{-1} \zeta_3^{-1}</math> |
|
|
<math>~=</math> |
<math>~- \frac{\zeta_2}{\zeta_3}\biggl[ \frac{(c/a)^2}{1 + (c/a)^2} \biggr] \biggl[ \frac{(b/a)^2}{1 + (b/a)^2} \biggr]^{-1} \, .</math> |
For our particular example, then,
|
<math>~\tan\theta</math> |
<math>~=</math> |
<math>~- \frac{2.2794}{1.9637}\biggl[ \frac{(0.4703)^2}{1 + (0.4703)^2} \biggr] \biggl[ \frac{(1.25)^2}{1 + (1.25)^2} \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~- \frac{2.2794}{1.9637}\biggl[ \frac{(0.4703)^2}{1 + (0.4703)^2} \biggr] \biggl[ \frac{(1.25)^2}{1 + (1.25)^2} \biggr]^{-1} = - 0.3448</math> |
|
<math>~\Rightarrow ~~~ \theta</math> |
<math>~=</math> |
<math>~\tan^{-1}(-0.3448) = - 19.0^{\circ} \, . </math> |
Now, given that,
|
<math>~x</math> |
<math>~=</math> |
<math>~x_t \, ,</math> |
|
<math>~y</math> |
<math>~=</math> |
<math>~y_t \cos\theta - z_t \sin\theta \, ,</math> |
|
<math>~z</math> |
<math>~=</math> |
<math>~z_t \cos\theta + y_t \sin\theta \, ,</math> |
we can generate the following expressions for the fluid velocity components in the tipped plane:
|
<math>~v_z\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~0\, ,</math> |
|
<math>~v_x\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~v_x\biggr|_\mathrm{body}</math> |
|
|
<math>~=</math> |
<math>~- \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 y + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 z</math> |
|
|
<math>~=</math> |
<math>~- \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl[ y_t \cos\theta - z_t \sin\theta \biggr] + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl[ z_t \cos\theta + y_t \sin\theta \biggr]</math> |
|
|
<math>~=</math> |
<math>~ y_t \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} + z_t\biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \sin\theta + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \cos\theta \biggr\} \, , </math> |
|
<math>~v_y\biggr|_\mathrm{tip}</math> |
<math>~=</math> |
<math>~v_y|_\mathrm{body}\cdot \cos\theta + v_z|_\mathrm{body}\cdot \sin\theta</math> |
|
|
<math>~=</math> |
<math>~\biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 x \cdot \cos\theta - \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 x \cdot \sin\theta</math> |
|
|
<math>~=</math> |
<math>~x_t \biggl\{ \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta - \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta \biggr\} \, .</math> |
Now, following our earlier analysis for S-Type ellipsoids, let's assume that,
<math>~x_t = x_\mathrm{max}\cos(\varphi t)</math> and, <math>~y_t = y_0 - y_\mathrm{max}\sin(\varphi t) \, .</math>
(Insertion of the extra term, y0, acknowledges that the center of the elliptical orbit in the "tipped" plane will be shifted off of the x-axis in the yt-direction; the size of this shift should correlate with zt, that is, with the vertical location of the tipped plane.) We have, then,
|
<math>~v_y\biggr|_\mathrm{tip} = \frac{dy_t}{dt} = -y_\mathrm{max} \varphi \cos(\varphi t)</math> |
<math>~=</math> |
<math>~x_\mathrm{max} \cos(\varphi t) \biggl\{ \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta - \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta \biggr\} \, ,</math> |
|
<math>~v_x\biggr|_\mathrm{tip} = \frac{dx_t}{dt} = -x_\mathrm{max}\varphi \sin(\varphi t)</math> |
<math>~=</math> |
<math>~ \biggl[ y_0 - y_\mathrm{max} \sin(\varphi t) \biggr]\biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} + z_t\biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \sin\theta + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \cos\theta \biggr\} \, . </math> |
|
|
<math>~=</math> |
<math>~ - y_\mathrm{max} \sin(\varphi t) \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} </math> |
|
|
|
<math>~ + y_0 \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} + z_t\biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \sin\theta + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \cos\theta \biggr\} \, . </math> |
So, in terms of the value of zt, the offset in yt must be,
|
<math>~ - y_0 \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} </math> |
<math>~=</math> |
<math>~ + z_t\biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \sin\theta + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \cos\theta \biggr\} \, ; </math> |
in which case,
|
<math>~ -y_\mathrm{max} \varphi \cos(\varphi t)</math> |
<math>~=</math> |
<math>~x_\mathrm{max} \cos(\varphi t) \biggl\{ \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta - \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta \biggr\} \, ,</math> |
|
<math>~ \Rightarrow ~~~ \varphi </math> |
<math>~=</math> |
<math>~-\biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl\{ \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta - \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta \biggr\} \, ,</math> |
and,
|
<math>~-x_\mathrm{max}\varphi \sin(\varphi t)</math> |
<math>~=</math> |
<math>~ - y_\mathrm{max} \sin(\varphi t) \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} </math> |
|
<math>~\Rightarrow ~~~ \varphi </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]\biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} \, . </math> |
In order for both of these expressions to be simultaneously true, we need,
|
<math>~\biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl\{ \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta - \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta \biggr\}</math> |
<math>~=</math> |
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]\biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta - \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \sin\theta - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \cdot \cos\theta - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta </math> |
|
<math>~\Rightarrow ~~~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggl\} \frac{\zeta_2 \sin\theta }{(a^2 + c^2)} </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggr\} \frac{\zeta_3 \cos\theta }{(a^2 + b^2)} \, . </math> |
First Trial Value of θ
Now, from above, we determined that the "tip" angle must obey the expression,
|
<math>~\tan\theta </math> |
<math>~=</math> |
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \biggl[ \frac{b^2}{a^2 + b^2} \biggr]^{-1} \zeta_3^{-1} \, .</math> |
Hence, we require,
|
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 \cdot \biggl[ \frac{b^2}{a^2 + b^2} \biggr]^{-1} \zeta_3^{-1} \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggl\} \frac{\zeta_2 }{(a^2 + c^2)} </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggr\} \frac{\zeta_3 }{(a^2 + b^2)} </math> |
|
<math>~ \Rightarrow ~~~ \biggl\{ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 - \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 \biggl\} \frac{c^2 \zeta_2^2 }{(a^2 + c^2)^2} </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggr\} \frac{b^2\zeta_3^2 }{(a^2 + b^2)^2} </math> |
|
<math>~ \Rightarrow ~~~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 \frac{a^2 c^2 \zeta_2^2 }{(a^2 + c^2)^2} - \frac{c^4 \zeta_2^2 }{(a^2 + c^2)^2} </math> |
<math>~=</math> |
<math>~ \frac{b^4 \zeta_3^2 }{(a^2 + b^2)^2} - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 \frac{a^2 b^2\zeta_3^2 }{(a^2 + b^2)^2} \, . </math> |
That is,
|
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{b^4 \zeta_3^2 }{(a^2 + b^2)^2} + \frac{c^4 \zeta_2^2 }{(a^2 + c^2)^2} \biggr] \biggl[ \frac{a^2 c^2 \zeta_2^2 }{(a^2 + c^2)^2} + \frac{a^2 b^2\zeta_3^2 }{(a^2 + b^2)^2} \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ b^4 \zeta_3^2 (a^2 + c^2)^2 + c^4 \zeta_2^2 (a^2 + b^2)^2 \biggr] \biggl[ a^2 c^2 \zeta_2^2 (a^2 + b^2)^2 + a^2 b^2\zeta_3^2 (a^2 + c^2)^2 \biggr]^{-1} \, . </math> |
So for the example parameters provided above, we have,
|
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 </math> |
<math>~=</math> |
<math>~ \biggl[ 14.04 + 1.669 \biggr] \biggl[ 7.546 + 8.985 \biggr]^{-1} = 0.9502 </math> |
|
<math>~ \Rightarrow~~~ \frac{y_\mathrm{max}}{x_\mathrm{max}} </math> |
<math>~=</math> |
<math>~ 0.9748 </math> |
Second Trial Value of θ
Instead, what if we obtain the tip angle straight from the tabulated expression,
|
<math>~\tan\theta</math> |
<math>~=</math> |
<math>~\frac{\zeta_3}{\zeta_2} \, .</math> |
Then we have,
|
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggl\} \frac{1 }{(a^2 + c^2)} </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggr\} \frac{1 }{(a^2 + b^2)} </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 (a^2 + b^2) - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 (a^2 + b^2) </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 (a^2 + c^2) - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 (a^2 + c^2) </math> |
|
<math>~\Rightarrow~~~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 </math> |
<math>~=</math> |
<math>~ \biggl[ c^2 (a^2 + b^2)- b^2 (a^2 + c^2) \biggr] \biggl[ a^2 (a^2 + b^2) - a^2 (a^2 + c^2) \biggr]^{-1} </math> |
|
|
<math>~=</math> |
<math>~ [ c^2 - b^2 ] [ b^2 - c^2 ]^{-1} = -1 \, . </math> |
And this is not physically acceptable. What if, instead, we set,
|
<math>~\tan\theta</math> |
<math>~=</math> |
<math>~- \frac{\zeta_3}{\zeta_2} ~~~\Rightarrow ~~~ \theta = -40.74^\circ \, .</math> |
Then we have,
|
<math>~ - \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggl\} \frac{1 }{(a^2 + c^2)} </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 \biggr\} \frac{1 }{(a^2 + b^2)} </math> |
|
<math>~\Rightarrow ~~~ - \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] c^2 (a^2 + b^2) + \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 (a^2 + b^2) </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] b^2 (a^2 + c^2) - \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] a^2 (a^2 + c^2) </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] [ a^2 (a^2 + b^2) + a^2 (a^2 + c^2) ] </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] [ b^2 (a^2 + c^2) + c^2 (a^2 + b^2) ] </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 </math> |
<math>~=</math> |
<math>~ [ b^2 (a^2 + c^2) + c^2 (a^2 + b^2) ] [ a^2 (a^2 + b^2) + a^2 (a^2 + c^2) ]^{-1} \, . </math> |
In our particular example, this means,
|
<math>~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr]^2 </math> |
<math>~=</math> |
<math>~ [ 2.4749 ] [ 3.7837 ]^{-1} = 0.6541 </math> |
|
<math>~\Rightarrow ~~~ \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \bigg] </math> |
<math>~=</math> |
<math>~ 0.8088 \, . </math> |
Try Again
Methodical Derivation of Orbital Parameters
Let the unprimed coordinates (x, y, z) represent the body frame of the ellipsoid, and the primed coordinates (x', y', z') represent a coordinate system in which the z'-axis is "tipped" — about the x' = x axis — away from the z-axis by an angle, θ. We assume that the motion of each Lagrangian fluid element will be restricted to an x'-y' equatorial plane — that is, we assume that <math>~\dot{z}' \equiv dz'/dt = 0</math> — but in general its velocity vector in the unprimed "body" frame will have the three nonzero components as specified in EFE and above. Here are some relevant transformations between these two coordinate systems.
|
|
||||||||||||||||||
|
|
NOTE: The center of each elliptical orbit is (x', y', z') = (0, y0, 0). In "body" coordinates, then, (x, y, z) = (0, y0 cosθ, z0 + y0 sinθ).
Focusing on the bottom-right quadrant of this equation-table, we note first that <math>~(\dot{x}, \dot{y}, \dot{z}) = (u_1, u_2, u_3)</math>, as provided above from EFE. Copying from the upper-right quadrant of this equation-table, let's rewrite these three velocity components in terms of the "tipped" plane coordinates, namely,
|
<math>~\dot{x} = u_1</math> |
<math>~=</math> |
<math>~ - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl[ y'\cos\theta - z'\sin\theta\biggr] + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl[ z_0 + z'\cos\theta + y'\sin\theta \biggr] \, ,</math> |
|
<math>~\dot{y} = u_2</math> |
<math>~=</math> |
<math>~+\biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 x' \, ,</math> |
|
<math>~\dot{z} = u_3</math> |
<math>~=</math> |
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 x' \, .</math> |
Now, if we assume that each Lagrangian particle executes a closed elliptical orbit in the plane of the tipped coordinate system (i.e., <math>~z' = \dot{z}' = 0</math> ), but whose orbit-center may be shifted by an amount, <math>~y_0</math>, away from the z'-axis, we expect …
|
<math>~x'</math> |
<math>~=</math> |
<math>~x_\mathrm{max}\cos(\varphi t)</math> |
and, |
<math>~y' - y_0</math> |
<math>~=</math> |
<math>~y_\mathrm{max}\sin(\varphi t) \, ,</math> |
which implies,
|
<math>~\dot{x}'</math> |
<math>~=</math> |
<math>~- x_\mathrm{max}~ \varphi \cdot \sin(\varphi t)</math> |
and, |
<math>~\dot{y}' </math> |
<math>~=</math> |
<math>~y_\mathrm{max}~\varphi \cdot \cos(\varphi t) \, .</math> |
The first of these equation pairs can be plugged directly into the expressions for u1, u2, and u3 — further fleshing out the LHS of the equations in the bottom-right quadrant of the above equation-table — while the second pair can be used to re-express the RHS of the equations in the bottom-right quadrant of this equation table. We obtain,
|
<math>~ - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl\{ \biggl[ y_0 + y_\mathrm{max} \sin(\varphi t) \biggr] \cos\theta - \cancelto{0}{z'}\sin\theta \biggr\} + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl\{ z_0 + \cancelto{0}{z'} \cos\theta + \biggl[ y_0 + y_\mathrm{max} \sin(\varphi t) \biggr]\sin\theta \biggr\} </math> |
<math>~=</math> |
<math>~-x_\mathrm{xmax} ~\varphi \cdot \sin(\varphi t) \, ,</math> |
|
<math>~+\biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 x_\mathrm{max}\cos(\varphi t) </math> |
<math>~=</math> |
<math>~y_\mathrm{max}~\varphi \cdot \cos(\varphi t) \cdot \cos\theta \, ,</math> |
|
<math>~- \biggl[ \frac{c^2}{a^2 + c^2} \biggr] \zeta_2 x_\mathrm{max}\cos(\varphi t) </math> |
<math>~=</math> |
<math>~y_\mathrm{max}~\varphi \cdot \cos(\varphi t) \cdot \sin\theta \, .</math> |
Combining the second and third of these conditions, we find first that,
|
<math>~\tan\theta </math> |
<math>~=</math> |
<math>~- \frac{\zeta_2 }{ \zeta_3 } \biggl[ \frac{a^2 + b^2}{a^2 + c^2} \biggr]\frac{c^2}{b^2} \, ,</math> |
which gives the "tipping" angle in terms of known parameter values; and second that …
|
<math>~\biggl[ \frac{x_\mathrm{max}}{y_\mathrm{max}} \biggr] \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 </math> |
<math>~=</math> |
<math>~\varphi \cdot \cos\theta \, ,</math> |
|
<math>~\Rightarrow ~~~ \varphi \biggl[ \frac{y_\mathrm{max}}{x_\mathrm{max}} \biggr] </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \frac{\zeta_3}{\cos\theta} \, ,</math> |
which gives the product of the two unknown quantities, <math>~\varphi</math> and the ratio <math>~y_\mathrm{max}/x_\mathrm{max}</math>, in terms of known parameter values. And from the first condition, we furthermore find that,
|
<math>~-x_\mathrm{max} ~\varphi \cdot \sin(\varphi t) </math> |
<math>~=</math> |
<math>~ - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl[ y_0 \cos\theta \biggr] - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl[ y_\mathrm{max} \sin(\varphi t) \biggr] \cos\theta + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl[ z_0 + y_0 \sin\theta \biggr] + \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl[ y_\mathrm{max} \sin(\varphi t) \biggr]\sin\theta </math> |
|
<math>~ \Rightarrow ~~~ x_\mathrm{max} ~\varphi \cdot \sin(\varphi t) + \biggl\{ \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta - \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta \biggr\} \biggl[ y_\mathrm{max} \sin(\varphi t) \biggr] </math> |
<math>~=</math> |
<math> \biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \biggl[ y_0 \cos\theta \biggr] - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 z_0 - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl[ y_0 \sin\theta \biggr] \biggr\} \, . </math> |
The LHS and the RHS must separately sum to zero, which means …
|
<math>~ \biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta \biggr\}y_0 </math> |
<math>~=</math> |
<math> \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 z_0 \, , </math> |
which gives the ratio, <math>~y_0/z_0</math> in terms of known parameter values; and,
|
<math>~ \biggl[ \frac{x_\mathrm{max}}{ y_\mathrm{max} } \biggr] ~\varphi </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta \, , </math> |
which in combination with the just-derived similar product relation can give expressions for both terms, namely,
|
<math>~ \biggl[ \frac{x_\mathrm{max}}{ y_\mathrm{max} } \biggr]^2 </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta \biggr\}\biggl[ \frac{a^2 + b^2}{b^2} \biggr] \frac{\cos\theta}{\zeta_3} \, , </math> |
and,
|
<math>~ \varphi^2 </math> |
<math>~=</math> |
<math>~ \biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \tan\theta \biggr\}\biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \, . </math> |
Example Implementation
Drawing from the set of Case I parameters listed above, we will set a = 1, b = 1.25, c = 0.4703, ζ2 = -2.2794, and ζ3 = -1.9637. This means that,
|
<math>~\tan\theta </math> |
<math>~=</math> |
<math>~- \frac{\zeta_2 }{ \zeta_3 } \biggl[ \frac{a^2 + b^2}{a^2 + c^2} \biggr]\frac{c^2}{b^2} = - 0.3448 ~~~\Rightarrow ~~~ \theta = -19.02^\circ \, .</math> |
We deduce as well that,
|
<math>~ \frac{y_0}{z_0} </math> |
<math>~=</math> |
<math> \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \biggl\{ \biggl[ \frac{a^2}{a^2 + b^2} \biggr] \zeta_3 \cos\theta - \biggl[ \frac{a^2}{a^2 + c^2} \biggr] \zeta_2 \sin\theta \biggr\}^{-1} = -1.8666 \cdot \{-0.7245 - 0.6084 \}^{-1} = 1.4004 \, ; </math> |
|
<math>~ \frac{x_\mathrm{max}}{ y_\mathrm{max} } </math> |
<math>~=</math> |
<math>~\biggl\{ \biggl[ \biggl( \frac{a^2}{a^2 + b^2} \biggr) \zeta_3 \cos\theta - \biggl( \frac{a^2}{a^2 + c^2} \biggr) \zeta_2 \sin\theta \biggr] \biggl[ \frac{a^2 + b^2}{b^2} \biggr] \frac{\cos\theta}{\zeta_3} \biggr\}^{1 / 2} = \{ [-1.3329] \cdot (-0.7895) \}^{1 / 2} = \sqrt{1.0524} = 1.0259 \, ; </math> |
|
<math>~ \varphi </math> |
<math>~=</math> |
<math>~\biggl\{ \biggl[ \biggl( \frac{a^2}{a^2 + b^2} \biggr) \zeta_3 - \biggl( \frac{a^2}{a^2 + c^2} \biggr) \zeta_2 \tan\theta \biggr] \biggl[ \frac{b^2}{a^2 + b^2} \biggr] \zeta_3 \biggr\}^{1 / 2} = 1.2993 \, . </math> |
|
It is important to keep in mind that each Lagrangian fluid element will complete one full orbit in its "tipped" plane when <math>~\varphi t = 2\pi</math>, where <math>~t</math> is in units of <math>~[\pi G \rho]^{-1 / 2}</math> and <math>~\varphi</math> is in units of <math>~[\pi G \rho]^{+1 / 2}</math>. Note, as well, that in our COLLADA animations, we have adopted the convention that <math>~t = 1</math> when <math>~\mathrm{TIME} = 4</math>. Hence, one complete orbit will be completed when,
That is to say, in the specific example being used here, on complete orbit is concluded when TIME = 19.343. |
Referring back to our earlier geometric prescription of a "tipped plane" we insert this new slope having m = tanθ = -0.3448, and choose a particular plane by setting a value of z0. Then we can map out a locus of points that show the intersection of the chosen plane with the surface of the ellipsoid by specifying various values of z, then calculating the corresponding values of y and x via the respective equations:
|
<math>~y </math> |
<math>~=</math> |
<math>~\frac{1}{\tan\theta} \biggl[ z - z_0 \biggr] \, ;</math> |
|
<math>~\frac{x}{a} </math> |
<math>~=</math> |
<math>~\biggl[ 1 - \biggl(\frac{z_0}{b \tan\theta}\biggr)^2 \biggl( \frac{z}{z_0} - 1 \biggr)^2 - \biggl( \frac{z}{c} \biggr)^2 \biggr]^{1 / 2} \, .</math> |
For each specified value of z0, the relevant range of z values is given by the pair of values for which x/a = 0. For example, if we set z_0 = b tanθ = -0.4310, this pair is given by the roots of the quadratic expression,
|
<math>~1 </math> |
<math>~=</math> |
<math>~\biggl( \frac{z}{z_0} - 1 \biggr)^2 + \biggl( \frac{z}{c} \biggr)^2 </math> |
|
<math>~\Rightarrow ~~~ 0 </math> |
<math>~=</math> |
<math>~z^2\biggl[ \frac{1}{z_0^2} + \frac{1}{c^2} \biggr] -\frac{2z}{z_0} </math> |
|
|
<math>~=</math> |
<math>~z \biggl\{ z - \biggl[ \frac{2 z_0 c^2}{(c^2 + z_0^2)} \biggr] \biggr\} \biggl[ \frac{(c^2 + z_0^2)}{c^2 z_0^2} \biggr] \, .</math> |
The roots are, then, <math>~z_\mathrm{max} = 0</math>, and
|
<math>~z_\mathrm{min}</math> |
<math>~=</math> |
<math>~\frac{2 z_0 c^2}{(c^2 + z_0^2)} = -0.468514 \, .</math> |
The middle three columns (table cells having bgcolor="lightblue") in the following table list values of |x| and y that correspond to various values of z that lie between this pair of limiting values.
| <math>~m = \tan\theta = -0.3448 ~~~ \Rightarrow ~~~ \theta = -19.02^\circ</math> | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| z_0 = -0.2500 | z_0 = b tanθ = -0.4310 | z_0 = -0.6000 | ||||||||
| z | y | |x| | z | y | |x| | z | y | |x| | ||
| 0.1564 | -1.1788 | 0.0000 | 0 | -1.2500 | 0 | -0.2182 | -1.1073 | 0.0000 | ||
| 0.1147 | -1.0577 | 0.4739 | -0.02 | - 1.1920 | 0.2981 | -0.2336 | -1.0626 | 0.1749 | ||
| 0.0729 | -0.9366 | 0.6439 | -0.05 | -1.1050 | 0.4553 | -0.2490 | -1.0179 | 0.2377 | ||
| 0.0312 | -0.8155 | 0.7550 | -0.08 | -1.0180 | 0.5548 | -0.2645 | -0.9732 | 0.2787 | ||
| -0.0106 | -0.6943 | 0.8312 | -0.1 | -0.9600 | 0.6042 | -0.2799 | -0.9285 | 0.3068 | ||
| -0.0524 | -0.5732 | 0.8817 | -0.125 | -0.8875 | 0.6521 | -0.2953 | -0.8838 | 0.3255 | ||
| -0.0941 | -0.4521 | 0.9106 | -0.15 | -0.8150 | 0.6879 | -0.3107 | -0.8390 | 0.3361 | ||
| -0.1359 | -0.3310 | 0.9200 | -0.175 | -0.7424 | 0.7133 | -0.3261 | -0.7943 | 0.3396 | ||
| -0.1776 | -0.2099 | 0.9106 | -0.2 | - 0.6699 | 0.7293 | -0.3415 | -0.7496 | 0.3361 | ||
| -0.2194 | -0.0887 | 0.8817 | -0.25 | - 0.5249 | 0.7356 | -0.3570 | -0.7049 | 0.3255 | ||
| -0.2612 | 0.0324 | 0.8312 | -0.30 | - 0.3799 | 0.7076 | -0.3724 | -0.6602 | 0.3068 | ||
| -0.3029 | 0.1535 | 0.7550 | -0.35 | - 0.2349 | 0.6410 | -0.3878 | -0.6155 | 0.2787 | ||
| -0.3447 | 0.2746 | 0.6439 | -0.4 | - 0.0899 | 0.5210 | -0.4032 | -0.5708 | 0.2377 | ||
| -0.3865 | 0.3957 | 0.4739 | -0.43 | - 0.0029 | 0.4050 | -0.4186 | -0.5261 | 0.1749 | ||
| -0.4282 | 0.5169 | 0.0 | -0.46 | +0.0841 | 0.1950 | -0.4340 | -0.4814 | 0.0000 | ||
| -0.4685 | +0.1088 | 0.0081 | ||||||||
More generally, letting <math>~q \equiv z_0/(b\tan\theta)</math>, the pair of limiting values of z are given by the roots of the quadratic expression,
|
<math>~1 </math> |
<math>~=</math> |
<math>~q^2 \biggl( \frac{z}{z_0} - 1 \biggr)^2 + \biggl( \frac{z}{c} \biggr)^2 </math> |
|
<math>~\Rightarrow ~~~0 </math> |
<math>~=</math> |
<math>~ z^2 \biggl[ \frac{ q^2c^2 + z_0^2)}{z_0^2 q^2 c^2} \biggr] - \frac{2z}{z_0} + \biggl[ 1 - \frac{1}{q^2} \biggr] \, .</math> |
That is,
|
<math>~z </math> |
<math>~=</math> |
<math>~ \biggl[ \frac{z_0^2 q^2 c^2}{ 2(q^2 c^2 + z_0^2)} \biggr]\biggl\{ \frac{2}{z_0} \pm \biggl[\frac{4}{z_0^2} - \frac{ 4 (1 - q^{-2})(q^2 c^2 + z_0^2)}{z_0^2 q^2 c^2} \biggr]^{1 / 2} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ \biggl[ \frac{z_0 q^2 c^2}{ (q^2 c^2 + z_0^2)} \biggr]\biggl\{ 1 \pm \biggl[1 - \frac{ (1 - q^{-2})(q^2 c^2 + z_0^2)}{ q^2 c^2} \biggr]^{1 / 2} \biggr\} \, .</math> |
And we see that the constraint set on z0 is given by the condition,
|
<math>~1</math> |
<math>~\ge</math> |
<math>~\frac{ (1 - q^{-2})(q^2c^2 + z_0^2)}{q^2c^2} </math> |
|
<math>~\Rightarrow ~~~ q^2 c^2</math> |
<math>~\ge</math> |
<math>~(1 - q^{-2})(q^2c^2 + z_0^2) </math> |
|
|
<math>~\ge</math> |
<math>~(q^2 - 1)(c^2 + b^2\tan^2\theta) </math> |
|
<math>~\Rightarrow ~~~ 0</math> |
<math>~\ge</math> |
<math>~ (q^2 - 1)b^2\tan^2\theta - c^2</math> |
|
|
<math>~\ge</math> |
<math>~ z_0^2 - c^2 - b^2\tan^2\theta</math> |
|
<math>~\Rightarrow ~~~ z_0^2</math> |
<math>~\le</math> |
<math>~ c^2 + b^2\tan^2\theta \, .</math> |
Therefore, for this example Type I ellipsoidal configuration, z0 must lie within the range,
|
<math>~-0.650165</math> |
<math>~\le z_0 \le</math> |
<math>~+0.650165 \, .</math> |
In the above table, we have detailed the loci of points along the surface trajectories that correspond to the values, z0 = - 0.2500 (the leftmost three columns of the table) and z0 = -0.6000 (the rightmost three columns of the table). The limiting values of z for these to choices of z0 are, respectively, [zmin = -0.4283, zmax = +0.1564] and [zmin = -0.4340, zmax = -0.2182].
Using COLLADA, we have constructed an animated and interactive 3D scene that displays in purple the surface of our example Type I ellipsoid; panels a and b of Figure 3 show what this ellipsoid looks like when viewed from two different perspectives. (As a reminder — see the explanation accompanying Figure 2, above — the ellipsoid is tilted about the x-coordinate axis at an angle of 61.25° to the equilibrium spin axis, which is shown in green.) Yellow markers also have been placed in this 3D scene at each of the coordinate locations specified in the above table. From the perspective presented in Figure 3b, we can immediately identify three separate, nearly circular trajectories; the largest one corresponds to our choice of z0 = -0.25, the smallest corresponds to our choice of z0 = -0.60, and the one of intermediate size correspond to our choice of z0 = -0.4310. When viewed from the perspective presented in Figure 3a, we see that these three trajectories define three separate planes; each plane is tipped at an angle of θ = -19.02° to the untilted equatorial, x-y plane of the purple ellipsoid.
As we have created each orbit in the xml-based COLLADA file, we have first manually typed in the (TIME, x, y, z) coordinates of each orbit that has a negative value of z0. Specifically, using the Figure 3c projection as a guide/reference, we typed in coordinates for the following orbits:
- FLUID ELEMENT 1: <math>~z_0 = -0.6000</math>
| nstep | TIME | x | y | z |
| 1 | 0.000 | 0.000 | -1.107 | -0.218 |
| 26 | 4.836 | -0.340 | -0.794 | -0.326 |
| 51 | 9.672 | 0.000 | -0.481 | -0.434 |
| 76 | 14.507 | +0.340 | -0.794 | -0.326 |
| 101 | 19.343 | 0.000 | -1.107 | -0.218 |
- FLUID ELEMENT 2: <math>~z_0 = -0.4300</math>
See Also
- Discussion of Ou's Riemann-Like Ellipsoids
- Riemann Meets COLLADA & Oculus Rift S
- Virtual Reality and 3D Printing
- Success Importing Animated Scene into Oculus Rift S
- Carefully (Re)Build Riemann Type S Ellipsoids Inside Oculus Rift Environment: Example (b/a, c/a) = (0.41, 0.385)
- Other Example S-type Riemann Ellipsoids:
Chandrasekhar's Detailed Analysis
- Bernhard Riemann (1876) Gesammelte Mathematische Werke und Wissenschaftlicher, especially Chapter X (p. 168) titled (something along the following line), "A Contribution to Research on Rotating Ellipsoidal Fluids"
- S. Chandrasekhar (1965), ApJ, 142, 890 - 961. The Equilibrum and the Stability of the Riemann Ellipsoids. I. — This work is referenced as Paper XXV in EFE and focuses on S-type Riemann ellipsoids.
- S. Chandrasekhar (1966), ApJ, 145, 842 - 877. The Equilibrum and the Stability of the Riemann Ellipsoids. II. — This work is referenced as Paper XXVIII in EFE and focuses on Riemann ellipsoids of Types I, II and III.
Finite-Amplitude Oscillations
- L. F. Rossner (1967), ApJ, 149, 145. The Finite-Amplitude Oscillations of the Maclaurin Spheroids — This work is referenced as Paper XXXVIII in EFE.
- M. Fujimoto (1968), ApJ, 152, 523. Gravitational Collapse of Rotating Gaseous Ellipsoids
- T. T. Chia & S. Y. Pung (1995), Astrophysics and Space Science, 229, issue 2, 215 - 233. Effects of Variations of Parallel Angular Velocity and Vorticity on the Oscillations of Compressible Homogeneous Rotating Ellipsoids
- T. T. Chia & S. Y. Pung (1997), Astrophysics and Space Science, 254, 269 - 294. Dynamical Behaviour of Compressible Homogeneous Uniformly Rotating Ellipsoids with Nonparallel Angular Velocity and Vorticity
In the Context of Galaxy Disks
- C. Hunter (1970), ApJ, 162, 97 - 103. The Disklike Riemann Ellipsoids.
Other

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |