User:Tohline/Appendix/Ramblings/StrongNuclearForce
Radial Dependence of the Strong Nuclear Force

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
Wikipedia as a Resource
QGP:
- "QGP (quark-gluon plasma) is a state of matter in which the elementary particles that make up the hadrons of baryonic matter are freed of their strong attraction for one another under extremely high energy densities."
- "In normal matter quarks are confined; in the QGP (quark-gluon plasma) quarks are deconfined."
-
"In classical QCD quarks are the fermionic components of hadrons (mesons and baryons) while the gluons are considered the boson components of such particles. The gluons are the force carriers, or bosons, of the QCD color force, while the quarks by themselves are their fermionic matter counterparts."
- Electrons (spin 1/2 particles) and (as a composite particle) protons are fermions; they obey Fermi-Dirac statistics.
- According to the Standard Model of Particle Physics, photons (spin 1 particles) are one of only 5 elementary bosons; they obey Bose-Einstein statistics.
- "[This] phenomenon can be understood qualitatively by noting that the force-carrying [bosonic] gluons of QCD have color charge [as well as do the fermionic quarks], unlike the photons of QED. Whereas the electric field between electrically charged particles decreases rapidly as those particles are separated, the gluon field between a pair of color charges forms a narrow flux tube (or string) between them. Because of this behavior of the gluon field, the strong force between the particles is constant regardless of their separation."
- "Unlike all other forces … the strong force does not diminish in strength with increasing distance between pairs of quarks. After a limiting distance (about the size of a hadron) has been reached, it remains at a strength of about 10,000 newtons, no matter how much farther the distance between the quarks." Hence, the effective potential has a term that is linear in r.
Tidbits
From an online chat:
-
From the study of the spectrum of quarkonium (bound system of quark and antiquark) and the comparison with positronium one finds as potential for the strong force,
<math>~V(r)</math>
<math>~=</math>
<math>~ - \frac{4}{3} \cdot \frac{\alpha_s(r) \hbar c}{r} + kr \, , </math>
where, the constant <math>~k</math> determines the field energy per unit length and is called string tension. For short distances this resembles the Coulomb law, while for large distances the <math>~kr</math> factor dominates (confinement). It is important to note that the coupling <math>~\alpha_s</math> also depends on the distance between the quarks.
This formula is valid and in agreement with theoretical predictions only for the quarkonium system and its typical energies and distances. For example charmonium: <math>~r \approx 0.4~\mathrm{fm}</math>.
- Of course, the "breaking of the flux tube" has no classical or semi-classical analogue, making this formulation better for hand waving than calculation.
- This is fine for the quark-qark interaction, but people reading this answer should be careful not to interpret it as a nucleon-nucleon interaction.
-
At the level of quantum hadron dynamics (i.e., the level of nuclear physics, not the level of particle physics where the real strong force lives) one can talk about a Yukawa potential of the form,
<math>~V(r)</math>
<math>~=</math>
<math>~ - \frac{g^2}{4\pi c^2} \cdot \frac{e^{-mr}}{r} \, , </math>
where <math>~m</math> is roughly the pion mass and <math>~g</math> is an effective coupling constant. To get the force related to this you would take the derivative in <math>~r</math>.
This is a semi-classical approximation, but it is good enough that Walecka used it briefly in his book.
- The nuclear force is now understood as a residual effect of the even more powerful strong force, or strong interaction, which is the attractive force that binds particles called quarks together, to form the nucleons themselves. This more powerful force is mediated by particles called gluons. Gluons hold quarks together with a force like that of electric charge but of far greater power. Marek is talking of the strong force that binds the quarks within the protons and neutrons. There are charges, called colored charges on the quarks, but protons and neutrons are color neutral. Nuclei are bound by the interplay between the residual strong force, the part that is not shielded by the color neutrality of the nucleons, and the electro magnetic force due to the charge of the protons. That also cannot be simply described. Various potentials are used to calculate nuclear interactions.
From the arXiv preprint of a review article by A. Deur, S. J. Brodsky, & G. F. de Téramond (2020) titled, The QCD Running Coupling:
- (middle of p. 10) "We illustrate this behavior" — that is, "… the scale dependence of the coupling" — "for the coupling that arises in the static case of heavy sources and which provides a simple physical picture. Historically, and in the case of linear theories with massless force carriers, a force coupling constant is a universal coefficient that links the force to the 'charges' of two bodies (e.g., the electric charge for electricity or the mass for gravity) divided by the distance dependence <math>1/r^2</math>."
- (middle of p. 10, continued) "In QFT (quantum field theory) … for weak enough forces, the first Born approximation dominates higher order contributions and the <math>1/q^2</math> propagator in momentum yields the familiar <math>1/r^2</math> factor in coordinate space. However, higher orders do contribute and deviations from the <math>1/r^2</math> law thus occur. This extra r-dependence is folded in the coupling which then acquires a scale dependence."
Pointers from Richard Imlay circa 1983
When I asked Richard Imlay (high-energy experimentalist at LSU) for a reference to high-energy physics articles in which quark-quark interactions have been expressed in terms of a radially dependent (e.g., logarithmic ) potential, he pointed me to the following:
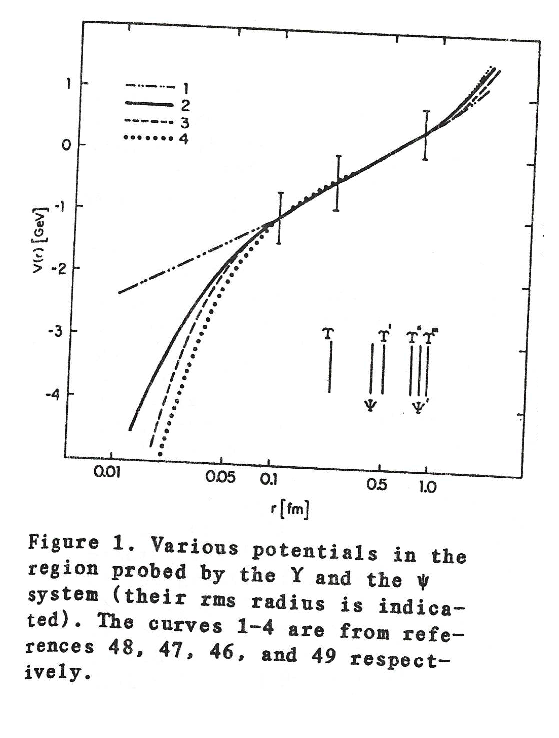
- Quigg, C. & Rosner, J. L. (1977), Physics Letters, 71B, pp. 153-157, Quarkonium level spacings
- Tuts, P. Michael (1983), Proceedings of the 1983 International Symposium on Lepton and Photon Interactions at High Energies, edited by D. G. Cassel & D. Kreinick (Cornell University, Ithaca, 1983), pp. 284-327, Experimental results in heavy quarkonia
| Figure 1 from Tuts (1983) | |
|---|---|
|
References Cited in Figure Caption:
|
|
Additionally, my handwritten notes, circa 1983, point to:
- S. M. Alladin & K. S. V. S. Narasimhan (1982), Physics Reports, 92 (#6), pp. 339 - 397, Gravitational interactions between galaxies — in 2018, this does not now seem relevant.
- J. Gasser & H. Leutwyler (1982), Physics Reports, 87, Issue 3, pp. 77 - 169, Quark masses
- G. Altarelli (1982), Physics Reports, 81, Issue 1, pp. 1 - 129. Partons in quantum chromodynamics
Cosmologies
Standard Presentation
-
Derivation of the Friedmann Equations in the context of our discussion of Newtonian free-fall collapse.
Newtonian Description of Pressure-Free Collapse
<math>~\biggl( \frac{\dot{R}}{R} \biggr)^2</math>
<math>~=</math>
<math>~\frac{8}{3}\pi G \rho - \frac{k(R_i, v_i)}{R^2} \, ,</math>
<math>~\frac{\ddot{R}}{R}</math>
<math>~=</math>
<math>~- \frac{4}{3}\pi G \rho \, ,</math>
where, <math>~k(R_i,v_i)</math>
<math>~=</math>
<math>~\frac{8}{3}\pi G \rho_i R_i^2 - v_i^2 \, .</math>
-
Frieman, Turner & Huterer (2008, ARAA, 46, 385 - 432) provide an excellent, very readable review of dark matter and dark energy in the context of various cosmologies; see also, chapter 29 of Carroll & Ostlie (2007, 2nd Edition). Their equations (2) and (3) are written in the following table — with factors of <math>~c^2</math> inserted to explicitly clarify how the dimensional units are the same for every term in each equation.
Friedmann equations:
Field equations of GR applied to the FRW metric<math>~H^2 = \biggl( \frac{\dot{a}}{a} \biggr)^2</math>
<math>~=</math>
<math>~\frac{8}{3}\pi G \rho - \frac{k}{a^2} + \frac{\Lambda c^2}{3}\, ,</math>
<math>~\frac{\ddot{a}}{a}</math>
<math>~=</math>
<math>~- \frac{4}{3}\pi G \biggl[\rho + \frac{3p}{c^2} \biggr] + \frac{\Lambda c^2}{3} \, .</math>
ASTR4422 Class Notes
Homework set #3 that was assigned to my ASTR4422 class in the spring of 2005 explored how solutions to the Newtonian free-fall collapse problem can be mapped directly to cosmological models of the expanding universe. The stated objective was to match the "closed universe," <math>~\Omega_0 = 2</math> model presented in Figure 27.4 (p. 1230) of the 1st edition of Carroll & Ostlie. (In the spring of 2009, this was assignment #5, and the aim was to match Figure 29.5 from the 2nd edition of Carroll & Ostlie.)
In the free-fall model, the collapse starts from rest at initial radius and density, <math>~r_0</math> and <math>~\rho_0</math>, respectively, in which case — see, for example, our discussion of the role of the integration constant —
|
<math>~k_i</math> |
<math>~=</math> |
<math>~\frac{2G}{r_i} \biggl[ \frac{4}{3} \pi \rho_i r_i^3 \biggr] \, .</math> |
Hence, we have,
|
<math>~H^2 = \biggl( \frac{\dot{R}}{R} \biggr)^2</math> |
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho - \frac{2G}{r_i} \biggl[ \frac{4}{3} \pi \rho_i r_i^3 \biggr] \frac{1}{R^2}</math> |
|
|
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ \frac{\rho}{\rho_i} - \frac{r_i^2}{R^2} \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ \biggl(\frac{r_i}{R}\biggr)^3 - \biggl(\frac{r_i}{R}\biggr)^2 \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ \sec^6\zeta - \sec^4\zeta \biggr] \, .</math> |
Now, adopting the terminologies, <math>~\Omega \equiv \rho/\rho_\mathrm{crit}</math> and, for any <math>~H</math>, <math>~\rho_\mathrm{crit} \equiv 3H^2/(8\pi G) ~~\Rightarrow ~~ H^2 = 8\pi G \rho/(3\Omega)</math>, we have,
|
<math>~\frac{8\pi G \rho}{3\Omega}</math> |
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ \sec^6\zeta - \sec^4\zeta \biggr]</math> |
|
<math>~\Rightarrow ~~~\frac{1}{\Omega}</math> |
<math>~=</math> |
<math>~\frac{\rho_i}{\rho} \biggl[ \sec^6\zeta - \sec^4\zeta \biggr] = 1 - \cos^2\zeta \, .</math> |
Hence, if in the present epoch [denoted by subscript 0], <math>~\Omega = \Omega_0 = 2</math> (as in the Carroll & Ostlie figure that we're trying to match), then in our "free-fall" model, the present epoch occurs at the dimensionless time given by,
|
<math>~1 - \cos^2\zeta_0</math> |
<math>~=</math> |
<math>~\frac{1}{2}</math> |
|
<math>~\Rightarrow ~~~ \cos^2\zeta_0</math> |
<math>~=</math> |
<math>~\frac{1}{2}</math> |
|
<math>~\Rightarrow ~~~ \zeta_0</math> |
<math>~=</math> |
<math>~\frac{\pi}{4} \, .</math> |
This, in turn, implies that,
|
<math>~H_0^2</math> |
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ \sec^6\zeta_0 - \sec^4\zeta_0 \biggr] </math> |
|
|
<math>~=</math> |
<math>~\frac{8}{3}\pi G \rho_i \biggl[ 2^3 - 2^2\biggr] = \frac{32}{3}\pi G \rho_i </math> |
|
|
<math>~=</math> |
<math>~ \frac{1}{\tau_\mathrm{ff}^2} \biggl[\frac{3\pi}{32G\rho_i}\biggr] \frac{32}{3}\pi G \rho_i = \biggl(\frac{\pi}{\tau_\mathrm{ff}} \biggr)^2 \, .</math> |
As our parametric solution of the Newtonian free-fall problem details, quite generally we can write,
|
<math>~t</math> |
<math>~=</math> |
<math>~\frac{2\tau_\mathrm{ff}}{\pi} \biggl[ \zeta + \frac{1}{2}\sin(2\zeta)\biggr]</math> |
|
|
<math>~=</math> |
<math>~\frac{2}{\pi} \biggl[\frac{3\pi}{32G\rho_i} \biggr]^{1 / 2} \biggl[ \zeta + \frac{1}{2}\sin(2\zeta)\biggr]</math> |
With Logarithmic Potential Included
Let's return to the Newtonian expression for the acceleration equation and replace the time-dependent density, <math>~\rho</math>, with the time-independent mass, that is,
|
<math>~\frac{\ddot{R}}{R}</math> |
<math>~=</math> |
<math>~- \frac{4}{3} ~\pi G\rho = - \frac{GM_R}{R^3} </math> |
|
<math>~\Rightarrow ~~~ \ddot{R}</math> |
<math>~=</math> |
<math>~- \frac{GM_R}{R^2} \, .</math> |
This is the form of the equation that has been integrated analytically in our separate discussion of Newtonian free-fall collapse. Now, in our published speculation about a modified force-law to explain flat rotation curves, we proposed (see that publication's equation 1) a gravitational acceleration of the form,
|
<math>~\ddot{R}</math> |
<math>~=</math> |
<math>~- \frac{GM_R}{R^2} \biggl[1 + \frac{R}{a_\mathrm{T}}\biggr] \, .</math> |
This was intended to represent the modified gravitational acceleration felt by a (massless) test particle moving outside of a point-mass, <math>~M_R</math>. When considering a position inside of a spherical mass distribution whose radius, <math>~R_2 > R</math>, the first term remains the same because material outside of the location, <math>~R</math>, does not exert a net gravitational acceleration. But the second term cannot be treated that way. Following our separate discussion of a 1/r force law, we propose the following acceleration due to such an extended mass source:
|
<math>~\ddot{R}</math> |
<math>~=</math> |
<math>~ - \frac{G}{R^2} \biggl[\frac{4}{3}\pi \rho R^3\biggr] - \frac{G}{a_T} \biggl[ \frac{4}{3}\pi\rho R_2\biggr] R \biggl\{ 1 - 3 \sum_{n=1}^{\infty} \biggl( \frac{R}{R_2} \biggr)^{2n} \biggl[(2n-1)(2n+1)(2n+3) \biggr]^{-1} \biggr\} \, . </math> |
Furthermore, let's equate <math>~R_2</math> with the "size of the universe," namely, <math>~ct</math>; and let's again define the mass inside of the Lagrangian <math>~R</math> as <math>~M_R</math>. Then we have,
|
<math>~\ddot{R}</math> |
<math>~=</math> |
<math>~ - \frac{GM_R}{R^2} - \frac{GM_R }{R^2} \biggl( \frac{R_2}{a_T}\biggr) \biggl\{ 1 - 3 \sum_{n=1}^{\infty} \biggl( \frac{R}{R_2} \biggr)^{2n} \biggl[(2n-1)(2n+1)(2n+3) \biggr]^{-1} \biggr\} </math> |
|
|
<math>~=</math> |
<math>~ - \frac{GM_R}{R^2} - \frac{GM_R }{R^2} \biggl( \frac{ct}{a_T}\biggr) \biggl\{ 1 - 3 \sum_{n=1}^{\infty} \biggl( \frac{R}{ct} \biggr)^{2n} \biggl[(2n-1)(2n+1)(2n+3) \biggr]^{-1} \biggr\} \, . </math> |
Insert Dependence on (Energy) Density
The QGP is a regime where the interaction between quarks and gluons is dominated by the Coulomb-like term in the interaction potential. The particles interact with one another as though they are not confined; this is the so-called asymptotically free regime. Generally speaking, a QGP is achieved in a very high energy-density environment.
We can mimic this behavior in our modified cosmology by assuming that the coefficient on the <math>1/r</math> term in the gravitational acceleration varies with the energy-density of the fluid. (More simply, let's have it vary with the mass-dentiy.) We want to kill off the <math>1/r</math> term when the density climbs above some threshold, <math>\rho_H</math>. Let's try …
| <math>\ddot{R}</math> | <math>=</math> |
<math>- \frac{GM}{R^2} \biggl\{ 1 + \biggl[\frac{\rho}{\rho_H} - 1\biggr]^{-2} \frac{R}{a_T} \biggr\} \, .</math> |
Note that the <math>~R-</math>dependent potential from which this expression for the acceleration is derived is,
| <math>~\Phi(R)</math> | <math>=</math> |
<math>+ \frac{GM}{R} - \frac{GM_r}{a_T} \biggl[\frac{\rho}{\rho_H} - 1\biggr]^{-2} \ln \biggl(\frac{R}{a_T}\biggr) \, .</math> |
This expression for the gravitational acceleration has the desired properties:
- In the early universe, when <math>\rho/\rho_H \gg 1</math>, the density-dependent coefficient of the second (confining) term goes to zero; we have an asymptotically free regime in which a Coulomb-like potential dominates throughout the universe.
- As the universe expands, the density will steadily drop. For <math>\rho/\rho_H \ll 1</math>, the density-dependent coefficient of the confining term approaches unity and we retrieve our originally proposed, modified cosmology; that is, the potential is dominated by a logarithmic term for all distances greater than <math>~a_T</math>.
Potentially Useful References
- Wikipedia — Semiempirical Formula for the Nuclear Binding Energy
- Walecka, John Dirk, Theoretical Nuclear and Subnuclear Physics, World Scientific (2004)

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |