Difference between revisions of "User:Tohline/SSC/VariationalPrinciple"
| Line 331: | Line 331: | ||
</div> | </div> | ||
So, given that a mapping from our notation to that used by Ledoux & Walraven (1958) requires <math>~ | So, given that a mapping from our notation to that used by Ledoux & Walraven (1958) requires <math>~xe^{i\omega t} \rightarrow \zeta/r_0</math>, I understand the origins of their equations (59.1) and (59.2). But I do not yet understand how … <font color="darkgreen">"Accordingly, the acting forces per unit volume can be considered as deriving from a potential density"</font> | ||
<div align="center"> | <div align="center"> | ||
<table border="0" cellpadding="5" align="center"> | <table border="0" cellpadding="5" align="center"> | ||
| Line 373: | Line 373: | ||
</table> | </table> | ||
</div> | </div> | ||
Noting that, <math>~\dot\zeta = i\omega r_0 x e^{i\omega t}</math>, this in turn gives, | |||
<div align="center"> | |||
<table border="0" cellpadding="5" align="center"> | |||
<tr> | |||
<td align="right"> | |||
<math>~L \equiv \int_0^R 4\pi r_0^2 \mathcal{L} dr_0</math> | |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 4\pi \int_0^R \biggl\{ \frac{\rho_0}{2} {\dot\zeta}^2 | |||
+ \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 4\pi e^{2i\omega t} \int_0^R \biggl\{ \frac{\rho_0}{2} (i \omega r_0 x)^2 | |||
+ \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 (r_0 x)^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^3 x)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^2 x^2 | |||
- 4 r_0 x^2 \frac{dP_0}{dr_0} - \frac{\gamma_\mathrm{g} P_0}{r_0^4} \biggl[ 3r_0^2 x + r_0^3 \frac{\partial x}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2 | |||
- \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 | |||
- 4 r_0^3 x^2 \frac{dP_0}{dr_0} | |||
- 3\gamma_\mathrm{g} P_0 \biggl[ 3r_0^2 x^2 + 2r_0^3 x \frac{\partial x}{\partial r_0} \biggr] \biggr\}dr_0 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2 | |||
- \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 | |||
- 4 r_0^3 x^2 \frac{dP_0}{dr_0} | |||
+r_0^3 x^2 \frac{d}{dr_0}\biggl(3\gamma_\mathrm{g}P_0\biggr) | |||
-\frac{d}{dr_0}\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr] | |||
\biggr\}dr_0 | |||
</math> | |||
</td> | |||
</tr> | |||
<tr> | |||
<td align="right"> | |||
| |||
</td> | |||
<td align="center"> | |||
<math>~=</math> | |||
</td> | |||
<td align="left"> | |||
<math>~ 2\pi e^{2i\omega t} \biggl\{ | |||
- \int_0^R \rho_0 \omega^2 r_0^4 x^2 dr_0 | |||
- \int_0^R \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0 | |||
+ \int_0^R \rho_0 r_0^3 x^2 \frac{d}{dr_0}\biggl[ (3\gamma_\mathrm{g} - 4)P_0\biggr]dr_0 | |||
-\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr]_0^{R} | |||
\biggr\} \, . | |||
</math> | |||
</td> | |||
</tr> | |||
</table> | |||
</div> | |||
The group of terms inside the curly braces, here, matches the group of terms inside the curly braces of Ledoux & Walraven's equation (59.8) if we acknowledge that: | |||
# Our <math>~\omega^2</math> has the same meaning, but the opposite sign, as their <math>~\sigma^2</math>. | |||
# Our last term goes to zero because, <math>~r_0 = 0</math> at the center, and <math>~P_0 = 0</math> at the surface. | |||
==Chandrasekhar (1964)== | ==Chandrasekhar (1964)== | ||
Revision as of 16:39, 29 May 2017
Ledoux's Variational Principle

|
|---|
| | Tiled Menu | Tables of Content | Banner Video | Tohline Home Page | |
All of the discussion in this chapter will build upon our derivation elsewhere of the,
LAWE: Linear Adiabatic Wave (or Radial Pulsation) Equation
|
<math>~ \frac{d^2x}{dr_0^2} + \biggl[\frac{4}{r_0} - \biggl(\frac{g_0 \rho_0}{P_0}\biggr) \biggr] \frac{dx}{dr_0} + \biggl(\frac{\rho_0}{\gamma_\mathrm{g} P_0} \biggr)\biggl[\omega^2 + (4 - 3\gamma_\mathrm{g})\frac{g_0}{r_0} \biggr] x = 0 </math> |
We will draw heavily from the paper published by Ledoux & Pekeris (1941), and from pp. 458-474 of the review by P. Ledoux & Th. Walraven (1958) in explaining how the variational principle can be used to identify the eigenvector of the fundamental mode of radial oscillation in spherically symmetric configurations.
Ledoux and Pekeris (1941)
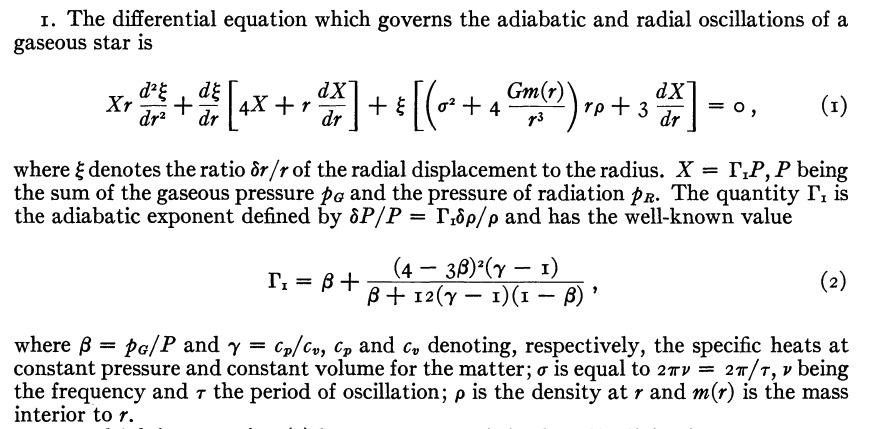
Historically, by the 1940s, the LAWE was a relatively familiar one to astrophysicists. For example, the opening paragraph of a 1941 paper by Ledoux & Pekeris (1941, ApJ, 94, 124), reads:
|
Paragraph extracted from P. Ledoux & C. L. Pekeris (1941)
"Radial Pulsations of Stars"
ApJ, vol. 94, pp. 124-135 © American Astronomical Society |
If we divide their equation (1) through by <math>~Xr = \Gamma_1 P r</math> and recognize that,
<math> \frac{dX}{dr} = \frac{dX}{dm}\frac{dm}{dr} = - \Gamma_1 g_0 \rho \, , </math>
we obtain,
<math> \frac{d^2\xi}{dr^2} + \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\frac{\rho}{\Gamma_1 P} \biggl[ \sigma^2 + (4 - 3\Gamma_1) \frac{g_0}{r} \biggr] \xi = 0 \, . </math>
Clearly, this 2nd-order, ordinary differential equation is the same as our derived LAWE, but with a more general definition of the adiabatic exponent that allows consideration of a situation where the total pressure is a sum of both gas and radiation pressure.
Multiplying this last equation through by <math>~\Gamma_1 P r^4</math>, and recognizing that,
|
<math>~(r^4 \Gamma_1 P)\frac{d^2\xi}{dr^2} </math> |
<math>~=</math> |
<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr] </math> |
we can write,
|
<math>~0</math> |
<math>~=</math> |
<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \frac{d\xi}{dr} \cdot \frac{d}{dr} \biggl[ r^4 \Gamma_1 P\biggr] + ( \Gamma_1 P r^4 ) \biggl[ \frac{4}{r} - \frac{g_0 \rho}{P} \biggr] \frac{d\xi}{dr} +\rho \biggl[ \sigma^2 r^4 + (4 - 3\Gamma_1) g_0 r^3\biggr] \xi </math> |
|
|
<math>~=</math> |
<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] - \biggl[4r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr} \biggr] \frac{d\xi}{dr} + \biggl[ 4 r^3\Gamma_1 P + \Gamma_1 r^4 \frac{dP}{dr}\biggr] \frac{d\xi}{dr} +\biggl[ \sigma^2 \rho r^4 - (4 - 3\Gamma_1) r^3 \frac{dP}{dr} \biggr] \xi </math> |
|
|
<math>~=</math> |
<math>~ \frac{d}{dr}\biggl[ r^4 \Gamma_1 P ~\frac{d\xi}{dr} \biggr] +\biggl[ \sigma^2 \rho r^4 + (3\Gamma_1 - 4) r^3 \frac{dP}{dr} \biggr] \xi </math> |
|
|
<math>~=</math> |
<math>~ \frac{d}{dr}\biggl[ \Gamma_1 P r^4 ~\frac{d\xi}{dr} \biggr] +\biggl[ \sigma^2 r^4 \rho + 4 Gm (r ) r \rho + 3\Gamma_1 r^3 \frac{dP}{dr} \biggr] \xi \, . </math> |
Assuming that <math>~\Gamma_1</math> is uniform throughout the configuration, this next-to-last expression is identical to equation (58.1) of Ledoux & Walraven (1958), while the last expression is the same as equation (3) of Ledoux & Pekeris (1941)
Review by Ledoux and Walraven
Here we are especially interested in understanding the origin of equation (59.10) of P. Ledoux & Th. Walraven (1958), which appears in §59 (pp. 464 - 466) of their Handbuch der Physik article.
From our accompanying summary of the set of nonlinear governing relations, we highlight the
Euler + Poisson Equations
<math>\frac{d^2 r}{dt^2} = - 4\pi r^2 \frac{dP}{dm} - \frac{Gm}{r^2} </math>
Repeating a result from our separate derivation, linearization of the two terms on the righthand side of this equation gives,
|
<math> r^2 \frac{dP}{dm} </math> |
<math> \rightarrow </math> |
<math> r_0^2 \biggl[1 + x~ e^{i\omega t} \biggr]^2 \biggl\{\frac{dP_0}{dm} \biggl[1 + p~ e^{i\omega t} \biggr] + P_0~e^{i\omega t} \frac{dp}{dm} \biggr\} \approx r_0^2 \frac{dP_0}{dm} \biggl[1 + (2x+p)~ e^{i\omega t} \biggr] + P_0 r_0^2~e^{i\omega t} \frac{dp}{dm} </math> |
|
<math> \frac{Gm}{r^2} </math> |
<math> \rightarrow </math> |
<math> \frac{Gm}{ r_0^2} \biggl[1 + x~ e^{i\omega t} \biggr]^{-2} \approx \frac{Gm}{ r_0^2} \biggl[1 -2 x~ e^{i\omega t} \biggr] \, . </math> |
Adopting the terminology of Ledoux & Walraven (1958), the "variation" of each of these terms is obtained by subtracting off the leading order pieces — which presumably cancel in equilibrium. In particular, drawing a parallel with their equation (59.1), we can write,
|
<math> ~\delta \biggl( - \frac{Gm}{r^2} \biggr) </math> |
<math> ~\approx </math> |
<math> \frac{Gm}{ r_0^2} \biggl[2 x~ e^{i\omega t} \biggr] \, . </math> |
And, drawing a parallel with their equation (59.2), we have,
|
<math> ~\delta \biggl( - \frac{1}{\rho} \cdot \frac{dP}{dr} \biggr) = \delta \biggl( - 4\pi r^2 \frac{dP}{dm} \biggr) </math> |
<math> ~\approx </math> |
<math> -4\pi r_0^2 \frac{dP_0}{dm} \biggl[(2x+p)~ e^{i\omega t} \biggr] - 4\pi P_0 r_0^2~e^{i\omega t} \frac{dp}{dm} </math> |
|
|
<math> ~= </math> |
<math>~\biggl\{ \frac{Gm}{r_0^2}\biggl[(2x)\biggr] -4\pi r_0^2 \frac{dP_0}{dm} \biggl[(p) \biggr] - 4\pi P_0 r_0^2 \frac{dp}{dm} \biggr\} e^{i\omega t} </math> |
|
|
<math> ~= </math> |
<math>~\biggl\{ \biggl( \frac{2 Gm}{r_0^2}\biggr) x -\frac{1}{\rho_0} \cdot \frac{d}{dr_0} \biggl[ P_0 p \biggr] \biggr\} e^{i\omega t} \, . </math> |
Now, if we combined the linearized continuity equation and the linearized (adiabatic form of the) first law of thermodynamics, as derived elsewhere, we can write,
|
<math>~p = \gamma_\mathrm{g} d</math> |
<math>~=</math> |
<math>~- \gamma_\mathrm{g} \biggl[ 3x + r_0 \frac{dx}{dr_0} \biggr] </math> |
|
|
<math>~=</math> |
<math>~- \frac{\gamma_\mathrm{g}}{r_0^2} \frac{d}{dr_0} \biggl( r_0^3 x \biggr) \, . </math> |
Hence,
|
<math> ~\delta \biggl( - \frac{1}{\rho} \cdot \frac{dP}{dr} \biggr) </math> |
<math> ~\approx </math> |
<math>~\biggl\{ \biggl( \frac{2 Gm}{r_0^2}\biggr) x +\frac{1}{\rho_0} \cdot \frac{d}{dr_0} \biggl[ \frac{\gamma_\mathrm{g} P_0}{r_0^2} \frac{d}{dr_0} \biggl( r_0^3 x \biggr) \biggr] \biggr\} e^{i\omega t} \, . </math> |
So, given that a mapping from our notation to that used by Ledoux & Walraven (1958) requires <math>~xe^{i\omega t} \rightarrow \zeta/r_0</math>, I understand the origins of their equations (59.1) and (59.2). But I do not yet understand how … "Accordingly, the acting forces per unit volume can be considered as deriving from a potential density"
|
<math>~\rho_0 \mathcal{V}</math> |
<math>~=</math> |
<math>~ - \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 + \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \, . </math> |
It is clear that, once I understand the origin of this expression for the potential density, I will understand how the "Lagrangian density" as defined by their equation (47.8), viz.,
<math>~\mathcal{L} = \rho_0 [\mathcal{K} - \mathcal{V}] \, ,</math>
becomes (see their equation 59.5),
|
<math>~\mathcal{L}</math> |
<math>~=</math> |
<math>~\frac{\rho_0}{2} {\dot\zeta}^2 + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \, . </math> |
Noting that, <math>~\dot\zeta = i\omega r_0 x e^{i\omega t}</math>, this in turn gives,
|
<math>~L \equiv \int_0^R 4\pi r_0^2 \mathcal{L} dr_0</math> |
<math>~=</math> |
<math>~ 4\pi \int_0^R \biggl\{ \frac{\rho_0}{2} {\dot\zeta}^2 + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 \zeta^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^2 \zeta)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 </math> |
|
|
<math>~=</math> |
<math>~ 4\pi e^{2i\omega t} \int_0^R \biggl\{ \frac{\rho_0}{2} (i \omega r_0 x)^2 + \biggl( \frac{2Gm}{r_0^3}\biggr) \rho_0 (r_0 x)^2 - \frac{\gamma_\mathrm{g} P_0}{2} \biggl[ \frac{1}{r_0^2} \frac{\partial(r_0^3 x)}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 </math> |
|
|
<math>~=</math> |
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^2 x^2 - 4 r_0 x^2 \frac{dP_0}{dr_0} - \frac{\gamma_\mathrm{g} P_0}{r_0^4} \biggl[ 3r_0^2 x + r_0^3 \frac{\partial x}{\partial r_0} \biggr]^2 \biggr\}r_0^2 dr_0 </math> |
|
|
<math>~=</math> |
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2 - \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 - 4 r_0^3 x^2 \frac{dP_0}{dr_0} - 3\gamma_\mathrm{g} P_0 \biggl[ 3r_0^2 x^2 + 2r_0^3 x \frac{\partial x}{\partial r_0} \biggr] \biggr\}dr_0 </math> |
|
|
<math>~=</math> |
<math>~ 2\pi e^{2i\omega t} \int_0^R \biggl\{ -\rho_0 \omega^2 r_0^4 x^2 - \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 - 4 r_0^3 x^2 \frac{dP_0}{dr_0} +r_0^3 x^2 \frac{d}{dr_0}\biggl(3\gamma_\mathrm{g}P_0\biggr) -\frac{d}{dr_0}\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr] \biggr\}dr_0 </math> |
|
|
<math>~=</math> |
<math>~ 2\pi e^{2i\omega t} \biggl\{ - \int_0^R \rho_0 \omega^2 r_0^4 x^2 dr_0 - \int_0^R \gamma_\mathrm{g} P_0 r_0^4\biggl( \frac{\partial x}{\partial r_0}\biggr)^2 dr_0 + \int_0^R \rho_0 r_0^3 x^2 \frac{d}{dr_0}\biggl[ (3\gamma_\mathrm{g} - 4)P_0\biggr]dr_0 -\biggl[3 \gamma_\mathrm{g} r_0^3 x^2 P_0\biggr]_0^{R} \biggr\} \, . </math> |
The group of terms inside the curly braces, here, matches the group of terms inside the curly braces of Ledoux & Walraven's equation (59.8) if we acknowledge that:
- Our <math>~\omega^2</math> has the same meaning, but the opposite sign, as their <math>~\sigma^2</math>.
- Our last term goes to zero because, <math>~r_0 = 0</math> at the center, and <math>~P_0 = 0</math> at the surface.
Chandrasekhar (1964)
S. Chandrasekhar (1964, ApJ, 139, 664)
See Also

|
|---|
|
© 2014 - 2021 by Joel E. Tohline |