BiPolytrope with <math>n_c = 1</math> and <math>n_e=5</math>

Here we construct a bipolytrope in which the core has an <math>~n_c=1</math> polytropic index and the envelope has an <math>~n_e=5</math> polytropic index. As in the case of our separately discussed, "mirror image" bipolytropes having <math>~(n_c, n_e) = (5, 1)</math>, this system is particularly interesting because the entire structure can be described by closed-form, analytic expressions. Bipolytropes of this type were first constructed by J. O. Murphy (1983, Proc. Astr. Soc. of Australia, 5, 175), and attributes of their physical structure were further discussed by J. O. Murphy & R. Fiedler (1985a, Proc. Astr. Soc. of Australia, 6, 219); additional, closely related references are given below. In the discussion that follows, we will be heavily referencing Murphy's (1983) work.

Steps 2 & 3

Based on the discussion presented elsewhere of the structure of an isolated <math>n=1</math> polytrope, the core of this bipolytrope will have the following properties:

<math> \theta(\xi) = \frac{\sin\xi}{\xi} ~~~~\Rightarrow ~~~~ \theta_i = \frac{\sin\xi_i}{\xi_i} ; </math>

<math> \frac{d\theta}{d\xi} = \biggl[ \frac{\cos\xi}{\xi}- \frac{\sin\xi}{\xi^2}\biggr] ~~~~\Rightarrow ~~~~ \biggl(\frac{d\theta}{d\xi}\biggr)_i = \biggl[\frac{\cos\xi_i}{\xi_i}- \frac{\sin\xi_i}{\xi_i^2} \biggr] \, . </math>

The first zero of the function <math>~\theta(\xi)</math> and, hence, the surface of the corresponding isolated <math>~n=1</math> polytrope is located at <math>~\xi_s = \pi</math>. Hence, the interface between the core and the envelope can be positioned anywhere within the range, <math>~0 < \xi_i < \pi</math>.

Step 4: Throughout the core (<math>0 \le \xi \le \xi_i</math>)

Specify: <math>~K_c</math> and <math>~\rho_0 ~\Rightarrow</math>
<math>~\rho</math>	<math>~=</math>	<math>\rho_0 \theta^{n_c}</math>	<math>~=</math>	<math>~\rho_0 \biggl( \frac{\sin\xi}{\xi} \biggr)</math>
<math>~P</math>	<math>~=</math>	<math>~K_c \rho_0^{1+1/n_c} \theta^{n_c + 1}</math>	<math>~=</math>	<math>~K_c \rho_0^{2} \biggl( \frac{\sin\xi}{\xi}\biggr)^{2}</math>
<math>~r</math>	<math>~=</math>	<math>~\biggl[ \frac{(n_c + 1)K_c}{4\pi G} \biggr]^{1/2} \rho_0^{(1-n_c)/(2n_c)} \xi</math>	<math>~=</math>	<math>~\biggl[ \frac{K_c}{2\pi G} \biggr]^{1/2} \xi</math>
<math>~M_r</math>	<math>~=</math>	<math>~4\pi \biggl[ \frac{(n_c + 1)K_c}{4\pi G} \biggr]^{3/2} \rho_0^{(3-n_c)/(2n_c)} \biggl(-\xi^2 \frac{d\theta}{d\xi} \biggr)</math>	<math>~=</math>	<math>~4\pi \biggl[ \frac{K_c}{2\pi G} \biggr]^{3/2} \rho_0 \biggl[\sin\xi - \xi \cos\xi \biggr]</math>

Step 5: Interface Conditions

			Setting <math>~n_c=1</math>, <math>~n_e=5</math>, and <math>~\phi_i = \phi_i ~~~~\Rightarrow</math>
<math>~\frac{\rho_e}{\rho_0}</math>	<math>~=</math>	<math>~\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta^{n_c}_i \phi_i^{-n_e}</math>	<math>~=</math>	<math>~\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta_i \phi_i^{-5}</math>
<math>~\biggl( \frac{K_e}{K_c} \biggr) </math>	<math>~=</math>	<math>~\rho_0^{1/n_c - 1/n_e}\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-(1+1/n_e)} \theta^{1 - n_c/n_e}_i</math>	<math>~=</math>	<math>~\biggl[\rho_0^{4}\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-6} \theta^{4}_i\biggr]^{1/5}</math>
<math>~\frac{\eta_i}{\xi_i}</math>	<math>~=</math>	<math>~\biggl[ \frac{n_c + 1}{n_e+1} \biggr]^{1/2} \biggl( \frac{\mu_e}{\mu_c}\biggr) \theta_i^{(n_c-1)/2} \phi_i^{(1-n_e)/2}</math>	<math>~=</math>	<math>~\biggl( \frac{1}{3} \biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c}\biggr) \phi_i^{-2}</math>
<math>~\biggl( \frac{d\phi}{d\eta} \biggr)_i</math>	<math>~=</math>	<math>~\biggl[ \frac{n_c + 1}{n_e + 1} \biggr]^{1/2} \theta_i^{- (n_c + 1)/2} \phi_i^{(n_e+1)/2} \biggl( \frac{d\theta}{d\xi} \biggr)_i</math>	<math>~=</math>	<math>~\biggl( \frac{1}{3} \biggr)^{1/2} \theta_i^{- 1} \biggl( \frac{d\theta}{d\xi} \biggr)_i \phi_i^3</math>

Alternative: In our introductory description of how to build a bipolytropic structure, we pointed out that, instead of employing these last two fitting conditions, Chandrasekhar [C67] found it useful to employ, instead, the ratio of the <math>3^\mathrm{rd}</math> to <math>4^\mathrm{th}</math> expressions, which in the present case produces,

<math> \frac{\eta_i \phi_i^{5}}{(d\phi/d\eta)_i} = \frac{\xi_i \theta_i}{(d\theta/d\xi)_i} \biggl( \frac{\mu_e}{\mu_c}\biggr) \, , </math>

and the product of the <math>3^\mathrm{rd}</math> and <math>4^\mathrm{th}</math> expressions, which in the present case generates,

<math> \frac{3\eta_i (d\phi/d\eta)_i}{ \phi_i } = \frac{\xi_i (d\theta/d\xi)_i}{ \theta_i } \biggl( \frac{\mu_e}{\mu_c}\biggr) \, . </math>

As is explained in an accompanying discussion, Murphy (1983) followed Chandrasekhar's lead and extracted fitting conditions from this last pair of expressions. In seeking the most compact analytic solution, we have found it advantageous to combine our standard <math>3^\mathrm{rd}</math> fitting expression with the last (i.e., the product) expression identified by Chandrasekhar.

Step 6: Envelope Solution

Following the work of Murphy (1983) and of Murphy & Fiedler (1985a), we will adopt for the envelope's structure the F-Type solution of the <math>~n=5</math> Lane-Emden function discovered by S. Srivastava (1968, ApJ, 136, 680) and described in an accompanying discussion, namely,

<math>~\phi</math>	<math>~=</math>	<math>~\frac{B_0^{-1}\sin[\ln(A_0\eta)^{1/2})]}{\eta^{1/2}\{3-2\sin^2[\ln(A_0\eta)^{1/2}]\}^{1/2}} </math>
	<math>~=</math>	<math>~\frac{B_0^{-1}\sin\Delta}{\eta^{1/2}(3-2\sin^2\Delta)^{1/2}} \, ,</math>

where <math>~A_0</math> is a "homology factor" and <math>~B_0</math> is an overall scaling coefficient — the values of both will be determined presently from the interface conditions — and we have introduced the notation,

<math>~\Delta \equiv \ln(A_0\eta)^{1/2} = \frac{1}{2} (\ln A_0 + \ln\eta) \, .</math>

The first derivative of Srivastava's function is,

<math>~ \frac{B_0^{-1}[3\cos\Delta-3\sin\Delta + 2\sin^3\Delta] }{2\eta^{3/2}(3-2\sin^2\Delta)^{3/2}} \, . </math>

As has been explained in the context of our more general discussion of Srivastava's function, if we ignore, for the moment, the additional "<math>m\pi</math>" phase shift that can be attached to a determination of the angle, <math>~\Delta</math>, the physically viable interval for the dimensionless radial coordinate is, <math>~e^{2\pi} \ge A_0\eta \ge \eta_\mathrm{crit} \equiv e^{2\tan^{-1}(1+2^{1/3})} \, .</math>

For this bipolytropic configuration, it is worth emphasizing how the dimensionless radial coordinate of the <math>~n_e = 5</math> envelope, <math>~\eta</math>, is related to the dimensionless radial coordinate of the <math>~n_c = 1</math> core, <math>~\xi</math>. Referring to the general setup procedure for constructing any bipolytropic configuration that has been presented in tabular form in a separate discussion, it is clear that in order for the radial coordinate, <math>~r</math>, to carry a consistent meaning throughout the model, we must have,

<math>~r = \biggl[ \frac{(n_c + 1)K_c}{4\pi G} \biggr]^{1/2} \rho_0^{(1-n_c)/(2n_c)} \xi</math>	<math>~=</math>	<math>~\biggl[ \frac{(n_e + 1)K_e}{4\pi G} \biggr]^{1/2} \rho_e^{(1-n_e)/(2n_e)} \eta</math>
<math>~\Rightarrow ~~~~\biggl( \frac{K_c}{3K_e} \biggr)\rho_e^{4/5} </math>	<math>~=</math>	<math>~\biggl(\frac{\eta}{\xi}\biggr)^2 \, .</math>

Referring back to the already established interface conditions, above, to relate <math>~\rho_e</math> to <math>~\rho_0</math>, and to re-express the ratio, <math>~K_e/K_c</math>, we therefore have,

<math>~\biggl(\frac{\eta}{\xi}\biggr)^2</math>	<math>~=</math>	<math>~\frac{1}{3} \biggl[ \rho_0^{4}\biggl( \frac{\mu_e}{\mu_c} \biggr)^{-6} \theta^{4}_i\biggr]^{-1/5} \biggl[ \rho_0\biggl( \frac{\mu_e}{\mu_c} \biggr) \theta_i \phi_i^{-5} \biggr]^{4/5} </math>
<math>~\Rightarrow ~~~~ \frac{\eta}{\xi}</math>	<math>~=</math>	<math>~\biggl(\frac{1}{3}\biggr)^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr) \phi_i^{-2} \, .</math>

While this result is not a surprise because the right-hand-side is the same expression that was presented, above, as the interface condition for the ratio, <math>~\eta_i/\xi_i</math>, it is nevertheless useful because it shows that the same relation works throughout the system — not just at the interface — and it clearly defines how we can swap back and forth between the two dimensionless radial coordinates when examining the structure and characteristics of this composite bipolytropic structure.

First Constraint

Calling upon Chandrasekhar's "product" expression, as just defined above — see also our accompanying discussion for elaboration on Murphy's (1983) "V_5F" and "V_1E" function notations — one fitting condition at the interface is,

<math>~- \frac{2\xi_i }{ 3\theta_i } \biggl( \frac{d\theta}{d\xi} \biggr)_i \biggl( \frac{\mu_e}{\mu_c}\biggr)</math>	<math>~=</math>	<math>~2\biggl[ \frac{\eta (- d\phi/d\eta)}{\phi} \biggr]_i </math>
	<math>~=</math>	<math>~\frac{[3\sin\Delta_i - 2\sin^3\Delta_i -3\cos\Delta_i ] }{\sin\Delta_i (3-2\sin^2\Delta_i)}</math>
	<math>~=</math>	<math>~\frac{3 - 2\sin^2\Delta_i -3\cot\Delta_i}{(3-2\sin^2\Delta_i)} \, .</math>

The left-hand side of this expression is inherently positive over the physically viable radial coordinate range, <math>~0 \ge \xi_i \ge \pi</math> and its value is known once the radial coordinate of the edge of the core has been specified. So, defining the interface parameter,

<math>~ \kappa_i \equiv - \frac{2\theta_i^' \xi_i}{3\theta_i} \biggl( \frac{\mu_e}{\mu_c} \biggr) \, ,</math>

we will recast the first constraint into, what will henceforth be referred to as, the

Key Nonlinear Interface Relation

<math>~\kappa_i</math>

<math>~\frac{3 - 2\sin^2\Delta_i -3\cot\Delta_i}{(3-2\sin^2\Delta_i)} \, .</math>

In a separate subsection of this chapter, below, we present a closed-form analytic solution, <math>~\Delta_i(\kappa_i)</math>, to this nonlinear equation.

Second Constraint

Obtained from Third Interface Condition

Our 3^rd interface condition, as detailed above, states that,

<math>~3^{-1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr) \phi_i^{-2} \, .</math>

If we now choose to normalize the interface amplitude such that, <math>~\phi_i = 1</math>, then this condition establishes two relations: First, from the 3^rd interface condition alone,

<math>~3^{-1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr) \xi_i \, ;</math>

and, second, from the definition of Srivastava's function, <math>~\phi</math>, we deduce that the overall scaling parameter is,

<math>~B_0^2</math>	<math>~=</math>	<math>~\frac{\sin^2\Delta_i}{\eta_i(3-2\sin^2\Delta_i)} </math>
	<math>~=</math>	<math>~\frac{\sqrt{3}}{\xi_i} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \biggl( \frac{3}{\sin^2\Delta_i} - 2\biggr)^{-1} \, . </math>

Notice that, after the solution, <math>~\Delta_i(\kappa_i)</math>, of the key nonlinear interface relation has been determined, the first of these two relations also permits us to write,

<math>~A_0\eta_i</math>	<math>~=</math>	<math>~e^{2\Delta_i}</math>
<math>~\Rightarrow ~~~~ A_0</math>	<math>~=</math>	<math>~3^{1/2} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \xi_i^{-1} e^{2\Delta_i} \, .</math>

Throughout the envelope, therefore, the angle,

<math>~\Delta</math>

<math>~\ln(A_0\eta)^{1/2} = \frac{1}{2} \ln\biggl[ \xi \cdot \xi_i^{-1} e^{2\Delta_i} \biggr] = \Delta_i + \ln\biggl( \frac{\xi}{\xi_i} \biggr)^{1/2} \, .</math>

Furthermore, because Shrivastava's function — and, along with it, the envelope's density — drops to zero when,

<math>\Delta = \Delta_s \equiv \pi \, ,</math>

we know that the radius, <math>~\xi_s</math>, of the bipolytropic configuration is given by the expression,

<math>~\pi</math>	<math>~=</math>	<math>~ \Delta_i + \ln\biggl( \frac{\xi_s}{\xi_i} \biggr)^{1/2} </math>
<math>~\Rightarrow ~~~~ \ln\biggl( \frac{\xi_s}{\xi_i} \biggr)^{1/2}</math>	<math>~=</math>	<math>~ \pi - \Delta_i </math>
<math>~\Rightarrow ~~~~ \xi_s</math>	<math>~=</math>	<math>~ \xi_i e^{2(\pi - \Delta_i )} \, . </math>

Obtained from Chandrasekhar's U-constraint

We shall now demonstrate that the same expression for the scaling coefficient, <math>~B</math>, can alternatively be obtained from Chandrasekhar's "U" constraint, without assuming that <math>~\phi_i = 1</math>, after taking into account the result that already has been obtained from the "V" constraint. As described above, the "U" constraint is an alternative interface condition that may be written as,

<math>~\frac{\xi_i \theta_i}{(-d\theta/d\xi)_i} \biggl( \frac{\mu_e}{\mu_c}\biggr)</math>

which, in the particular case being examined here, becomes,

<math>~\frac{2\xi_i^2}{3\kappa_i} \biggl( \frac{\mu_e}{\mu_c}\biggr)^2 </math>	<math>~=</math>	<math>~\biggl( U_\mathrm{5F} \biggr)_i </math>
	<math>~=</math>	<math>~ \frac{2B_0^{-4} \sin^4\Delta_i}{(3-2\sin^2\Delta_i)(3 - 2\sin^2\Delta_i - 3\cot\Delta_i)} \, . </math>

Now, from our discussion, above, of the first constraint, we know that,

<math>~(3 - 2\sin^2\Delta_i - 3\cot\Delta_i)</math>

<math>~(3-2\sin^2\Delta_i)\kappa_i \, .</math>

Hence, Chandrasekhar's U-constraint becomes,

<math>~\frac{2\xi_i^2}{3\kappa_i} \biggl( \frac{\mu_e}{\mu_c}\biggr)^2 </math>	<math>~=</math>	<math>~ \frac{2B_0^{-4} \sin^4\Delta_i}{(3-2\sin^2\Delta_i)^2 \kappa_i} </math>
<math>~\Rightarrow ~~~~ B_0^4 </math>	<math>~=</math>	<math>~ \frac{3\sin^4\Delta_i}{\xi_i^2 (3-2\sin^2\Delta_i)^2} \biggl( \frac{\mu_e}{\mu_c}\biggr)^{-2} </math>
<math>~\Rightarrow ~~~~ B_0^2</math>	<math>~=</math>	<math>~\frac{\sqrt{3}}{\xi_i} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{-1} \biggl( \frac{3}{\sin^2\Delta_i} - 2\biggr)^{-1} \, ,</math>

which, as predicted, is identical to what we learned from the third interface condition, alone.

Analytic Solution of Key Interface Relation

Returning to our previously derived,

Key Nonlinear Interface Relation

<math>~\kappa_i</math>

<math>~\frac{3 - 2\sin^2\Delta_i -3\cot\Delta_i}{(3-2\sin^2\Delta_i)} \, ,</math>

and, as in our separate discussion of the properties of Srivastava's function, adopting the shorthand notation,

<math>~y_i \equiv \tan\Delta_i \, ,</math>

this key interface condition becomes,

<math>~\kappa_i</math>	<math>~=</math>	<math>~ \frac{3 - 2y_i^2(1+y_i^2)^{-1}- 3y_i^{-1} }{3-2y_i^2(1+y_i^2)^{-1}} </math>
	<math>~=</math>	<math>~ \frac{3y_i(1+y_i^2)- 2y_i^3 -3(1+y_i^2)}{3y_i(1+y_i^2)-2y_i^3} </math>
	<math>~=</math>	<math>~ \frac{y_i^3 -3y_i^2 + 3y_i -3 }{3y_i+y_i^3} </math>
<math>~\Rightarrow~~~~ \kappa_i(3y_i+y_i^3)</math>	<math>~=</math>	<math>~ y_i^3 -3y_i^2 + 3y_i -3 </math>
<math>~\Rightarrow~~~~ y_i^3(1-\kappa_i) -3 y_i^2 + 3(1-\kappa_i)y_i -3</math>	<math>~=</math>	<math>~ 0 \, . </math>

This is a cubic equation whose solution, <math>~y_\mathrm{root}(\kappa_i)</math>, will also immediately supply the desired interface angle, <math>~\Delta_i</math>, and an interface coordinate root, <math>~(A_0\eta)_\mathrm{root}</math>.

ASIDE: Analytic Solution of Cubic Equation

As is well known and documented — see, for example Wolfram MathWorld or Wikipedia's discussion of the topic — the roots of any cubic equation can be determined analytically. In order to evaluate the root(s) of our particular cubic equation, we have drawn from the utilitarian online summary provided by Eric Schechter at Vanderbilt University. For a cubic equation of the general form,

a real root is given by the expression,

where,

<math>~p \equiv -\frac{b}{3a} \, ,</math> <math>~q \equiv \biggl[p^3 + \frac{bc-3ad}{6a^2} \biggr] \, ,</math> and <math>~r=\frac{c}{3a} \, .</math>

In our particular case,

<math>~a =(1-\kappa_i)\, ,</math> <math>~b =-3\, ,</math> <math>~c = 3(1-\kappa_i) \, ,</math> and <math>~d = - 3 \, .</math>

Hence,

<math>~p = \frac{1}{(1-\kappa_i)} \, ,</math> <math>~r=+1 \, ,</math> and <math>~q = p^3 = \frac{1}{(1-\kappa_i)^3} \, ,</math>

which implies that the real root, <math>~y_\mathrm{root}</math>, is given by the expression,

<math>~y_\mathrm{root}</math>	<math>~=</math>	<math>~ p + \{p^3 + [p^6 + (1-p^2)^3]^{1/2}\}^{1/3} + \{p^3 - [p^6 + (1-p^2)^3]^{1/2}\}^{1/3} </math>
	<math>~=</math>	<math>~ p + \{p^3 + p^3[1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} + \{p^3 - p^3[1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} </math>
<math>~\Rightarrow ~~~~ p^{-1} y_\mathrm{root}</math>	<math>~=</math>	<math>~ 1 + \{1 + [1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} + \{1 - [1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} \, . </math>

(There is also a pair of imaginary roots, but they are irrelevant in the context of our overarching astrophysical discussion.)

In summary, then,

Once the location, <math>~\xi_i</math>, of the outer edge of the core has been specified, which determines <math>~\theta_i</math> and <math>~\theta^'_i</math> as well, the value of the parameters, <math>~\kappa_i</math> and <math>~p</math>, are known via the expressions,

<math>~\kappa_i \equiv - \frac{2\theta_i^' \xi_i}{3\theta_i} \biggl( \frac{\mu_e}{\mu_c} \biggr)</math>

<math>~\frac{2}{3} \biggl(1 - \xi_i \cot\xi_i \biggr)\biggl( \frac{\mu_e}{\mu_c} \biggr) \, ,</math>

and,

<math>~p \equiv (1-\kappa_i)^{-1} </math>

The value of <math>~y_\mathrm{root}</math> is determined from the just-derived solution to the governing cubic equation, which then gives the interface value of the envelope parameter (adjusted by a <math>~\pi m</math> phase angle, where <math>~m</math> is an, as yet unspecified, integer),

<math>~\Delta_i = \tan^{-1}(y_\mathrm{root}) +\pi m \, .</math>

This, in turn, can be interpreted as a coordinate root — which we shall refer to as <math>~\eta_\mathrm{root}</math> — via the expression,

<math>~(A_0\eta)_\mathrm{root} = e^{2\Delta_i} = e^{2\pi m} \cdot e^{2\tan^{-1}(y_\mathrm{root})} \, .</math>

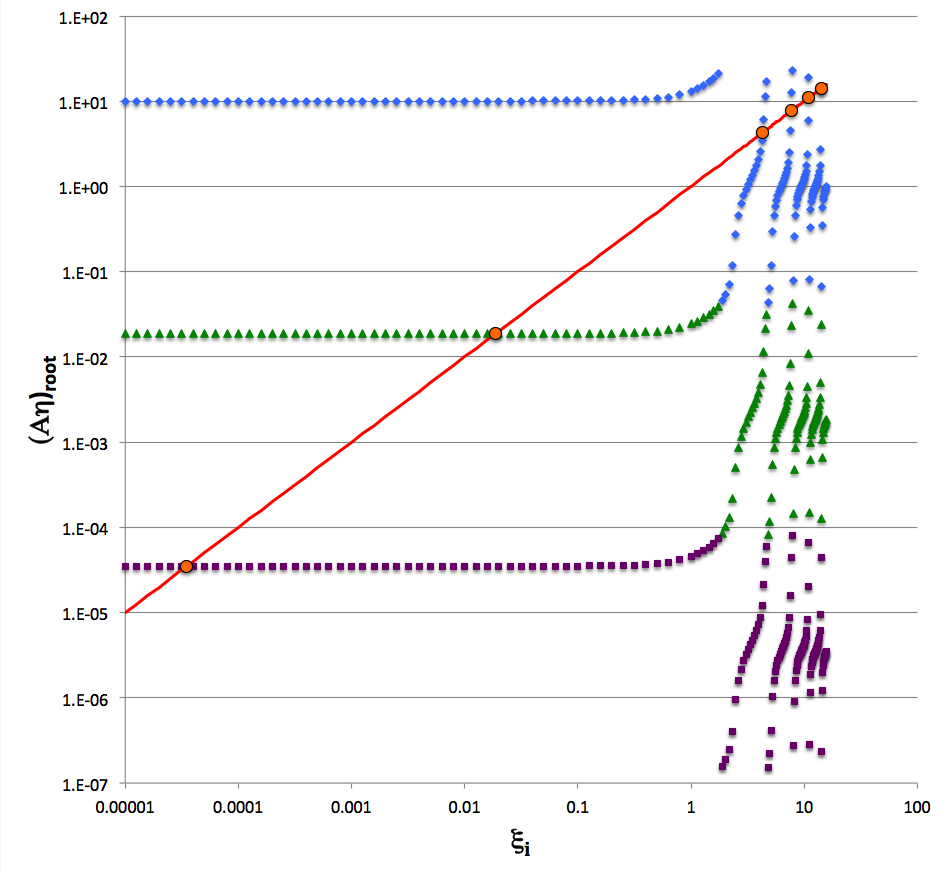
The following log-log plot shows what value(s) of <math>~(A_0\eta)_\mathrm{root} </math> (vertical axis) result from a wide range of interface coordinate specifications, <math>~\xi_i</math> (horizontal axis), under the simplifying assumption that <math>~(\mu_e/\mu_c) = 1</math>; solid blue diamonds identify roots resulting from setting <math>~m=0</math> in the phase-shift specification, solid green triangles identify roots for which <math>~m=-1</math>, and solid purple squares identify roots for which <math>~m=-2</math>. Notice that when <math>~\xi_i \ll 1</math> — and, hence, <math>~\kappa_i \ll 1</math> and <math>~p \rightarrow 1</math>,

<math>~y_\mathrm{root} \rightarrow (1 + 2^{1/3}) ~~~~~~~ \Rightarrow ~~~~~~~ (A_0\eta)_\mathrm{root} \rightarrow e^{2\pi m} \cdot e^{2\tan^{-1}(1 + 2^{1/3})} = 10.05837e^{2\pi m} \, .</math>

Hence, as displayed in the figure, the curve traced by blue diamonds (m = 0) asymptotically approaches (to the left) <math>~10.05837</math>; the curve traced by green triangles (m = -1) asymptotically approaches <math>1.87834\times 10^{-2}</math>; and the curve traced by purple squares (m = -2) asymptotically approaches <math>3.50770\times 10^{-5}</math>.

Examination of F2 Function Discussed by J. O. Murphy (1983, Proc. Astr. Soc. of Australia, 5, 175)

Murphy's

Roots of

F2 = 0

Function

3.5076E-05

1.8785E-02

4.2993E+00

7.6882E+00

1.0913E+01

1.4101E+01

The red line in the figure shows where the coordinate value along the vertical axis equals the coordinate value along the horizontal axis. Hence the points where this red line intersects the other curves in the figure can be interpreted as identifying solutions to the cubic equation for which,

<math>~(A_0\eta)_\mathrm{root} = \xi_i \, .</math>

The points of intersection that are highlighted in this figure are also what Murphy (1983) refers to as roots of his <math>~F2 = 0</math> function. The values of ten successive coordinate roots of this function are listed in the right-hand column of Table 1 in Murphy (1983). We have re-listed the values of six of these roots in a column immediately to the right of the above figure and have marked with orange circles the locations of these six points in the figure. As they should, Murphy's identified roots lie precisely at the intersection of the red line with the other curves.

Murphy's Example Model Characteristics

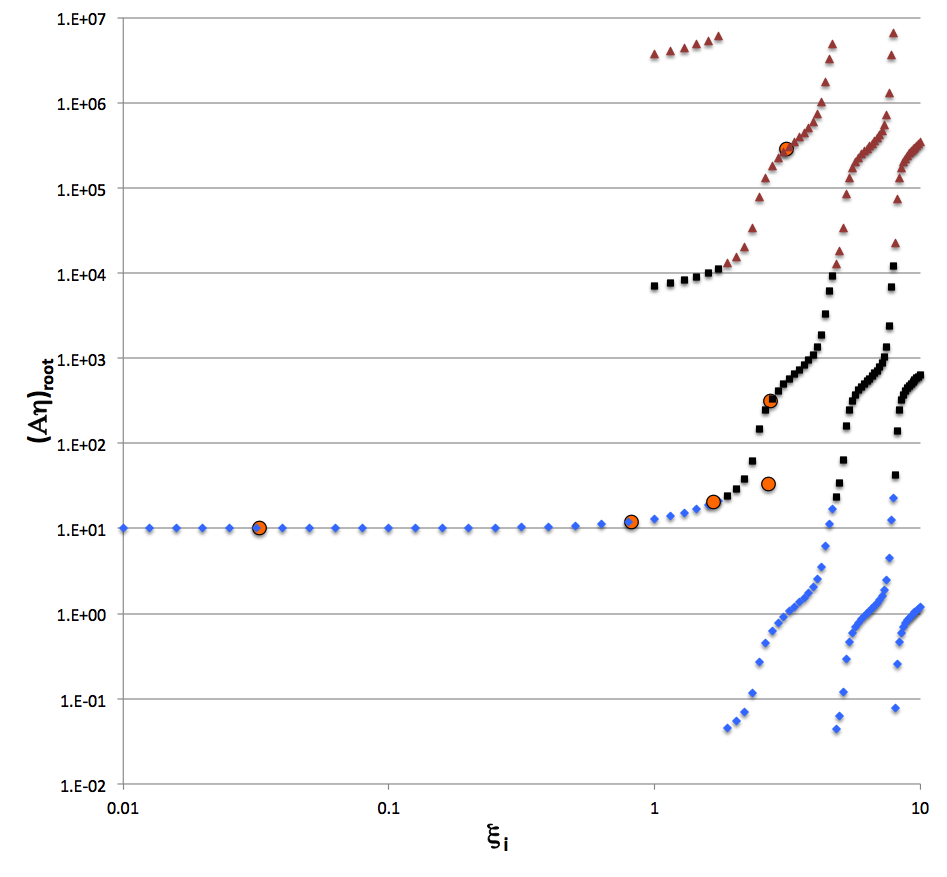
As in the previous figure, the following log-log plot shows what value(s) of <math>~(A_0\eta)_\mathrm{root} </math> (vertical axis) result from a wide range of interface coordinate specifications, <math>~\xi_i</math> (horizontal axis), under the simplifying assumption that <math>~(\mu_e/\mu_c) = 1</math>. The solid blue diamonds present exactly the same information as in the previous figure, that is, they identify roots resulting from setting <math>~m=0</math> in the phase-shift specification. But here, focusing on a regime that highlights larger roots of the first constraint equation, solid black squares identify roots for which <math>~m=+1</math>, and solid maroon triangles identify roots for which <math>~m=+2</math>.

Examination of Table 3 Data Presented in J. O. Murphy (1983, Proc. Astr. Soc. of Australia, 5, 175)

Table 3 Model Characteristics by Murphy (1983)

Murphy's Table 3 Data
<math>~\zeta_J \rightarrow \xi_i</math>	<math>~\xi_J \rightarrow (A_0\eta)_\mathrm{root}</math>
3.2678E-02	10.0164
0.8154	12.0083
1.6598	20.4312
2.6914	33.0249
2.7302	100<math>\pi</math>
3.1415	91275<math>\pi</math>

In his Table 3, Murphy (1983) presents six pairs of "coordinate" roots, <math>~(\zeta_J, \xi_J)</math>, that not only satisfy the first constraint equation but that also, in his interpretation, identify physically viable model solutions. The values of all six of these coordinate pairs have been re-listed immediately to the right of the log-log plot, in a separate panel of the above figure. After mapping Murphy's coordinate notation to ours — specifically invoking the mapping <math>~(\zeta_J, \xi_J) ~\rightarrow~ (\xi_i, A_0\eta_\mathrm{root})</math> — the locations of all six models have been marked by orange circles on the plot. Five of Murphy's models fall directly onto our generated curves, giving us considerable confidence that our analysis matches his in considerable detail. As is explained more fully, below, we strongly suspect that the single Murphy model that does not lie on one of our generated curves — the one which, as published in Murphy's Table 3, has coordinates<math>~(\zeta_J, \xi_J) = (2.6914, 33.0249)</math> — is misplaced because of a typographical error in the published table.

As a supplement to the preceding two figures, the following table shows in quantitative detail how we are able to analytically derive individual coordinate roots, <math>~(A_0\eta)_\mathrm{root}</math> — see the next-to-last column of the table — from ten different interface-coordinate locations, <math>~\xi_i = \zeta_J</math>, as specified in the first (left-most) column of the table. The interface parameter, <math>~\kappa_i</math>, is determined directly from the specified value of <math>~\xi_i</math>; the parameter, <math>~p=(1-\kappa_i)^{-1}</math>; then, given <math>~p</math>, the root to the cubic equation and, in turn, the value of <math>~\Delta_i</math>, are determined; and, finally, a choice of the integer phase-shift parameter, <math>~m</math>, leads to the calculation of <math>~(A_0\eta)_\mathrm{root}</math>. For comparison, the last (right-most) column of the table lists the corresponding coordinate, <math>~\xi_J</math>, as originally derived and published by Murphy (1983). Coordinate pairs shown in the orange-colored panels of our table correspond to the six models whose characteristics are provided in Murphy's Table 3; coordinate pairs shown in the green-colored panels correspond to two of the ten "F2 = 0" roots identified in Murphy's Table 1; and the additional three coordinate pairs shown in the blue-colored panels have been drawn from Murphy's Table 2.

Murphy (1983) Tables 1, 2, & 3	Here … setting <math>\xi_i = \zeta_J</math> … and assuming <math>\mu_e/\mu_c = 1</math>						Murphy (1983) Tables 1, 2, & 3
Murphy (1983) Tables 1, 2, & 3	1^st Constraint						Murphy (1983) Tables 1, 2, & 3
<math>~\zeta_J</math>	<math>~\kappa_i</math>	<math>~p</math>	<math>~y_\mathrm{root}</math>	<math>~(\Delta_i-m\pi)</math> <math>~= \tan^{-1}(y_\mathrm{root})</math>	<math>~m </math>	<math>~(A_0\eta)_\mathrm{root} = e^{2\Delta_i}</math>	<math>~\xi_J</math>
3.2678E-02	2.3732E-04	1.00023737	2.26083	1.15435	-3	6.55237E-08	6.55E-08
					-2	3.50874E-05	3.508E-05
					-1	1.87890E-02	1.8789E-02
					0	10.0614	10.0164
0.8154	0.15474	1.18307	2.93939	1.24287	0	12.0101	12.0083
1.6598	0.76541	4.26281	12.6311	1.49179	0	19.7585	20.4312
[ 2.0914 ]	1.46609	-2.14552	-6.11816	-1.40878	1	31.9964	33.0249
[ 2.10961 ]	1.50744	-1.97067	-5.56379	-1.39296	1	33.0249
2.6914	4.37925	-0.29592	-0.31486	-0.30503	1	290.943
2.7302	4.83853	-0.26052	-0.27316	-0.26666	1	99.997<math>\pi</math>	100<math>\pi</math>
3.1415	22604.6	-4.42408E-05	-4.42408E-05	-4.42408E-05	2	91268<math>\pi</math>	91275<math>\pi</math>
4.2993	-0.58961	0.62908	0.89355	0.72924	0	4.2994	4.2993
7.6882	-0.19091	0.83969	1.6268	1.01964	0	7.6851	7.6882

As this table and its two preceding figures illustrate, by and large, the agreement between our analytically determined coordinate roots and those derived & published by Murphy (1983) is excellent. There are a couple of mismatches, but we attribute both to typesetting errors in the Murphy publication:

The coordinate, <math>~\xi_J = 10.0164</math>, that has been displayed using a red font in the right-most column of our table is the value drawn directly from Murphy's Table 3. It appears to us that two digits of this number were inadvertently transposed during publication and that the coordinate value should have appeared as <math>~\xi_J = 10.0614</math>. We are convinced that, as published, this is a typographical error in Murphy's Table 3 because: (a) the corrected value matches our derived value of that coordinate to more significant figures, and (b) the same model is also listed in Murphy's Table 2 with this corrected value.
The coordinate, <math>~\zeta_J = 2.6914</math>, that has been displayed using a red font in the left-most column of our table is the value drawn directly from Murphy's Table 3. As shown in our table, from this coordinate value we derive a root coordinate of <math>~(A_0\eta)_\mathrm{root} = 290.943</math>, which is almost a factor of ten larger than Murphy's published root coordinate value, <math>~\xi_J = 33.0249</math>. Working backwards via an iterative procedure, we have determined that Murphy's listed root of <math>33.0249</math> can be obtained if the originating value of <math>~\zeta_J \leftrightarrow \xi_i</math> is set to <math>2.10961</math> (see the black-on-white panel entry in the left-most column of our table). By replacing the "6" by a zero in Murphy's published coordinate parameter — that is, by assuming that there was a typographical error and Murphy's interface coordinate was actually <math>~\zeta_J = 2.0914</math>, we are able to derive a root coordinate value (31.9964) that is quite close to Murphy's published value (33.0249).

We can now construct a table of our analytically determined model characteristics that is patterned after and largely matches Murphy's (1983) Table 3.

Some Model Characteristics (assuming <math>\mu_e/\mu_c</math> = 1)

Model

Specified

Analytically Determined Here

… and Constraint Implications^†

<math>~\Delta_i</math>

<math>~(A_0\eta)_\mathrm{root}</math>

<math>~\frac{A_0}{3^{1/2}} \biggl( \frac{\mu_e}{\mu_c} \biggr) </math>

<math>~\frac{B_0}{3^{1/4}} \biggl(\frac{\mu_e}{\mu_c}\biggr)^{1/2} \theta_i^{-1} </math>

1

3.2678E-02

0

1.1544

10.0614

307.894

5.77929

4.39209

1.7392

3.5598E-04

1.1866E-4

1.000

2.9998

1.000

2

0.8154

0

1.2429

12.0101

14.729

1.25567

1.06865

36.356

0.23212

0.077372

1.000

2.8644

1.000

3

1.6598

0

1.4918

19.7585

11.9041

1.01206

1.28146

44.984

1.14812

0.38271

1.000

2.3995

1.000

4

2.0914

1

1.7328

31.9964

15.2990

0.87563

1.60398

35.0016

2.19913

0.73304

1.000

1.98894

1.000

5

2.7302

1

2.8749

100.00<math>\pi</math>

115.065

0.12408

0.64370

4.6539

7.25779

2.41926

1.000

1.02703

1.000

6

3.1415

2

6.283141

91268<math>\pi</math>

170.44223<math>e^{2\pi}</math>

1.8966E-05

0.48862

3.141778

3.3907E+04

1.1302E+04

1.000

2.9106E-04

1.000

^†See an accompanying discussion for definitions of the functions, <math>~U_{1E}</math>, <math>~V_{1E}</math>, <math>~U_{5F}</math>, and <math>~V_{5F} \, .</math>

Given <math>~\xi_i</math>, <math>~m</math>, and <math>~\mu_e/\mu_c</math>:

<math>~p = (1-\kappa_i)^{-1} </math>	<math>~=</math>	<math>~3[3-2(\mu_e/\mu_c)(1-\xi_i \cot\xi_i)]^{-1}</math>
<math>~y_\mathrm{root}</math>	<math>~=</math>	<math>~p\biggl[ 1 + \{1 + [1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} + \{1 - [1 + (p^{-2}-1)^3]^{1/2}\}^{1/3} \biggr]</math>
<math>~\Delta_i</math>	<math>~=</math>	<math>~\tan^{-1}(y_\mathrm{root}) + m\pi</math>
<math>~(A_0\eta)_\mathrm{root}</math>	<math>~=</math>	<math>~e^{2\Delta_i}</math>
<math>~\xi_s</math>	<math>~=</math>	<math>~\xi_ie^{2(\pi-\Delta_i)}</math>
<math>~\biggl[ \frac{A_0}{3^{1/2}} \biggl( \frac{\mu_e}{\mu_c} \biggr) \biggr]</math>	<math>~=</math>	<math>~\xi_i^{-1} e^{2\Delta_i}</math>
<math>~\biggl[\frac{B_0}{3^{1/4}} \biggl( \frac{\mu_e}{\mu_c} \biggr)^{1/2} \biggr]</math>	<math>~=</math>	<math>~\biggl[ \xi_i \biggl( \frac{3}{\sin^2\Delta_i} - 2\biggr) \biggr]^{-1/2} = \theta_i \biggl[ \frac{\xi_i^{1/2}}{\sin\xi_i}\biggl( \frac{3}{\sin^2\Delta_i} - 2\biggr)^{-1/2} \biggr] </math>

Physically Viable Parameter Regime

Key References

S. Srivastava (1968, ApJ, 136, 680) A New Solution of the Lane-Emden Equation of Index n = 5
H. A. Buchdahl (1978, Australian Journal of Physics, 31, 115): Remark on the Polytrope of Index 5 — the result of this work by Buchdahl has been highlighted inside our discussion of bipolytropes with <math>~(n_c, n_e) = (5, 1)</math>.
J. O. Murphy (1980a, Proc. Astr. Soc. of Australia, 4, 37): A Finite Radius Solution for the Polytrope Index 5
J. O. Murphy (1980b, Proc. Astr. Soc. of Australia, 4, 41): On the F-Type and M-Type Solutions of the Lane-Emden Equation
J. O. Murphy (1981, Proc. Astr. Soc. of Australia, 4, 205): Physical Characteristics of a Polytrope Index 5 with Finite Radius
J. O. Murphy (1982, Proc. Astr. Soc. of Australia, 4, 376): A Sequence of E-Type Composite Analytical Solutions of the Lane-Emden Equation
J. O. Murphy (1983, Australian Journal of Physics, 36, 453): Structure of a Sequence of Two-Zone Polytropic Stellar Models with Indices 0 and 1
J. O. Murphy (1983, Proc. Astr. Soc. of Australia, 5, 175): Composite and Analytical Solutions of the Lane-Emden Equation with Polytropic Indices n = 1 and n = 5
J. O. Murphy & R. Fiedler (1985a, Proc. Astr. Soc. of Australia, 6, 219): Physical Structure of a Sequence of Two-Zone Polytropic Stellar Models
J. O. Murphy & R. Fiedler (1985b, Proc. Astr. Soc. of Australia, 6, 222): Radial Pulsations and Vibrational Stability of a Sequence of Two-Zone Polytropic Stellar Models

Related Discussions

User:Tohline/SSC/Structure/BiPolytropes/Analytic1 5

Contents

BiPolytrope with <math>n_c = 1</math> and <math>n_e=5</math>

Steps 2 & 3

Step 4: Throughout the core (<math>0 \le \xi \le \xi_i</math>)

Step 5: Interface Conditions

Step 6: Envelope Solution

First Constraint

Second Constraint

Obtained from Third Interface Condition

Obtained from Chandrasekhar's U-constraint

Analytic Solution of Key Interface Relation

Murphy's Example Model Characteristics

Key References

Related Discussions

Navigation menu

Search