Properties of Homogeneous Ellipsoids (2)

In addition to pulling from §53 of Chandrasekhar's EFE, here, we lean heavily on the papers by M. D. Weinberg & S. Tremaine (1983, ApJ, 271, 586) (hereafter, WT83) and by D. M. Christodoulou, D. Kazanas, I. Shlosman, & J. E. Tohline (1995a, ApJ, 446, 472) (hereafter, Paper I).

Sequence-Defining Dimensionless Parameters

A Riemann sequence of S-type ellipsoids is defined by the value of the dimensionless parameter,

<math>~\equiv</math>

<math>~\frac{\zeta}{\Omega} = </math> constant,

[ EFE, §48, Eq. (31) ]
[ WT83, Eq. (5) ]
[ Paper I, Eq. (2.1) ]

where, <math>~\zeta</math> is the system's vorticity as measured in a frame rotating with angular velocity, <math>~\Omega</math>. Alternatively, we can use the dimensionless parameter,

<math>~\equiv</math>

<math>~\biggl[\frac{ab}{a^2 + b^2} \biggr]f \, ,</math>

[ EFE, §48, Eq. (40) ]
[ Paper I, Eq. (2.2) ]

or,

<math>~\Lambda</math>

<math>~\equiv</math>

<math>~-\biggl[\frac{ab}{a^2 + b^2} \biggr] \Omega f = -\Omega x \, .</math>

[ WT83, Eq. (4) ]

Conserved Quantities

Algebraic expressions for the conserved energy, <math>~E</math>, angular momentum, <math>~L</math>, and circulation, <math>~C</math>, are, respectively,

<math>~E</math>	<math>~=</math>	<math>~\frac{1}{2}v^2 + \frac{1}{2}(a^2 + b^2)(\Lambda^2 + \Omega^2) - 2ab\Lambda\Omega - 2I </math>
	<math>~\rightarrow</math>	<math>~\cancelto{0}{\frac{1}{2}v^2} + \frac{1}{2} [(a+bx)^2 + (b+ax)^2]\Omega^2 - 2I \, ,</math>

[ 1^st expression — EFE, §53, Eq. (239) ]
[ 2^nd expression — Paper I, Eq. (2.7) ]

where — see an accompanying discussion for the definitions of <math>~A_1</math>, <math>~A_2</math>, and <math>~A_3</math>,

[ 1^st expression — EFE, §53, Eq. (239) ]
[ 2^nd expression — Paper I, Eq. (2.8) ]

<math>~\frac{5L}{M}</math>	<math>~=</math>	<math>~(a^2 + b^2)\Omega - 2ab\Lambda</math>
	<math>~=</math>	<math>~ (a^2 + b^2 + 2abx)\Omega \, ;</math>

[ 1^st expression — EFE, §53, Eq. (240) ]
[ 2^nd expression — Paper I, Eq. (2.5) ]

<math>~\frac{5C}{M}</math>	<math>~=</math>	<math>~(a^2 + b^2)\Lambda - 2ab\Omega</math>
	<math>~=</math>	<math>~- [2ab + (a^2 + b^2)x ]\Omega \, .</math>

[ 1^st expression — EFE, §53, Eq. (241) ]
[ 2^nd expression — Paper I, Eq. (2.6) ]

If we rewrite the expression for the system's free energy in terms of <math>~L</math> (and x) instead of <math>~\Omega</math> (and x), we have,

<math>~\frac{1}{2} \biggl(\frac{5L}{M}\biggr)^2 \frac{(a+bx)^2 + (b+ax)^2}{(a^2 + b^2 + 2abx)^2} - 2I \, ,</math>

[ Paper I, Eq. (3.4) ]

Note that, based on the units chosen in Paper I, <math>~M = 5</math>, and <math>~abc = 15/4</math>.

Aside: Chandra's Notation

According to equation (107) in §21 of EFE, it appears as though,

And, according to equation (105) in §21 of EFE, it appears as though,

So, for example,

<math>~-\biggl[ \frac{A_1 - A_2}{a_1^2 - a_2^2} \biggr] \, ,</math>

and,

<math>~B_{12} </math>	<math>~=</math>	<math>~A_2 + a_1^2\biggl[ \frac{A_1 - A_2}{a_1^2 - a_2^2} \biggr] </math>
	<math>~=</math>	<math>~\frac{(a_1^2 - a_2^2)A_2 + a_1^2(A_1 - A_2)}{a_1^2 - a_2^2} </math>
	<math>~=</math>	<math>~\frac{a_1^2A_1 - a_2^2A_2 }{a_1^2 - a_2^2} \, .</math>

Free Energy Surface(s)

Scope

Consider a self-gravitating ellipsoid having the following properties:

Semi-axis lengths, <math>~(x,y,z)_\mathrm{surface} = (a,b,c)</math>, and corresponding volume, <math>~4\pi/(3abc)</math> ; and consider only the situations <math>0 \le b/a \le 1</math> and <math>0 \le c/a \le 1</math> ;
Total mass, <math>~M</math> ;
Uniform density, <math>~\rho = (3 M)/(4\pi abc) </math> ;
Figure is spinning about its c axis with angular velocity, <math>~\Omega</math> ;
Internal, steady-state flow exhibiting the following characteristics:

No vertical (z) motion;
Elliptical (x-y plane) streamlines everywhere having an ellipticity that matches that of the overall figure, that is, <math>~e = (1-b^2/a^2)^{1/2}</math> ;
The velocity components, <math>~v_x</math> and <math>~v_y</math>, are linear in the coordinate and, overall, characterized by the magnitude of the vorticity, <math>~\zeta</math> .

Such a configuration is uniquely specified by the choice of six key parameters: <math>~a</math>, <math>~b</math>, <math>~c</math>, <math>~M</math>, <math>~\Omega</math>, and <math>~\zeta</math> .

Free Energy of Incompressible, Constant Mass Systems

We are interested, here, in examining how the free energy of such a system will vary as it is allowed to "evolve" as an incompressible fluid — i.e., holding <math>~\rho</math> fixed — through different ellipsoidal shapes while conserving its total mass. Following Paper I, we choose to set <math>~M = 5</math> — which removes mass from the list of unspecified key parameters — and we choose to set <math>~\rho = \pi^{-1}</math>, which is then reflected in a specification of the semi-axis, <math>~a</math>, in terms of the pair of dimensionless axis ratios, <math>~b/a</math> and <math>~c/a</math>, namely,

<math>~\frac{3Ma^2}{4\pi(bc)\rho} = \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1}\, .</math>

Moving forward, then, a unique ellipsoidal configuration is identified via the specification of four, rather than six, key parameters — <math>~b/a</math>, <math>~c/a</math>, <math>~\Omega</math>, and <math>~x</math> — and the free energy of that configuration is given by the expression,

<math>~E\biggl(\frac{b}{a}, \frac{c}{a}, \Omega, x\biggr)</math>	<math>~=</math>	<math>~\frac{a^2}{2} \biggl[\biggl(1+\frac{b}{a} \cdot x\biggr)^2 + \biggl(\frac{b}{a}+x\biggr)^2\biggr]\Omega^2 - 2I </math>
	<math>~=</math>	<math>~\biggl[ \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1} \biggr]^{2/3} \biggl\{\frac{1}{2} \biggl[\biggl(1+\frac{b}{a} \cdot x\biggr)^2 + \biggl(\frac{b}{a}+x\biggr)^2\biggr]\Omega^2 - \frac{2I}{a^2}\biggr\} \, ,</math>

where,

<math>~x</math>	<math>~\equiv</math>	<math>~\biggl[\frac{(b/a)}{1 + (b/a)^2} \biggr]\frac{\zeta}{\Omega} \, ,</math>
<math>~\frac{I}{a^2}</math>	<math>~=</math>	<math>~\biggl[A_1 + A_2\biggl(\frac{b}{a}\biggr)^2 + A_3\biggl(\frac{c}{a}\biggr)^2 \biggr] \, ,</math>

and the functional behavior of the coefficients, <math>~A_1</math>, <math>~A_2</math>, and <math>~A_3</math>, are given by the expressions provided in an accompanying discussion.

Alternatively, replacing <math>~\Omega</math> in favor of <math>~L</math>, we have,

<math>~E\biggl(\frac{b}{a}, \frac{c}{a}, L, x\biggr)</math>	<math>~=</math>	<math>~\frac{L^2}{2a^2} \biggl[ \biggl(1+\frac{b}{a}\cdot x \biggr)^2 + \biggl(\frac{b}{a}+x \biggr)^2 \biggr] \biggl[ 1 + \biggl(\frac{b}{a}\biggr)^2 + 2\biggl(\frac{b}{a}\biggr)x \biggr]^{-2} - 2I </math>
	<math>~=</math>	<math>~\frac{L^2}{2} \biggl[ \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1} \biggr]^{-2/3} \biggl[ \biggl(1+\frac{b}{a}\cdot x \biggr)^2 + \biggl(\frac{b}{a}+x \biggr)^2 \biggr] \biggl[ 1 + \biggl(\frac{b}{a}\biggr)^2 + 2\biggl(\frac{b}{a}\biggr)x \biggr]^{-2} </math>
		<math>~- 2\biggl[ \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1} \biggr]^{2/3} \biggl[A_1 + A_2\biggl(\frac{b}{a}\biggr)^2 + A_3\biggl(\frac{c}{a}\biggr)^2 \biggr]\, .</math>

Adopted Evolutionary Constraints

Conserve Only L

Let's fix the total angular momentum, <math>~L</math>, of a triaxial configuration and examine how the configuration's free energy varies as we allow it to contort through different triaxial shapes — that is, as its pair of axis ratios varies, always maintaining <math>~\tfrac{b}{a} < 1</math> — and as we vary <math>~x</math>, which characterizes the fraction of angular momentum that is stored in internal spin versus overall figure rotation. The desired free-energy function, <math>~E(\tfrac{b}{a},\tfrac{c}{a}, x)|_L</math>, has just been defined, but visualizing its behavior is difficult because, in this situation, the free energy is a warped, three-dimensional surface draped across the four-dimensional domain, <math>~(\tfrac{b}{a},\tfrac{c}{a}, x, E_L)</math>.

Acknowledging that we are primarily interested in identifying extrema of this free-energy function, the discussion presented in §3.2 of Paper I shows us how to reduce the dimensionality of this problem by one. There, it is shown that, as long as <math>~\tfrac{b}{a} \ne 1</math>, extrema exist in the <math>~x</math>-coordinate direction — that is, <math>~\partial E_L/\partial x = 0</math> — only if <math>~x = 0.</math> For a given choice of <math>~L</math>, therefore, the relevant two-dimensional free-energy surface is defined by the expression,

<math>~E\biggl(\frac{b}{a}, \frac{c}{a}, x=0\biggr)\biggr\|_L</math>	<math>~=</math>	<math>~\frac{L^2}{2} \biggl[ \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1} \biggr]^{-2/3} \biggl[ 1 + \biggl(\frac{b}{a}\biggr)^2\biggr]^{-1} </math>
		<math>~ - 2\biggl[ \frac{15}{4}\biggl(\frac{b}{a}\biggr)^{-1} \biggl(\frac{c}{a}\biggr)^{-1} \biggr]^{2/3} \biggl[A_1 + A_2\biggl(\frac{b}{a}\biggr)^2 + A_3\biggl(\frac{c}{a}\biggr)^2 \biggr]\, .</math>

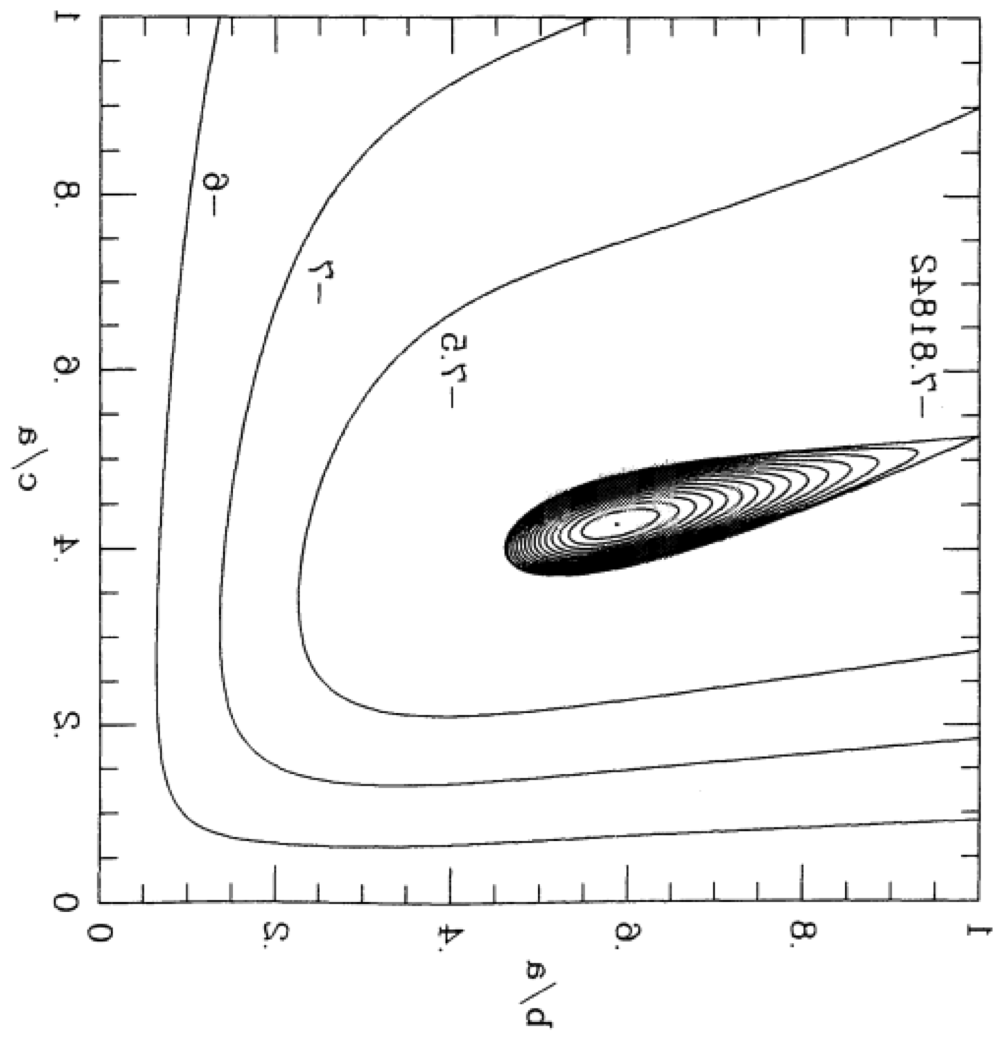
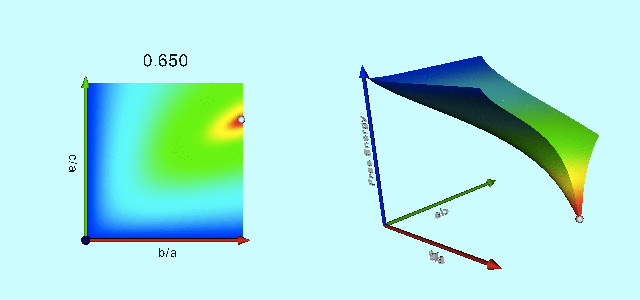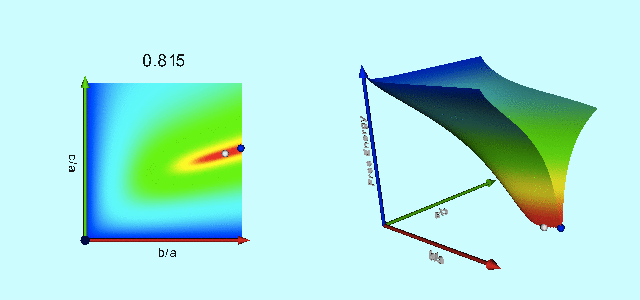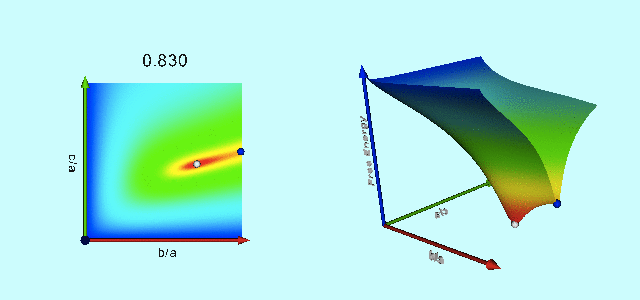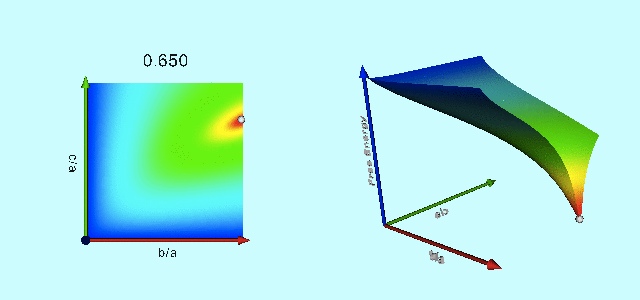
Figure 3 of Paper I presents a black-and-white contour plot of this <math>~E_L</math> function for the specific case of <math>~L = 4.71488</math>, which, for reference, is the total angular momentum of an equilibrium Maclaurin spheroid having an eccentricity, <math>~e = 0.85</math> (see Table 1, below). We have digitally extracted this black-and-white contour plot from p. 477 of the (PDF-formatted) Paper I publication and have reprinted it as the left-hand panel of our Figure 1. Note that we have flipped the plot horizontally and rotated it by 90° so that the orientation of the axis pair, <math>~(\tfrac{b}{a},\tfrac{c}{a})</math>, conforms with the orientation of a related, information-rich diagram presented by Chandrasekhar (1965) — see also our accompanying discussion of equilibrium sequence plots.

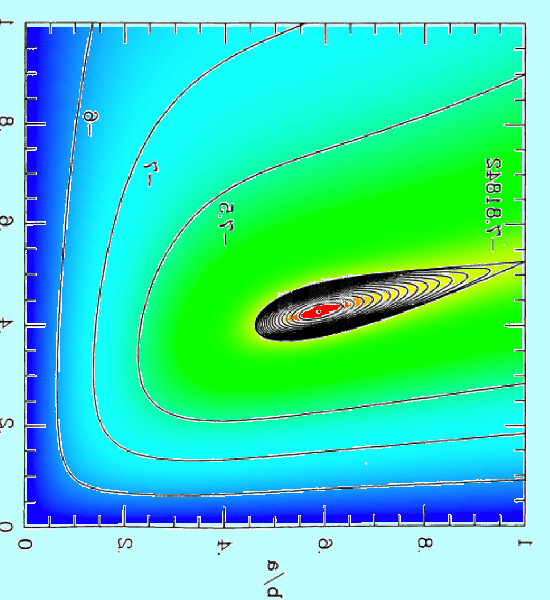
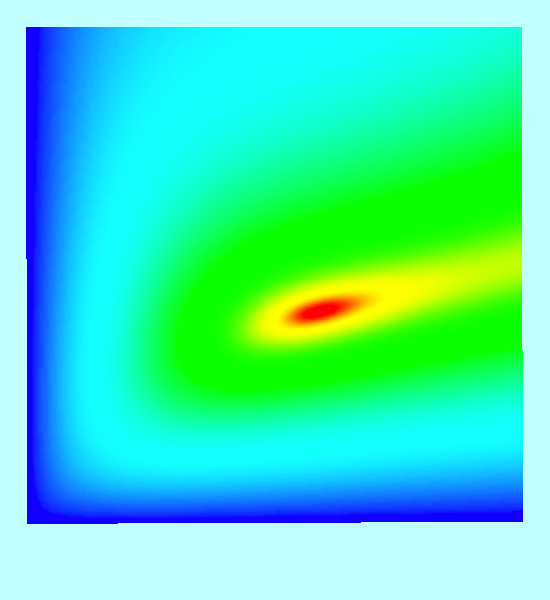
Figure 1: Free-Energy Surface Projected onto the <math>~(\tfrac{b}{a},\tfrac{c}{a})</math> Plane

All three contour plots show how the free-energy, <math>~E_L</math>, varies across the <math>~(\tfrac{b}{a}, \tfrac{c}{a})</math> domain for the specific case of <math>~L = 4.71488</math>. Horizontal axis is <math>~0 \le \tfrac{b}{a} \le 1</math> and vertical axis is <math>~0 \le \tfrac{c}{a} \le 1</math>.

Left-hand Panel:
Black-and-white contour plot

extracted from p. 477 of Paper I

"Phase-Transition Theory of Instabilities. I. Second-Harmonic Instability and Bifurcation Points"

Middle Panel:
Black-and-white contour plot digitally overlaid on color contour plot.

Right-hand Panel:
Color contour plot
created here as a projection of the free-energy surface shown in Fig. 2.

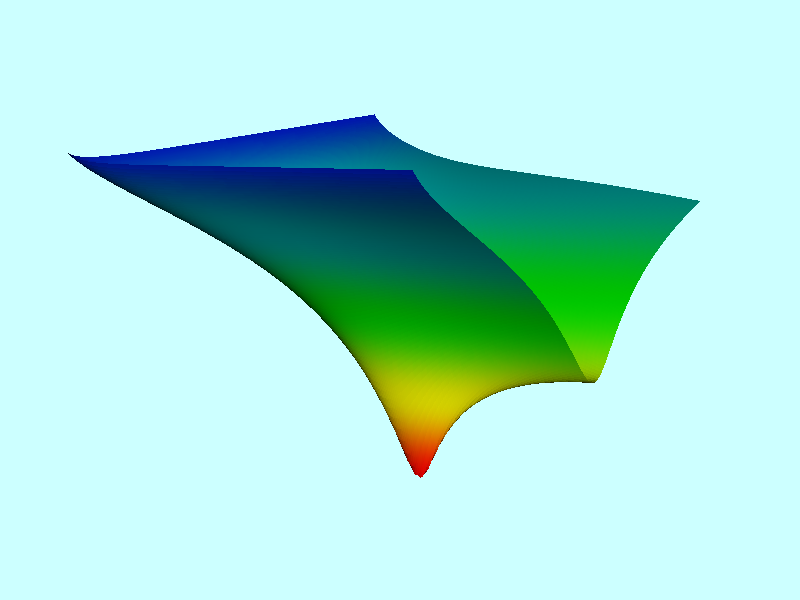
In our Figure 2, this same <math>~E_L</math> function has been displayed as a warped, two-dimensional free-energy surface draped across the three-dimensional <math>~(\tfrac{b}{a},\tfrac{c}{a},E)</math> domain, where depth as well as color has been used to tag energy values. The two-dimensional, colored contour plot presented in the right-hand panel of our Figure 1 results from the projection of this free-energy surface onto the <math>~(\tfrac{b}{a},\tfrac{c}{a})</math> plane; it reproduces in quantitative detail the black-and-white contour plot that we have extracted from Paper I. In an effort to (qualitatively) illustrate this agreement, we have digitally "pasted" the black-and-white contour plot from Paper I onto our colored contour plot and presented the combined image in the middle panel of our Figure 1.

Our Figure 2 image of the free-energy surface helps illuminate the description of this surface that appears in the caption of Fig. 3 from Paper I. Quoting from that figure caption: "The [equilibrium] Maclaurin spheroid sits on a saddle point <math>~[(\tfrac{b}{a},\tfrac{c}{a}) = (1.0,0.52678); E_0 = -7.81842]</math>, while a global minimum with <math>~E_0 = -7.83300</math> exists at <math>~(\tfrac{b}{a},\tfrac{c}{a}) = (0.588,0.428)</math>."

Figure 2: Free-Energy Surface

Animation

The animation sequence presented, below, as Figure 3 displays the warped free-energy surface (right) in conjunction with its projection onto the <math>~(\tfrac{b}{a},\tfrac{c}{a})</math> plane (left) for configurations having nineteen different total angular momentum values, <math>~L</math>, as detailed in column 5 of Table 1. The four-digit number that tags each frame of this animation sequence identifies the eccentricity (column 1 of Table 1) of the Maclaurin spheroid that is associated with each selected value of <math>~L</math>. In each frame of the animation, the equilibrium configuration associated with that Maclaurin spheroid is identified by the extremum of the free energy that appears along the right-hand edge <math>~(\tfrac{b}{a} = 1)</math> of the warped surface. For values of <math>~e < 0.81267</math> — corresponding to <math>~L < 4.23296</math> — the Maclaurin spheroid (marked by a small white circle/sphere) sits at the location of the absolute minimum of the free-energy surface and the configuration is stable. But for all larger values of the eccentricity/angular momentum, the Maclaurin spheroid (marked by a small dark-blue circle/sphere) is associated with a saddle point of the free-energy surface — that is, the configuration is in equilibrium, but it is (secularly) unstable — and the absolute energy minimum shifts off-axis to the location of a Jacobi ellipsoid (marked by a small white circle/sphere) having the same total angular momentum. As Paper I points out, evolution from the unstable axisymmetric equilibrium configuration to the stable triaxial configuration occurs along the narrow valley/canyon connecting the two extrema of the free energy.

Figure 3: Animation

Table 1: Parameter Values Associated with Each Frame of the Figure 3 Animation (parameter values associated with Figures 1 & 2 are highlighted in pink)
Maclaurin Spheroid				<math>~L^\dagger</math>	Jacobi Ellipsoids
<math>~e</math>	<math>~\frac{c}{a}</math>	<math>~E_L</math>	<math>~E_\mathrm{plot}^\ddagger</math>	<math>~L^\dagger</math>	<math>~\frac{b}{a}</math>	<math>~\frac{c}{a}</math>	<math>~E_\mathrm{Jac}</math>
0.650	0.7599342	-8.9018255	0.0	2.8270256	---	---	---
0.675	0.7378177	-8.8165100	0.0	2.9985043	---	---	---
0.700	0.7141428	-8.7216343	0.0	3.1820090	---	---	---
0.725	0.6887489	-8.6155943	0.0	3.3796768	---	---	---
0.750	0.66143783	-8.4963506	0.0	3.5942337	---	---	---
0.775	0.6319612	-8.3612566	0.0	3.8292360	---	---	---
0.790	0.6131068	-8.2711758	0.0	3.9819677	---	---	---
0.795	0.6066094	-8.2394436	0.0	4.0351072	---	---	---
0.800	0.6000000	-8.2067933	0.0	4.0894508	---	---	---
0.805	0.5932748	-8.1731817	0.0	4.1450581	---	---	---
0.810	0.5864299	-8.1385621	0.0	4.2019932	---	---	---
0.815	0.5794610	-8.1028846	0.0064	4.2603252	0.880967	0.545588	-8.102934
0.820	0.5723635	-8.0660955	0.0524	4.3201286	0.797543	0.516311	-8.066596
0.825	0.5651327	-8.0281369	0.1116	4.3814839	0.744298	0.496028	-8.029578
0.830	0.5577634	-7.9889461	0.1665	4.4444785	0.702967	0.479341	-7.991848
0.835	0.5502499	-7.9484555	0.2140	4.5092074	0.668439	0.464724	-7.953367
0.840	0.5425864	-7.9065917	0.2551	4.5757737	0.638420	0.451485	-7.914095
0.845	0.5347663	-7.8632747	0.2912	4.6442903	0.611646	0.439241	-7.873990
0.850	0.5267827	-7.8184175	0.3232	4.7148806	0.587337	0.427750	-7.833003
^†Here, the units of angular momentum are as used in Paper I. In order to convert to units of <math>~L</math> as used in EFE (see, for example, Table I, in Chapter 5, §32), multiply by <math>~[2^2/(3\cdot 5^{10})]^{1/6} = 0.0717585</math>.
^‡<math>~E_\mathrm{plot}</math> is a normalized value of <math>~E_L</math> that has been used for plotting purposes. It's definition is: <math>E_\mathrm{plot} = 0.25*\biggl\{ \log_{10}\biggl[0.0001 + \frac{(E_L + \|E_\mathrm{Jac}\|)}{\|E_\mathrm{Jac}\|} \biggr] + 4\biggr\}</math>

PROPERTIES OF VARIOUS MACLAURIN SPHEROIDS

          eccenL       covera        omega2      ellChandra          L

            0.815  5.7946096D-01  3.7625539D-01  3.0571438D-01  4.2603252D+00
            0.820  5.7236352D-01  3.8058727D-01  3.1000578D-01  4.3201286D+00
            0.825  5.6513273D-01  3.8489420D-01  3.1440854D-01  4.3814839D+00
            0.830  5.5776339D-01  3.8917054D-01  3.1892894D-01  4.4444785D+00
            0.835  5.5024994D-01  3.9341001D-01  3.2357378D-01  4.5092074D+00
            0.840  5.4258640D-01  3.9760569D-01  3.2835048D-01  4.5757737D+00
            0.845  5.3476630D-01  4.0174986D-01  3.3326712D-01  4.6442903D+00
            0.850  5.2678269D-01  4.0583395D-01  3.3833257D-01  4.7148806D+00
            0.855  5.1862800D-01  4.0984835D-01  3.4355656D-01  4.7876802D+00
            0.860  5.1029403D-01  4.1378236D-01  3.4894980D-01  4.8628384D+00
            0.865  5.0177186D-01  4.1762394D-01  3.5452411D-01  4.9405200D+00
            0.870  4.9305172D-01  4.2135955D-01  3.6029264D-01  5.0209081D+00
            0.875  4.8412292D-01  4.2497391D-01  3.6626999D-01  5.1042063D+00
            0.880  4.7497368D-01  4.2844972D-01  3.7247248D-01  5.1906420D+00
            0.885  4.6559102D-01  4.3176729D-01  3.7891846D-01  5.2804708D+00
            0.890  4.5596052D-01  4.3490417D-01  3.8562861D-01  5.3739810D+00
            0.895  4.4606614D-01  4.3783459D-01  3.9262639D-01  5.4714996D+00
            0.900  4.3588989D-01  4.4052888D-01  3.9993856D-01  5.5733994D+00
            0.905  4.2541157D-01  4.4295266D-01  4.0759585D-01  5.6801086D+00
            0.910  4.1460825D-01  4.4506586D-01  4.1563375D-01  5.7921218D+00
            0.915  4.0345384D-01  4.4682147D-01  4.2409362D-01  5.9100155D+00
            0.920  3.9191836D-01  4.4816395D-01  4.3302405D-01  6.0344667D+00
            0.925  3.7996710D-01  4.4902713D-01  4.4248265D-01  6.1662784D+00
            0.930  3.6755952D-01  4.4933139D-01  4.5253852D-01  6.3064134D+00
            0.935  3.5464771D-01  4.4897998D-01  4.6327550D-01  6.4560401D+00
            0.940  3.4117444D-01  4.4785386D-01  4.7479681D-01  6.6165969D+00
            0.945  3.2707033D-01  4.4580450D-01  4.8723156D-01  6.7898831D+00
            0.950  3.1224990D-01  4.4264348D-01  5.0074442D-01  6.9781934D+00

PROPERTIES OF JACOBI ELLIPSOIDS THAT HAVE THE SAME ANGULAR MOMENTA (L) AS THE ABOVE MACLAURIN SPHEROIDS

      e      b/a       c/a        A1        A2        A3       omega2       a        L_C        L      energy

     0.815  0.880967  0.545588  0.474189  0.557354  0.968456  0.371826  1.983364  0.305714  4.260325 -8.102934
     0.820  0.797543  0.516311  0.441622  0.589410  0.968968  0.366634  2.088279  0.310006  4.320129 -8.066596
     0.825  0.744298  0.496028  0.419233  0.611283  0.969484  0.361394  2.165672  0.314409  4.381484 -8.029578
     0.830  0.702967  0.479341  0.400927  0.629069  0.970004  0.356104  2.232633  0.318929  4.444479 -7.991848
     0.835  0.668439  0.464724  0.384983  0.644489  0.970527  0.350761  2.293990  0.323574  4.509207 -7.953367
     0.840  0.638420  0.451485  0.370620  0.658325  0.971055  0.345362  2.351945  0.328350  4.575774 -7.914095
     0.845  0.611646  0.439241  0.357403  0.671009  0.971588  0.339905  2.407740  0.333267  4.644290 -7.873990
     0.850  0.587337  0.427750  0.345063  0.682812  0.972126  0.334386  2.462170  0.338333  4.714881 -7.833003
     0.855  0.564969  0.416851  0.333417  0.693915  0.972668  0.328802  2.515795  0.343557  4.787680 -7.791082
     0.860  0.544173  0.406427  0.322334  0.704450  0.973216  0.323150  2.569038  0.348950  4.862838 -7.748172
     0.865  0.524676  0.396390  0.311717  0.714514  0.973770  0.317425  2.622239  0.354524  4.940520 -7.704210
     0.870  0.506269  0.386673  0.301490  0.724181  0.974329  0.311624  2.675686  0.360293  5.020908 -7.659127
     0.875  0.488788  0.377221  0.291594  0.733511  0.974895  0.305741  2.729638  0.366270  5.104206 -7.612848
     0.880  0.472100  0.367988  0.281979  0.742554  0.975467  0.299772  2.784332  0.372472  5.190642 -7.565289
     0.885  0.456097  0.358937  0.272604  0.751348  0.976047  0.293710  2.840003  0.378918  5.280471 -7.516357
     0.890  0.440687  0.350033  0.263435  0.759930  0.976635  0.287549  2.896880  0.385629  5.373981 -7.465947
     0.895  0.425792  0.341249  0.254440  0.768329  0.977230  0.281283  2.955205  0.392626  5.471500 -7.413941
     0.900  0.411344  0.332556  0.245593  0.776572  0.977835  0.274902  3.015230  0.399939  5.573399 -7.360207
     0.905  0.397284  0.323929  0.236868  0.784683  0.978449  0.268398  3.077231  0.407596  5.680109 -7.304592
     0.910  0.383556  0.315345  0.228242  0.792685  0.979073  0.261762  3.141512  0.415634  5.792122 -7.246923
     0.915  0.370112  0.306780  0.219694  0.800598  0.979708  0.254980  3.208416  0.424094  5.910016 -7.187000
     0.920  0.356903  0.298209  0.211202  0.808442  0.980356  0.248041  3.278337  0.433024  6.034467 -7.124589
     0.925  0.343885  0.289608  0.202744  0.816240  0.981017  0.240927  3.351732  0.442483  6.166278 -7.059417
     0.930  0.331013  0.280950  0.194298  0.824010  0.981692  0.233621  3.429144  0.452539  6.306413 -6.991160
     0.935  0.318242  0.272206  0.185842  0.831774  0.982384  0.226102  3.511226  0.463276  6.456040 -6.919428
     0.940  0.305523  0.263344  0.177348  0.839558  0.983094  0.218342  3.598775  0.474797  6.616597 -6.843746
     0.945  0.292805  0.254325  0.168790  0.847385  0.983825  0.210310  3.692789  0.487232  6.789883 -6.763530
     0.950  0.280029  0.245105  0.160133  0.855288  0.984579  0.201966  3.794537  0.500744  6.978193 -6.678040

User:Tohline/ThreeDimensionalConfigurations/EFE Energies

Contents

Properties of Homogeneous Ellipsoids (2)

Sequence-Defining Dimensionless Parameters

Conserved Quantities

Aside: Chandra's Notation

Free Energy Surface(s)

Scope

Free Energy of Incompressible, Constant Mass Systems

Adopted Evolutionary Constraints

Conserve Only L

Animation

See Also

Navigation menu

Search